J. Service Science & Management, 2009, 2: 282-288
doi:10.4236/jssm.2009.24034 Published Online December 2009 (www.SciRP.org/journal/jssm)
Dalian High-Tech SMEs Growth Evaluation Based
on Catastrophe and Principal Component
Projection Method
Lin LI1, Pengfei ZHOU2, Zhenghe LI3
1School of Management, Dalian Jiaotong University, Dalian, China; 2School of Hydrolic Engineering, Dalian University of Tech-
nology, Dalian, China; 3Headmaster Office, Dalian Jiaotong University, Dalian, China.
Received July 21, 2009; revised September 9, 2009; accepted October 16, 2009.
ABSTRACT
In the course of rapid economic development, high-tech small and medium enterprises (SMEs) are gradually playing an
important role, which become important support to regional economic growth and science and technology development.
So SMEs growth becomes a universal problem. And how to evaluate the SMEs growth becomes an important step, es-
pecially to high-tech SMEs growth. In this paper, catastrophe theory and improved principal components projection
method are used and a mutation series of high-tech SMEs growth evaluation index system is built. Taking Dalian
high-tech SMEs as an example, high-tech SMEs growth is evaluated, which contributes to high-tech SMEs growth fore-
cast and government to formulate policies to support high-tech SMEs devel opment.
Keywords: Catastrophe Theory, Improved Principal Components projection Method, Enterprises Growth, High-tech
Small and Medium Enterprises (SMEs), Principal Component Projection Method
1. Introduction
In recent years, with the development of high technology,
enormous high-tech SMEs are build, but in which fast
growth small and medium high-tech enterprises only
account for about 5%[1]. Though the number is low, yet
they make a large contribution to job enlargement, wea-
lth increase, new start industry promotion, etc. What
factor influences the growth of high-tech SMEs？ That
becomes a problem concerned by industrialist, governor,
policymaker, banker (especially venture investor) and
scholar together. About 20 or 30 years ago, many schol-
ars have discussed this problem from different view-
points. These researches help understand the high-tech
SMEs growth process. But now the research achievement
can’t be used to design a model, which can preview the
growth potential of high-tech SMEs. In addition, like
hypotheses and methods used to different national small
and medium-sized enterprises, sometimes unlike empiri-
cal analysis outcome is got. The internationalization
process of high-tech SMEs is often different from that of
more mature industries [2].
Although there is no single agreed definition of
high-tech SMEs, there are generally characterized by
high-tech SMEs with advanced knowledge and capabili-
ties in technology, and educated workforce, and the abil-
ity to adapt quickly to fast changing environments. These
characteristics facilitate the internationalization of
high-tech SMEs, which have been known to act quickly
when window s of opportunity in fore ign markets present
themselves [3–7].
In China there are many vibrant SMEs, especially the
representation of high-tech SMEs, whose survival are the
basic form of expression in the contemporary conditions
of socialization of production and specialization, and is
an important part of the modern collaboration division of
labor system. They have become the most active ele-
ments to China's economy, which contribute much to
China's economic development, technology innovation,
and so on.
For the strength, stability and development of the
anti-risk capability, the curren t situation on the high-tech
SMEs growth is worrisome. High-tech SMEs growth is
fraught with difficulties and hardships, which make the
growth become a univ ersal problem [8]. How to evaluate
the high-tech SMEs growth is very important. At presen t,
domestic and foreign scholars focus on high-tech SMEs
growth study from two aspects [9–10]. That is, how to

Dalian High-Tech SMEs Growth Evaluation Based on Catastrophe and Principal Component Projection Method283
establish indicators and which methods are used to
evaluate. In this paper, catastrophe theory and improved
principal components projection method are used and a
mutation series of high-tech SMEs growth evaluation
index system is built. Taking Dalian high-tech SMEs as
an example, high-tech SMEs growth is evaluated, which
contributes to their growth forecast and government to
formulate policies to support SMEs developments.
2. High-Tech SMEs Evaluation Based on
Combination of Catastrophe Theory and
Principal Component Projection Method
2.1 High-Tech SMEs Evaluation Based on
Catastrophe Theory
Catastrophe theory is a mutation research (qualitative)
founded by French mathematician Majorelle Thom,
which is about the characteristics of system variables to
control the variables following the mathematical theory
and taken as ‘a revolution after mathematical calculus
[11]. Catastrophe theory is actually a multi-dimensional
fuzzy membership function.
To evaluate the complexity an d abstract goals, the sys-
tematic goals of multi-level contradictions are decom-
posed. And combining of fuzzy math with the Catastrophe
theory, the fuzzy membership functi on mutation is got.
Using normalization formula to a comprehensive
quantitative computing, the final as a parameter is got
[12]. The main advantage of the method is not used for
indicators of weight. However, it is considered the rela-
tive importance of various evaluation indicators, and
qualitative integrates and quantitative analysis, which
reduce the subjectivity and yet scientific, and is a rea-
sonable, simple and accurate calculation. So Catastrophe
theory is a worthwhile evaluation method. To a dynamic
system, the influence function of Catastrophe system is
()
f
x. According to Catastrophe theory, all of its critical
point congregates into balance-surface. Through the
equation to get the first derivative zero That is, ()
f
x

=0,
and the singular point can be got through solving ()
f
x
the second derivative, and got ()
f
x
 =0. ()
f
x
=0 and
()
f
x
 =0 eliminate x, the differences point set equation
of Catastrophe can b e got. Differences point set equation
shows that when all control variab les meet this equation ,
the system will mutate. Through the differences point set
equation that in decomposition form, a formula can be
got. By a formula, hanging different variables with dif-
ferent states are quality into the same state quality.
At present, the total Catastrophe system types is sev en,
the most common are three. That is, cusp catastrophe
system, dovetail catastrophe system and butterfly catas-
trophe system
The model of cusp catastrophe system is:
42
()
f
xxaxbx


The model of dovetail catastrophe system is:
532
11 1
() 532
f
xxaxbx cx


The model of butterfly catastrophe system is:
6432
111 1
() 6432
f
xxaxbxcx dx


()
f
x means a influence function of a state variable x
in a system. And the coefficient of x is a, b, c, d, which
represents the control variables of the state variables. If
an indicator only is decomposed into two sub-indexes,
the system can be seen cusp catastrophe system. If an
indicator is decomposed into three sub-indexes, the sys-
tem can be seen as dovetail catastrophe system. And if an
indicator is only decomposed into four sub-indexes, the
system can be seen butterfly catastrop he sy stem [13].
According to catastrophe theory, in a cusp catastr ophe
system, the differences point set equation of()
f
x

=0 and
()
f
x

 =0 can be got {a =2
6
x
，b =83
x
}. Then the
catastrophe fuzzy membership function formula can be
gotten.
1 1
3 2,
ab
x
axb

.
In the formula, a
x
means x corresponding to a, b
x
means x corresponding to b. Similarly, the formula of
dovetail catastrophe system is:
1 11
3 24
,,
abc
x
ax bxc


The butterfly catastrophe system is:
11 11
35 24
,,,
abcd
x
ax bxcxd


In essence, it is a multi-dimensional fuzzy membership
function, any state variables and control variables are
within the scope of 0-1. If the raw data is not the number
of 0-1, according to the principle ‘add, subtraction, mul-
tiplication and division’ the decision-making results un-
changed.
2.1.1 The Establishment of the Hierarchical Structure
Mode
Firstly, according to the purpose of evaluation, the eval-
uation purposes are decomposed into multi-level sub-
primary and secondary indicators. It just needs to know
the bottom original data, and the high-tech SMEs evalua-
tion data can be got.
It not only analyzes the business growth impacting in-
dicators and the classification indicators, but also needs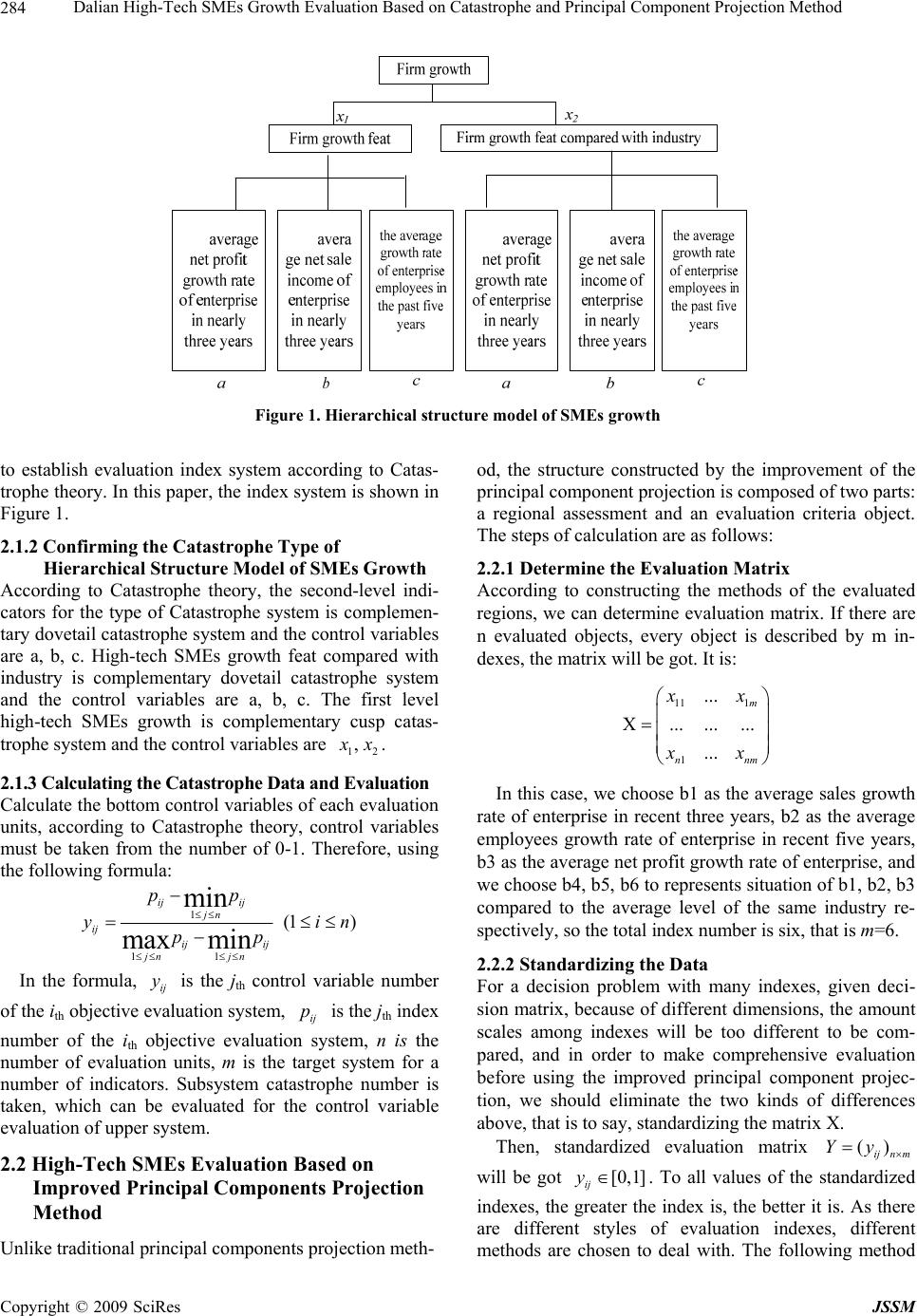

Dalian High-Tech SMEs Growth Evaluation Based on Catastrophe and Principal Component Projection Method 284
Figure 1. Hierarchical structure model of SMEs growth
to establish evaluation index system according to Catas-
trophe theory. In this paper, the index system is shown in
Figure 1.
2.1.2 Confirming the Catastrophe Type of
Hierarchical Structure Model of SMEs Growth
According to Catastrophe theory, the second-level indi-
cators for the type of Catastrophe system is complemen-
tary dovetail catastrophe system and the control variables
are a, b, c. High-tech SMEs growth feat compared with
industry is complementary dovetail catastrophe system
and the control variables are a, b, c. The first level
high-tech SMEs growth is complementary cusp catas-
trophe system and the control variables are 12
,
x
x.
2.1.3 Calculating the Catastrophe Data and Evaluation
Calculate the bottom control variables of each evaluation
units, according to Catastrophe theory, control variables
must be taken from the number of 0-1. Therefore, using
the following formula:
1
1 1
(1 )
min
max min
ij ij
jn
ij
ij ij
jn jn
pp
y
pp

 


in
m
In the formula, is the jth control variable number
of the ith objective evaluation system, is the jth index
number of the ith objective evaluation system, n is the
number of evaluation units, m is the target system for a
number of indicators. Subsystem catastrophe number is
taken, which can be evaluated for the control variable
evaluation of upper system.
ij
y
ij
p
2.2 High-Tech SMEs Evaluation Based on
Improved Principal Components Projection
Method
Unlike traditional principal components projection meth-
od, the structure constructed by the improvement of the
principal component projection is composed of two parts:
a regional assessment and an evaluation criteria object.
The steps of calculation are as follows:
2.2.1 Determine the Evaluation Matrix
According to constructing the methods of the evaluated
regions, we can determine evaluation matrix. If there are
n evaluated objects, every object is described by m in-
dexes, the matrix will be got. It is:
11 1
1
...
X... ......
...
m
nn
x
x
x
x





In this case, we choose b1 as the averag e sales growth
rate of enterprise in recent three years, b2 as the average
employees growth rate of enterprise in recent five years,
b3 as th e aver age net profit gro wth r ate of enterpri se, and
we choose b4, b5, b6 to represents situation o f b1, b2, b3
compared to the average level of the same industry re-
spectively, so the total index number is six, that is m=6.
2.2.2 Standardizing the Data
For a decision problem with many indexes, given deci-
sion matrix, because of different dimensions, the amount
scales among indexes will be too different to be com-
pared, and in order to make comprehensive evaluation
before using the improved principal component projec-
tion, we should eliminate the two kinds of differences
above, that is to say, standardizing the matrix X.
Then, standardized evaluation matrix ()
ijn m
Yy


will be got [0,1]
ij
y

. To all values of the standardized
indexes, the greater the index is, the better it is. As there
are different styles of evaluation indexes, different
methods are chosen to deal with. The following method

Dalian High-Tech SMEs Growth Evaluation Based on Catastrophe and Principal Component Projection Method285
is used to normalize the values of indexes regarding
evaluation matrix.
1
1 1
(1 )
min
max min
ij ij
jn
ij
ij ij
jn jn
xx
yi
xx

 


n

2.2.3 Determine the Index Weight and Building up
Empower-Decided Matrix
There are many methods to determine the index weight,
such as subjective method, objective method, empower
combination method. In this section, the method of level
analysis will be chosen to determine the index weight
ij

, then, weighting the standardized matrix according to
weight determined, and ordering that level vectors of
empower-decision matrix

() ,
ijn mijijij
Z
Zz zx 


Correspond to the evaluated objects, vertical vectors
correspond to the indexes are empowered.
The steps on confirming weight with improved prin-
cipal component projection method: Firstly, building up
the hierarchical structure in terms of the characteristics of
evaluation indexes. As the table 1 described, indexes of
upper levels corresponding to b1, b2 and b3 are repre-
sented by B1, indexes of upper levels corresponding to
b4, b5 and b6 are represented by B2. Then, comparison
and judge matrix are built up, which are used to judge
one to one. After the establishment of the target
level-structure, the relations of indexes between up and
down will be determined. As indexes are in the same
level, comparison between one and one according to 9
scales are made (Table 1).
We will get judge-matrix A={. }
ij
a
The values in A should meet the following conditions:
1,
1
0,  ii
ji
ijija
a
aa .
Table 1. The meaning of 9 scales
Number The importance of grades ij
a
1 i, j are equally important 1
2 i is slightly important 3
3 i is obviously important 5
4 i is strongly important 7
5 i is extremely important 9
6 i is slightly less important 1/3
7 i is obviously less important 1/5
8 i is strongly less i mportant 1/7
9 i is extremely less important 1/9
The results are as follows:
12
,
B
B
A=
12
1/21



123
,,bbb
AA==
456
,,bbb
121/2
1/2 1 1/3
231





Finally, the weight is determined.
1
i
in
i
i
M
M




In this equation,
1
n
n
ii
jj
M
a

.
The results are as follows:
121 2
,(,) (0.667,0.333) BBB B
W   ;
1234561 2 3
456
,,,, (,,)
(,,) (0.315,0.236,0.449)
bbbbbbb bb
bbb
WW






1234561 1121 13
24 25 26
,,,,, (, ,
,,
bbbbbbBb BbBb
Bb Bb Bb
W,
)





= (0.210, 0.157, 0.299, 0.106, 0.079,
0.149)
Judging the consistency ratio of the matrix,
CR= CI
RI

0.0045
0.52<0.1, which meets the require-
ments of consistency, and we will find that the largest
characteristic value.
max

=3.009
max 3.009 3
131
n
CI n
 


=0.0045.
2.2.4 Orthogonal Transformation of Indexes
Due to the large number of evaluation indexes, interre-
lated relationship among them will result in overlap
evaluation information, and it will interfere with the de-
termination of relatively important positions about in-
dexes. But if the values of the indexes are handled by
means of orthogonal transformation, the overlap infor-
mation among indexes will be filtered. Supposing that
W=Z'Z, we could calculate the result with Matlab, then,
characteristic values of matrix W will be got.
12 n

 .
Corresponding to unit characteristic, vectors are
, named A= (). If we order that
U=ZA, orthogonal decision-making matrix U=
12
,,,
n
aa a12 ,,,
n
aa a
()
ijnm
u

can be got, decision-making vector is U= (12
,,uu ,
n
u).

Dalian High-Tech SMEs Growth Evaluation Based on Catastrophe and Principal Component Projection Method 286
2.2.5 Constructing an Ideal Decision-Makct ing Obje
Treat nal
Then uniting wi
and Calculating t h e Values ab o ut Projec tion
ing each decision-making object as a dimensio
vector and constructing an ideal decision-making object
*
d=12 ( ,,...,)
m
ddd
*
dth
j
d=()
1
max ij
u
in, we could get the
result as follows:
*
*d
d *
0*22 2
12
... m
d
ddd d


represents the ideal decision-making object, calcu-
*
0
d
lating projection values of every evaluated vector about
ideal decision-making object:
1
*
022 2
12
...
m
jij
j
ii
m
du
Dud dd d

 


2.2.6 Ordering the Projection Values and
By thn values calculated with
Determining the Level
e magnitude of projectio
the equation above, we can judge the closing degree be-
tween every evaluated object and ideal object, projection
values 01
i
D, and to Di, the greater, the better. The
greater esents that the evaluated object value repri u is
better.
If we choose Di as comprehensive evaluation values of
th
it
th Evaluation of High-Tech SMEs
Thealuate the growth of the
using …to deal with the two kinds of results,
se
e n evaluated objects, according to the greater-better
principle, the final results of evaluation order could be got .
The lastly new k objects also have k projection results,
is that the values of the endpoints about evaluation
criteria intervals also have projection values, these k val-
ues form k-1 intervals, every interval corresponds to one
kind of evaluation criteria level. And n-k projection val-
ues of evaluated objects must fall into these k-1 intervals,
that is to say, projection values of evaluated objects fall
on projection values intervals of endpoints about evalua-
tion criteria intervals. Eventually, the interval fell on in-
dicates that evaluated objects are in the corresponding
situation.
2.3 Grow
Based on Combination
re are many methods to ev
enterprise, such as Catastrophe Theory, Principal Com-
ponent Projection Method. If we combine the evaluate
results of two methods just mentioned to play their own
strengths, it is clear that the effect of the evaluation will
be improved. And we combine the two kinds of results to
make the weighted evaluation. The basic processes are as
follows.
Firstly,
condly, using the formula 12 (1 )yy y

  to get the
results of combined evaluati: 1
y rep-
resents evaluation results which are dealt with Catastro-
phe Theory after normalization, 2
y represents evalu-
ation results which are dealt with ncipal Component
Projection Method after normalization,
on, in the formula
Pri

represents
the weighted factor. Due to a number of exrt advisory,
and the specific circumstances of data collection, we se-
lect 0.5 as the reasonable value of
pe

.
3. Empirical Analysis on Daanli High-Tech
3.1
All result from surveying enterprises.
we get the
O
oriented en-
te
Befnd reli-
SMEs Growth Evaluation
Source of the Data and Description of the
Data Structure
data of this study
The region where questionnaires are released is Dalian,
the person in charge of the business or senior managers
knowing about the enterprise very well are chose to fill
in the questionnaires. The number of the questionnaires
released is 167, among them, 112 recovered are valid,
recovery rate is 67.1%. The enterprises selected in this
time meet the needs of the research basically.
By analyzing the data of 112 valid sample,
wnership situation: Collective enterprises account for
2.66%, private enterprises account for 50.67%, stock
companies limited account for 5.33%, limited liability
companies account for 21.33%, joint-stock cooperative
enterprises accoun t for 1.33%, for eign-fund ed enterprises
account for 5.33%, equity joint venture companies ac-
count fo r 1 0.67%, o thers account for 2.67%.
Situation of the business type: Produ ction-
rprises account for 24%. Trade Enterprises account for
20%; Consulting, service-oriented en terprises account for
29.33%. Financial investment companies account for
1.33%. Outsourcing service accounts for 5.33%. Other
types account for 14.67%; others account for 5.33%.
3.2 The Effectiveness and Reliability of
High-Tech SMEs Growth Evaluation
ore data analysis, to test the effectiveness a
ability of data, data reliability test is needed. Cronbath

is a major test of the inherent reliability, through
which the

of six grow feat is got, that is 0.910. That
means the results of the questionnaire have high internal
consistency coefficient. And the performance of the
overall business growth correlation coefficient shows in
Table 2.
Before a large sample investigation, 20 high-tech
SMEs are randomly selected to be interviewed and pre-
investigated in Dalian high-tech park. Table 3 lists the
evaluation results of 20 SMEs based on Catastrophe the-

Dalian High-Tech SMEs Growth Evaluation Based on Catastrophe and Principal Component Projection Method 287
er Content Overall SMEs growth
performance coefficient
Table 2. Overall high-tech SMEs growth performance coef-
ficient
Numb
Y 1 Average growth rate of enterprise in nearly
net profit
three years .725
Y 2 n nearly three .713
Y 3 ise employees in the .781
Y 4 e compared .786
Y 5 ith .727
Y 6 es com-.773
Average net sale income of
enterprise i
years
The average growth rate of
enterpr
past five years
Average net profit growth
rate of enterpris
with other firm in a indus-
try in nearly three years
Average net sale income of
enterprise compared w
other firm in a industry in
nearly three years
The average growth rate of
enterprise employe
pared with other firm in a
industry in the past five
years
ory and principle component projection method. Ac-
cording to the 112 SMEs research results, the statistics
on high-tech SMEs growth are got in Table 4.
High-tech SMEs growth is a process that make enter-
prises resource value-added. To individual enterprise, it
always grows up with the scale expansion and corre-
sponding complexity increase companions. According to
the evaluation findings of SMEs growth, we take that the
enterprises score between 0.6-1.0 the enterprises as the
fast-growing enterprises score between 0.0-0.4. The en-
terprises are as the low-growing enterprises.
Evaluating on the high-tech SMEs growth based on
catastrophe theory and principle component projection
method, in addition to provide a new tool for the effec-
tive analysis and comprehensive evaluation of high-tech
SMEs, but also have the following practical value:
The government can judge the overall development
situation according to the evaluation results, which con-
tributes to develop targeted strategies, policies an d meas-
ures to promote high-tech SMEs development.
High-tech SMEs can have a comprehensive under-
standing of the overall development of other High-tech
SMEs, which help to judge enterprises in the industry
performance level. Analyzing on the firm growth and
potential sources can help to strengthen the growth of
enterprise management clearly in the specific direction.
Table 3. The growth evaluation of high-tech SMEs
Caprojection method tastrophe theory Principle component
Firm Number Before Normaliormalization Befon Combination
zation After Nore NormalizationAfter Normalizati
1 0.794 0.835 0.206 0.587 0.711
2 0.0.697 773 0.813 0.204 0.581
3 0.390 0.410 0.077 0.219 0.315
4 0.951 1.000 0.351 1.000 1.000
6 0.624 0.656 0.171 0.487 0.572
7 0.390 0.410 0.077 0.219 0.315
8 0.856 0.900 0.249 0.709 0.805
9 0.411 0.432 0.050 0.142 0.287
10 0.913 0.960 0.308 0.877 0.919
11 0.781 0.821 0.195 0.556 0.688
12 0.446 0.469 0.060 0.171 0.320
13 0.841 0.884 0.233 0.664 0.774
14 0.902 0.948 0.292 0.832 0.890
15 0.841 0.884 0.233 0.664 0.774
16 0.841 0.884 0.233 0.664 0.774
17 0.841 0.884 0.233 0.664 0.774
18 0.781 0.821 0.195 0.556 0.688
19 0.781 0.821 0.195 0.556 0.688
20 0.841 0.884 0.233 0.664 0.774
21 0.438 0.461 0.058 0.165 0.313

Dalian High-Tech SMEs Growth Evaluation Based on Catastrophe and Principal Component Projection Method 288
Table 4. Study on the eva results of SMEs
Growth 0.0-0.4 0.4-0.6 0.6-1.0
luation growth
evaluation Total
Sample Quantity 55 28 29 112
Sample percent 50.8% 23.5% 25.7% 100%
T reo esearch in-
titutions for more in-depth analysis of empirical and
brant SMEs, especially the
h-tech SMEs, whose survival are the
y National Social Science
iaoning Financial Research
[1] L Li, “High-tech firm growth mechanism and stra
based on knoical style),” T
esses in internationalizingMEsdical
styleoduction EconVol. 89, –378,
he evaluationsults contribute tsocial r
s
theoretical research. Study on the high-tech SMEs de-
velopment is still in the initial stage. All the available
research data, information is also scarce. Evaluation on
high-tech SMEs growth can provide a lot of empirical
analysis and data support, which help to deepen the
high-tech SMEs research.
4. Conclusions
any viIn China there are m
representative of hig
olo
basic form of expression in the contemporary conditions
of socialization of production and specialization, and is
an important part of the modern collaboration division of
labor system. Catastrophe theory is used and a mutation
series of high-tech SMEs growth evaluation index system
is built. Evidence shows the growth of Dalian high-tech
SMEs is relatively slow, nearly 50% of Dalian high-tech
SMEs grows slowly, which need to be regarded by the
government and Dalian high-tech SMEs. Some counter-
measures should be put forward by the industry or the
government to support the fast growth of Dalian
high-tech SMEs.
5. Acknowledgements
This research was supported b
Fund Project (09BJY055), L of M
Fund Project (08D008); Social Science Project of Dalian
(09DLSK193) and Dalian S&T Plan Fund Project
(2008D12ZC102.2009D11ZC099).
REFERENCES
tegy
ech-
gement, Vol. 24, No. 2,
pp. 235–262, 1998.
[13] X. H. Chen, “Evaluation of SME growth model based on
Catastrophe theory,” Financial Research, Vol. 11, pp.
13–17, 2006.
wledge alliance (Period
nology Management Research, Vol. 12, pp. 68–73, 2007.
[2] S. Saarenketo, K. Puumalainen, O. Kuivalainen and K.
Kylaheiko, “Dynamic knowledge-related learning proc-
high-tech S (Perio
),” Promics, pp363
2004.
[3] L. G. Weinzimmer, P. C. Nystrom, and S. J. Freeman,
“Measuring organizational growth: Issues, consequences
and guidelines (Periodical style),” Journal of Manage-
ment, Vol. 24, No. 2, pp 235–262, 1998.
[4] J. Baldwin and G. Gellatly, “Are there high-tech indus-
tries or only high-tech firms? Evidence from new tech-
nology-based firms,” Analytical Studies Branch, Mi-
cro-Economic Analysis Division, Statistics Canada (Re-
search report style),” pp. 24–32, 1998.
[5] M. Lindqvist, “Infant multinationals: Internationalisation
of small technology-based firms (Research report style),”
In D. Jones, &M Klofsten (Eds.), Techngy, innovation
and everprise: The European Experience, pp. 111–117,
2002.
[6] N. Karagozoglu and M. Lindell, “Internationalisation of
small and medium-sized technology-based firms: An ex-
ploratory study(Periodical style),” Journal of Small Busi-
ness Management, Vol. 36, No. 1, pp. 59–65, 1998.
[7] M. Lindell and N. Karagozolu, “Global strategies of US
and Scandinavian R&D intensive small and medium sized
companies (Periodical style),” European Management
Journal, Vol. 15, No. 1, pp. 92–101, 1997.
[8] D. Crick and M. Spence, “The internationalisation of
‘high performing’ UK high-tech SMEs: A study of
planned and unplanned strategies (Periodical style),” In-
ternational Business Review, Vol. 14, pp. 167–185, 2005.
[9] N. Coviello and H. Munro, “Growing the entrepreneurial
firm: Networking for international market develop-
ment(Periodical style),” European Journal of Marketing,
Vol. 29, No. 7, pp. 49–61, 1999.
[10] J. Bell, “The internationalization of small computer soft-
ware firms--A further challenge to ‘stage’ theories (Peri-
odical style),” European Journalarketing, Vol. 29,
No. 8, pp. 60–75, 1995.
[11] J. Wiklund, “Small firm growth and performance: Entre-
preneurship and beyond,” Jonkoping International Busi-
ness School, pp. 138–139, 1998.
[12] L. G. Weinzimmer, P. C. Nystrom, and S. J. Freeman,
“Measuring organizational growth: Issues, consequences
and guidelines”, Journal of Mana