J. Service Science & Management, 2009, 2: 265-269
doi:10.4236/jssm.2009.24031 Published Online December 2009 (www.SciRP.org/journal/jssm)
265
Periodicity of Cycle Time in a U-Shaped Production
Line with Heterogeneous Workers under Carousel
Allocation
Mikihiko Hiraiwa, Koichi Nakade
Department of Architecture, Civil Engineering and Industrial Management Engineering, Nagoya Institute of Technology, Japan.
Received September 3, 2009; revised October 12, 2009; accepted November 17, 2009.
ABSTRACT
A U-shaped production line with multiple machines and multiple workers is considered under carousel allocation in
which all workers take charge of all machines in the same order. Nakade and Ohno (2003) show that, when the proc-
essing, operation and walking times are constant, the overall cycle time, which is a time interval between successive
outputs of finished goods, is the greatest value of the maximal sum of the processing and operation times among ma-
chines and the time required for a worker to operate and walk around the production line without waiting for process-
ing divided by the number of workers. In this paper, it is considered that operation times at each machine may be dif-
ferent between workers. If processing time is short, it is expected that the overall cycle time will be equal to the time for
a worker to operate and walk arou nd the line divided by the number of workers. However, under some specified cases,
the overall cycle time is longer than that of this time, and the overall cycle time changes periodically. From numerical
examples, it is shown that the order of arrivals of workers at machine 1 affects the overall cycle time. We give some
properties on the periodicity of cycle times and discuss about cycle times.
Keywords: U-Shaped Production Line, Carousel Allocation, Operation Times, Cycle Time, Periodicity of Cycle Times
1. Introduction
In these days, U-shaped layout is commonly used in
many production lines. In a U-shaped production line,
there are two types of worker allocations, which are
separate type and carousel type. In the separate allocation,
each worker deals with a unique set of machines. In the
carousel allocation, workers deal with all machin es in the
same route. In this paper, the carousel allocation is con-
sidered. An advantage of U-shaped layout is that it is
easy to adjust the throughput of finished products from
the last machine for fluctuation of demand by changing
the number of workers [1,2].
Nakade and Ohno [3] have considered a U-shaped
production line with multiple multi-function workers,
and show the overall cycle time which is a time interval
between successive outputs of finished products. When
the processing, op eration, and walking times are constan t,
the overall cycle time is the greatest value of the maxi-
mal sum of the processing and operation times among
machines and the time required for a worker to operate
and walk around the production line without waiting for
processing divided by the number of workers. They as-
sume that operation times at each machine are the same
among workers.
Recently, many temp staffs are employed as workers
and they are committed into production lines because of
cost reduction. For example, many foreigners and part
time workers are employed for a short span. Therefore it
becomes difficult to maintain the workers well-skilled in
the long time, so the worker’s skills remain mutually
different.
Nakade and Nishiwaki [4] have considered a U-
shaped production line with multiple heterogeneous
multi-function workers and propose an algorithm for
computing an optimal allocation of workers to machines
which minimizes the overall cycle time.
The formula of cycle time derived in Nakade and Ni-
shiwaki [4] is simple and understandable, where it is as-
sumed that different workers are assigned to the different
machines. In the other allocation scheme, all workers
have operations at all machines and as a carousel workers
go around machines. We say this scheme carousel allo-
cation.

Periodicity of Cycle Time in a U-Shaped Production Line with Heterogeneous Workers under Carousel Allocation 266
In this paper we consider the case that operation ti mes
at each machine are different among workers under car-
ousel allocation. In this case, if the processing times are
very small, then it may be guessed that the overall cycle
time is equal to the greatest value of times required for
each worker to operate and walk around the line divided
by the number of workers. However, under some speci-
fied cases, the overall cycle time is larger than this time,
and the overall cycle time changes periodically. We dis-
cuss the periodicity of cycle times and observe some
properties on cycle times.
In the next section we describe a U-shaped production
line. In Section 3 we show that the overall cycle time
changes periodically by numerical examples and discuss
the periodicity of cycle times. In Section 4 we conclude
and discuss future research.
2. A U-Shaped Production Line
We consider a U-shaped production line with
K
ma-
chines and
J
workers, which is shown in Figure 1. It is
assumed that
J
K. There is enough raw material in
front of machine 1. The material is processed at ma-
chines 1 to
K
sequentially, and customers receives
finished products from machine
K
. Workers deal with
all machines in the same route and operate an item at
each machine. Operation times at each machine may be
different among workers, and the operation time of
worker at machine is denoted by
jk,
j
k
s
, which is
deterministic. Worker starts his first operation at
machine
j
j
k and visits machines
sequentially. The deterministic walking time
from machine k to
1,
jj
kk2,,,K
1, 2,
1k


K
11
1, 2k, , is denoted
by , where it is assumed that
,kk
r1
K

k
i
. The proc-
essing time at machine is denoted by , which is
deterministic. When a worker visits a machine, if the
preceding worker is operating at the machine or the
k
Figure 1. U-shaped production line
machine is processing the preceding item, then the
worker must wait for the completion of operating or
processing. The overall cycle time is defined as a time
interval between successive outputs of finished products.
We also define the cycle time of worker as the time
interval between the successive arrivals of worker at
machine . It is noted that the overall cycle time is the
cycle time divided by the number of workers. The first
cycle time of worker is the time interval between a
start of first operation and the first arrival of worker
at machine . The th
j
j
1
j
j
1n


2n cycle time of worker
is the time interval between the th and th
arrivals for worker at machine 1.
j

n

1n
j
3. Cycle Time
3.1 Case of the Same Operation Times among
Workers
In the case that operating times at each machine are the
same among workers, from Nakade and Ohno (2003),
when processing, operation and walking times are con-
stant, the overall cycle time is expressed as
overall
C

,1
ˆˆ
1
max max,,
overall
ckkk
kK kK kK
Csis
J

kk
r







 




 (1)
where k
s
denotes the operation time at machine
and
k


ˆ2, ,1,
K
K. The overall cycle time is the great-
est value of the maximal sum of the processing and op-
eration times among machines and the time required for a
worker to operate and walk around the production line
without waiting for processing divided by the number of
workers.
3.2 Case of Different Operation Times among
Workers
We consider the case that operating times at each ma-
chine may be different among workers. In this case, from
Equation (1), the overall cycle time may be gue-
ssed as
overall
C


,
ˆ1,2, ,
,,1
1,2, ,ˆˆ
1
max max,
1max .
overall jk k
kK jJ
jk kk
jJ
kK kK
Cs
J
sr
J












i










(2)
In what follows, we consider the cycle time instead of
the overall cycle time. Since the overall cycle time is the
cycle time divided by the number of workers, the cycle
time may be guessed as C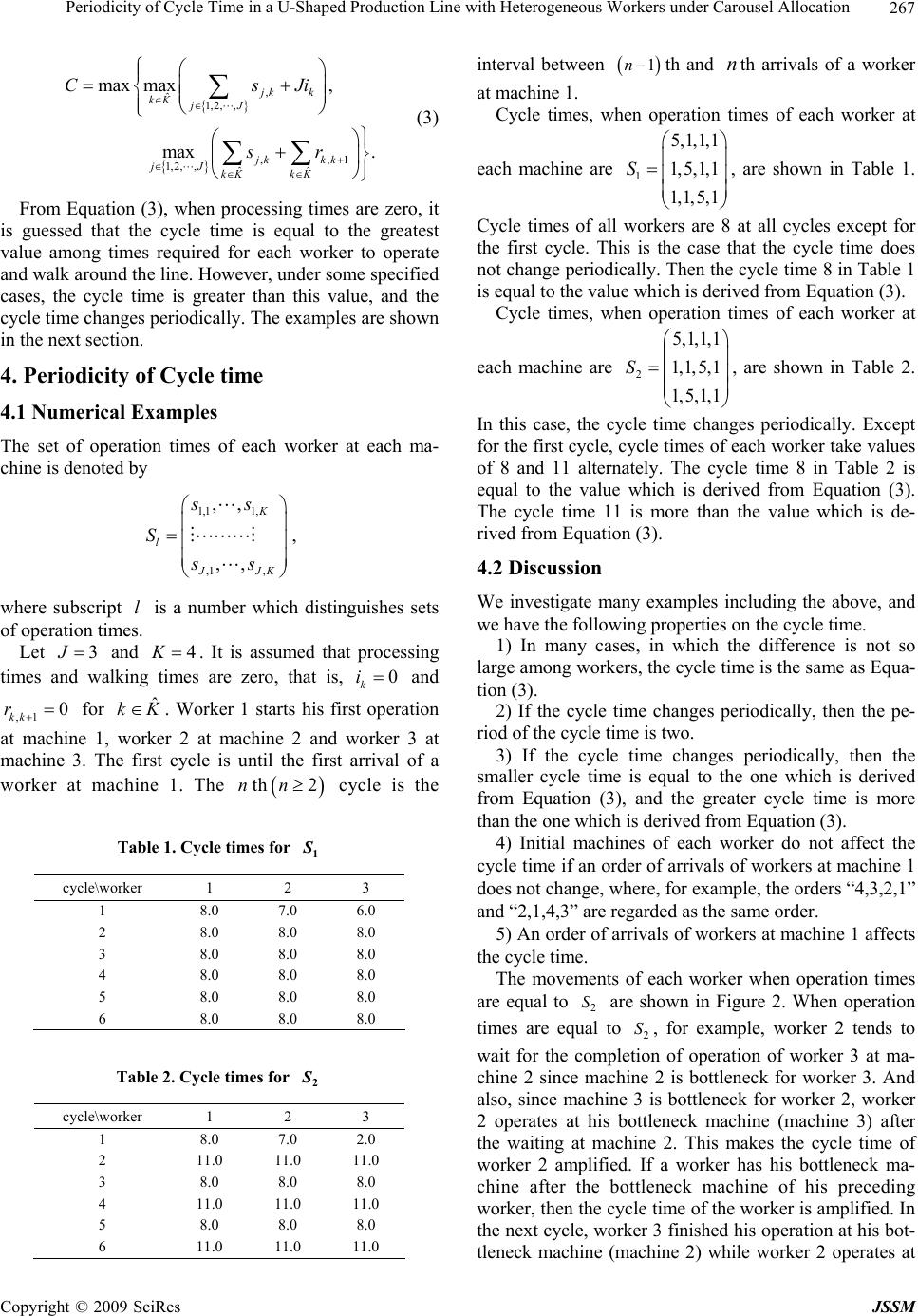

Periodicity of Cycle Time in a U-Shaped Production Line with Heterogeneous Workers under Carousel Allocation267
Ji


,
ˆ1,2, ,
,,1
1,2, ,ˆˆ
max max,
max .
jk k
kK jJ
jk kk
jJ
kK kK
Cs
sr






















(3)
From Equation (3), when processing times are zero, it
is guessed that the cycle time is equal to the greatest
value among times required for each worker to operate
and walk around th e lin e . However, under some specified
cases, the cycle time is greater than this value, and the
cycle time changes periodically. The examples are shown
in the next section.
4. Periodicity of Cycle time
4.1 Numerical Examples
The set of operation times of each worker at each ma-
chine is denoted by
1,1 1,
,1 ,
,,
,,
K
l
JJK
ss
S
ss








,
where subscript is a number which distinguishes sets
of operation times.
l
Let and
3J4
K
. It is assumed that processing
times and walking times are zero, that is, 0
k
i

and
for . Worker 1 starts his first operation
at machine 1, worker 2 at machine 2 and worker 3 at
machine 3. The first cycle is until the first arrival of a
worker at machine 1. The th cycle is the
,1kk
r0ˆ Kk
n

2n

Table 1. Cycle times for 1
S
cycle\worker 1 2 3
1 8.0 7.0 6.0
2 8.0 8.0 8.0
3 8.0 8.0 8.0
4 8.0 8.0 8.0
5 8.0 8.0 8.0
6 8.0 8.0 8.0
Table 2. Cycle times for 2
S
cycle\worker 1 2 3
1 8.0 7.0 2.0
2 11.0 11.0 11.0
3 8.0 8.0 8.0
4 11.0 11.0 11.0
5 8.0 8.0 8.0
6 11.0 11.0 11.0
interval between


1n

th and th arrivals of a worker
at machine 1.
n
Cycle times, when operation times of each worker at
each machine are , are shown in Table 1.
Cycle times of all workers are 8 at all cycles except for
the first cycle. This is the case that the cycle time does
not change periodically. Then the cycle time 8 in Table 1
is equal to the value which is derived from Equation (3).
1
5,1,1,1
1, 5,1,1
1,1,5,1
S









Cycle times, when operation times of each worker at
each machine are , are shown in Table 2.
In this case, the cycle time changes periodically. Except
for the first cycle, cycle times of each worker take values
of 8 and 11 alternately. The cycle time 8 in Table 2 is
equal to the value which is derived from Equation (3).
The cycle time 11 is more than the value which is de-
rived from Equation (3).
2
5,1,1,1
1,1,5,1
1, 5,1,1
S





4.2 Discussion
We investigate many examples including the above, and
we have the following properties on the cycle time.
1) In many cases, in which the difference is not so
large among workers, the cycle time is the same as Equa-
tion (3).
2) If the cycle time changes periodically, then the pe-
riod of the cycle time is two.
3) If the cycle time changes periodically, then the
smaller cycle time is equal to the one which is derived
from Equation (3), and the greater cycle time is more
than the one which is derived from Equation (3).
4) Initial machines of each worker do not affect the
cycle time if an order of arrivals of workers at machine 1
does not change, where, for example, the orders “4,3,2,1”
and “2,1,4,3” are regarded as the same order.
5) An order of arrivals of workers at machine 1 affects
the cycle time.
The movements of each worker when operation times
are equal to are shown in Figure 2. When operation
times are equal to , for example, worker 2 tends to
wait for the completion of operation of worker 3 at ma-
chine 2 since machine 2 is bottleneck for worker 3. And
also, since machine 3 is bottleneck for worker 2, worker
2 operates at his bottleneck machine (machine 3) after
the waiting at machine 2. This makes the cycle time of
worker 2 amplified. If a worker has his bottleneck ma-
chine after the bottleneck machine of his preceding
worker, then the cycle time of the worker is amplified. In
the next cycle, worker 3 finished his operation at his bot-
tleneck machine (machine 2) while worker 2 operates at
2
S
2
S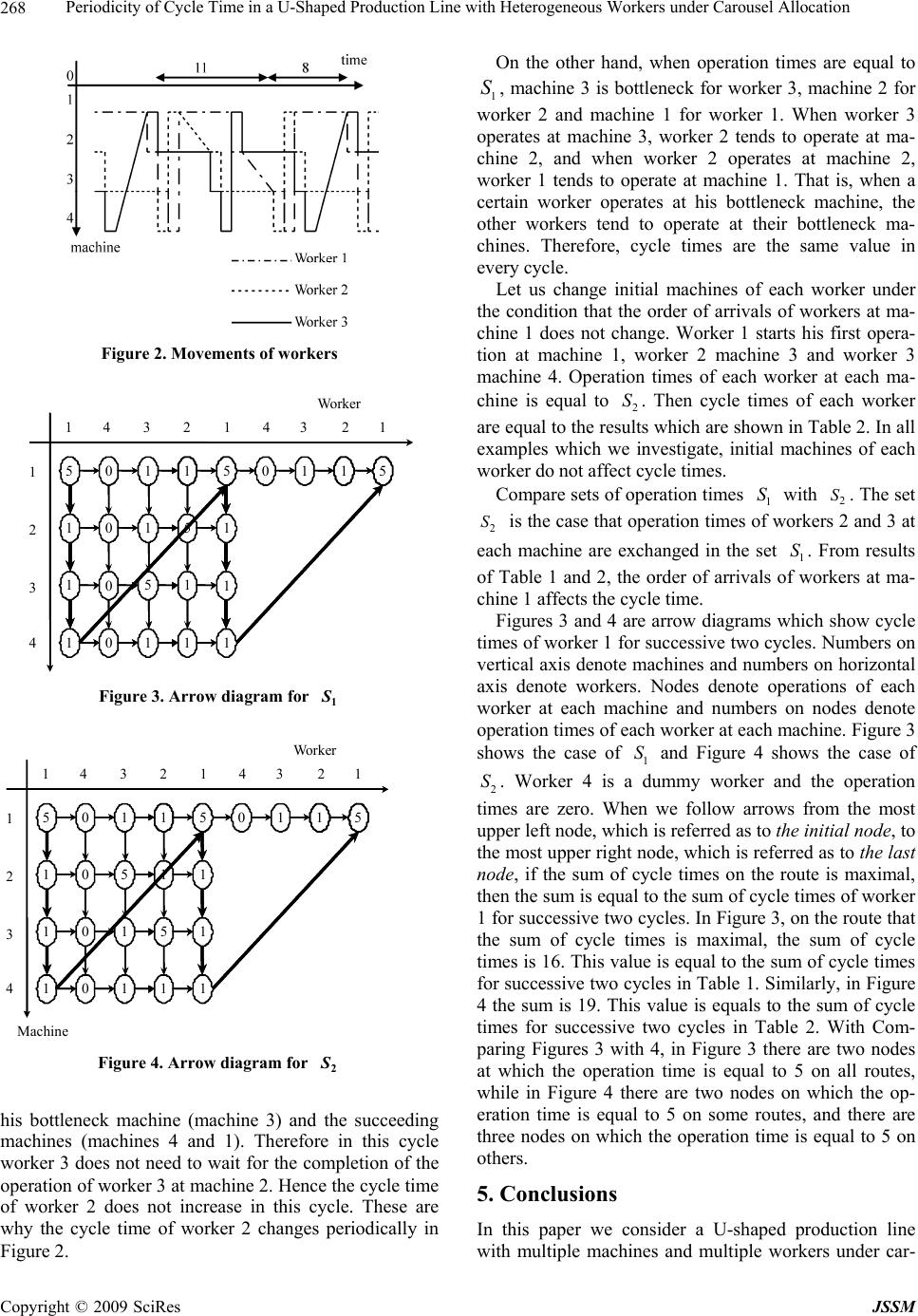

Periodicity of Cycle Time in a U-Shaped Production Line with Heterogeneous Workers under Carousel Allocation 268
Figure 2. Movements of workers
14 32 14 3 2
5011 501
1
1
2
3
4
1 5
1
1
1
0
0
0
1
5
1
5
1
1
1
1
1
Worker
Figure 3. Arrow diagram for 1
S
14 32 1 43 2
5011 501
1
1
2
3
4
1 5
1
1
1
0
0
0
1
11
1
1
1
Machine
5
1 5
Worker
Figure 4. Arrow diagram for 2
S
his bottleneck machine (machine 3) and the succeeding
machines (machines 4 and 1). Therefore in this cycle
worker 3 does not need to wait for the completion of the
operation of worker 3 at machine 2. Hence the cycle time
of worker 2 does not increase in this cycle. These are
why the cycle time of worker 2 changes periodically in
Figure 2.
On the other hand, when operation times are equal to
, machine 3 is bottleneck for worker 3, machine 2 for
worker 2 and machine 1 for worker 1. When worker 3
operates at machine 3, worker 2 tends to operate at ma-
chine 2, and when worker 2 operates at machine 2,
worker 1 tends to operate at machine 1. That is, when a
certain worker operates at his bottleneck machine, the
other workers tend to operate at their bottleneck ma-
chines. Therefore, cycle times are the same value in
every cycle.
1
S
Let us change initial machines of each worker under
the condition that the order of arrivals of workers at ma-
chine 1 does not change. Worker 1 starts his first opera-
tion at machine 1, worker 2 machine 3 and worker 3
machine 4. Operation times of each worker at each ma-
chine is equal to . Then cycle times of each worker
are equal to the results which are shown in Table 2. In all
examples which we investigate, initial machines of each
worker do not affect cycle times.
2
S
Compare sets of operation times with . The set
is the case that operation times of workers 2 and 3 at
each machine are exchanged in the set . From results
of Table 1 and 2, the order of arrivals of workers at ma-
chine 1 affects the cycle time.
1
S2
S
2
S
1
S
Figures 3 and 4 are arrow diagrams which show cycle
times of worker 1 for successive two cycles. Numbers on
vertical axis denote machines and numbers on horizontal
axis denote workers. Nodes denote operations of each
worker at each machine and numbers on nodes denote
operation times of each worker at each machine. Figure 3
shows the case of and Figure 4 shows the case of
. Worker 4 is a dummy worker and the operation
times are zero. When we follow arrows from the most
upper left node, which is referred as to the initial node, to
the most upper right node, which is referred as to the last
node, if the sum of cycle times on the route is maximal,
then the sum is equal to the sum of cycle times of worker
1 for successive two cycles. In Figure 3, on the route that
the sum of cycle times is maximal, the sum of cycle
times is 16. This value is equal to the sum of cycle times
for successive two cycles in Table 1. Similarly, in Figure
4 the sum is 19. This value is equals to the sum of cycle
times for successive two cycles in Table 2. With Com-
paring Figures 3 with 4, in Figure 3 there are two nodes
at which the operation time is equal to 5 on all routes,
while in Figure 4 there are two nodes on which the op-
eration time is equal to 5 on some routes, and there are
three nodes on which the operation time is equal to 5 on
others.
1
S
2
S
5. Conclusions
In this paper we consider a U-shaped production line
with multiple machines and multiple workers under car-

Periodicity of Cycle Time in a U-Shaped Production Line with Heterogeneous Workers under Carousel Allocation 269
ousel allocation. In the case that operation times at each
machine are different among workers, we investigate the
cycle time. Under some specific cases, the cycle time
changes periodically. We have some insights into the
periodicity of the cycle time and discuss cycle times. For
future research the condition that the cycle time changes
periodically is derived.
REFERENCES
[1] Y. Monden, “Toyota production system,” An integrated
Approach, 3rd edition, Engineering and Management
Press, Georgia, 1998.
[2] G. J. Miltenburg, “One-piece flow manufacturing on
u-shaped production lines: A tutorial,” IIE Transactions,
Vol. 33, pp. 303–321, 2001.
[3] K. Nakade and K. Ohno, “Separate and carousel type
allocations of workers in a U-shaped production line,”
European Journal of Operational Research, Vol. 145, pp.
403–424, 2003.
[4] K. Nakade and R. Nishiwaki, “Optimal allocation of het-
erogeneous workers in a U-shaped Production Line,”
Computers & Industrial Engineering, Vol. 54, pp. 432–
440, 2008.