Journal of Quantum Informatio n Science, 2011, 1, 142-148
doi:10.4236/jqis.2011.13020 Published Online December 2011 (http://www.SciRP.org/journal/jqis)
Circular Scale of Time Applied in Classifying the
Quantum-Mechanical Energy Terms Entering the
Framework of the Schrödinger Perturbation Theory
S. Olszewski
Institute of Physical Chemistry, Polish Academy of Sciences, Warsaw, Poland
Received September 6, 2011; revised Octobe r 20, 2011; accepted November 3, 2011
Abstract
The paper applies a one-to-one correspondence which exists between individual Schrödinger perturbation
terms and the diagrams obtained on a circular scale of time to whole sets of the Schrödinger terms belonging
to a definite perturbation order. In effect the diagram properties allowed us to derive the recurrence formulae
giving the number of higher perturbative terms from the number of lower order terms. This recurrence for-
malism is based on a complementary property that any perturbation order
N
can be composed of two posi-
tive integer components a
N
, b
N
combined into
N
in all possible ways. Another result concerns the de-
generacy of the perturbative terms. This degeneracy is shown to be only twofold and the terms having it are
easily detectable on the basis of a circular scale. An analysis of this type demonstrates that the degeneracy of
the perturbative terms does not exist for very low perturbative orders. But when the perturbative order ex-
ceeds five, the number of degenerate terms predominates heavily over that of nondegenerate terms.
Keywords: Circular Scale of Time, Quantum-Mechanical Energy Terms, Complementary Relations,
Schrödinger’s Perturbation Theory
1. Introduction
As soon as quantum mechanics in its wave-mechanical
form was developed, the perturbation problem of the
eigenenergies and eigenstates came into consideration
[1]. A necessity of the perturbation theory was dictated
by the fact that only very few systems on the atomic
level could be examined in an exact quantum-mechanical
way. In an overwhelming part of physical problems, the
approximate methods had to be developed, and one of
them was the Rayleigh-Schrödinger (RS) perturbation
framework [2]. Rayleigh’s name was involved because a
similar perturbation method was applied by that author in
a treatment of the acoustic waves; see [2]. More devel-
oped approaches to the perturbation theory than [2] are in
[3-8].
In fact, perturbation theory is a complicated formalism
already at the level of a one-particle (one-electron) sys-
tem that occupies a non-degenerate unperturbed state [1,
2]. Essentially the method is based on the calculations of
the matrix elements between the unperturbed wave func-
tions
p, q, (1)
and the potential function V which represents a per-
turbation of an originally unperturbed energy Hamilto-
nian. If any matrix element is considered as a result of a
single particle scattering on the potential , the number
of the RS terms required to calculate the perturbation
energy increases dramatically, especially when many
different scatterings on V are taken into account. In
principle, a full RS series is obtained when the number of
the different types of scatterings on is allowed to go
to infinity. In practice, however, we consider only the
perturbations of some finite order , where de-
fines the number of factors of the type
V
V
N N
pq
UpVq (2)
entering a product of terms (2) forming a single pertur-
bation term. In general, any of these factors is based on
the eigenstates of the kind of (1) of an unperturbed Ham-
iltonian.

143 S. OLSZEWSKI
The RS formalism of the perturbation of a non-de-
generate state strictly limits the number
N
S of kinds of
perturbative terms characteristic for a given . This
number is represented by
N


22
!1
N
N
SNN


!
!
. (3)
Different values of
N
S for different numbers are
listed in Table 1.
N
The derivation of (3) is based on a complicated com-
binatorial formalism [9,10]. But, in many cases, we look
for a recurrence formulae that allows us to calculate
N
S
from the lower-order terms
1232 1
,,,, ,
NN
SSSS S

. (4)
The aim of the present paper is to provide such for-
mulae. This treatment is based on a circular time scale.
The idea of introducing the time variable as a tool to
place the perturbative terms in order according to the size
of came from Feynman [4,11]. The scattering events
with the perturbation are arranged along a straight-
linear time scale and all diagrams connecting a given
number of events should be taken into account. However,
the number
NV

1!
N
PN

(5)
of Feynman diagrams necessary to calculate the RS
terms for a given exceeds
N
N
S; see Table 1.
Consequently, the energy contributions from the Feyn-
man diagrams should be appropriately combined to give
the proper contributions coming from the individual RS
terms. The necessity of such a combination of diagrams
makes the Feynman formalism especially cumbersome
when is large. For example, for we have
N20N
20 1 767 263 190S (6)
Table 1. The number N
S
of the RS perturbative terms
[see (3)] and the number N
P
of the Feynman diagrams
[see (5)] of a given perturbative order .
N
N N
S N P
1 1 1
2 1 1
3 2 2
4 5 6
5 14 24
6 42 120
7 132 720
8 429 5040
9 1430 40320
10 4862 362880
and


17
20 101 !1.21610P . (7)
Consequently, a combination of
N
P energy terms
into
N
S terms becomes an uneasy task.
But the difficulties of the Feynman formalism can be
avoided when its straight-linear time scale is replaced by
a circular time scale. In the latter case, a one-to-one cor-
respondence exists between the diagrams obtained on the
circular scale and the RS perturbation terms [12-16]. In
effect, any component term entering the set of
N
S
terms corresponds to a separate diagram contributing a
definite formula of the RS perturbation energy of order
. This result is attained by applying an appropriate
contraction rule for the scattering events on the circular
scale. Any contraction prescribed by this rule is different,
and the whole number of diagrams obtained in this way
becomes exactly equal to
N
N
SN. Moreover, an analysis of
all contractions for a given gives precisely the same
energy terms, as they are provided by the RS theory.
In Section 3 we present the recurrence formulae for
N
S attained on the basis of a graphical analysis applied
in [12-16]. In fact, these formulae represent the comple-
mentary relations for
N
S obtained on the basis of
N
a
and
S
N
b having a, b. The property of com-
plementarity becomes evident if we note that any com-
ponent of
SN NN
N
S is a product of
N
a
S and
N
b
S for which
there is satisfied the relation:
ab
NNN (8)
for any pair of integer numbers and b. The
changes of
a
N N
N
S due to the change of are reported in
Section 3.
N
Another advantage of the circular scale is its use in
detecting the degeneracy of the Schrödinger perturbation
terms. In fact, any diagram representing the perturbation
term is either symmetrical for itself, or asymmetrical
with respect to another diagram; see Section 4. This
property provides us with a simple rule that there is no
degeneracy of energy for a symmetrical diagram, but a
twofold degeneracy is connected with any pair of asym-
metrical diagrams. No other kind of degeneracy is ob-
tained on the basis of the symmetry analysis of the dia-
grams. Sections 4 and 5 demonstrate that the twofold
degeneracy due to a circular character of the time scale
holds for the most part of the perturbative energy terms
belonging to a given on condition .
N5N
2. Recurrence Formulae for N
S
and Their
Complementary Character
In the first step, we point out that the recurrence formal-
ism for
N
S can be obtained without an analysis of all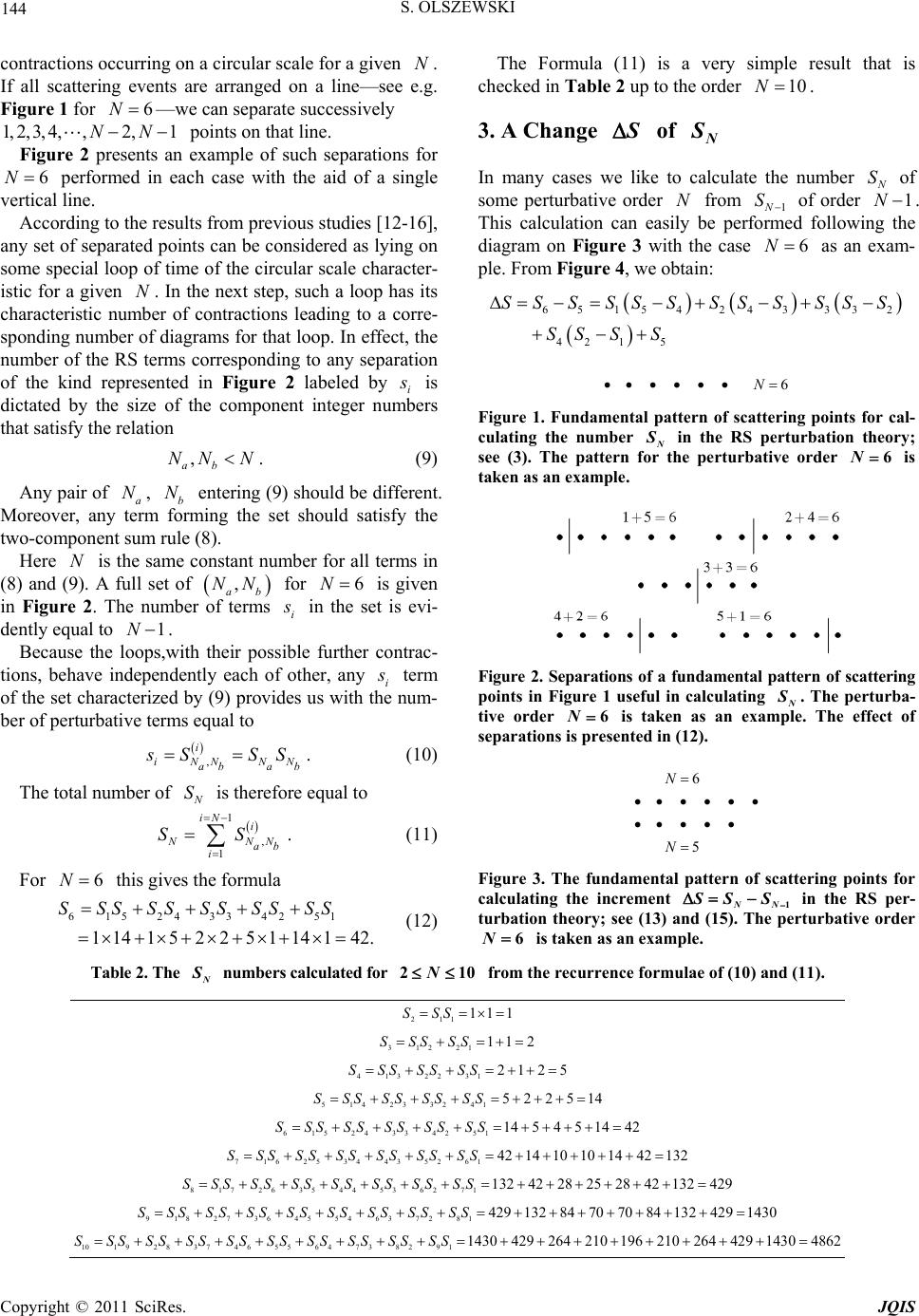

S. OLSZEWSKI
144
contractions occurring on a circular scale for a given .
If all scattering events are arranged on a line—see e.g.
Figure 1 for —we can separate successively
points on that line.
N
6N
2,N
N
1,2,3, 4,,1N
6N
The Formula (11) is a very simple result that is
checked in Table 2 up to the order . 10N
3. A Change

S
of N
S
Figure 2 presents an example of such separations for
performed in each case with the aid of a single
vertical line.
In many cases we like to calculate the number
N
SN of
some perturbative order from 1N of order NS 1

.
This calculation can easily be performed following the
diagram on Figure 3 with the case as an exam-
ple. From Figure 4, we obtain:
6N
According to the results from previous studies [12-16],
any set of separated points can be considered as lying on
some special loop of time of the circular scale character-
istic for a given . In the next step, such a loop has its
characteristic number of contractions leading to a corre-
sponding number of diagrams for that loop. In effect, the
number of the RS terms corresponding to any separation
of the kind represented in Figure 2 labeled by i
s
is
dictated by the size of the component integer numbers
that satisfy the relation

 



65 154243332
42 15
SSSSS SSSSSS S
SS SS
  

6N

 
Figure 1. Fundamental pattern of scattering points for cal-
culating the number N
S
in the RS perturbation theory;
see (3). The pattern for the perturbative order 6N

is
taken as an example.
,
ab
NN N. (9)
Any pair of a, b entering (9) should be different.
Moreover, any term forming the set should satisfy the
two-component sum rule (8).
N N
N Here is the same constant number for all terms in
(8) and (9). A full set of for is given
in Figure 2. The number of terms

,
ab
NN 6N
i
s
in the set is evi-
dently equal to .
1N
Because the loops,with their possible further contrac-
tions, behave independently each of other, any i
s
term
of the set characterized by (9) provides us with the num-
ber of perturbative terms equal to
Figure 2. Separations of a fundamental pattern of scattering
points in Figure 1 useful in calculating N
S
. The perturba-
tive order 6N

is taken as an example. The effect of
separations is presented in (12).

,
i
i
NN ab
ab
s
NN
SSS . (10)
6
5
N
N



   

The total number of
N
S is therefore equal to

1
,
1
iN i
N
N
N
ab
i
SS


. (11)
Figure 3. The fundamental pattern of scattering points for
calculating the increment 1NN
S
SS

  in the RS per-
turbation theory; see (13) and (15). The perturbative order
6N

is taken as an example.
For this gives the formula
6
15
14 1
SS
 
N
6 2
15
S S

4334251
225 114142.
SSSSSSS
   (12)
Table 2. The N
S
numbers calculated for 210N

 from the recurrence formulae of (10) and (11).
211
11 1SSS


31
SSS
221
11 2SS


41 231
212 5SSSSSS
32
S


5142 3241
522514SSSS SSS 
3
SS
34
SS
35
S
36
S
7
SSS
82
S
615243 251
14 54 51442SSSSSS SSS 
71625344 261
42 14 10 10 1442132SSSSSSSSS SSS 
8172635445271
132 42 28 25 28 42132429SSSSSSSSSSS SSS  
9
S
19 2
SSS 
182736455463 281
429 13284707084 1324291430SSSSSSSSSSS SS 
10837 46 55 6473911430 429 264 210196 210 264 42914304862SSSSSSSSSSSSS SS 

S. OLSZEWSKI 145
The result of (14) agrees with the Huby-Tong formula
of
Figure 4. Separations of a fundamental pattern of scattering
points in Figure 3 useful in calculation of 1NN
S
SS

 
for 6N; see (13).



1145 1522 21 51114
28.


(13)
The difference (13) added to gives:
(14)
Calculations similar to those presented
pe
5
S
65 14 2842SS S. 
in (13) and (14)
rformed for N not exceeding 10 are presented in
Table 3. The dirences other than (15), such as those
between
ffe
N
S and 2N S, or
N
S and 3N S, can be cal-
culated onhe samoting the dince between te foasffere
N
S and 1N S.
(3); cf. here the data in Table 1. A general formula for
S

is


 
11 12
22 3221
.
NN N
NN NN
SS SSSS
SSSSS SS
N
1


 

(15)
. Symmetry of the Time Scale and rms
ular
 
4Degeneracy of the Perturbation Te
topological symmetry of energy diagrams on a circA
scale can be easily demonstrated by an example.
Suppose we have the energy terms of the perturbative
order 6N

. In Figure 5 we present four diagrams of
6N

; a ful
D
l set of the 642S diagrams is given in
[1iagram (a), also calain time loop, has no
contractions; it is symmetrical with respect to the line
joining the beginning-end point of the loop with point 3,
which is the most distant point from
2]. led the m

.
The line divides the loop into halves. Diagram (d) has
a similar symmetry that is characteristic by the time con-
traction 2:4. On the other hand, diagrams (b) and (c) rep-
resent a different kind of symmetry: a mirror reflection
with respect to the line joining points

and 3 gives
diagram (c) from (b), and similar reflection of (c) gives
Ta3. ble The numbers and

,
i
NN
ab
SN
S
terms calculated from the recurrence Formula (13) for 310N.
3N

12 12011SSSSS 
32
1112SS
4N
 
13222 13
12 3SSS SSS SS
43
3235SS

5 N



143 232 321 4315 9SSSSSSSSSS S 
54
914SS
6 N





1542433324215
9321428S SSSSSSSSSSSSS  
65
28 42SS
7 N







16 525 434 343 252 16289654290SSSSSS SSS SSSSSS SS   
76
90 132SS
8N









17 626 535 444 353262 179028 18 1514 132297SSSSSS SSSSSS SSSSSS SS   
87
297 429SS 
9 N














18 727 636 545 454 363 27218
29790564542424291001
SSS SSS SSS SSS SSS SSS SSSSS      
  
98
1001 1430SS 
10 N
 







 
19 828 737646 555 464 373 28219
10012971805614012612613214303432
SSSSSSS SSS SSSSSS SSSSSS SSSS    
 
1093432 4862SS 

S. OLSZEWSKI
146
(a) (b) (c) (d)
Figure 5. Two symmetric and two asymmetric diagrams for
energy terms belonging to . Symmetric diagrams (a)
and (d) represent non-deergy termymmetri
diag of
respect to the line joining point
6N
generte enas, asc
rams (b) and (c) lead to two degenerate components
the perturbation energy.
diagram (b). Either of symmetry mentioned above is cha-
racteristic for any diagram of order N, so the diagrams
can be classified as either symmetric, or asymmetric with

on the main loop of
time with a point that is the most distant from

. For
even N, the second point is labeleby a point d 2N
on the loop. However, for odd N, the most distant point
from

does not coincide with acattering event on the
time scale, but is half of the time interval between the
scattering points, labeled by
s

12 Nand

1
 
12 1N. For example, fo1N, this is no point
on the loop beyond the beginning-end point
r

and
there are no contractions on that loop; see Figure 6.
The symmetry behavior of the dias the
energy: the symmetriagrams are non-
degenerate, which means that the perurbative ter cor-
responding to such diagram is different from all o
grams affect
perturbation ic d
m
ther
terms. But the asymmetric diagrams give pairs of degen-
erate energy terms. This degeneracy is only twofold be-
cause it is due solely to the property of asymmetry of
diagrams entering given pair. According to the rules ap-
plied earlier [12-16] the diagrams presented in Figure 5
provide us with the following perturbation terms:



;
a
nppq qr rsst tn
npnqnrnsnt
E
UUUUUU
EEEEEEEEEE


(16)


 



2
2
;
nppq qr rs sn
nn b
np nqnrns
nppq qr rs sn
nn
c
npnqnrns
UUUUU
EU
EEEEEEEE
UUUUU
EU
EEEEEEEE


 

(17)




22
nppq qr rnnppn
d
npnqnr np
UUUU UU
EEEEE EEEE 
 
.
(18)
Figure 6. Diagram of the perturbative order N


) give
nd the be
repre
senting the side loops of diagrams (b) and (cn in
Figure 5. There is no scatter ing point bey oginning-
end point
-

on the loop.
In cases (b) and (c) [see (17)], the side loop provides
us with a factor that represents the first-order per-
turbation energy
nn
U
of 1N

. Another factor is entering
the energy (18):

2
np pn
np
UU
EE
. (18a)
This factor corresponds (apart of its sign) to the sec-
on
non-d
ntum state
d-order perturbation energy

2N because the side
loop of diagram (d) has two points on it. In the Formulae
(16)-(18a) n
E is a egenerate energy of an original
(unperturbed) quan.
The sym
la
bols
,,
pqr
EEE (19)
bel unperturbed energies of states ,,pqr The
formula for the matrix element
p
q
U between states
p and q is given in (2). The repetition of the indi-
ces ,,,pqr in the numerator of the energy expres-
sions given in (16)-(18a) implies a summation over p, q,
r, 5, we give a scti··· In Section
ate perturbation
elee for the degen-
er terms of a given
mpply
on rul
order N.
5. Algebra of the Time Loops in Diagrams
and Selection of Degenerate Perturbation
Terms
Graphical presentation of the perturbation terms is a
rather tedious way to select the degenerate terms, but an
algebraic expression of the diagrams can be developed to
implify the selection problem. As an exaple, we as
this kind of algebraic expression for diagrams of order
6N

.
Any diagram can be represented as a product referring
the numb er of points lying on the loops in that dia-to
gram. The side loops are considered in chronological
order, which means that the loops containing earlier scat-
tering points are represented before those containing later
points. An exception is the loop having the beginning-
end point

: this loop is presented regularly as the end
factor of any product. For example, the loops in Figure 5
have the following notation:

6represents diagram,La

147 S. OLSZEWSKI

Table 5. A list of twld degenerate contributions to the
perturbation energy of order . Their algebe-
presentations are gven in T The non-degenerate
energy terms are listed in Table 6. The diagrams on a
circular scale correonding to the listed terms are pre-
sented in [12].

is given in [12]; a list of
their algebraic represetions is presented in Table 4.
When one of two eqloops is nearto the point

15
15
24
represents diagram,
represents diagram,
represents diagram.
LL b
LL c
LL d
(20)
As a rule, the sum of the loop indices of L in a given
product is equal to the perturbative order N. A full set
of 42 diagrams with 6N
nta
ual side er

than another loopt loop is labeled by a su-
perscript andher loop by
, the firs
the fart

n a superscript

f
. Wheore than oe of equal login in a
n cont por numbby a
se
or ex
of
n d
iden
ut a two-fold one due to an ar-
ra
n m
raction
n
int, thei
ops be
er is indicated give
power exponent of L.
Every pair of degenerate terms is reprented by the
same product of L, fample, the second and the
third row in (20). Nevertheless, a sequence of L in
degenerate products may be different, with the exception
the last term of the product, which should be the same
for a giveegenerate pair. The degenerate pairs are
tified in Table 5, and the non-degenerate energy
terms are listed in Table 6. A characteristic point is that
no kind of degeneracy b
ngement of scattering points on the time scale is de-
Table 4. Algebraic representation of 42 energy diagrams of
perturbative order 6N. For the diagrams see [12].
66
0
L
 3
613
XXI
L
L

616
In
L
L 2
6122
XXII
L
LL

624
II n
L
L 61212
XXIII
L
LLL
633
III
L
L 2
6212
XXIV
L
LL
642
IV
L
L 3
613
XXV
L
L

f
615
V
L
L 61
2
XXVI 4
L
L
624
VI
L
L 6114 XXVII
L
LL
63
VII 3
L
L 612 XXVIII
ofo 6N
able 4.raic r i
sp
66
IX; 66 XXI XXV;
66
II IX;
; ;
66
XXII XXIV;
66
III VII; 66 XXVII XXXII;
66
VVIII; 66 XXVIII XXXI;
66
XI XX; 66 XXX XXXIII;
66
XII XIV; 66 XXXIV XXXIX;
66
XIII XVI 66 XXXV XXXVII
66
XVIII X; IX 66 XL XLI;
Table 6. Non-degerate terms contributing to the pertur-
bation energy of oer 6N
ne
rd

. Their algebraic repenta-
tions are given in Table 4, for te diagrams see [12].
;
res
h
6
0; 6 IV; 6 VI; 6 XV; 6 XVII; 6 XIII
6
XXVI ; 6 IX; XX 6 XXXVI ; 6 XXXVIII .
tected for the perturbation terms.
(21)
for
The number of degenerate terms is equal to 2 multi-
plied by
0, 0,1,4,16, 56
2,3, 4, 6,N
3
L
LL

61
VIII f
5
L
L 611
XXIX 4
L
LL

62
IX n
4
L
L 2
611
XXX 3
L
LL

61
Xn
5
L
L 6 213
XXXI
L
LL
2
61
XI 4
L
L 611 XXXII4
L
LL
612
XII 3
L
LL 2
611
XXXIII 3
L
LL
613
XIII 2
L
LL 612 XXXIV 3
L
LL
6 213
XIV
L
LL 613 XXXV 2
L
LL
2
62
XV 2
L
L 622 XXXVI 2
L
LL
631
XVI 2
L
LL 613 XXXVII2
L
LL
2
61
XVII 4
L
L 2
612
XXXVIII 2
L
LL
612
XVIII 3
L
LL 612 XXXIX 3
L
LL
621
XIX 3
L
LL 6121 XL 2
L
LLL
2
61
XX 4
L
L 6112 XLI 2
L
LLL
,5 7


5
, ecy. Binnresptiveleging with
N

this numbr is meuch la numrger than theber of
on-degenerate terms, which is
e as they are listed below (21).
It can be noted that an equality of t
sentations of the two diagrams is a
cient condition for
energi
example, XXVII6 and
XXXII6 in Table 4 give degenerate te
a non-degenerate contribution.
6.
n
1,2,3,6,10, 20 (22)
for the samN
he algebraic repre-
necessary but not
suffi the degeneracy of the diagram
es: some equal algebraic representations can also
give non-degenerate terms. For
rms, but XXIX6 is
Summary
In the first step, we presented a graphical and algebraic
derivation of the number
N
S of the RS perturbative
components of the energy for some perturbative order
N from the values
N
a
S,
N
b
S for the lower perturbat-
ive orders a N, b N, where a
rt
n i
N
The
e
and satisfy the
urbetum state is
ate one. A ilar recur-
ce formula is obtaincrem
b
N
d quan
sim
ent
complementarity r
assumed to be a
u
no
le (8).
n-deg
ned
e p
ner
for arenS

which
should be added to 1N S

to obtain
N
S, so

S. OLSZEWSKI
148
deacy for the
energy terms belonging to very low p
fo
an Mechanics,” Cambridge
ss, Cambridge, 1967.
, “A Guide to Feynman Diagrams in the
1NN
SS S

. (23)
The calculations make reference to the properties of
the time contractions characteristic for a circular scale of
time along which the perturbative effect of a quantum-
mechanical system is developed.
The property of asymmetry of the time loops on a cir-
cular scale is applied in examining the degeneracy of the
perturbation terms. An absence of gener
erturbative orders is
und. However, a twofold degeneracy of most of per-
turbative terms can be detected when the perturbative
order N becomes larger than 5.
7. References
[1] E. Schrödinger, “Quantization as an Eigenvalue Problem
III,” Annalen der Physik, Vol. 80, 1926, pp. 437-490.
[2] P. M. Morse and H. Feshbach, “Methods of Theoretical
Physics, Part 2,” McGraw-Hill, New York, 1953.
[3] N. H. March, W. H. Young and S. Sampanthar, “The
My-Body Problem in Quantum
University Pre
4] R. D. Mattuck[
Many-Body Problem,” 2nd Edition, McGraw-Hill, New
York, 1976.
J. Killingbeck, “Quantum-Mechanical Perturbation T[5] he-
ory,” Reports on Progress in Physics, Vol. 40, No. 9,
1977, pp. 963-1031. doi:10.1088/0034-4885/40/9/001
y, Vol. 21, 1982, pp.
Guillou and J. Zinn-Justin, “Large-Order Behav-
ysical Re-
Order Perturbation Theory and Summation Methods in
Quantum Mechanics,” Springer, Berlin, 1990.
[8] J. C. Le
iour of Perturbation Theory,” North-Holland, Amsterdam,
1990.
[9] R. P. Feynman, “The Theory of Positrons,” Ph
view, Vol. 76, No. 6, 1949, pp. 749-759.
doi:10.1103/PhysRev.76.749
[10] R. Huby, “Formulae for Rayleigh-Schrödinger Perturba-
6.
s for Non-Degenerate Ray-
Topology for Some Classical and
owski, “A Topological Ap-
1998, pp. 445-
tion Theory in Any Order,” Proceedings of the Physical
Societ, London, Vol. 78, 1961, pp. 529-53
[11] B. Y. Tong, “On Huby’s Rule
leigh-Schrodinger Perturbation Theory in Any Order,”
Proceedings of the Physical Society, London, Vol. 80,
1962, pp. 1101-1104.
[12] S. Olszewski, “Time Scale and Its Application in the
Perturbation Theory,” Zeitschrift fur Naturforschung A,
Vol. 46, 1991, pp. 313-320.
[13] S. Olszewski, “Time
Quantum Non-Relativistic Systems,” Studia Philosophiae
Christianae, Vol. 28, 1992, pp. 119-135.
[14] S. Olszewski and T. Kwiatk
proach to Evaluation of Non-Degenerate Schrodinger Per-
turbation Energy Based on a Circular Scale of Time,”
Computation Chemistry, Vol. 22, No. 6,
461. doi:10.1016/S0097-8485(98)00023-0
[15] S. Olszewski, “Two Pathways of the Time Parameter
Characteristic for the Perturbation Problem in Quantum
Chemistry,” Trends in Physical Chemistry, Vol. 9, 2003,
pp. 69-101.
[6] P. O. Löwdin, “Proceedings of the International Work-
shop on Perturbation Theory of Large Order,” Interna-
tional Journal of Quantum Chemistr
[16] S. Olszewski, “Combinatorial Analysis of the Rayleigh-
Schrodinger Perturbation Theory Based on a Circular
Scale of Time,” International Journal of Quantum Chem-
istry, Vol. 97
1-353.
[7] G. A. Arteca, F. M. Fernandez and E. A. Castro, “Large
, No. 3, 2004, pp. 784-801.
doi:10.1002/qua.10776