Int'l J. of Communications, Network and System Sciences
Vol.3 No.6(2010), Article ID:2008,6 pages DOI:10.4236/ijcns.2010.36075
Design and Simulation of Intelligent Optical WDM Switching Node Based on Erlang Traffic Model
Electrical and Electronics Engineering Department, Birla Institute of Technology & Science, Pilani Rajasthan, India
E-mail: mkdutta13@gmail.com, vkc@bits-pilani.ac.in
Received February 6, 2010; revised March 17, 2010; accepted April 25, 2010
Keywords: WDM Networks, Erlang Traffic, Optical Node, Blocking Probability, Traffic Fraction
Abstract
In this paper we propose a new architectural switching nodes consisting of two processing nodes that follow Erlang B and Erlang C traffic respectively. The developed model is used to best utilize the given number of output channels to achieve the least blocking probability. An appropriate mathematical model has been further devised and its call blocking probability has been enunciated. Performance of the model has been evaluated for different values of blocking probabilities. It has been observed that the performance of the network is satisfying for different design parameters.
1. Introduction
Wavelength division multiplexing (WDM) is a promising technology which, in conjunction with wavelength routing, enables the optical networks to provide a throughput of the order of Gbs/sec and to handle hundreds of nodes simultaneously. This is possible because of all optical wavelength routed networks exploit wavelength reuse and removes the electro-optic bottleneck from the networks [1-3]. Tremendous traffic demand in all optical networks needs an appropriate optical control layer supported with configurability, restorability, bandwidth utilization and node accessibility [4-8]. Various application based traffic to support quality of service in the wavelength division-multiplexed (WDM) system needs effective control protocols and efficient algorithms [9-10]. Several approaches have been proposed to manage the optical traffic through WDM network involving optical circuit switching, optical packet switching or optical burst switching with appropriate routing algorithms [11-13]. WDM technology along with optical packet switching has changed the static usage of WDM network into an intelligent optical network capable of efficient routing and switching [14-15]. The performance of packet switched optical networks is characterized by evaluating the blocking probability and optimum management of available channels. This traffic management requires optical logic processing and data buffering [16]. In optical wavelength-division multiplexing (WDM) networks, traffic can be very bursty in nature. The instantaneous characterization of such traffic shows that the irregular oscillation of the traffic load and the occurrence of blockings in a light-loaded network are highly correlated. Specifically, most blockings occur concentratively at the peaks of the instantaneous load. In some other time, network resources may not be sufficiently utilized. To make better utilization of network resources, a novel and intelligent channel assignment scheme is necessary. By releasing a portion of available channel resources under light loading and recapturing them when the load goes up, a number of blockings brought by the irregular oscillation of the traffic load can be reduced [17].
In the present paper we propose an appropriate model of WDM optical switch having two processing nodes (node B, node C) with fixed number of output channels. In the node architectural design the processing node B is assumed to follow Erlang B traffic model while the node C serves with Erlang C traffic model. Further it is also assumed Poisson arrivals of traffic from the source to the intermediate router which then arbitrarily routes the incoming traffic to the nodes. The flow of traffic to node B and node C is highly irregular i.e., at any instant of time one node may be heavily loaded and other one is lightly loaded. This may cause a rise in blocking probability of the heavily loaded node to increase beyond the desired value but at the same time other node may be left with some unused channels and thus the available channels of the nodes are not always best utilized. Here we have introduced one controller unit which will reallocate (reserve or release) the available output channels of the nodes depending on the traffic load of the respective node. In the present model if the traffic flows to a particular node becomes high then the traffic flow through the other node falls due to sharing of the incoming traffic by the two nodes. In this situation the heavily loaded node will not be able to maintain the desired blocking probability with its own output channels where as the lightly loaded node has unused channels which can be shared by the busy node and vice-versa. Traffic flow through the nodes are purely random but can be measured. From this measured value of traffic the controller unit with the help of some empirical formula will continuously calculate the number of output channels required to meeting the desired blocking probability and hence the necessary action will be taken.
Evaluation of these channel shifting properties and the corresponding blocking probability is of utmost importance and in this paper an attempt has been made to develop a simple analytical model and mathematical expressions for an optical switching network capable to implement different blocking probabilities and to provide best channels utilization. The performance of the switch has been evaluated to show their qualitative proximity with existing result.
2. Modeling of Optical Network
Here we have assumed that source is generating maximum ρ amount of traffic and sending them to the traffic router. The traffic router will route a fraction ‘k’ of the incoming traffic to node B, Erlang B traffic and the rest of the traffic i.e., (1 – k) fraction to node C, Erlang C traffic. The value of traffic fraction ‘k’ may vary from zero to one. Value of k equal to zero means the entire incoming traffic is routed to node C and k equal to one means the entire traffic is routed to node B. Figure 1 depicts the switch architecture showing N and M output channels for node B and node C respectively, to support a critical value of traffic fraction k to be processed through B for a particular blocking probability at the node. If the value of ‘k’ goes beyond that value then blocking probability in Node B will start to increase and that in Node C will decrease and vice-versa. In the next section a mathematical model has been developed to estimate the critical value of ‘k’ to ensure a desired blocking probability. The traffic router will continuously check the value of ‘k’ and will send a corresponding control signal to the channel shifter as shown in the Figure 1. Here the function of the channel shifter is to shift some channels between node B and node C as and when required. The number of channels required to be shifted (n) to keep the blocking probability unchanged has also been developed through the simulation results in the next section. If required number of channels to meet the desired blocking probability is not available for the other lightly loaded node, then the shifter may shift the instantaneous available free channels to reduce the blocking probability of the heavily loaded node to some extent. Three different types of control signals will be generated by the traffic router and will be transmitted to the channel shifter so that it can take proper actions, viz. no action is necessary, shift maximum ‘n’ number of channels from node C to node B or reverse. This proposed model may be used to best utilize the given number of output channels for least blocking probability. The complete functional behavior of the proposed switching network has been described by the flowchart as shown in the Figure 2.
3. Mathematical Model
In order to evaluate the performance of the proposed WDM optical network we need to derive the probabilistic evaluation of Node B and Node C. The Node B and Node C of the present WDM network contain N and M number of output channels respectively. The well known Erlang B formula is derived from the assumption that node B has no queue so an arriving call is either served or rejected. Blocking probability of Erlang B formula is
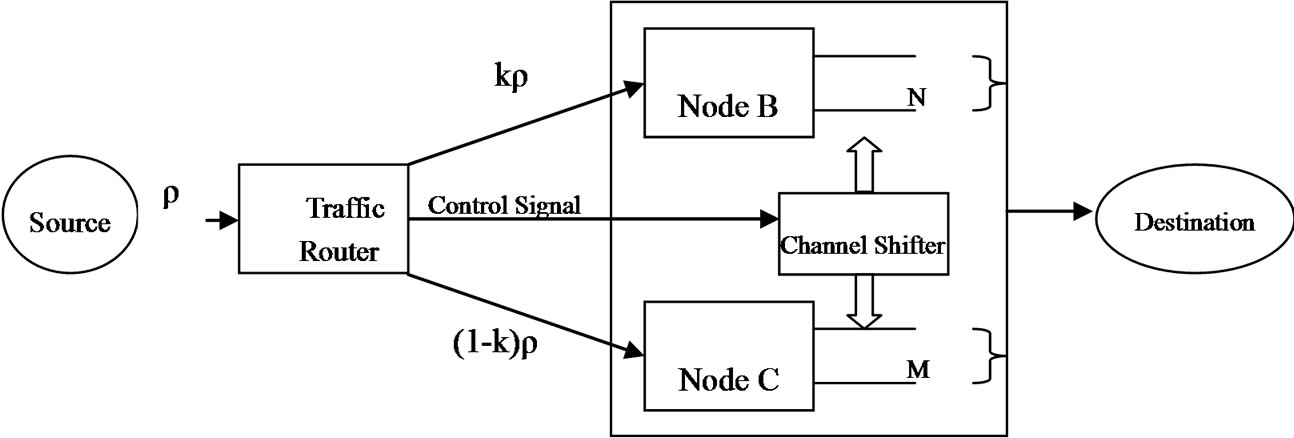
Figure 1. Node Architecture.
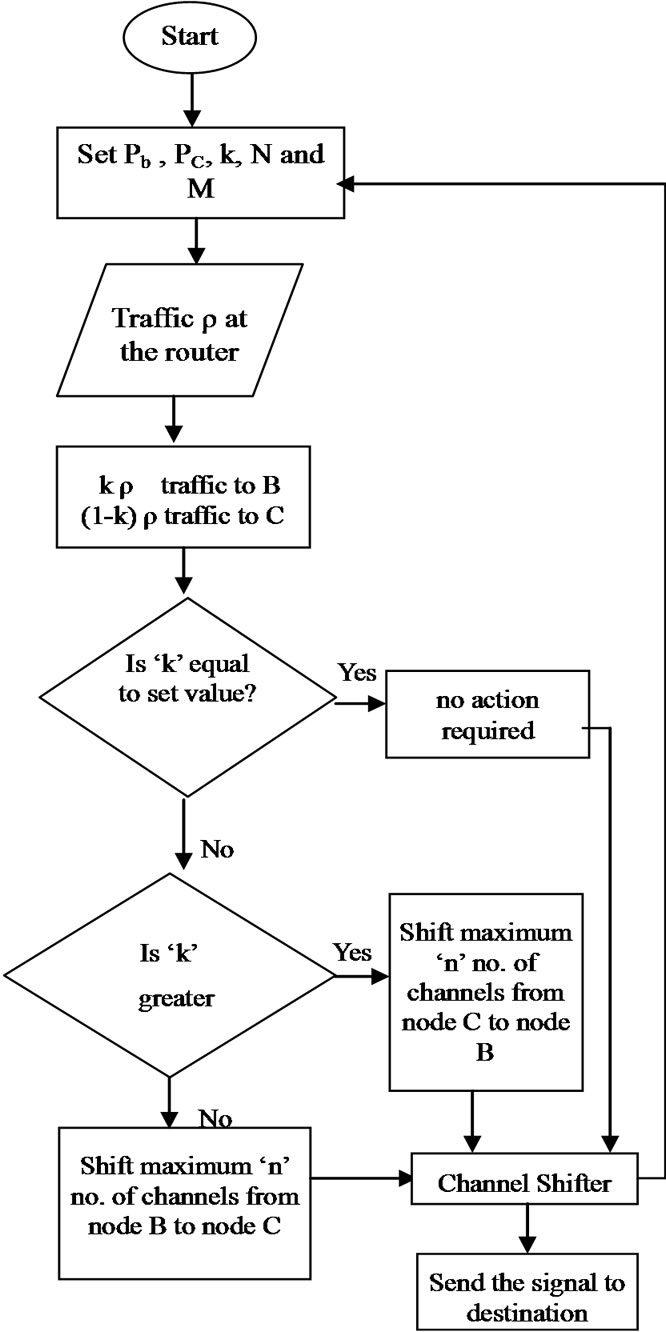
Figure 2. Flow chart of the proposed switching node.
given by
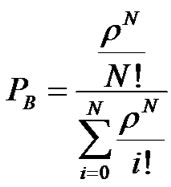 (1)
(1)
where, 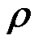 is the incoming traffic. In our model the traffic through the nodes are purely random in nature. The traffic router will route ‘
is the incoming traffic. In our model the traffic through the nodes are purely random in nature. The traffic router will route ‘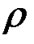 k’ amount of the incoming traffic to node B, and
k’ amount of the incoming traffic to node B, and 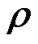 (1 – k) amount of traffic to node C. So in this case the above Erlang B blocking probability will be modified and
(1 – k) amount of traffic to node C. So in this case the above Erlang B blocking probability will be modified and
 (2)
(2)
Erlang C formula is derived from assumption that a queue is used a queue is used to hold all request calls which cannot be immediately assigned a channel. The Erlang C formula is given by
 (3)
(3)
and the blocking probability is given by
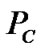 =
=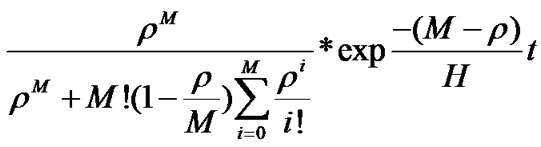 (4)
(4)
where, t is the delay time and H is the average duration of the call. But in our network the equation will be modified and
 (5)
(5)
The above equations have been used to find out the critical value of ‘k’ to ensure a desired blocking probability and the result is shown in the table below. From the simulation results which have been listed in Table 1, the following empirical formula has been developed to find out the required number of output channels to maintain the desired blocking probability for different values of traffic fraction ‘k’. n = [a (1.6k) – N], where ‘a’ is some constant and whose value will depend on node parameters.
These equations have been used in blocking probability calculation in the MATLAB environment under the appropriate node and traffic assumptions.

Table 1. Call blocking probability for various traffic fraction.
4. Simulation and Results
The simulations are carried out for the proposed optical switching node for a generic traffic with variable traffic routing factor. Case 1 presents the dependence of routed incoming traffic on the required number of output channels at processing nodes for different blocking probabilities considering 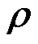 as 5 Earlang. In case 2 the dependency of blocking probability on the number of output channels for three different values of incoming traffic fraction have been evaluated and the corresponding curves have been drawn in Figures 5 and 6.
as 5 Earlang. In case 2 the dependency of blocking probability on the number of output channels for three different values of incoming traffic fraction have been evaluated and the corresponding curves have been drawn in Figures 5 and 6.
It is inferred from Figures 3 and 4 that a higher traffic needs a larger number of output channels to maintain a required value of blocking probability. Similarly it is also clear from the graph that, addition of output channels decreases the blocking probability for both types of nodes. It is found from the figures that the number of channels required to maintain same blocking probability is significantly higher in case of node B as compared to node C for the same circumstances. It may also be noted that to maintain a blocking of 4.1 × 10-5 at 4.5 Erlang load,
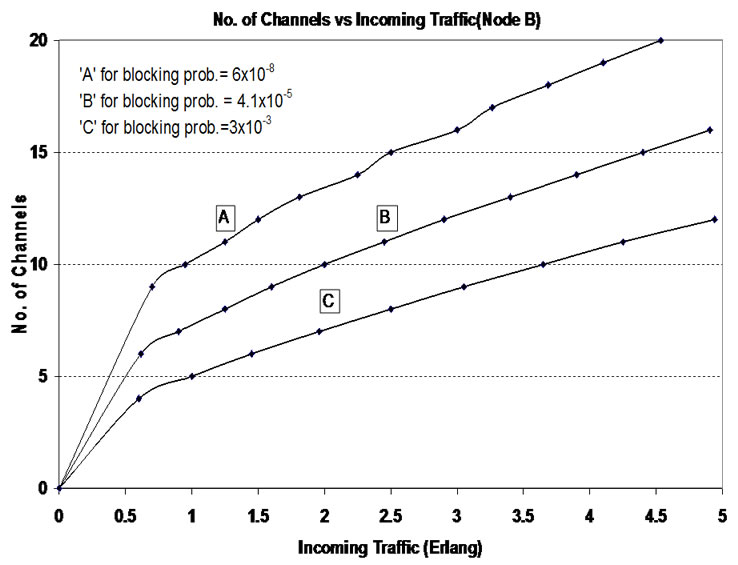
Figure 3. No of channels vs incoming traffic for different.
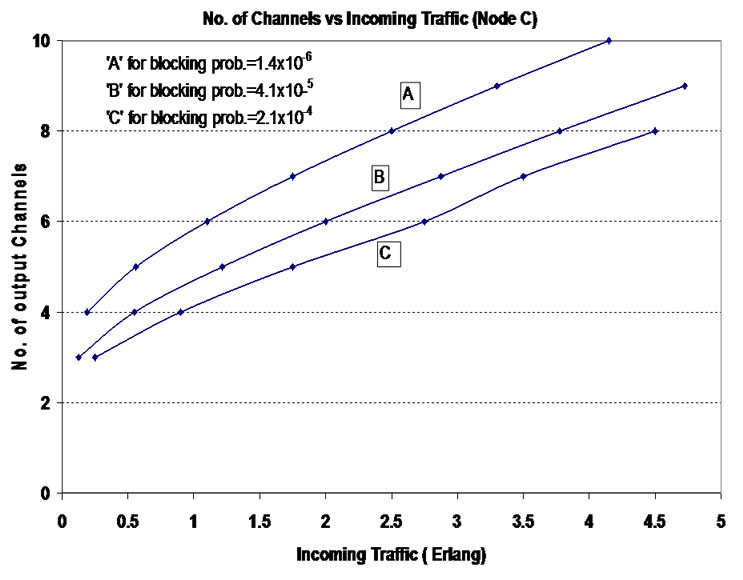
Figure 4. No of channels vs incoming traffic for different.

Figure 5. Blocking probability vs no of channels for different values of traffic fraction in nod B.
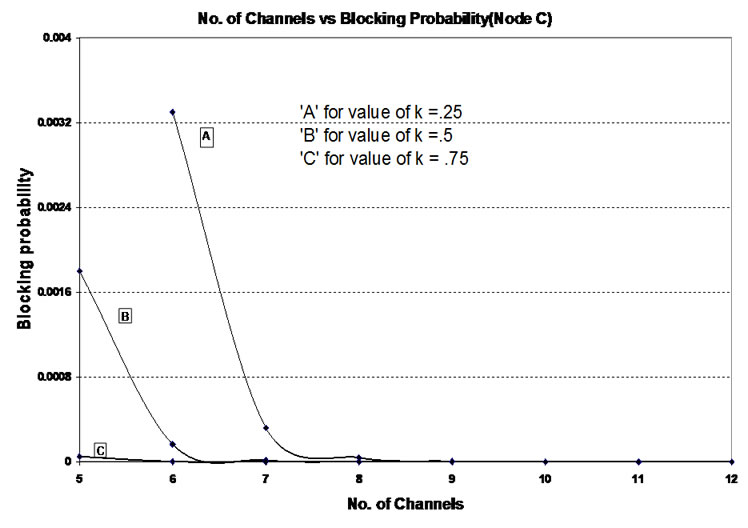
Figure 6. Blocking probability vs no of channels for different values of traffic fraction in node C.
the desired output channels for node B to process alone will be 15, however this number get reduced to 9 in case of traffic to be processed by node C alone. This observation is quite obvious due to different traffic handling capacities of the nodes. For a given number of available output channels Erlang C model is superior to Erlang B model but implementation of previous adds cost and the network complexity. Therefore in our proposed switching node we have used the combination of both types of traffic models. This approach may lead to a compromise between cost and complexity with the node throughput.
Figure 3, curve ‘A’ indicates that, for 8 available output channels node B can process upto 0.5 Erlang of incoming traffic to maintain a blocking probability of 6 × 10-8. Similarly the channel requirement graph for a larger allowed blocking probability has been depicted by curves B and C, showing a lower channel requirement implying a compromise with the throughput. The channel requirement increases almost linearly with the amount incoming traffic for a fixed blocking probability but the slope of curve becomes steeper to yield low blocking probability. On the other hand the node C with 8 output channels can process upto 2.5 Erlang of traffic even after maintaining blocking probabilities of 1.4 × 10-5 as is evident by curve ‘A’ in Figure 4. Node C shows the similar behavior as in case of node B, but with smaller quantitative difference. It is interesting to note that as the incoming traffic is distributed between the two nodes unevenly, one of the nodes gets highly loaded leaving the other with a lesser load. At this condition the lightly loaded node is left with few unused channels but the highly loaded node suffers from scarcity of output channels, leading to poor performance. In this situation if the unused channels of lightly loaded node are available to the heavily loaded node then blocking probability of the entire switching node will improve significantly.
The variation of blocking probability with number of output channels has shown in Figures 5 and 6 for both the cases. Three different values of incoming traffic fraction ‘k’ have been considered to execute the blocking probability with the number of output channels for both of the processing nodes. It can be inferred from the curves of Figures 5 and 6 that blocking probability reduces as the number of output channel increases for both types of nodes. This change is more prominent in node C; showing a significant improvement in the blocking probability. This improvement may be attributed to the efficient traffic model applicable to node C.
These observations can be used to employ channel shifting property of the proposed node architecture to obtain a satisfactory reduction in blocking probability at high traffic flow. Thus present analysis is useful for the node designer to optimize the available channels for the minimum blocking probability through switching node utilizing channel shifter effectively.
5. Conclusions
This paper presents an analytical approach to investigate the performance of an optical WDM switching node under variable Erlang traffic condition. A new architectural model with two different types of processing nodes has been proposed for the switching action. Mathematical model also been developed to evaluate the blocking probability of the switching node for variable incoming traffic. The proposed architecture may be used to utilize the given number of output channels for least blocking probability. The approach adopted is quite simple and involves basic node system behavior but still provides a well acceptable performance. The results are validated by MATLAB simulations and show a distinct influence of the incoming traffic over usable channel and blocking probability of the switching node as well.
6. References
[1] A. Sridharan and K. N. Sivarajan, “Blocking in All-Optical Networks,” IEEE/ACM Transactions on Networking, Vol. 12, No. 2, 2004, pp. 384-397.
[2] M. K. Dutta and V. K. Chaubey, “Priority Based Wavelength Routed WDM Networks: A Queueing Theory Approach,” International Journal of Recent Trends in Engineering (Electrical & Electronics), Vol. 1, No. 3, 2009, pp. 253-256.
[3] M. De Leenheer, C. Develder, F. De Truck, B. Dhoedt and P. Demeester, “Erlang Reduced Load Model for Optical Burst Switched Grids,” Erlang-gobs, 2007.
[4] Y. L. Hsue, M. S. Rogge, S. Yamamoto and L. G. Kazovsky, “A Highly Flexible and Efficient Passive Optical Network Employing Dynamic Wavelength Allocation,” IEEE/OSA Journal of Lightwave Technology, Vol .23, No. 1, 2005, pp. 277-286.
[5] A. A. Saleh and J. M. Simmons, “Evolution towards the next Generation Core Optical Network,” IEEE/OSA Journal of Lightwave Technology, Vol. 24, No. 1, 2006, pp. 3303-3320.
[6] L. Guo, J. Cao, H. Yu and L. Li, “Path Based Routing Provisioning with Mixed Shared Protection in WDM Mesh Networks,” IEEE/OSA Journal of Lightwave Technology, Vol. 24, No. 3, 2006, pp. 1129-1141.
[7] Y.-L. Hsue, J. Kim, C.-F. Su, R. Rabbat, T. Hamada, C. Tian and L.G. Kazovsky, “Traffic Grooming on WDM Ring Using Optical Burst Transport”, IEEE/OSA Journal of Lightwave Technology, Vol. 24, No. 1, 2006, pp. 44-52.
[8] V. K. Chaubey and K. Divakar, “Modeling and Simulation of WDM Optical Netwroks under Traffic Control Protocols,” Optical Fiber Technology, Vol. 15, No. 2, 2009, pp. 95-99.
[9] M. K. Dutta and V. K. Chaubey, “Optical Network Traffic Control Algorithm under Variable Loop Delay: A Simulation Approach,” International Journal of Communication, Network and System Sciences, Vol. 7, 2009, pp. 651-655.
[10] G. S. Zervas, M. D. Leenheer, et al., “Multi Granular Optical Cross-Connect Design, Analysis and Demonstration,” Journal of optical communication Netwroking, Vol. 1, No. 1, 2009, pp. 69-84.
[11] B. Wen, R. Shenai and K. Sivalingam, “Routing, Wavelength and Time-Slot-Assignment Algorithms for Wavelength-Routed Optical,” IEEE/OSA Journal of Lightwave Technology, Vol. 23, No. 9, 2005, pp. 2598-2609.
[12] A. Mukherjee, S. P. Singh and V. K. Chaubey, “Wavelength Conversion Algorithm in an Intelligent WDM Network,” Optics Communications, Vol. 230, 2004, pp. 59-65.
[13] R. Kundu and V. K. Chaubey, “Analysis of Optical WDM Network Topologies with Application of LRWC under Symmetric Erlang-C Traffic, Novel Algorithms and Techniques in Telecommunication, Automation and Industrial Electronics,” Springer Science, 2008, pp. 468- 473.
[14] F. C. G. Corazza and C. Raffaelli, “An Optical Packet switch with a Multi-Stage Buffer for IP traffic, Optical Networking,” A. Banoni, Ed., Springer, 1999, pp. 300-311.
[15] F. Masetti, et al., “High Speed, High Capacity ATM Optical Switches for Future Telecommunication Transport Networks,” IEEE Journal on Selected Areas in Communications, Vol. 14, No. 5, 1996, pp. 979-999.
[16] J. Y. Wei, C. D. Lie, S. Y. Park, K. M. Liu, R. S. Ramamurthy, H. Kim and M. W. Marda, “Network Control and Management for Next Generation Internet,” IIEICE Transactions on Communications, Vol. E83-B, No. 10, 2000, pp. 2191-2209.
[17] N. Hua, X. P. Zheng, H. Y. Zhang and B. K. Zhou, “A Wavelength-Buffering Scheme for Dynamic Traffic in Optical Wavelength-Division Multiplexing Networks,” Photonic Network Communications, Vol. 16, 2008, pp. 43-51.
[18] J. C. Bellamy, “Digital Telephony,” 3rd Edition, John Wiley & Sons, New York, 2002.

