Journal of Modern Physics
Vol.07 No.09(2016), Article ID:66817,16 pages
10.4236/jmp.2016.79086
Three Clifford Algebras for Four Kinds of Interactions
Claude Daviau1, Jacques Bertrand2
1Le Moulin de la Lande, Pouillé-les-Coteaux, France
215 Avenue Danielle Casanova, Saint-Gratien, France

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 26 April 2016; accepted 24 May 2016; published 27 May 2016
ABSTRACT
Three Clifford algebras are sufficient to describe all interactions of modern physics: The Clifford algebra of the usual space is enough to describe all aspects of electromagnetism, including the quantum wave of the electron. The Clifford algebra of space-time is enough for electro-weak interactions. To get the gauge group of the standard model, with electro-weak and strong interactions, a third algebra is sufficient, with only two more dimensions of space. The Clifford algebra of space allows us to include also gravitation. We discuss the advantages of our approach.
Keywords:
Geometry, Invariance Group, Dirac Equation, Electromagnetism, Weak Interactions, Strong Interactions, Clifford Algebras, Gravitation

1. Introduction
Why Clifford algebras are necessary in physics? Physics uses waves and the Fourier theorem says that any periodic function may be decomposed in a sum of sin and cos functions. These functions are more easily studied with the complex exponential function. Moreover the exponential function is the main tool in Lie groups. The exponential function needs products, so we must also know how to multiply numbers and vectors and products of vectors: we need a Clifford algebra. Why space algebra? The first reason is simply that our physical space is 3-dimensional [1] . The second reason is the spin 1/2 of all fundamental fermions, which uses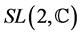 , that is a subgroup of
, that is a subgroup of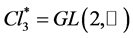 . This greater group is the group of form invariance of electromagnetism, wave of the electron included [2] - [6] . And this form invariance rules [7] - [9] also the other kinds of interactions. We first recall how the group of the invertible elements in the space algebra
. This greater group is the group of form invariance of electromagnetism, wave of the electron included [2] - [6] . And this form invariance rules [7] - [9] also the other kinds of interactions. We first recall how the group of the invertible elements in the space algebra 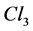 acts. Time and space take naturally place in the auto-adjoint part of this algebra, with
acts. Time and space take naturally place in the auto-adjoint part of this algebra, with
 (1)
(1)
Quantum mechanics lets, since 1927 [10] :
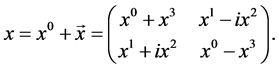 (2)
(2)
Space-time is then the auto-adjoint part of the space algebra [1] :


 (3)
(3)
This allows us to read the Dirac wave of the electron in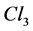 . The link1 with the old complex formalism is simple only if we use the left and right Weyl spinors
. The link1 with the old complex formalism is simple only if we use the left and right Weyl spinors 












With




where



Only one M term is present in the transformation of 



is not an isomorphism, but only an homomorphism from 






if they satisfy


der the electric gauge transformation which reads in

We have three reasons for enlarging the group of invariance from 






The space algebra 





where J is the current of density of probability and 


We have previously explained how classical electromagnetism, without or with magnetic monopoles, reads in space algebra (chapter 4 of [8] and [9] ):

where F is the electromagnetic field, A is the vector electric potential, 


2. Numeric Dimension
We name “numeric-dimension” of any physical quantity the power of the ratio 












This is important when we want to put together electromagnetism and gravitation. The numeric-dimension has also predictive value: we said previously, as anyone, that J is the current of probability, but the true current of probability is
3. The Electro-Weak Gauge in Space-Time Algebra
Two non equivalent homomorphisms [10] exist from 

This is the origin of the existence of left and right waves, that are fundamental for weak interactions. We have obtained [20] in the 




where indices e, n, p and a indicate the respective waves of the electron, the electronic neutrino, the positron and the electronic anti-neutrino. They satisfy:

The Weinberg-Salam model [21] has no use for the right wave 

The charge conjugation is the same as in the standard model of quantum physics. Instead of the 



The invariant wave equation of electron + neutrino reads

where 



with







The covariant derivative of electro-weak interactions uses:

with




let of right-handed particle. Y is the weak hypercharge, 

We have proved [7] - [9] [25] that the covariant derivative (21) of the Weinberg-Salam model is exactly equivalent to

The wave Equation (18) is invariant under the R transformation generated by any M element of




This implies the relativistic invariance of the wave equation. It is also gauge invariant (exactly!) under the gauge transformation:

where 


The proper mass in (18) is the proper mass of the lone electron, then with (19) the wave equation of electron + neutrino is reduced to the invariant wave Equation (6) when the wave of the electronic neutrino is canceled. If the wave of the electron is canceled the mass term is canceled and we get for the neutrino the Dirac equation without mass:

The question: “does the neutrino have a proper mass?” is not a correct question: only the pair electron + neutrino has a mass term, and this term is not m but
The real part of the invariant wave equation is also

We have studied [24] the solutions of our wave equation for electron + neutrino in the case of the hydrogen atom, because this case has made the fame of the Dirac equation. It is astonishing to see that a set of solutions exists, with the same quantum numbers, the same number of states, the same energy levels as for the electron alone. But these solutions are obtained with other potentials:

where 

And the potentials are obviously a domain where many surprises remain before us. These new solutions of an old problem have a left chirality. We suspect that this left chirality is the origin of the left chirality in bio-che- mistry.
4. The Gauge Group of the Standard Model in Cl1,5
To get a wave function including electron + neutrino plus two quarks u and d with three states of color each the space-time algebra is not enough: even if the quarks have only left waves these 9 spinor waves (two spinors for the electron) necessitates 36 parameters and 





Three states of “color” are named r, g, b (red, green, blue). So we build a wave with all fermions of the first generation as

where 




These definitions use the matrix8 representation:

The color is then here only the particular location of a matrix bloc. The wave contains not only all particles of the first generation, but also all anti-particles of these objects. We let

for 

We use two projectors 

Three operators act on the quark sector as on the lepton sector :

The fourth operator acts differently on the lepton wave and on the quark sector:

The value −1/3 gives the four correct values of the charges of quarks and antiquarks (see [9] 6.1). To get the gauge group of chromodynamics we need the projectors

The operators corresponding to the eight generators of 



We can extend the covariant derivative of electro-weak interactions (23):

where 






commutes with all operators




and we get, using exponentiation which is a function from Lie algebra into Lie group (see [9] 6.2):

The set of these operators is a 


The 


We get then a 

The wave equation for the complete wave reads:

The mass term

contains three 




Since the only 


The wave Equation (50) is equivalent to

which is the Equation (18) if we forget that the definition of 


Like in the lepton case, the real part of the wave equation is simply the equality (see [9] 7.6)

This link between the wave equation and the Lagrangian density is very strong from the mathematical point of view, since it comes from an algebraic calculation, similar to taking the real part of a complex number. The way going from the Lagrangian density, by the variation calculus and an integration by parts, is on the contrary very dubious from the physical point of view in the case of propagating waves. This method is nevertheless always available from the mathematical point of view. Similarly to (28) only one of the numeric equations equivalent to (53) is simple, the law of conservation of the total current:

The wave Equation (47) is form invariant under the group 


The form invariance of the wave Equation (47) is compatible with the gauge invariance under the 


5. Gravitation
Quantum physics was unable to account for gravitation until now because gravitation necessitated a relativistic formulation. And the relativistic part of quantum physics used in the standard model suppressed10 the mass term of the electron to get the electro-weak gauge invariance. Now we have a relativistic wave equation with mass term able to get the electro-weak and strong interactions from gauge transformations. The mass terms present in our wave equations are able to account for both aspects of the mass: inertial mass and gravitational mass.
5.1. Inertia
Many attempts were developed to reconcile gauge theories and gravitation. General relativity gives the restricted relativity and its Lorentz group as approximation in the case of very low gravity. Since M and R were always confused, since the difference between rotations with 




We previously considered as an example ( [9] 9.1.1) a frame rotating with the Earth. We let

And we get

This gives

which is the centrifugal acceleration.
Any variable 

where




Christoffel’s symbols 

this gives

Only 28 amongst the 32 numeric functions are present in Christoffel’s symbols: the four 






with the same

Therefore we get for covariant vectors the usual

This gives

where we have let

This introduces 8 space-time vectors that we name “potentials of inertia”:

These eight potentials become under a dilation R induced by a constant M

In space-time algebra we shall need

And the covariant derivative unifying inertia to gauge interactions becomes

Contrary to all other terms that contains projectors, the term of inertia acts on the whole wave. This universality is a characteristic of inertia.
5.2. Wave Normalization
One of the differences between classical and quantum wave is the necessity to normalize the quantum wave. In a unified theory we must explain this necessity. Now we know that we always have a Lagrangian density, real part of the invariant wave equation. The invariance of the Lagrangian under all translations, like with the linear Dirac theory, induces the existence of a conservative impulse-energy tensor, the Tetrode’s tensor, which in the case of the electron, for instance, reads:

Since the wave equation is homogeneous, the Lagrangian is null and we get:

For a stationary state of an electron with energy E we have:

So we get:

The energy of the electron, linked to the frequency of the whole wave, is necessarily equal to the sum of the local energy of the wave, we must then have:

that is equivalent, for a bound state, to

This relation was always thought as: 


5.3. Gravitation
A recurrent problem in the unification of gravitation with the spinor wave of quantum mechanics is the difference of symmetry between the symmetric Ricci tensor of General Relativity and the non-symmetric tensor coming from the spinor wave. At large scales, we can approach differently the momentum-energy tensor: the global energy E of the electron is the temporal component of a space-time vector, the energy-momentum vector. Since the integration has been made in a frame where this momentum is null, this vector reads 




where 


6. The Advantages of This Approach
The use of the three Clifford algebras


We reunite the frame of classical physics, which was the space-time and vectors or tensors built on space-time, with the frame of quantum mechanics, since all interactions are described with real Clifford algebras.
Quantum waves used here are rather similar to the other waves of classical physics: they are well-defined functions of space and time with value in algebras that are also linear spaces on the real field. The quantum wave of the photon is exactly and simply Maxwell’s electromagnetic field (see [9] Sec. 4.4). The relativistic invariance is simply obtained from multiplications on the left place and gauge invariances are obtained from multiplications on the right place.
Since the geometric algebra of the physical space is isomorphic to the complex linear space of 

Waves, for fermions and antifermions but also for systems of particles (see ( [9] Sec. 5.2) are well defined functions of space and time with value in a real Clifford algebra: we are no more disturbed by the difference between space coordinates and time coordinate coming from the non-relativistic quantum wave of systems.
We integrate in a classical frame all novelties coming from quantum mechanics:
The fermion wave is made of spinors, objects that are part of the geometric algebra, with the peculiarity that they turn on a half angle in a spacial rotation. The fermion wave has value in a linear space which is also an algebra. Each value may have or not an inverse: we have found (see [9] Sec. 6.4 and 7.5) identities justifying the existence of this inverse.
The charge conjugation is a pure quantum transformation that changes only the differential part of the quantum wave (see [9] 3.4).
The group of form invariance accounts for the spin 1/2 and it is then necessarily greater than the Lorentz group. Consequently the fundamental group of invariance of all physical interactions is
Because there are two inequivalent homomorphisms of 
The invariance group is compatible with an oriented space and with an oriented time. It does not create these orientations, it only conserves them.
There are three generations of fermions and four kinds of neutrinos (see [9] Sec. 5.1 and [26] ).
The integration of two quarks in each generation, with three color states each, is made by adding two and only two dimensions to the usual space. This is compatible with the group of invariance 

The mass term of the wave equation is compatible with the form invariance under 
We integrate gravitation:
If an inertial frame is heavy enough to include a quantum wave which is stationary in this frame, a double link exists between the wave equation and the Lagrangian density.
Non-inertial frames are frames coming from the use of variable terms in
The normalization of the wave and the existence of a density of probability are both consequences of the principle of equivalence between inertial and gravitational mass-energy.
After integration on all physical space, the impulse-energy tensor of the quantum wave generates the symmetric tensor of General Relativity.
We comfort the standard model by diminishing the too numerous free parameters:
Only one proper mass can exist in each generation.
The Weinberg-Salam angle has a fixed calculable value [23] . One number gives the four values of charges of quarks and antiquarks, and the value of this number is compulsory [22] [27] .
The value of the electric charge is determined by the existence of magnetic monopoles (see [9] Sec. 8.3.2).
We justify: Three and only three similar generations and a fourth neutrino (see [26] and below).
Three colors for each quark, two quarks and two leptons in each generation.
The value of the electric charge of leptons and quarks.
The existence of the Planck constant (see [9] Sec. 3.3).
The normalization of the wave and the existence of a density of probability.
The insensitivity of leptons to strong interactions.
The strict conservation of the baryonic number, linked to this insensitivity.
The existence of a Lagrangian formalism, treating separately the three generations (see below).
The Pauli principle [27] .
7. Concluding Remarks
The Weinberg-Salam model was unable to account for an electron with a proper mass, because the mass term of the Dirac equation linked the right to the left wave of the electron. But our wave equation has a mass term and is fully gauge invariant. Therefore the complicated mechanism of spontaneous broken symmetry needed to recover a mass term is useless.
The Higgs boson exists, but it shall not give a way to compute the proper mass of the different fermions. Moreover it is possible that each generation has one Higgs boson.
The relativistic quantum theory was based on the invariance under the Poincaré group. This group contains the translations and all Lorentz transformations, conserving or not space and time orientation. In this frame the proper mass is the fundamental invariant.
On the contrary the group of Lorentz dilations contains only the subgroup of Lorentz transformations conserving, separately, the space orientation and the time orientation. The Poincaré group is both too great and too small: it is too great, because P and T transformations are purely theoretical, they are impossible to realize in the physical world.
And it is too small, because it does not account for the spin 1/2 and the dilations induced by any M of

The existence of three and only three generations of fermions comes from the existence of a privileged third direction in the wave Equation (47) and also in the linear Dirac equation [9] . We get two and only two similar equations:

The first one has a privileged first direction and the second has a privileged second direction. The privileged direction is the direction where the spin is always measured. The 








Several theoretical attempts beyond the standard model were studied. The “great unified” theories used a compact Lie group like 


The principal results of the standard model of quantum physics come from the first quantization, not from the second quantization of the quantum field theory which appears successful only for electromagnetic laws, only for the electric gauge.
Most of our results are about fermions. The boson part of the quantum world has been nevertheless not completely neglected. We have transposed in Clifford algebra the construction of the wave of a system of electrons by antisymmetric product (see [9] Sec. 5.2) and (see [9] Chapter 4) the De Broglie’s theory of light [28] [29] . This theory of light is a beginning for the wave equations of the twelve bosons of the standard model, the interactions between fermions and bosons and between bosons. A construction is possible from the fermion wave to build the wave of all other objects of the quantum world by antisymmetric products. An even number of waves gives a boson, an odd number gives a fermion. This is completely different from the supersymmetry, a theory based on the transformation of fermions into bosons and vice versa. Such a transformation is impossible if the boson or fermion nature of the wave comes from the even or odd number of fundamental fermions building this wave.
The De Broglie’s construction gives the classical electromagnetic field and Maxwell’s laws, therefore it appears as a better method to build boson waves. This construction, based on the linear Dirac equation, had the same difficulty than the Weinberg-Salam model with the mass term, which was incompatible with the gauge. We can hope now, with a mass term compatible with the gauge group, a new departure [30] for De Broglie’s method of fusion.
Since all electromagnetic laws and also the gravitation use only
Cite this paper
Claude Daviau,Jacques Bertrand, (2016) Three Clifford Algebras for Four Kinds of Interactions. Journal of Modern Physics,07,936-951. doi: 10.4236/jmp.2016.79086
References
- 1. Baylis, W.E. (1996) Clifford (Geometric) Algebras. The Paravector Model of Spacetime, Birkhauser, Boston, 237-296.
http://dx.doi.org/10.1007/978-1-4612-4104-1_17 - 2. Daviau, C. (2005) Annales de la Fondation Louis de Broglie, 30, 409-428.
- 3. Daviau, C. (2011) L’espace-Temps Double. Je Publie, Pouillé-les-coteaux.
- 4. Daviau, C. (2012) Advances in Applied Clifford Algebras, 22, 611-623.
http://dx.doi.org/10.1007/s00006-012-0351-7 - 5. Daviau, C. (2012) Double Space-Time and More. Je Publie, Pouillé-Les-Coteaux.
- 6. Daviau, C. (2012) Nonlinear Dirac Equation, Magnetic Monopoles and Double Space-Time. CISP, Cambridge.
- 7. Daviau, C. (2013) Advances in Imaging and Electron Physics, 179, 1-137.
- 8. Daviau, C. and Bertrand, J. (2014) New Insights in the Standard Model of Quantum Physics in Clifford Algebra. Je Publie, Pouillé-Les-Coteaux.
http://hal.archives-ouvertes.fr/hal-00907848 - 9. Daviau, C. and Bertrand, J. (2015) The Standard Model of Quantum Physics in Clifford Algebra. World Scientific Publishing, New York.
http://dx.doi.org/10.1142/9780 - 10. Naïmark, M.A. (1962) Les représentations linéaires du groupe de Lorentz. Dunod, Paris.
- 11. Hestenes, D. (1966, 1987, 1992) Space-Time Algebra. Gordon and Breach, New York.
- 12. Hestenes, D. (1982) Space-Time Structure of Weak and Electromagnetic Interactions. Foundations of Physics, 12, 153-168.
http://dx.doi.org/10.1007/BF00736846 - 13. Hestenes, D. (1986) A Unified Language for Mathematics and Physics and Clifford Algebra and the Interpretation of Quantum Mechanics. In: Chisholm and Common, A., Eds., Clifford Algebras and Their Applications in Mathematics and Physics, Reidel, Dordrecht.
- 14. Lasenby, A., Doran, C. and Gull, S. (1993) Foundations of Physics, 23, 1295-1327.
http://dx.doi.org/10.1007/BF01883781 - 15. Doran, C. and Lasenby, A. (2003) Geometric Algebra. Cambridge University Press, Cambridge.
- 16. Daviau, C. (1993) Equation de Dirac non linéaire. Ph.D. Thesis, Université de Nantes, Nantes.
- 17. Lochak, G. (1983) Annales de la Fondation Louis de Broglie, 8, 345.
- 18. Lochak, G. (1984) Annales de la Fondation Louis de Broglie, 9, 5.
- 19. Lochak, G. (1985) International Journal of Theoretical Physics, 24, 1019-1050.
http://dx.doi.org/10.1007/BF00670815 - 20. Daviau, C. and Bertrand, J. (2014) Journal of Modern Physics, 5, 1001-1022.
http://dx.doi.org/10.4236/jmp.2014.511102 - 21. Weinberg, S. (1967) Physical Review Letters, 19, 1264-1266.
http://dx.doi.org/10.1103/PhysRevLett.19.1264 - 22. Daviau, C. and Bertrand, J. L’onde leptonique générale: électron + monopôle magnétique. Annales de la Fondation Louis de Broglie, Submitted to Publication.
- 23. Daviau, C. and Bertrand, J. (2015) Journal of Modern Physics, 6, 2080-2092.
http://dx.doi.org/10.4236/jmp.2015.614215 - 24. Daviau, C. and Bertrand, J. (2015) Journal of Modern Physics, 6, 1647-1656.
http://dx.doi.org/10.4236/jmp.2015.611166 - 25. Daviau, C. and Bertrand, J. (2014) Journal of Modern Physics, 5, 2149-2173.
http://dx.doi.org/10.4236/jmp.2014.518210 - 26. Daviau, C. and Bertrand, J. (2013) Annales de la Fondation Louis de Broglie, 38, 57-82.
- 27. Daviau, C. and Bertrand, J. (2015) Annales de la Fondation Louis de Broglie, 40, 181-209.
- 28. de Broglie, L. (1940) La mécanique du photon, Une nouvelle théorie de la lumière: Tome 1 La lumière dans le vide. Hermann, Paris.
- 29. de Broglie, L. (1942) Tome 2 Les interactions entre les photons et la matière. Hermann, Paris.
- 30. Daviau, C. and Bertrand, J. (2015) Annales de la Fondation Louis de Broglie, 40, 113-138.
NOTES
1This link is important and non trivial. The matrices of the Dirac theory are defined only up a unitary matrix. Studying the electron, D. Hestenes [11] - [13] , A. Lasenby [14] , C. Doran [15] and one of us [16] , we have implicitly used the set of Dirac matrices coming from the non relativistic Pauli equation. Another set is used for the relativistic electron, the Weinberg-Salam model and the theory of Lochak’s magnetic monopole [17] - [19] . These matrices are linked to the left and right Weyl spinors.
2The proper mass m and 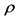 are invariant under
are invariant under , covering group replacing the awaited Lorentz group of special relativity, but the Nature uses a greater strain, the true group of invariance of physical laws is not
, covering group replacing the awaited Lorentz group of special relativity, but the Nature uses a greater strain, the true group of invariance of physical laws is not 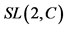 but
but . Consequently only the product
. Consequently only the product 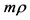 remains invariant under the full group.
remains invariant under the full group.
3This means that the orientation of space and the orientation of time are both conserved in any Lorentz dilation.
4The Lagrangian density is null for any solution of the Lagrange equations because the wave equation is homogeneous. This property results from the Noether’s theorem.
5The knowledge of the reason for the existence of the extremum principle is obviously an important result.
6The complete wave, with 

7Only 16 of these densities were known from the Dirac matrix theory.
8We use three Clifford algebras which are also matrix algebras. This has been sometimes not very well considered. So it is necessary to remark: first it is not the structure of complex matrices which is used, but the structure of algebra on the real field. Next most complex matrix algebras are not Clifford algebras, and we use only 

9This is not completely true because 

10The weak gauge necessitates a distinction between the left and the right wave of the electron. And the linear mass term of the Dirac equation links these two parts of the wave. The suppression of the mass term was a necessity, justified from the physical point of view by the very small mass of the electron in comparison with the huge masses of W and 
11Many attempts have been made to describe by means of differential geometry the quantum world. Since the methods of differential geometry are local, they use easily infinitesimal transformations. And these infinitesimal transformations act in the Lie algebra of the studied group of transformations: it is then easy to do not see the difficulty: the group of the M and the group of the R are very different groups. The distinction between the rotation of the wave, with a q angle, and the rotation of the space, with a 2q angle, is compulsory to build a quantum theory including geometry.









