Journal of Modern Physics
Vol.4 No.9(2013), Article ID:37438,5 pages DOI:10.4236/jmp.2013.49163
Einstein’s Pseudo-Tensor in n Spatial Dimensions for Static Systems with Spherical Symmetry
P. O. Box 928211, San Diego, CA 92192, USA
Email: frtan96@gmail.com
Copyright © 2013 Frank R. Tangherlini. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Received May 25, 2013; revised June 27, 2013; accepted July 21, 2013
Keywords: Field Equations; Point Particle; Dimensionality of Space; Einstein’s Pseudo-Tensor
ABSTRACT
It was noted earlier that the general relativity field equations for static systems with spherical symmetry can be put into a linear form when the source energy density equals radial stress. These linear equations lead to a delta function energymomentum tensor for a point mass source for the Schwarzschild field that has vanishing self-stress, and whose integral therefore transforms properly under a Lorentz transformation, as though the particle is in the flat space-time of special relativity (SR). These findings were later extended to n spatial dimensions. Consistent with this SR-like result for the source tensor, Nordström and independently, Schrödinger, found for three spatial dimensions that the Einstein gravitational energy-momentum pseudo-tensor vanished in proper quasi-rectangular coordinates. The present work shows that this vanishing holds for the pseudo-tensor when extended to n spatial dimensions. Two additional consequences of this work are: 1) the dependency of the Einstein gravitational coupling constant κ on spatial dimensionality employed earlier is further justified; 2) the Tolman expression for the mass of a static, isolated system is generalized to take into account the dimensionality of space for n ≥ 3.
1. Introduction
Some years ago, the author [1] obtained Einstein’s field equations for static systems with spherical symmetry in n spatial dimensions, and one time dimension, which led to a generalization of Schwarzschild’s solution for three spatial dimensions. An earlier investigation by the author [2] had shown that for this symmetry with , and for a suitable choice of coordinates, when the field equations were suitably rewritten, they were linear. Other cases for which the field equations are linear are mentioned, but these fall outside the scope of this work. The linearity which will be dealt with here is associated with the fact that for the source tensor, energy density equals radial stress, i.e., in the notation to be employed below,
, and for a suitable choice of coordinates, when the field equations were suitably rewritten, they were linear. Other cases for which the field equations are linear are mentioned, but these fall outside the scope of this work. The linearity which will be dealt with here is associated with the fact that for the source tensor, energy density equals radial stress, i.e., in the notation to be employed below, 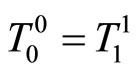 , a condition that is obviously satisfied for the homogenous solution. This linearity explains why the solution with an electrically charged source, the Reissner-Nordström solution, for which the Maxwell energymomentum tensor satisfies this condition, as well as the solution that includes the cosmological term, superimposes on the Schwarzschild solution for a point mass, so that for the standard line element (c = 1),
, a condition that is obviously satisfied for the homogenous solution. This linearity explains why the solution with an electrically charged source, the Reissner-Nordström solution, for which the Maxwell energymomentum tensor satisfies this condition, as well as the solution that includes the cosmological term, superimposes on the Schwarzschild solution for a point mass, so that for the standard line element (c = 1), 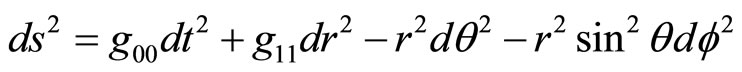 , one has
, one has 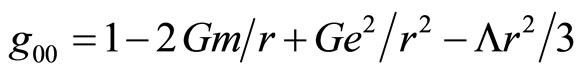 , with
, with . This remarkable linearity enabled the author to extrapolate to the origin to find the source tensor for a point mass in terms of the Dirac delta function. Thus, for the source tensor
. This remarkable linearity enabled the author to extrapolate to the origin to find the source tensor for a point mass in terms of the Dirac delta function. Thus, for the source tensor 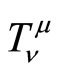 of a point particle source, one has
of a point particle source, one has
 (1)
(1)
where  is the radial delta function. This tensor has the important property that the trace of the spatial stresses vanishes,
is the radial delta function. This tensor has the important property that the trace of the spatial stresses vanishes, . (Latin indices run 1, 2, 3, and Einstein’s summation convention holds.) Because of this vanishing of the self-stress, see, e.g., Panofsky and Phillips [3], also Rohrlich [4], if one rewrites
. (Latin indices run 1, 2, 3, and Einstein’s summation convention holds.) Because of this vanishing of the self-stress, see, e.g., Panofsky and Phillips [3], also Rohrlich [4], if one rewrites 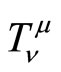 in terms of quasi-rectangular coordinates (this will be done in sect. 3 for n dimensions), then upon making a Lorentz transformation for a “boost” with velocity v, and integrating for the energy and momentum of the point particle, one finds
in terms of quasi-rectangular coordinates (this will be done in sect. 3 for n dimensions), then upon making a Lorentz transformation for a “boost” with velocity v, and integrating for the energy and momentum of the point particle, one finds , unlike the case for the classical electron based solely on the Maxwell energy-momentum tensor for which, as is well-known, the self-stress does not vanish, and one does not obtain the correct special relativistic values for the energy and momentum in the absence of compensating stresses.
, unlike the case for the classical electron based solely on the Maxwell energy-momentum tensor for which, as is well-known, the self-stress does not vanish, and one does not obtain the correct special relativistic values for the energy and momentum in the absence of compensating stresses.
The surprising consistency with special relativity (SR) of the delta function energy-momentum tensor for a point mass source for the Schwarzschild field raises the interesting question about the gravitational energy that might be associated with the solution. If one thinks of SR as the limiting case when the Einstein coupling constant  vanishes, where
vanishes, where 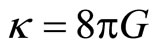 for three spatial dimensions, then it would seem reasonable that in this quasi-rectangular coordinate system, the Einstein gravitational energy-momentum pseudo-tensor should vanish for the Schwarzschild solution, if the analogy with SR continues to hold. This was indeed found to be the case by Nordström [5], and by Schrödinger [6], albeit without reference to SR. Nordström showed that the pseudo-tensor vanished for
for three spatial dimensions, then it would seem reasonable that in this quasi-rectangular coordinate system, the Einstein gravitational energy-momentum pseudo-tensor should vanish for the Schwarzschild solution, if the analogy with SR continues to hold. This was indeed found to be the case by Nordström [5], and by Schrödinger [6], albeit without reference to SR. Nordström showed that the pseudo-tensor vanished for , and explicitly showed that the pseudo-tensor energy density vanished for this case, while Schrödinger, in an independent analysis, found that all the components of the pseudo-tensor vanished. In an investigation of the Reissner-Nordström solution for a charged particle in [7], the author calculated the pseudotensor in the same quasi-rectangular coordinates for the more general case in which
, and explicitly showed that the pseudo-tensor energy density vanished for this case, while Schrödinger, in an independent analysis, found that all the components of the pseudo-tensor vanished. In an investigation of the Reissner-Nordström solution for a charged particle in [7], the author calculated the pseudotensor in the same quasi-rectangular coordinates for the more general case in which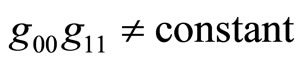 , and found
, and found
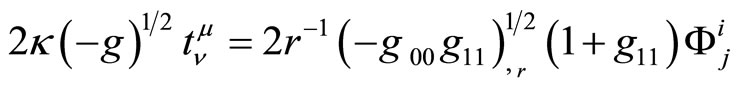 , (2)
, (2)
where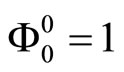 ,
,  ,
, 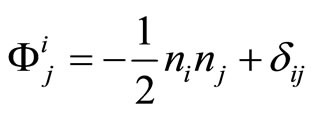 , where
, where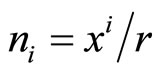 . Thus it is clear that the constancy of
. Thus it is clear that the constancy of 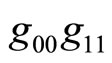 is sufficient for the pseudo-tensor to vanish in this quasirectangular coordinate system. It should be emphasized, however, that if one transforms to the quasi-rectangular coordinates associated with the isotropic form of the above line element, for which the spatial metric is conformally flat, the pseudo-tensor does not vanish, and in particular, outside the Schwarzschild event horizon, the energy density falls off as the fourth power of the radial coordinate, just as does the Maxwell tensor for a charged particle. However, a further discussion of this wellknown problem concerning the pseudo-tensor, is outside the scope of this work, and will be taken up in a later work.
is sufficient for the pseudo-tensor to vanish in this quasirectangular coordinate system. It should be emphasized, however, that if one transforms to the quasi-rectangular coordinates associated with the isotropic form of the above line element, for which the spatial metric is conformally flat, the pseudo-tensor does not vanish, and in particular, outside the Schwarzschild event horizon, the energy density falls off as the fourth power of the radial coordinate, just as does the Maxwell tensor for a charged particle. However, a further discussion of this wellknown problem concerning the pseudo-tensor, is outside the scope of this work, and will be taken up in a later work.
Returning then to the situation at hand, the question naturally arises as to whether or not this surprising SR behavior of the point mass tensor, associated with the vanishing of the self-stress and the vanishing of the Einstein pseudo-tensor, continues to hold after one extends the Schwarzschild solution to 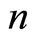 spatial dimensions? Thus, in Section 2, after recapitulation of the field equations for static systems with spherical symmetry for arbitrary n as given earlier [1], in which there will be a clarification of the form given there for Einstein’s gravitational coupling constant, i.e., its dependency on the dimensionality of space, and also there will be given a correction to one of the terms in the field equations that contained a typographical sign error, the n dimensional generalization of (1) will be given and discussed. In Section 3, the Einstein pseudo-tensor for n dimensions will be given, and the results will be shown to be consistent with the findings for three dimensions. In Section 4, there are concluding remarks.
spatial dimensions? Thus, in Section 2, after recapitulation of the field equations for static systems with spherical symmetry for arbitrary n as given earlier [1], in which there will be a clarification of the form given there for Einstein’s gravitational coupling constant, i.e., its dependency on the dimensionality of space, and also there will be given a correction to one of the terms in the field equations that contained a typographical sign error, the n dimensional generalization of (1) will be given and discussed. In Section 3, the Einstein pseudo-tensor for n dimensions will be given, and the results will be shown to be consistent with the findings for three dimensions. In Section 4, there are concluding remarks.
2. The Field Equations in n Spatial Dimensions
The field equations were given in [1], but are given here again for convenience, and because one of the field equations (here individually numbered) contained a sign error of typographical origin, and is given here in corrected form. The line element, with a change in notation for the angles, is given by
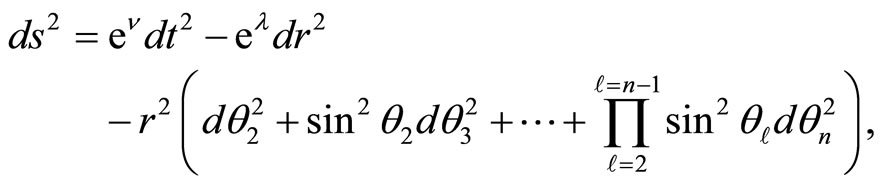 (3)
(3)
where  and
and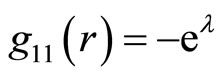 . After a standard, albeit lengthy, calculation for the field equations in mixed form,
. After a standard, albeit lengthy, calculation for the field equations in mixed form, 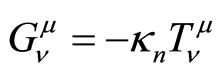 , where the form for
, where the form for 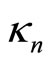 is irrelevant at this point, and will be discussed later below, the equations reduce to
is irrelevant at this point, and will be discussed later below, the equations reduce to
 , (4)
, (4)
 , (5)
, (5)
 (6)
(6)
and 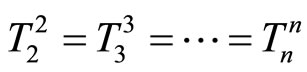 because of spherical symmetry. In [1], in the third equation of the group labeled (3.3), there was a plus sign before the fifth term in the square parentheses instead of a minus sign, given now correctly above as
because of spherical symmetry. In [1], in the third equation of the group labeled (3.3), there was a plus sign before the fifth term in the square parentheses instead of a minus sign, given now correctly above as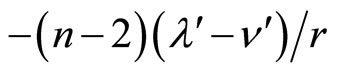 . Upon setting
. Upon setting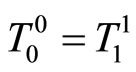 , as is trivially the case for the vacuum, it follows that
, as is trivially the case for the vacuum, it follows that , from which, for suitable normalization assuming asymptotic flatness, one has
, from which, for suitable normalization assuming asymptotic flatness, one has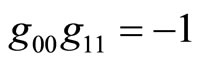 . Then, upon setting
. Then, upon setting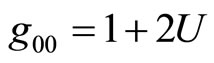 , Equations (4)-(6) reduce to the following two linear equations
, Equations (4)-(6) reduce to the following two linear equations
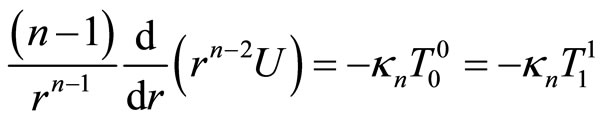 , (7)
, (7)

 , (8)
, (8)
Where 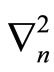 is the radial LaPlacian for n dimensions,
is the radial LaPlacian for n dimensions,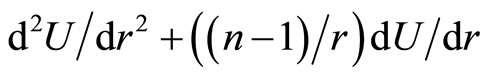 . The stress equilibriumequation that follows from the contracted Bianchi identities, and physically from the covariant energy-momentum conservation law, for the static case under consideration (i.e., with
. The stress equilibriumequation that follows from the contracted Bianchi identities, and physically from the covariant energy-momentum conservation law, for the static case under consideration (i.e., with ) is given by
) is given by
 . (9)
. (9)
In [1] it was assumed that
 , (10)
, (10)
where  is the total solid angle, or area of the unit sphere, in n spatial dimensions. However, the factor
is the total solid angle, or area of the unit sphere, in n spatial dimensions. However, the factor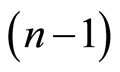 ) was introduced solely on the basis that the left hand side of the field equations for
) was introduced solely on the basis that the left hand side of the field equations for  and
and  vanished for
vanished for , and it seemed reasonable that the right hand side, proportional to
, and it seemed reasonable that the right hand side, proportional to  and
and  respectively, should vanish as well. Further, it was noted that combining the factor
respectively, should vanish as well. Further, it was noted that combining the factor 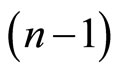 for
for 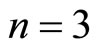 with
with  gives the correct Einstein numerical factor of
gives the correct Einstein numerical factor of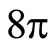 . More recently, it was recognized that instead of this somewhat ad hoc way of obtaining the factor (n − 1), there is an argument based on the signature difference for the metric tensor. Thus, choose coordinates such that the metric tensor is locally diagonalized, and the individual terms normalized to plus or minus unity. Let
. More recently, it was recognized that instead of this somewhat ad hoc way of obtaining the factor (n − 1), there is an argument based on the signature difference for the metric tensor. Thus, choose coordinates such that the metric tensor is locally diagonalized, and the individual terms normalized to plus or minus unity. Let 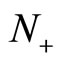 be the number of positive metric coefficients, and
be the number of positive metric coefficients, and  the number of negative metric coefficients, the signature difference is
the number of negative metric coefficients, the signature difference is . (Note, this is sometimes referred to as the signature itself.) For a time-like metric
. (Note, this is sometimes referred to as the signature itself.) For a time-like metric  yields
yields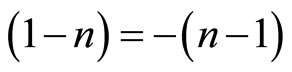 , and clearly for a space-like metric,
, and clearly for a space-like metric, 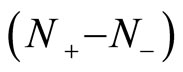 yields
yields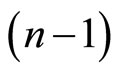 , and hence if the Einstein the field equations are written as
, and hence if the Einstein the field equations are written as
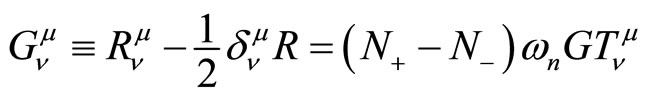 , (11)
, (11)
they hold in this form, not only for n spatial dimensions, but independently of whether or not the metric is spacelike, or time-like; the minus sign that appears, or doesn’t appear, on the right hand side is automatically taken care of by this signature-dependent form of the coupling constant. Thus (11) suggests that instead of the definition of  in (10), it is preferable to define it as
in (10), it is preferable to define it as 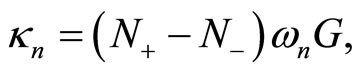 but it will not be used here. Further discussion is in the concluding section.
but it will not be used here. Further discussion is in the concluding section.
It is readily shown that the solution to the homogeneous Equations (7) and (8) for 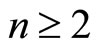 is
is
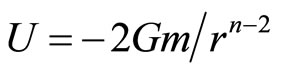 , as given in [1], so that
, as given in [1], so that
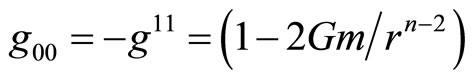 . To obtain the delta function expression for the
. To obtain the delta function expression for the 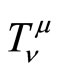 of a point particle, it is convenient to introduce the step function
of a point particle, it is convenient to introduce the step function 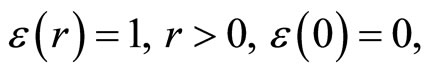 and set
and set 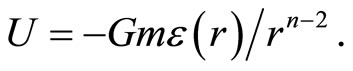 Then from Equation (7) one has
Then from Equation (7) one has
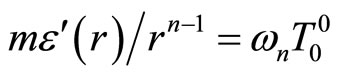 , (12)
, (12)
and since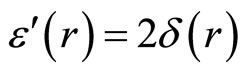 , one has
, one has 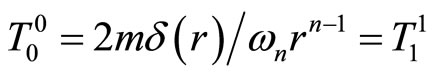 . To obtain,
. To obtain, 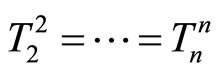 , it is convenient to use (9) and
, it is convenient to use (9) and 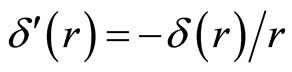 to obtain
to obtain 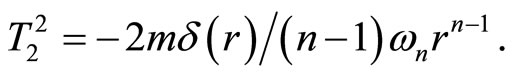 Hence the energy-momentum tensor for the point mass in
Hence the energy-momentum tensor for the point mass in  dimensions,
dimensions, 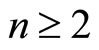 , is given by
, is given by
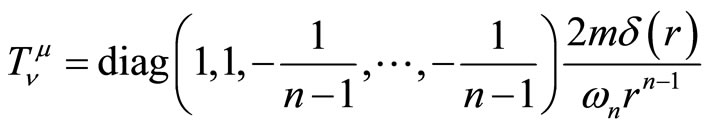 , (13)
, (13)
as obtained in [1], albeit with 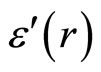 used there replaced with
used there replaced with 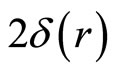 here. As was true for the
here. As was true for the  case, the trace of the spatial stresses vanishes,
case, the trace of the spatial stresses vanishes,
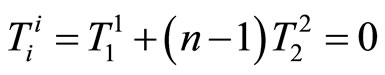 . Upon transforming to quasi-rectangular coordinates,
. Upon transforming to quasi-rectangular coordinates,  , the energy-momentum tensor takes form
, the energy-momentum tensor takes form
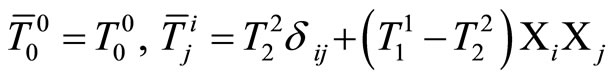 , (14)
, (14)
here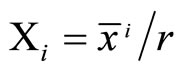 , and
, and 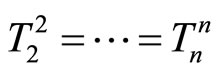 has been used. Since
has been used. Since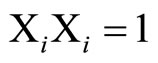 , the trace of the spatial stresses in the quasirectangular coordinates is given by
, the trace of the spatial stresses in the quasirectangular coordinates is given by 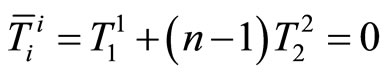 , as must be the case since the spatial trace is invariant under purely spatial transformations. Thus, not only in three spatial dimensions, but in
, as must be the case since the spatial trace is invariant under purely spatial transformations. Thus, not only in three spatial dimensions, but in 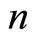 spatial dimensions, the energy-momentum tensor of the point particle source for the generalized Schwarzschild field transforms properly under a pure Lorentz transformation to yield the correct SR energy-momentum relations for a particle of mass m. However, the question arises as to whether the Einstein pseudo-tensor continues to vanish for
spatial dimensions, the energy-momentum tensor of the point particle source for the generalized Schwarzschild field transforms properly under a pure Lorentz transformation to yield the correct SR energy-momentum relations for a particle of mass m. However, the question arises as to whether the Einstein pseudo-tensor continues to vanish for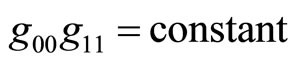 ? This will be shown to be the case in the next section.
? This will be shown to be the case in the next section.
3. Einstein’s Pseudo-Tensor in n Spatial Dimensions
The line element is that in (3), and it is necessary to convert it to quasi-rectangular coordinates to obtain the appropriate metric tensor to use in calculating the pseudotensor. This is most simply accomplished by rewriting 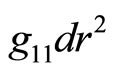 as
as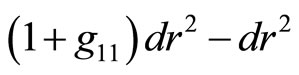 , and then combining the
, and then combining the 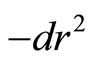 term with the angular terms to give the contribution to the spatial metric as
term with the angular terms to give the contribution to the spatial metric as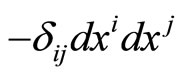 , where the bars over the spatial coordinates have been dropped, so that at this point the spatial line element is
, where the bars over the spatial coordinates have been dropped, so that at this point the spatial line element is  . Then, using
. Then, using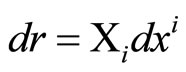 , so that
, so that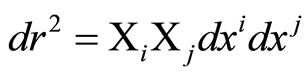 , and setting
, and setting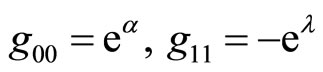 , the line element takes the form
, the line element takes the form
 , (15)
, (15)
and therefore the spatial metric is 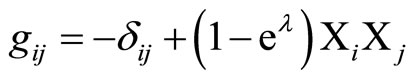 . The contravariant spatial metric is readily found to be
. The contravariant spatial metric is readily found to be , and likewise it is readily shown that in this new coordinate system
, and likewise it is readily shown that in this new coordinate system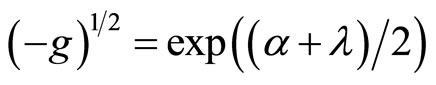 . The Einstein pseudotensor is given by
. The Einstein pseudotensor is given by
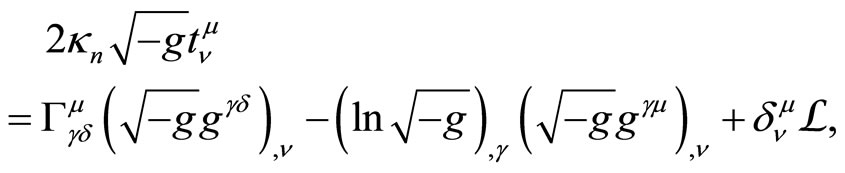 (16)
(16)
where  is
is  times the Lagrangian density, and is given by
times the Lagrangian density, and is given by
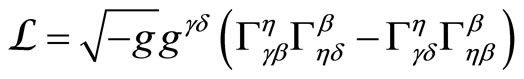 . (17)
. (17)
The Christoffel symbols of the second kind for the metric associated with (15) are
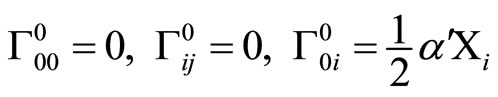 , (18)
, (18)
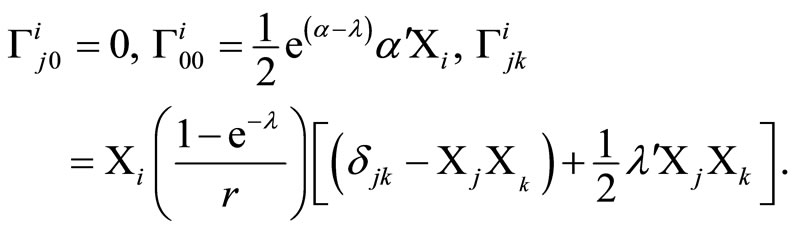 (19)
(19)
Also, from (18) and (19),
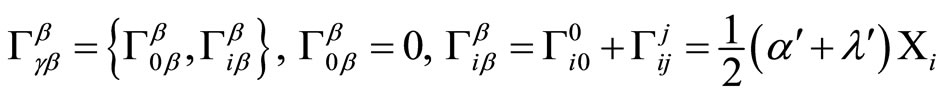 .
.
This also follows directly using . Because the system is static, and time derivatives as well as terms in
. Because the system is static, and time derivatives as well as terms in 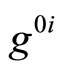 vanish, the gravitational energy density is proportional to
vanish, the gravitational energy density is proportional to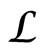 . Hence upon carrying out the analysis, it follows that
. Hence upon carrying out the analysis, it follows that
 . (20)
. (20)
Note that the right hand side vanishes for , consistent with the fact that both
, consistent with the fact that both 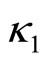 and (4) and (5) vanish. Since
and (4) and (5) vanish. Since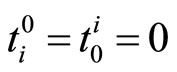 , the only remaining terms left are the stresses
, the only remaining terms left are the stresses 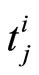 which from (16) can be written as a sum of two terms,
which from (16) can be written as a sum of two terms, 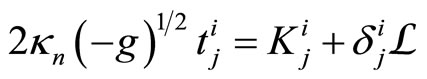 , and again, carrying out the analysis, it follows that
, and again, carrying out the analysis, it follows that
 , (21)
, (21)
which upon combining with 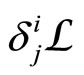 yields finally
yields finally
 (22)
(22)
It should be noted that the right hand side, although it does not explicitly exhibit the quantity , nevertheless vanishes for
, nevertheless vanishes for , since under these circumstances there is only
, since under these circumstances there is only 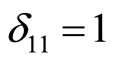 and
and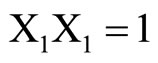 , and hence the term in parentheses involving the difference of these two quantities vanishes. Also if one takes the trace,
, and hence the term in parentheses involving the difference of these two quantities vanishes. Also if one takes the trace, 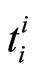 , then one has
, then one has , so in this case the factor appears explicitly. Moreover, since there is spherical symmetry, the diagonal terms
, so in this case the factor appears explicitly. Moreover, since there is spherical symmetry, the diagonal terms 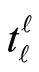 (no sum) are
(no sum) are  times the trace, and since there are no off-diagonal terms for
times the trace, and since there are no off-diagonal terms for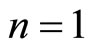 , the vanishing for
, the vanishing for 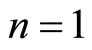 follows. Since both
follows. Since both  and
and 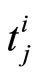 are proportional to
are proportional to  the pseudo-tensor vanishes for
the pseudo-tensor vanishes for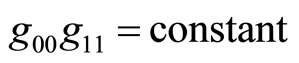 , so that the findings of Nordström [5] and Schrödinger [6] continue to hold in n dimensions. Thus the tensor for the point mass in n dimensions not only transforms properly under a Lorentz transformation, but, in this coordinate system, it is unaccompanied by gravitational energy and momentum, as if it were in the flat space-time of SR; indeed, since one can obviously set
, so that the findings of Nordström [5] and Schrödinger [6] continue to hold in n dimensions. Thus the tensor for the point mass in n dimensions not only transforms properly under a Lorentz transformation, but, in this coordinate system, it is unaccompanied by gravitational energy and momentum, as if it were in the flat space-time of SR; indeed, since one can obviously set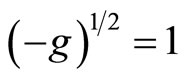 , the space- time volume element is simply
, the space- time volume element is simply  as in SR. Regrettably, the underlying physical reason for these surprising results is still unknown.
as in SR. Regrettably, the underlying physical reason for these surprising results is still unknown.
There is an interesting property of the Einstein pseudotensor for static systems that was found by Papapetrou [8] for the case n = 3, and is known as Papapetrou’s identity. He found that 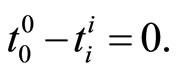 In a study of the source of the Schwarzschild field. the author [9] observed, using Euler’s theorem, and the general form for the pseudo-tensor from the action principle, that the trace of the spatial stresses, for a static system, in the notation of this paper, satisfies
In a study of the source of the Schwarzschild field. the author [9] observed, using Euler’s theorem, and the general form for the pseudo-tensor from the action principle, that the trace of the spatial stresses, for a static system, in the notation of this paper, satisfies
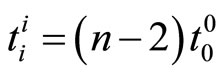 , (23)
, (23)
and observed that therefore the Papapetrou identity only holds in three spatial dimensions. Examination of (20) and (22) show that they obey (23), and this agreement can be seen as a check of the calculations leading to these two equations. The generalization of the Papapetrou identity given in (23), together with other assumptions given below, leads to a generalization of the Tolman [10] equations for the mass of an isolated static system. Thus, for n ≥ 3, one has
 (24)
(24)
where the last integral has made use of the fact that since
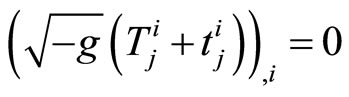 , upon multiplication by
, upon multiplication by , and the use of the divergence theorem, together with sufficient fall-off of the stresses so that the surface integral of
, and the use of the divergence theorem, together with sufficient fall-off of the stresses so that the surface integral of
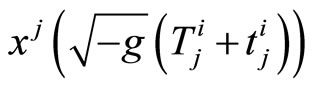 vanishes, at infinity, one has that
vanishes, at infinity, one has that
 .
.
4. Concluding Remarks
The primary purpose of this work was to investigate whether the Einstein pseudo-tensor continued to vanish in n spatial dimensions as it had for 3 dimensions for  As the above work shows, this is indeed the case, so that the vanishing is invariant with respect to a change of spatial dimensionality. Since this condition on the metric tensor via the field equations is directly tied to the requirement on the energy-momentum tensor that
As the above work shows, this is indeed the case, so that the vanishing is invariant with respect to a change of spatial dimensionality. Since this condition on the metric tensor via the field equations is directly tied to the requirement on the energy-momentum tensor that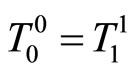 , there is obviously a physical reason underlying this influence on the metric tensor, as well as the resulting linearity of the field equations which continues to hold in n dimensions. However, as emphasized above, there is no known physical principle that leads one to expect these results. Equally interesting is the fact that when one transforms to isotropic coordinates, one finds that the pseudo-tensor does not vanish for
, there is obviously a physical reason underlying this influence on the metric tensor, as well as the resulting linearity of the field equations which continues to hold in n dimensions. However, as emphasized above, there is no known physical principle that leads one to expect these results. Equally interesting is the fact that when one transforms to isotropic coordinates, one finds that the pseudo-tensor does not vanish for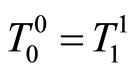 , and, as is well-known, no longer does g00g11 = constant for the Schwarzschild solution. A further study is in preparation in which the pseudo-tensor for isotropic coordinates in n spatial dimensions will be given, and compared with the results obtained in the coordinate system used here. Finally, it is of interest that the work strongly suggests that when one goes outside three spatial dimensions, one should allow for a dimensionality dependence of Einstein’s coupling constant, since the standard value of the constant,
, and, as is well-known, no longer does g00g11 = constant for the Schwarzschild solution. A further study is in preparation in which the pseudo-tensor for isotropic coordinates in n spatial dimensions will be given, and compared with the results obtained in the coordinate system used here. Finally, it is of interest that the work strongly suggests that when one goes outside three spatial dimensions, one should allow for a dimensionality dependence of Einstein’s coupling constant, since the standard value of the constant, 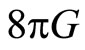 , is obtained to insure agreement with Newtonian theory in the limit of weak fields for
, is obtained to insure agreement with Newtonian theory in the limit of weak fields for . Interestingly, the proposed behavior of the coupling constant in this work impacts on string theory, since the latter, in later models, leads to a spatial dimensionality of
. Interestingly, the proposed behavior of the coupling constant in this work impacts on string theory, since the latter, in later models, leads to a spatial dimensionality of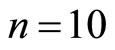 , and so naturally the question arises—how does this higher dimensionality influence the gravitational coupling constant for this theory? An answer to this question could facilitate a comparison of string theory with general relativity for higher dimensions, as well as three dimensions. Likewise to be compared are the energy-momentum tensors for gravitation in the two theories, bearing in mind that a spin-two theory would seem to predict a true tensor, whereas in accordance with the principle of general covariance, and the demand for a true conservation law, general relativity predicts a pseudo-tensor.
, and so naturally the question arises—how does this higher dimensionality influence the gravitational coupling constant for this theory? An answer to this question could facilitate a comparison of string theory with general relativity for higher dimensions, as well as three dimensions. Likewise to be compared are the energy-momentum tensors for gravitation in the two theories, bearing in mind that a spin-two theory would seem to predict a true tensor, whereas in accordance with the principle of general covariance, and the demand for a true conservation law, general relativity predicts a pseudo-tensor.
Also, it will be noted that unlike string theory, Einstein’s field equations do not in themselves impose a restriction on the dimensionality of space, although to be sure, for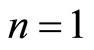 , according to (11), the gravitational coupling vanishes, while for n = 2 outside the source, the homogeneous solution indicates space-time is flat, and one therefore does not have the Newtonian logarithmic potential; for n ≥ 3, one has quasi-Newtonian behavior. The way n = 3 arrived at in [1] which was by requiring that there be stable bound orbits in the generalized Schwarzschild field. This approach was a follow-up to the study of the dimensionality of space problem by Ehrenfest [11,12] who studied the stability of orbits in Newtonian theory extended to n dimensions. Ehrenfest’s approach for n ≥ 3 was later arrived at independently by Whitrow [13,14], who justified the stability assumption by an appeal to the anthropic principle. Of interest to the present work is the fact that in [1], the pseudo-tensor is not involved, and so the question arises as to whether the behavior of the pseudo-tensor in n dimensions has bearing on the dimensionality of space problem? A possible affirmative answer to this question seems to be provided by (23) that shows that Papapetrou’s identity for static systems,
, according to (11), the gravitational coupling vanishes, while for n = 2 outside the source, the homogeneous solution indicates space-time is flat, and one therefore does not have the Newtonian logarithmic potential; for n ≥ 3, one has quasi-Newtonian behavior. The way n = 3 arrived at in [1] which was by requiring that there be stable bound orbits in the generalized Schwarzschild field. This approach was a follow-up to the study of the dimensionality of space problem by Ehrenfest [11,12] who studied the stability of orbits in Newtonian theory extended to n dimensions. Ehrenfest’s approach for n ≥ 3 was later arrived at independently by Whitrow [13,14], who justified the stability assumption by an appeal to the anthropic principle. Of interest to the present work is the fact that in [1], the pseudo-tensor is not involved, and so the question arises as to whether the behavior of the pseudo-tensor in n dimensions has bearing on the dimensionality of space problem? A possible affirmative answer to this question seems to be provided by (23) that shows that Papapetrou’s identity for static systems, 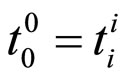 , only holds for n = 3, and hence Tolman’s expression for the mass of an isolated static system as well. Consequently, further studies of the behavior of Einstein’s pseudo-tensor may lead to another way to tackle the dimensionality of space problem in the framework of general relativity, and perhaps give insight as to how to involve the stability principle in a less ad hoc way.
, only holds for n = 3, and hence Tolman’s expression for the mass of an isolated static system as well. Consequently, further studies of the behavior of Einstein’s pseudo-tensor may lead to another way to tackle the dimensionality of space problem in the framework of general relativity, and perhaps give insight as to how to involve the stability principle in a less ad hoc way.
REFERENCES
- F. R. Tangherlini, Nuovo Cimento, Vol. 27, 1963, pp. 636-651.
- F. R. Tangherlini, Physical Review Letters, Vol. 6, 1961, pp. 147 -149.
- W. H. Panofsky and M. Phillips, “Classical Electricity and Magnetism,” 2nd Edition, Addison-Wesley, Reading, 1962.
- F. Rohrlich, “Classical Charged Particles,” 3rd Edition, World Scientific, Singapore, 2007.
- G. Nordström, Proceedings of Amsterdam Academy of Sciences, Vol. 20, 1918, pp. 1238-1245.
- E. Schrödinger, Physikalische Zeitschrift, Vol. 19, 1918, pp. 4-7.
- F. R. Tangherlini, Nuovo Cimento, Vol. 26, 1962, pp. 497-524.
- A. Papapetrou, Proceedings of the Royal Irish Academy, Vol. A51, 1947, pp. 191-204.
- F. R. Tangherlini, Nuovo Cimento, Vol. 38, 1965, pp. 153-171.
- R. C. Tolman, “Relativity, Thermodynamics, and Cosmology,” Clarendon Press, Oxford, 1934.
- P. Ehrenfest, Proceedings of the Amsterdam Academy of Sciences, Vol. 20, 1917, pp. 200-209.
- P. Ehrenfest, Annals of Physics (Leipzig), Vol. 61, 1920, pp. 440-446.
- G. J. Whitrow, British Journal for the Philosophy of Science, Vol. 6. 1955, pp. 13-31.
- G. J. Whitrow, “The Structure and Evolution of the Universe,” Harper Torchbooks, New York, 1959, pp. 199- 201.

