Received 25 February 2016; accepted 25 April 2016; published 28 April 2016

ABSTRACT

In this paper, based on classical Lie group method, we study multi-dimensional Landau-Lifshitz equation, and get its infinitesimal generator, symmetry group and new solutions. In particular, we build the connection between new exact solutions and old exact solutions. At the same time, we also prove that the initial boundary value condition of the three-dimensional Landau-Lifshitz equation admits a unique solution and discuss the stability of the solution.

Keywords:

Lie Group, Multidimensional Landau-Lifshitz Equation, Explicit Solutions, Stability

1. Introduction

In 1935, the famous Landau-Lifshitz equations were proposed by Landau and Lifshitz [1] to describe the evolution of spin fields in continuum ferromagnet [2] . In this paper we study two important equations as follows

(1)

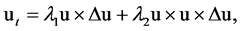(2)

where 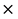denotes the vector cross-product in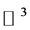, 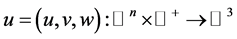is the spin density, 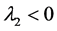is a damping parameter. Emphasizing its parabolic character, (2) can also be considered as a quasilinear pertur- bation of the heat flow for harmonic maps by the (conservative) precession term. The n-dimensional cylindrical symmetrical form of (1) is

(3)

where.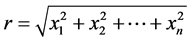

For the multidimensional case. Zhou and Guo proved the global existence of weak solution for the generalized Landau-Lifshitz equations at absence of Gilbert term [3] . Chang et al. considered the initial value problem for the 2-dimensional cylindrical symmetric Landau-Lifshitz equation without external magnetic field [4] . The soliton solutions to the Landau-Lifshitz equations with and without external magnetic field have been studied by many physicists and mathematicians [5] - [7] . For the Equation (3), when, Guo and Yang have constructed an exact solution in unit sphere [8] . In [9] and [10] , Guo and Han as well as Yang have also obtained an exact blow up solution for the n-dimensions form. In [11] and [12] , Yang considered the relations between (1) and (2).

It is of great importance to find exact solutions of Landau-Lifshitz equations. But it is difficult to solve Landau-Lifshitz equations. As is known, the symmetry group technique is one of the powerful tools for solving a nonlinear differential equation (see [13] - [22] ): the classical Lie group method [15] [16] , the non-classical Lie group method [17] [18] . Xu and Liu have studied n-dimensional radial symmetric Landau-Lifshitz equation with external magnetic field in [19] .

In this paper, the symmetry group of the n-dimensional Landau-Lifshitz equation is obtained by using the classical method in Section 2. The transformations leave the solutions invariant. In Section 3, we give the new solutions of Landau-Lifshitz equation from the known solutions [10] . Finally, the uniqueness and stability of the Landau-Lifshitz equation and the Landau-Lifshitz-Gilbert equation are given [20] , respectively, in Section 4 and 5.

2. Lie Symmetry Group of the Landau-Lifshitz Equation

Here are four independent variables being spatial coordinates and t the time, together with four dependent variables, the velocity field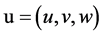. In vector notation, the system has the form

(4)

According to the method of determining the infinitesimal generator of nonlinear partial differential equation [16] , we take the infinitesimal generator of equation as follows:

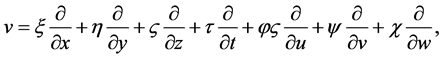
where 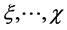are functions of, (1) is of second order. Applying the first prolongation, we find

Applying to (4), we find the following system of symmetry equations

(5)

which must be satisfied whenever u satisfy (1). Here, etc. are the coefficients of the first order

derivatives, etc. appearing in.

According to the formula, we have

Similarly, we can get.

we find the determining equations for the symmetry group of the (1) Equation (5) to be the following:

Since we have now satisfied all the determining equations, we conclude that most general infinitesimal symmetry of (1) has coefficient functions of the form:

where are arbitrary constants. Thus the Lie-algebra of infinitesimal of the Landau-Lifshitz equation is spanned by eight vector fields:

so we have

The one-parameter groups generated by the. The entries give the transformed point :

where is a Galiean transformation, are space translations, is a time translation. is an arbitrary constant.

Theorem 1. If are known solutions of (1), then by using the symmetry groups, so are the functions

where is any real number.

For the known solutions, by using one-parameter symmetry groups continuously, we can obtain a new solution which can be expressed as the following form:

where are arbitrary constants.

In vector notation, the system has the form

(6)

when in (2).

In view of the vector identities

,

Equation (6) can equivalently be written as

(7)

We transformed equations as follows:

(8)

Applying to (8), we find the following system of symmetry equations

(9)

Then use the same method, we can find most generated infinitesimal symmetry of (6) has coefficient functions of the form:

where are arbitrary constants. Thus the Lie-algebra of infinitesimal of the Landau-Lifshitz-Gilbert equation equation is spanned by seven vector fields:

So we have

where are space transformations, is a space translation, are Galiean translations, is an arbitrary constant.

Theorem 2. If are known solutions of (6), then by using the symmetry groups, so are the functions

where is any real number.

For the known solutions, by using one-parameter symmetry groups continuously, we can obtain a new solution which can be expressed as the following form:

where are arbitrary constants.

Remark If is a known solution, we can get is a new solution through Lie group method, where A is arbitrary constant orthogonal matrices.

3. Exact Solutions of the Landau-Lifshitz Equation

In this section, we choose the known blow-up solutions and explicit dynamic spherical cone symmetric solutions from [10] and [14] to get the relevant group invariant solutions.

According to [10] ,

(10)

has a blow-up solution:

(11)

where.

Case 1 Using in Theorem 1, we get the new blow-up solutions of (1) as follows:

(12)

where satisfying the following initial value:

(13)

Case 2 Using in Theorem 1, we get the new blow-up solutions of (1) as follows:

(14)

where satisfying the following initial value:

(15)

According to [10] ,

(16)

for, has a blow-up solution:

(17)

Case 3 Using in Theorem 1, we get the new blow-up solutions for the cylindrical symmetric Landau- Lifshitz Equation (3), typically as follows:

(18)

for, satisfying the following initial value:

(19)

According to [14] ,

(20)

has the explicit dynamic spherical cone symmetric solutions:

(21)

Case 4 Using in Theorem 1, we get the new explicit dynamic spherical cone symmetric solutions of (1) as follows:

(22)

satisfying the following initial value:

(23)

Case 5 Using in Theorem 1, we get the new explicit dynamic spherical cone symmetric solutions of (1) as follows:

(24)

satisfying the following initial value:

(25)

Remark Similarly, we can utilize the different seed solutions of [10] [14] , repeatedly using to obtain different group invariant solutions, so extend the known exact solutions in [9] [13] .

4. Uniqueness and Stability of the Landau-Lifshitz Equation

In this section, we study the uniqueness and stability of the initial boundary value problem for (1) and we have the following results and it should be results that matter instead.

4.1. Uniqueness

Theorem 3. There exists is a bounded domain and are nonzero constants. Then we the following initial value problem:

(26)

has a unique smooth blow-up solution.

Proof. To prove the uniqueness we consider two smooth solutions. Let their difference be, where. Then, subtracting the equations each other in (1), we have

(27)

Multiplying the first equation of (27) by, integrating over, and using the Gauss formula [23] , we obtain

(28)

(29)

for. By using,

, in case 1, we obtain

(30)

where.

(31)

Inserting (31) into (29), it follows that

(32)

Thanks to the Gronwall inequality [23] , we have the following:

therefore we can prove the uniqueness of the solution in the sense of.

In a similar way, by using

in case 3, we obtain

(33)

which.

(34)

Inserting (34) into (29), it follows that

(35)

Thanks to the Gronwall inequality, we have the following:

therefore we can get the uniqueness of the solution from this.

Theorem 4. There exists is a bounded domain. Then we the following initial boundary value problem:

(36)

has a unique explicit dynamic spherical cone symmetric solution

.

Proof. To prove the uniqueness we consider two solutions.

Let their difference be, where. Then, subtracting the equations each other in (1), we have

(37)

Multiplying the first equation of (37) by, integrating over, and using the Gauss formula, we obtain

(38)

(39)

for.

By using, in case

4, we obtain

(40)

where

(41)

Inserting (41) into (39), it follows that

(42)

Thanks to the Gronwall inequality, we have the following:

therefore we prove uniqueness of the solution in the sense of.

4.2. Stability

In this section we discuss the stability of the solution, in and for the problem (1), respec- tively.

Let is a solution of (1), where from case 1, denote

the solution of a little disturbance, where. Let, be the difference of, with initial value in the sense of and boundary value in the sense of. Then, subtracting one equation from the other, we can get

(43)

for is a bounded domain. Multiplying this by, integrating over, and using the Gauss formula, we obtain

(44)

(45)

(46)

(47)

By using,

in case 1, we obtain

(48)

Hence

(49)

since in the sense of, we can make, for every given

(50)

Using the Gronwall inequality in (44)-(47) for every:

as in the sense of

and. So we reach the stability of the solution in finite time.

In a similar way, by using

in case 3, and by using

in case 4, we can get the same conclusions.

5. Uniqueness and Stability of the Landau-Lifshitz-Gilbert Equation

Because of the Landau-Lifshitz-Gilbert equation in director fields with values in the unit sphere where typically, we find the Gilbert damping term, it would be easier and the stability of it has been done. We can see it in [20] . In this section, we study the uniqueness and stability of the initial boundary value problem for the Landau-Lifshitz-Gilbert equation below:

(51)

observe that, and we have the following results and it should be results that matter instead.

5.1. Uniqueness

Theorem 5. There exists is a bounded domain. Then we the following initial value problem:

(52)

has a unique smooth solution.

Proof. Let their difference be, where, ,. Then, subtracting the equations each other in (52), we have

(53)

we obtain that if are known solutions, we can get. Multiplying the first equation of (53) by, integrating over, and using the Gauss formula, we obtain

(54)

As

for.

Thanks to the Gronwall inequality, we have the following:

therefore we can prove the uniqueness of the solution in the sense of.

5.2. Stability

In this section we discuss the stability of the solution, in and for the problem (51), respec- tively.

Assume, where. Let denote the solution of a little disturbance, where. Let be different of, with initial value in the sense of and boundary value in the sense of . Then, subtracting one equation from the other, we can get

(55)

for is a bounded domain. Multiplying this by, integrating over, and using the Gauss formula, we obtain

(56)

since in the sense of, we can make, for every given:

where.

Thanks to the Gronwall inequality, we have the following:

therefore we prove the stability of the solution in the sense of.

6. Conclusion

In this paper, we study the symmetry reductions and explicit solutions by means of classical Lie group method. First, we get the infinitesimal generator and group invariant solutions to multidimensional Landau-Lifshitz equation. Then, we build the relations between new solutions and olds have been found. Finally, via these explicit solutions,we study the uniqueness and stability of initial-boundary problem on multidimensional Landau- Lifshitz equation.

Acknowledgements

This work was supported by the Natural Foundation of China (No. 11561076, No. 11101356).

Cite this paper

Jiali Yu,Fuzhi Li,Hui Yang,Ganshan Yang, (2016) Lie Group Classifications and Stability of Exact Solutions for Multidimensional Landau-Lifshitz Equations. Applied Mathematics,07,665-680. doi: 10.4236/am.2016.77061

References

NOTES

^*Corresponding author.