Journal of High Energy Physics, Gravitation and Cosmology
Vol.02 No.03(2016), Article ID:68057,18 pages
10.4236/jhepgc.2016.23032
Can Thermal Input from a Prior Universe Account for Relic Graviton Production? Implications for the Cosmological Landscape
Andrew W. Beckwith
Physics Department, College of Physics, Chongqing University, Huxi Campus, Chongqing, China

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 29 April 2016; accepted 4 July 2016; published 8 July 2016
ABSTRACT
The author presents how to make a link between the low temperature and low entropy of pre big bang state of cosmology as given by Carroll and Chen in 2005, to the quantum cosmology conditions predicted by Weinberg when the temperature reaches 1032 degrees Kelvin. We do this bridge building in our model construction as a way to get about the fact that cosmological CMB is limited by a red shift about z = 1100, so in order to get our suppositions consistent with observations, we also examine what happens in our model when we introduce quantization via a shift in values of the Hartle-Hawking wave function from a lower value of nearly zero to one which is set via an upper bound of the Planck’s constant of the order of 360 times the square of the Planck’s mass.
Keywords:
Relic Graviton Production, Cosmological Landscape

1. Preliminary Analogy with Regards to Seth Lloyds Universe as a Quantum Computer Paper
We begin by using the formula given by Seth Lloyd [1] in 2001 with respect to the number of operations the “Universe” can “compute” during its evolution. To begin with, we use formula
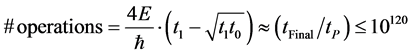 (1)
(1)
We assume that 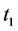 = final time of physical evolution, whereas
= final time of physical evolution, whereas 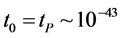 seconds and that we can set an energy input via assuming in early universe conditions that
seconds and that we can set an energy input via assuming in early universe conditions that 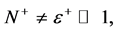 and
and 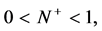
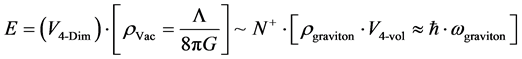 (2)
(2)
Furthermore, if we use the assumption that the temperature is close to 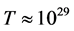 Kelvin initially, we have that if
Kelvin initially, we have that if
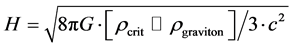 and
and 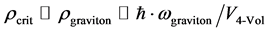 (3)
(3)
Then
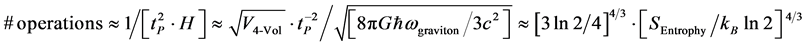 (4)
(4)
So, using the principle of maximum entropy, this leads to certain consequences with regards to the cosmological constant which changes in our model, and initial volume we will elaborate upon in the conclusion.
2. Introduction to Physical Inputs into This Problem
First of all we need to consider if there is an inherent fluctuation in early universe cosmology which is linked to a vacuum state nucleating out of “nothing”. The answer we have is yes and no.
The vacuum fluctuation leads to production of a dark energy density which we can state is initially due to contributions from an axion wall, which is dissolved during the inflationary era. What we will be doing is to reconcile how that wall was dissolved in early universe cosmology with quantum gravity models, brane world models, and Weinberg’s [2] published as of 1972 prediction of a threshold of 10 to the 32 power Kelvin for quantum effects becoming dominant in quantum gravity models. All of this is leading up to conditions in which we can expect relic graviton production which could account for the presence of strong gravitational fields in the onset of Guth style inflation, which would be in line with Penrose’s predictions via the Jeans inequality as to low temperature, low entropy conditions for pre inflationary cosmology.
It is noteworthy that Barvinsky et al. [3] in late 2006 recently predicted a range of values of four dimensional Planck’s constant values between upper and lower bounds, i.e. this is a way to incorporate the existence of a cosmological constant at about a Planck’s time  with the formation of scale factors which permit the existence of definable space time metrics. A good argument can be made that prior to Planck’s time
with the formation of scale factors which permit the existence of definable space time metrics. A good argument can be made that prior to Planck’s time 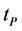 that conventional space time metrics, even those adapting to strongly curved space do not apply. Park et al. [4] predict an upper range of cosmological constant values far in excel of Barvinsky [3] , and we explain away the difference in terms of a thermal/vacuum energy input into graviton production. We will from now on investigate how this would affect the emergence of an initial state for the scale factor, in the cases where the cosmological constant is first a constant, and then where the cosmological constant parameter is initially far larger.
that conventional space time metrics, even those adapting to strongly curved space do not apply. Park et al. [4] predict an upper range of cosmological constant values far in excel of Barvinsky [3] , and we explain away the difference in terms of a thermal/vacuum energy input into graviton production. We will from now on investigate how this would affect the emergence of an initial state for the scale factor, in the cases where the cosmological constant is first a constant, and then where the cosmological constant parameter is initially far larger.
In order to do this, we are first going to examine how the Friedman equation gives us an evolution of the scale factor a(t), in two cases, Case one will be with a constant cosmological constant. And case two will be when the cosmological constant is far larger than it is today.
Making the case for an initially very, very large cosmological constant will lead to giving a road map into solving the land scape problem. i.e. [3] 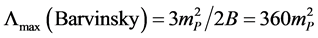 as a peak value, after graviton production would lead to after graviton production would lead to a Hartle-Hawking’s universe wave function of the form
as a peak value, after graviton production would lead to after graviton production would lead to a Hartle-Hawking’s universe wave function of the form
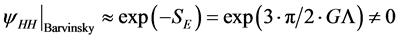 (5)
(5)
Whereas, having Parks [4] value for a nearly infinite cosmological constant parameter, due to high temperatures would lead to, prior to graviton production
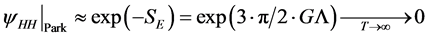 (6)
(6)
This would allow us to make inroads into a solution to the cosmological landscape problem discussed by Guth in 2003 [2] at the Kalvi institute in UC Santa Barbara, i.e. why have 101000 or so independent vacuum states as predicted by String theory?
3. Statement of the General Problem We Are Investigating
If one looks at the range of allowed upper bounds of the cosmological constant, we have that the difference between what Barvinsky [3] et al. in late 2006 recently predicted, and Park’s upper limit as of 2003, based upon thermal input is a giveaway that a phase transition is occurring at or before Planck’s time. This allows for a brief interlude of quintessence.
Begin with assuming that the absolute value of the five dimensional cosmological “constant” parameter is inversely related to temperature, i.e. [4]
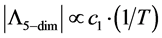
As opposed to working with the more traditional four dimensional version of the same, minus the minus sign of the brane world theory version [4]

We should note that this is assuming that a release in gravitons occurs which leads to a removal of graviton energy stored contributions to this cosmological parameter which is using a construction from [3]

Needless to say, right after the gravitons are released one still is seeing a drop off of temperature contributions to the cosmological constant. Then we can write, for small time values


We can do this for length (radii) values proportional to the value of the inverse of what the Hubble parameter is when the absolute value of five dimensional cosmological “constant” parameter is of the order of the four dimensional cosmological “constant” parameter, i.e. when the critical initial nucleation length we consider obeys

where initially we have temperatures of the order of 1.4 times 10 to the 32 power Kelvin as a thresh hold for the existence of quantum effects. This would pre suppose answering the issue raised by Weinberg [5] . As of 1972, he wrote that for quantum effects to be dominant in cosmology, with a value of critical energy we will use in setting a template for relic graviton production later on.

This is pre supposing that we have a working cosmology which actually gets to such temperatures at the instance of quantum nucleation of a new universe. Appendix IA. As accessed below gives us a working format as to the dynamics of quantum nucleation as outlined in this article. Appendix 1B which is in the same page gives commentary as to temperature dependence of the cosmological constant. Furthermore, Appendix II gives temperature dependence of four and five dimensional versions of a “cosmogical constant”. And if there is no temperature dependence, in the 5th dimensional cosmological constant se set as having magnitude

Also Appendix III states what we can expect from a difference in the upper limit of Park’s four dimensional inflation value for high temperatures, of the order of 10 to the 32 Kelvin, and the upper bound, Barvinsky [3] predicts, which if put into the Hartle-Hawking’s wave function is equivalent to a nucleation―quantization condition which we claim is a way to delineate a solution to the cosmic land scape problem Guth discussed in 2003. Appendix V focuses upon a frequency of relic graviton can be expected, and Also, Appendix IV focuses upon why there is a point back in time 





4. Weinberg Graviton Production Formula for Relic Gravitons
As is well known, a good statement about the number of gravitons per unit volume with frequencies between 



This formula predicts what was suggested earlier. A surge of gravitons commences due to a rapid change of temperature, i.e. if the original temperature were low, and then the temperature rapidly would heat up.
The hypothesis so presented is that input thermal energy given by the prior universe being inputted into an initial cavity/region dominated by an initially configured low temperature axion domain wall would be thermally excited to reach the regime of temperature excitation permitting an order of magnitude drop of axion density
from an initial temperature
5. Graviton Power Burst/Where Did the Missing Contributions to the Cosmological “Constant” Parameter Go?
To do this, we need to refer to a power spectrum value which can be associated with the emission of a graviton. Fortunately, the literature contains a working expression as to power generation for a graviton being produced for a rod spinning at a frequency per second


The point is though that we need to say something about the contribution of frequency needs to be understood as a mechanical analogue to the brute mechanics of graviton production. We can view the frequency 



And then one can set a normalized “energy input” as


The outcome is that there is a distinct power spike associated with Equation (9) and Equation (10), which is congruent with a relic graviton burst. Our next task will be to configure the conditions via brane world dynamics leading to graviton production.
Table 1. How to outline the existence of a relic graviton burst.
To build the Kaluza-Klein theory, one picks an invariant metric on the circle S1 that is the fiber of the U (1)-bundle of electromagnetism. This leads to construction of a two component scalar term with contributions of different signs. i.e. [10]

We should briefly note what an effective potential is in this situation [10] .
We get

This above system has a metastable vacuum for a given special value of


Part of the integrand in Equation (20) is known as an action integral [9] , 




And using the method [13] [14]

We should note that the quantity 
This requires,

So that one can make equivalence between the following statements. These need to be verified via serious analysis.


Proof of Claim: There is a way, for finite temperatures for defining a given four-dimensional cosmological constant. Check Appendix II below in working with these values, we should pay attention to how 
defined by Park, et al. [4] with 


that by [4]


6. Detection of Gravitons as Spin 2 Objects via Available Detector Systems?
First of all, we will state that in describing Gravitons, we are not precluding what t’Hooft said about a possibly necessary Deterministic foundation to quantum physics. The discussion so engendered is to help parse the likelihood that the conjecture in [15] has merit. But to do so, we should determine if the entire idea of.
To briefly review what we can say now about standard graviton detection schemes, as mentioned above, Rothman wrote Dyson [16] [17] doubts we will be able to detect gravitons via present detector technology. The conundrum is that if one defines the criterion for observing a graviton as [16]

Here,

This has 







We should state that we will generally be referring to a cross section which is frequently the size of the square of Planck’s length 


sions

volume ≈ 10 - 15 mm per side.
As stated in the manuscript, the problem then becomes determining a cross section 

7. Conclusions
So far, we have tried to reconcile the following.
First is that Brane world models will not permit Akshenkar’s quantum bounce [18] [19] . The quantum bounce idea is used to indicate how one can reconcile axion physics with the production of dark matter/dark energy, later on in the evolution of the inflationary era where one sees Guth style chaotic inflation for times 
In addition, we need to remember that references [20] [21] are predicting a lower temperature, and low initial entropy state prior to the big bang. This is a detail which needs to be reviewed so as to keep consistency in our cosmological model building. We need to not forget the radical nature of a build-up of temperature to the Kelvin temperature threshold of 1032 degrees Kelvin at the start of the big bang, and make certain that our bridge from pre big bang states, with presumably no quantum gravity, is not inconsistent with what happens at 1032 degrees Kelvin, which is mentioned as the onset of quantum gravity effects in cosmology. How does one ramp up to the high energy values greater than temperatures 1012 Kelvin during nucleosynthesis? The solution offered is novel and deserves further inquiry and investigation.
Thirdly is the issue of relic graviton production. How to observe it? The last section about limitations of graviton detectors, as opposed to gravity waves, points to the obvious problems. Current estimates speak of a detector system of the size of Jupiter to be able to detect a single graviton. This is patently absurd and needs to be addressed, likely by coupling graviton production with neutrino physics.
Now for suggestions as to future research. We are in this situation making reference to solve the cosmological “constant” problem without using G. Gurzadyan and She-Sheng Xue’s [22] approach which is fixed upon the scale factor 

One of our challenges ahead will be to link the onset of graviton production as indicated above, with the critical threshold energy, which we assert is a trigger for graviton production. In doing this we will be looking, maybe via the Sach-Wolfe effect, evidences for not only the higher frequency range of Graviton radiation, but also experimental evidence for the existence of short term quintessence, as outlined in Appendix VII in this paper. Furthermore, looking at Equation (4) of the first page, we would have a dramatically lowered value for a net range of graviton frequencies if the initial volume of space for graviton production is localized in the regime near the Planck time interval, i.e. we may need to, for information theory reasons go out to the 
We have the paradoxical result that we may need a huge influx of gravitons to give the initially low temperature, low entropy initial conditions given by J. Chen, and Sean Carroll in [20] [21] and the initially low temperature conditions changed by a gigantic cosmological constant, which after graviton production would lead to Barvinsky’s lower value of the maxim value of 360 times the square of Planck’s mass to give us an answer to the cosmological constant problem, i.e. The Hartle-Hawking wave function comes into being right AFTER graviton production, whereas it is zero initially beforehand. This would lead to a favored state as to the nucleation of the cosmological landscape due to changes in the cosmological constant at or before a Planck’s time interval, where we would have

Finally, the chaotic conditions given in Appendix IV argue for a signal/causal relationship discontinuity between a prior universe, and our present universe, as it is evolving.
Reference [23] is the traditional way to handle the matter of causal structure. We claim in our document that it is not guaranteed and that it is debatable (causal structure continuity).
So we study it and this also ties into the question if we have classical versus quantum conditions at the start of cosmological evolution.
In doing so, we need to also consider the possibility of getting more information about the quantum versus classical nature of (signal) waveforms representing gravitational waves from our work, and this in lieu of [24] which is due to binary black hole generation of GW at a frequency range of 35 to 250 Hz with a peak gravitational-wave strain of 1.0 × (10 to the −21 power). As stated by [24] the LIGO findings match the waveform predicted by general relativity for the in spiral and merger of a pair of black holes and the ring down of the resulting single black hole. We next need to build on our own techniques, to determine if the results of [24] are comparable with relic gravitational waves, and what this says as far as detection characteristics. In addition, the causal discontinuity as alluded to in, should be seen to be a way to delve into what Corda in [25] [26] brought up. i.e. if there is a third polarization of Gravitation, and if so do we need to examine Scalar-tensor theories of Gravity, or if GR is necessary and sufficient for explaining the observed in the early universe for the genesis of Gravitation. Note that [27] has also some considerations as to massive gravity which are intertwined with the issue brought up by Corda in [25] [26] .
Acknowledgements
This work is supported in part by National Nature Science Foundation of China grant No. 11375279.
Cite this paper
Andrew W. Beckwith, (2016) Can Thermal Input from a Prior Universe Account for Relic Graviton Production? Implications for the Cosmological Landscape. Journal of High Energy Physics, Gravitation and Cosmology,02,344-361. doi: 10.4236/jhepgc.2016.23032
References
- 1. Lloyd, S. (2001) Computational Capacity of the Universe. http://arxiv.org/abs/quant-ph/0110141
- 2. Weinberg, S. (1972) Gravitation and Cosmology: Principles and Applications of the General Theory of Relativity. John Wiley and Sons, Inc., New York.
- 3. Barvinsky, K. and Yu, A. (2006) Thermodynamics from Nothing: Limiting the Cosmological Constant Landscape. Physical Review D, 74, 121502.
- 4. Park., D.K., Kim, H. and Tamarayan, S. (2002) Nonvanishing Cosmological Constant of Flat Universe in Brane-World Senarios. http://arxiv.org/abs/hep-th/0111081
- 5. Guth, A.H. (2003) Eternal Inflation.
http://online.itp.ucsb.edu/online/strings_c03/guth/pdf/KITPGuth_2.pdf - 6. Wesson, P.S. (2000) Space-Time-Matter: Modern Kaluza-Klein Theory. World Press Scientific, P.t.e., Ltd., Singapore.
- 7. Brustein, R. and De Alwis, S.P. (1990) Non-Perturbative Divergence in Critical String Theory. Physics Letters B, 247, 31-35. http://dx.doi.org/10.1016/0370-2693(90)91044-C
- 8. Traschen, J. (1984) Causal Cosmological Perturbations and Implications for the Sachs-Wolfe Effect. Physical Review D, 29, 1563. http://dx.doi.org/10.1103/PhysRevD.29.1563
- 9. Fontana, G. (2005) Gravitational Wave Propulsion. In: El-Genk, M.S., Ed., CP746, Space Technology and Applications International Forum-STAIF, American Institute of Physics, Melville.
- 10. Sundrum, R., (2005) Extra Dimensions. SLAC Summer Institute: Gravity in the Quantum World and the Cosmos. http://www-conf.slac.stanford.edu/ssi/2005/lec_notes/Sundrum1/sundrum1.pdf
- 11. Buusso, R. and Randall, L. (2001) Holographic Domains of Anti-de Sitter Space.
https://arxiv.org/abs/hep-th/0112080 - 12. Lee, J. and Sorkin, R.D. (1988) Derivation of a Bogomolnyi Inequality In Five Dimensional Kaluza-Klein Theory. Communications in Mathematical Physics, 116, 353-364. http://dx.doi.org/10.1007/BF01229199
- 13. Zee, A. (2003) Quantum Field Theory in a Nutshell. Princeton University Press, Princeton, 279-280.
- 14. Beckwith, A.W. (2006) An Open Question: Are Topological Arguments Helpful In Setting Initial Conditions For Transport Problems in Condensed Matter Physics. Modern Physics Letters B, 20, 233-243.
http://dx.doi.org/10.1142/s0217984906010585 - 15. Hooft, G. (2002) Determinism beneath Quantum Mechanics.
http://arxiv.org/PS_cache/quant-ph/pdf/0212/0212095v1.pdf - 16. Rothman, T. and Boughn, S. (2006) Can Gravitons Be Detected? Foundations of Physics, 36, 1801-1825.
http://arxiv.org/abs/gr-qc/0601043 - 17. Dyson, F. (2012) Is a Graviton Detectable?
http://publications.ias.edu/sites/default/files/poincare2012.pdf - 18. Ashtekar, A., Pawlowski, T. and Singh, P. (2006) Quantum Nature of the Big Bang. Physical Review Letters, 96, 141301. http://dx.doi.org/10.1103/PhysRevLett.96.141301
- 19. Ashtekar, A., Pawlowski, T. and Singh, P. (2006) Quantum Nature of the Big Bang: An Analytical and Numerical Investigation. Physical Review D, 73, 124038. http://arxiv.org/abs/gr-qc/0604013
- 20. Carroll, S.M. and Chen, J. (2005) Does Inflation Provide Natural Initial Conditions for the Universe? International Journal of Modern Physics D, 14, 2335-2340. http://arxiv.org/abs/gr-qc/0505037
- 21. Carroll, S.M. and Chen, J. (2004) Spontaneous Inflation and the Origin of the Arrow of Time.
http://arxiv.org/abs/hep-th/0410270 - 22. Gurzadyan, G. and Xue, S.-S. (2003) On the Estimation of the Current Value of the Cosmological Constant. Modern Physics Letters A, 18, 561-568.
- 23. Dowker, F. (2005) Causal Sets and the Deep Structure of Space-Time.
http://arxiv.org/abs/gr-qc/0508109 - 24. Abbott, B.P., et al. (2016) Observation of Gravitational Waves from a Binary Black Hole Merger. Physical Review Letters, 116, 061102.
https://physics.aps.org/featured-article-pdf/10.1103/PhysRevLett.116.061102 - 25. Corda, C. (2008) Massive Gravitational Waves from the R2 Theory of Gravity: Production and Response of Interferometers. International Journal of Modern Physics A, 23, 1521-1535.
http://dx.doi.org/10.1142/S0218271809015904 - 26. Corda, C. (2009) Interferometric Detection of Gravitational Waves: The Definitive Test for General Relativity. International Journal of Modern Physics D, 18, 2275-2282.
http://arxiv.org/abs/0905.2502
http://dx.doi.org/10.1142/S0218271809015904 - 27. Beckwith, A. (2016) Gedanken Experiment for Fluctuation of Mass of a Graviton, Based on the Trace of GR Stress Energy Tensor-Pre Planckian Conditions that Lead to Gaining of Graviton Mass, and Planckian Conditions That Lead to Graviton Mass Shrinking to 10 -62 Grams. Journal of High Energy Physics, Gravitation and Cosmology, 2, 19-24. http://dx.doi.org/10.4236/jhepgc.2016.21002
- 28. Beckwith, A. (2016) Does a Randall-Sundrum Brane World Effective Potential Influence Axion Walls Helping to Form a Cosmological Constant Affecting Inflation? Journal of High Energy Physics, Gravitation and Cosmology, 2, 125-153. http://dx.doi.org/10.4236/jhepgc.2016.21013
- 29. Padmanabhan, T. (2005) 100 Years of Relativity, Space-Time Structure: Einstein and Beyond. World Scientific Publishing Co. Pte. Ltd., Singapore, 175-201.
- 30. Puthoff, H. (2009) Private Communications at the Institute of Advanced Study, in 2009, in Austin, Texas in a Visit to the Center, as a Guest of Dr. Eric Davis.
- 31. Dodelson, S. (2003) Modern Cosmology. Academic Press, Cambridge.
- 32. Chen, P. (1995) Resonant Photon-Graviton Conversion and Cosmic Microwave Background Fluctuations. Physical Review Letters, 74, 634-637. http://dx.doi.org/10.1103/PhysRevLett.74.634
Appendix IA: First Table with Respect to Phenomenology
Appendix IB: Second Table, What Can be Said about Cosmolgical Λ in 5 & 4 Dim
Appendix II: How to Define a Temperature Dependent Four and Five Dimensional Cosmological Constant Parameter
We define, via Park’s article [4]
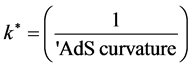
Park et al. note that if we have a “horizon” temperature term

We can define a quantity

Then there exists a relationship between a four-dimensional version of the

So

And set

This is important because of the datum that the defined an initial peak value of 



Appendix III: How a Difference in Values of the Cosmological Constant, as Given by Park et al. (2003) and Barvinsky et al. (2006) Implies Quantization of an Initial Vacuum State via Different Initial Conditions of the Hartle-Hawking Wave Function
In order to reference this argument, it is useful to note that Barvinsky [3] in late 2006 came up with an upper value of the cosmological constant as given by:

And a minimum value of

In contrast to the nearly infinite value of the Planck’s constant as given by Park et al. (2003).





As opposed to a minimum value of

When applied w.r.t. to the Hartle-Hawking wave function of [3]

We will observe having

As opposed to a nucleated value of

De facto, the dissolving of axion walls alluded to by when we have a thermal input with back ground temperatures at or greater than 


Is consistent with temperatures which are nearly infinite, which is alluded to by Barvinsky’s temperature scaling of [4]

Where we have a scaled Hubble value of

and Planck’s constant as given by

This correlates well with Carroll’s estimated value of a peak gravitational frequency value of , when Temerature [20] [21] is about 10 to the 32 Kelvin greater than or equal to the electro weak temperature



logical constant, as outlined.
Appendix IV: How the Evolution of the Scale Factor Is Influenced by Initial Choices of the “Cosmological Constant” Parameter at the Onset of Inflation
We will begin with an analysis of the Friedman equation and how the information about an alleged phase transition alluded to in Appendix IV above affects the evolution of the scale factor


With

And

This leads to a largely undefined statement about forming a consistent statement about scale expansion factors

involves a change of scale factor of the form [28] [29]

We take into account the axion type physics given in [28] but Equation (5) is straight from [29] .
The first hint of trouble can be seen when considering when the axion walls as mentioned in our model disappear,

But before that we need to understand if or not we can even define what happens if and when

This is in a largely instantaneous period of time. The guess we are offering is that this discontinuity, largely eliminates being able to analyze the evolution of a scale factor.
The two equations we are going to work with are as follows. First of all, we will consider what we can do with when we do not consider a constant 

This leads us to a highly non linear integral equation for evaluation of the scale factor as follows
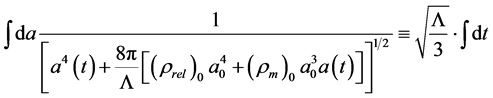
The second case we will consider will be what if we consider a constant negative 

We wish to note that in analyzing both Equation (9) above, and Equation (10) above we will be looking at different values of the cosmological factor











This means we can make the following approximations to Equation (9) above: If

Applying Equation (11) to the denominator, and integrating leads to the following polynomial expression for

We could go considerably higher in polynomial roots of Equation (12) above, depending upon the degree of accuracy we wished to obtain. This truncation so picked above is assuming a non infinite value of






If we go to Equation (9) above and assume small scale factor 
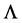

This allows us to write a value of the scale factor along the lines of solving the equation of state of

This leads to the following value of the scale factor, namely for times slightly greater than values at the order of Planck’s constant

What is interesting in this situation is that this evokes a time dependence which is not dissimilar to the situation with the radiation dominated era, except that it is roughly slightly more than the square of the radiation dominated era. It is now time to consider what if we are looking at a situation where we have.
Equation (10) gives a homogenous solution with the following behavior for sufficiently small time intervals. This assumes of course that time is or the order of Planck’s constant

For sufficiently large values of

This is a non linear equation for the scale factor, to put it mildly. If we have that


Leading to a value for the particular solution to Equation (10) for a scale factor for times in the neighborhood of the Planck constant value of

Needless to say this would be particularly relevant to the solution of the initial value of the Cosmological constant parameter, as given by Barvinsky [3] , i.e. that one would have for a maximum value of the cosmological constant greater than or equal to 316 times the value of the Planck mass
Appendix V: Forming the Fraction of a Critical Density, 
We first of all start off with an estimate for a stringy value of the red shift, taking into account the transition from radiation dominance (in early universe conditions) to matter dominance. This entails setting 




over frequency


In doing this, we also have to consider frequency ranges. The base line frequency range is set at about

This is for the 





This is for

As I have been told by Dr. Puthoff in person [30] , there is no reason why the value of the denominator in Equation (4) above could not be set at Planck’s frequency value of 


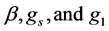



As well as

If 


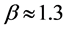


Appendix VI: Sach-Wolfe as a Function of CMB, and Gravitons Creating a CMB Profile
The enormous number of frequency values permitted for relic Graviton production leads up to measurable consequences for photonic related CMB production. We will attempt to delineate what these are and to also look at when the interaction of gravitons commenced with early universe conditions to initiate CMB. Sach-Wolfe effect considerations are a way of quantifying the onset of large scale anisotropic contributions to variations of temperature picked up by CMBR measurements, and we will discuss their interaction.
We shall start off with Padmanabhan’s temperature fluctuations of [29]

Here we have that the index of n so written is usually greater than or equal to 2 leading to, for

With this in mid we really need to consider the role which back ground density variations play in the evolution of photonic contributions to CMBR. If we have a contribution of graviton frequency values with usually a strict inequality, and often a situation where 

So we have a threshold of when we have comparatively low frequency contributions of when gravitons start to contribute to CMBR as writable up as, for a set of values of




If we have a situation where the ratio of graviton frequency as given by 
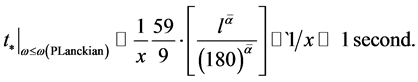
We can then for frequencies far larger than what we would expect for the typical values of the LISA experiment see

i.e. the beginning of interaction of the gravitons with early universe conditions would be at the onset of the inflationary era. Big surprise, NOT.
We should compare this set of estimates for more traditional bounds for the onset of nucleosynthesis in the early universe as given by Dodelson [31] in his book “Modern Cosmology”. As an example, we find that the onset of when deuterium and other light elements are created after the big bang can be predicted via, for

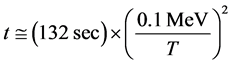
This also, after we go from a red shift of


In order to make sense of what Equation (5) and Equation (6) are telling us, we need to among other things consider initial energy density values which are relativistic in nature. Dodelson [31] writes a first order baryonic approximation of critical density values via considering the effective number of relativistic degrees of freedom 

This can be compared with Dodelson’s [31] critical energy value of about 

It is important to note that Pisen Chen [32] as of 1994 denoted the onset of graviton interaction with the generation of a huge gravitation surge with a relic magnetic field. His value of that magnetic field was 

What Pisen Chen gives us in his 1994 paper is a way to tie in the purported value of the relic magnetic field, as proportional to temperature fluctuations, as given by the following upper bound inequality. This is for the Planck “constant value” 



If the ratio of 


To get an idea of what this is saying, we can state that for large anisotropic variations in CMB we have for even strong initial magnetic fields a much larger value of the critical density of early space time matter-energy as given by the denominator of the left hand side of Equation (10) above at even the one minute or so mark for times after the big bang. This would lead to us asking how we could determine both the relic magnetic field 




Appendix VII: A Working Mathematical Model for Short Term Quintessence, up to a Planck Interval of Time
CASE I:
Temperature T very small, a.k.a. Carroll and Chen’s [20] [21] suppositions and time less than

CASE II:
Temperature T very large and time in the neighborhood of


We then get a general, and a particular solution with



CASE III:
Temperature T very large and time in the neighborhood of



Case IV:
Temperature T not necessarily large but on the way of becoming large valued, so the axion mass is not negligible, YET, and time in the neighborhood of


Then we obtain

This will lead to as the temperature rises we get that the general solution has definite character as follows

The upshot is, that for large, but shrinking axion mass contributions we have a cyclical oscillatory system, which breaks down and becomes a real field if the axion mass disappears. First of all, though, we have to understand how the conditions presented by S. Carroll, and J. Chen [20] [21] came about via brane theory. Our point is that we need brane theory to establish the initial starting point of reference for low temperature conditions at the onset of inflation.

















