Received 21 October 2015; accepted 9 January 2016; published 12 January 2016

ABSTRACT

We look at what may occur if Boltzmann equations, as presented by Murayama in 2007, Les Houches, are applied to graviton density in a pre-Planckian universe setting. Two restrictions are in order. First of all, we are assuming a graviton mass on the order of 10⁻⁶² grams, as if the pre- Planckian regime does not change the nature of Graviton mass, in its low end. Secondly, we are also assuming that a comparatively low temperature regime (far below the Planckian temperature) exists. Finally we are leaving unsaid what may happen if Gravitational waves enter the Planck regime of ultra-high temperature. With those three considerations, we proceed to examine a Graviton density value resulting from perturbation from low to higher temperatures. In the end an ultra- hot Pre big bang cosmology will yield essentially no early universe information transfer crossovers to our present cosmological system. This is not affected by the choice if we have a single repeating universe, or a multiverse. A cold pre inflationary state yields a very different situation. Initial frequencies of Gravitons, though, as outlined may be different in the multiverse case, as opposed to the single repeating universe case. We close with comments as to Bicep 2, and how this document has material as to how to avoid the BICEP 2 disaster. And about choosing between either the possibility of massless Scalar-Tensor Gravity as the correct theory of gravitation or conventional GR.

Keywords:

Boltzmann Equation, Stress Energy Tensor, Quantum Bounce, Infinite Quantum Statistics, Heavy Gravity, Third Polarization, Massless Scalar-Tensor Gravity

1. Introduction

We will start off first, with the result of H. Murayama [1] as to an equilibrium number density, of pre-Planckian physics, just before the Planckian physics regime. In this work we wish to allude to several restraints on the following presentation. First, we look at temperatures far below the Plank Temperature scale. Secondly the heavy gravity graviton mass, roughly 10⁻⁶² grams [2] is assumed to be invariant and not changing in spite of the transition from a cold pre-Planckian state, to right after the big bang. The author leaves, as a future investigation if the invariance of presumed graviton mass is defensible given a cold to a hot cosmology regime. An appendix entry is put as to presumed multiverse contributions to what is otherwise a single repeating universe, whereas we will be detailing the single repeating universe as our main result and the multiverse as a work in progress, an extension of the Gedanken experiment.

2. The Murayama Result, i.e. Equilibrium Number Density with Infinitesimal Values to the LHS of Equation (1) Below

We go on the assumptions, as follows that there are two givens as far as the initial thought experiment. First that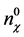 which is the initial numerical density of particle “flux” is almost the same as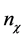, where 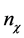is, most likely a graviton count, in the vicinity of a Planck time, and that then what we will be doing is to consider Murayama’s treatment of the Boltzmann equation.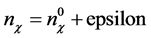. i.e. an infinitesimal amount in the pre-Planckian to Planckian space-time physics regime. If we consider that the 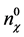as the number of relic graviton particles, the identification given above, as from 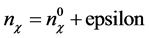is not so fantastic if the time interval is 1/2 a Plank interval of time to a full Planck interval of time. The existence of pre-Planckian generation for Gravitons was worked out by Beckwith, in [3] [4] .

A suggestion written as by a reviewer as to allegedly refocusing this paper’s theme and allegedly proper reworking, Suggested title may be something like: “On exact numerical solution of Boltzmann equation, and its implications to relic density and dark matter prediction.” was turned down by the author for several explicit reasons. First of all, while Murayama is focused on the applications to Dark Matter, we chose this formalism because it does refer to bosons, and a graviton is a boson, and secondly the DM creation meme is not even relevant at the sub Planckian to Planckian regime of space-time physics. According to even [1] [5] there was never any chance of DM production in the sub Plankian to Planckian regime of space-time, i.e. what Beckwith postulates in [3] [4] which is integral to this presentation is way before the formation of DM. Secondly that reviewer did not understand that the sole reason for the selection of the Boltzmann equations is for a bosonic particle and that the solution which is offered by Murayama which is what will be written up has the intriguing possibility of a temperature dependence which will come in play in terms of applications.

We will not reference cyclic universes in this document as a main result, but in terms of an appendix entry, which we view as timely and in the spirit of the offered thought experiment. So for the time being we will be not citing a cyclic multiverse universe as the main result, but as a future project in the works, with tentative suggestions outlined to that effect. See the appendix entry for this with a summary of findings.

Debatable as this may be, the assumption will be that what H. Murayama postulated in [1] in pre-Planckian physics is appropriate as a thought experiment. Murayama postulated that the conditions which exist are for light particles is a given, which would simplify a Boltzmann equation to have the form given by the first equation, with the following derivatives as far as answers.

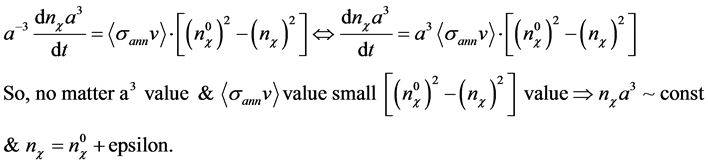(1)

Murayama also postulates [1] [3] that

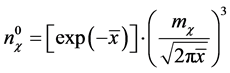(2)

The approximation we will use is that the right hand side of Equation (1) is almost zero, i.e.

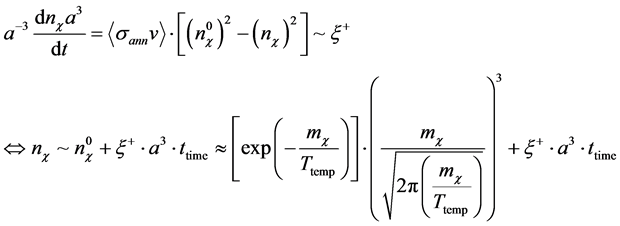(3)

Note that for the record, the 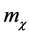here is a relic graviton mass, and that we are assuming a range of temperature variations, 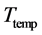in the pre-Planckian to Planckian time of cosmology, which will form a large part of the future discussion. Last but not least, the interplay between

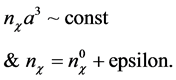(4)

Will in part mean a rich interplay between Equation (3) and the inverse behavior of a scale factor, cubed which we will comment upon fully in the later part of this text. From here, we will fill in some would be parameters

3. Looking at What Is a Range of Would Be Values to Insert in Equation (3) and Its Linkage to Equation (4)

First of all, assuming in Pre inflation mass (energy) and temperature a ratio of about, for what was given through [1] that in non-dimensionalized units

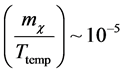(5)

This would imply for a pre-Planckian density, for a pre-Planckian bounce radii of one Planck length 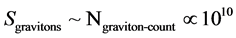[6]

(6)

4. Implications as Far as Single Repeating Universe

To start off with, we will be considering having [10]

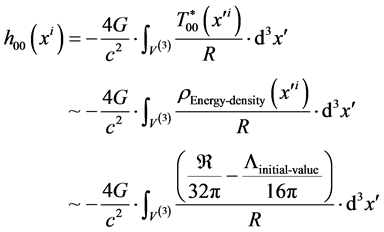(7)

The relevant quantity to consider here would be, then, with Equation (5), and [6]

(8)

(9)

This value for the initial time step would be probably lead to pre-Planckian time, i.e. smaller than 10^-43 seconds, which then leads us to consider, what would happen if a multi verse contributed to initial space-time conditions, then by [11] we would have a metric tensor contribution of for a single universe

Note that the values of E-V are discussed in Beckwith’s [1] [2] references, we leave this as an exercise for the reader. Beckwith has given specific details as to

(10)

Recall that as of [1] [2] there is a formulation, and the end result of that was that there was, in the pre-Planckian space-time a situation for which we had the mass of the graviton in the pre- Planckian regime would be 10⁻⁵⁵ grams, instead of the 10⁻⁶² grams as of the Planckian era, and also then, that due to the dominance of kinetic energy in the pre-Planckian space-time, that there would be a low value for the lower bound for the functional. We generalize this in Appendix A.

See Appendix A for the multiverse construction. We then will have, if we follow Penrose

(11)

We first will start at the Penrose hypothesis for a cyclic conformal universe, starting with [7] [8]

(12)

However, in the multiverse contribution to [8] which is tied into Appendix A

(13)

The end result in terms of GW frequencies to be parsed is

(14)

Please look at Appendix A as to details of how this second frequency is possible

5. Conclusion. Frequency and Wavelength Minimum for Pre-Planckian Gravitons. Importance of Comparing a Single Repeating Universe versus a Multiverse. And Temperature Influences

First of all we will consider frequency, of relic “gravitons”, and next we will consider the impact of temperature, starting off first with frequency as to single repeating universe, then a multiverse.

The conclusions of Equation (13) are such that if we look at the value of h, i.e. strain, as given by Tong, Zhang Zhao, Liu, Zhao, and Yang [12] , the following becomes a possibility, namely for a strain, one has an inversion of contributions from N contributing universes, in a multiverse generalization of the Penrose hypothesis, will lead to, if we look at from Appendix A in terms of a multiverse

(15)

In terms of the repeating single universe, we would have, say

(16)

In the case of Equations (15)-(16) there is no statistical averaging. It is the case of Equation (15) which we will be most concerned about. The contribution of the “strain”, in terms of the term for each of the N sub set universes (of the multiverse) contributions, j = 1 to N, of metric tensor fluctuations, as for each of the N universes, will mean that this fluctuation contribution is really almost a statistical averaging, since it is divided, by N after the N contributions of each conformal “resizing” is finalized. This Equation (14) should be compared with Equation (6) above, in terms of absolute magnitude, and will be, especially

if for every value of R, and used in Equation (6). That will be done

in the future, as far as research work initiated by the author to give more analogies along the line of [9] . With reference [10] giving us.

Distinguishing between Equation (15) and Equation (16) should be our next task. It is unlikely that if there was just one universe, that it would be identical to N universes, statistically averaged, since it would be incredibly unlikely for the same frequency to be the resonant frequency in question from N (maybe almost infinite number of ) universes.

Next, the main event. What does temperature have to do with this business A strict inspection of Equation (3) yields the startling result that the higher the temperature is, the lower the value of the LHS of Equation (3) is, i.e. a hot Pre big bang cosmology will yield essentially no early universe information transfer crossovers to our present cosmological system. This is not affected by the choice if we have a single repeating universe, or a multiverse, i.e. the main thing being that the number, n, is inversely proportional to the initial scale factor, cubed, a detail, which is due to the structure of pre-Planckian cosmology, which the author things is behind the datum that the initial lower mass of the graviton was higher in the pre-Planckian state, than immediately afterwards.

As to Equation (15) and Equation (16), this issue is more important, than people appreciate in ascertaining distinguishing between either multiple sources, or a single spatial origin for gravitational waves. This is in lieu as to C. VAN DEN BROECK in [13] namely that we need to distinguish between multiple early universe sources of gravitational waves, or the onset of inflation generated early gravitational waves, as seen in the quotation below

“Omni-directional gravitational wave background radiation could arise from fundamental processes in the early Universe, or from the superposition of a large number of signals with a point-like origin. Examples of the former include parametric amplification of gravitational vacuum fluctuations during the inflationary era”

Distinguishing between Equation (15) and Equation (16) must be done in a way as to avoid the conundrum mentioned above. The multiple frequencies, averaged out, as given in Equation (15) are not the same as parametric amplification of gravitational vacuum during the inflationary era, and refining data picked up by instrumentation as to avoid any overlap of such pre big bang gravitational wave frequency mixing with parametric amplification of vacuum fluctuations will be supremely challenging. This though is not the only issue at hand. In a recently accepted by JPEPHC publication, [14] the author writes

“It is worth noting that Dr. Corda in [15] has extended the Maggiorie results [18] as given in the prior reference [15] section and that indeed Maggiore studied the detectability only for GWs having a wavelength very much longer than the interferometer’s arms, while Corda [15] extended the results to all the GWs wavelengths. The importance of this contribution is, if we find out if there is a third polarization as indicated above, due possibly due to a dominance of kinetic energy”

We have in our situation that the third polarization issue may arise in Equation (15), for reasons as given in Dr. Corda’s reference according to [15] , whereas it is likely that Equation [16] will avoid third polarization issues. The third polarization issues is important due to what was cited in reference [14] , of reference [15] ’s cited paragraph

“Thus, if advanced projects on the detection of GWs will improve their sensitivity allowing to perform a GWs astronomy (this is due because signals from GWs are quite weak) [18] , one will only have to look the interferometer response functions to understand if General Relativity is the definitive theory of gravity. In fact, if only the two response functions (2) and (19) will be present, we will conclude that General Relativity is definitive. If the response function (22) will be present too, we will conclude that massless Scalar- Tensor Gravity is the correct theory of gravitation. Finally, if a longitudinal response function will be present, i.e. Equation (25) for a wave propagating parallel to one interferometer arm, or its generalization to angular dependences, we will learn that the correct theory of gravity will be massive Scalar-Tensor Gravity which is equivalent to f(R) theories. In any case, such response functions will represent the definitive test for General Relativity. This is because General Relativity is the only gravity theory which admits only the two response functions (2) and (19) [15] . Such response functions correspond to the two “canonical” polarizations h+ and h×. Thus, if a third polarization will be present, a third response function will be detected by GWs interferometers and this fact will rule out General Relativity like the definitive theory of gravity”

We are not done though, i.e. the final task is to avoid the Bicep 2 disaster. The overlay of dust as given in [16] and [17] cannot ever be repeated. In either Equation (15) and Equation (16) if one does not pick the relevant frequency range (ranges?) appropriately, we could re duplicate dust contamination. That is will require avoiding frequencies which are known to be commensurate to dust generation of gravitational waves. This is going to require serious data time work, especially if Equation (15) is the relevant pick, in this document. Doing so is mandatory if we wish to adhere to experimental work which has picked up relic gravitational waves. The current status of gravitational wave research, is seen by reference [13] in that pre inflationary physics may have to be considered as far as avoiding dust [16] [17] and the Bicep 2 disaster, which also is in tandem with our observation as to the over whelming current data preference, at the time as to GR, as the usually preferred cosmological theory. That we are looking at if or not [15] presages a third polarization, is in part due to [14] but even more to the choice given by Equation (15) and Equation (16) in which there may be the only real remaining competitor to GR. i.e. we are choosing between either the possibility of massless Scalar-Tensor Gravity as the correct theory of gravitation or conventional GR. Especially if there is a choice to be made as far as Equation (15) and Equation (16) in the present document. If Equation (16) is picked, this in conjunction with reference [14] increases the likelihood that GR must be accepted as the venue for GW physics modeling.

Acknowledgements

This work is supported in part by National Nature Science Foundation of China grant No. 11375279.

Cite this paper

Andrew WalcottBeckwith, (2016) Gedanken Experiment for Using Boltzmann Equation for Relic Graviton Frequencies, in Pre-Planckian Physics and the Independence of Relic Graviton Density from Either Single Repeating Universe Models or Multiverses. Journal of High Energy Physics, Gravitation and Cosmology,02,83-91. doi: 10.4236/jhepgc.2016.21009

References

Appendix A, In Terms of Contribution for Graviton Frequency by a Multiverse

But, then if one is looking at a multiverse, we first will start at the Penrose hypothesis for a cyclic conformal universe, starting with [7] [8]

(1A)

However, in the multiverse contribution to [8]

(2A)

So, does something like this hold? In a general sense? If N is the number of contributing multiverse single universes contributing to the beginning of space time, then we would be looking at [8]

(3A)

Then the contribution of the multiverse to the beginning

(4A)

And the graviton mass for a multiverse, would be then like

(5A)

Then for multiverse there would be an energy density looking like, for all this

(6A)

If so, there would then be a net Graviton based energy we would set up as given below which is a way to obtain, via a multiverse input to be as follows:

(7A)

of the following equation which we will then lead to setting the graviton frequency as following, with N the number of universe contributions to the new universe, and the frequency, as averaged out by

(8A)