Journal of Applied Mathematics and Physics
Vol.04 No.12(2016), Article ID:73127,7 pages
10.4236/jamp.2016.412217
Designing Sensors Using Nano-Junctions
Vijay K. Lamba1, O. P. Garg2
1Global College of Engineering & Technology, Khanpur Khui, India
2RKSD College, Kaithal, India

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: September 23, 2016; Accepted: December 26, 2016; Published: December 29, 2016
ABSTRACT
Nanowire field effect transistors can be modeled for ultrasensitive charge detection based bio- or chemical sensors. As critical dimensions of the nanowire sensor can be of the same order of size of biological molecules or chemical species yielding exceptional sensing possibilities. In addition, the large surface/volume ratio will give high sensitivities simply because surface effects dominate over bulk properties. Thus, we modeled Si nanowire with different geometries in the different chemical environment using NEGF approach. To analyze the performance, the sensitivity of Si nanowire with different cross sections including circular, rectangular, and triangular is derived by two definitions. It is calculated that the sensitivity of Si nanowire with different structures is a function of geometrical parameters and doping density. It is illustrated that the sensitivity varies inversely with cross-section area, doping density, and also the length of nanowire.
Keywords:
SINWs, Doping, NEGF, DFT

1. Introduction
In recent years, silicon nanostructures have attracted great interest as a building block for micro-electro-mechanical systems (MEMS), nano-electro-mechanical systems (NEMS) and nano-electronic devices. For example, silicon nanostructures find applications in diverse areas such as sensors, bio-sensors, medical technology, and communication technologies [1] [2] . Developing accurate and efficient models to predict the material properties of silicon nanostructures plays an important role in the design, characterization, and optimization of MEMS/NEMS and nano-electronic devices [3] [4] [5] [6] . Since the typical dimension of silicon nanostructures can vary from a few nanometers to several hundred nanometers or even micrometers, the development of an appropriate model to accurately and efficiently predict the mechanical, and electrical response due to the external loadings and morphologies at different length scales is one of the main issues in a full device simulation of nanostructures.
Atomistic simulation methods such as first-principles quantum-mechanical methods [7] [8] , molecular dynamics (MD) and Monte Carlo (MC) simulations [9] [10] are generally accurate for the analysis of nanostructures. However, the extremely high computational cost prohibits the application of the atomistic methods at the device level. On the other hand, classical continuum theories which are based on continuum assumptions are efficient and accurate at macroscopic scale, but they may not be directly applicable for devices with nanometer features. To achieve the goal of accurately capturing the atomistic physics and yet retaining the efficiency at various length scales, multi-scale modeling and simulation techniques have recently gained significant interest. So we used NEGF theory for Si nanowire with different geometries in the different chemical environment to analyze the performance, the sensitivity of Si nanowire with different cross sections.
In Section 2, we discuss the system model we used; Section 3 consists of NEGF-DFT formalism; in Section 4, we model the effect of encapsulation, & deformed structures, with results & conclusions in Section 5.
2. Model System
A general model for detecting molecules using Si-NWs is shown in Figure 1. The system consists of a Si-NW between two electrodes whose surface is functionalized. The electrodes are protected from the external environment by an oxide layer to avoid any undesired conductance change due to modification of electrode work function.
Due to the presence of native oxide on the NW surface, we assume that the negligible charge transfer is expected to take place between the molecule and the semiconductor. However, it is reported in [11] that the complimentary change in conductance for P-type and N-type doped NWs due to same organic molecules, which indicates that electrostatic interaction dominates the response. In this paper, we consider the analysis of sensitivity of Si nanowire with different cross sections only and neglect the effect of any surface states, as the response of a sensor is characterized in terms of its selectivity, settling time, and sensitivity.


Figure 1.(a) Schematic of SiNW model, (b) view of SiNW with dopant in virtual NanoLab.
Selectivity denotes the ability of receptors to bind with the desired target in the presence of various other (possibly similar) molecules and is entirely determined by the functionalization schemes [12] . The time taken by the sensor to produce a stable signal change defines the settling time, and is determined by molecule concentration, diffusion coefficients, and conjugation affinity to the receptor molecules [13] . Finally, sensitivity corresponds to the relative change in sensor characteristics upon attachment of target molecules on nanowire surface, which can be determined by the electrostatics of the system.
3. Theoretical Approach
The device model applied in the transport calculation consists of three parts, the studied material and two electrodes under bias Vb. Thus the Hamiltonian H for full systems can be of the form:
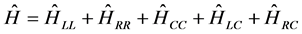 (1)
(1)
were HLL/RR are the Hamiltonian for left/right electrode and HCC + HLC + HRC gives Hamiltonian for extended molecule, consisting of molecule in addition to three layer of surface atoms of two electrodes. Here, each term is represented as
 (2)
(2)
were 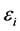 represents site energy of electron/hole positioned on I molecule, and
represents site energy of electron/hole positioned on I molecule, and 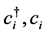 symbolize the creation and annihilation operator. As the definition of the current from the left electrode to the pinned system is
symbolize the creation and annihilation operator. As the definition of the current from the left electrode to the pinned system is
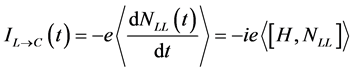 (3)
(3)
Thus using
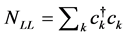 (4)
(4)
we get
 (5)
(5)
Combining green function we get
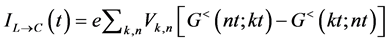 (6)
(6)
Here 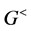 stands for retarded green function defined as
stands for retarded green function defined as
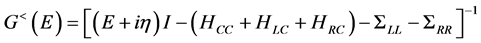
were 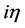 and
and  are an infinitesimal imaginary value and self energy elements which includes influence of electrodes. For the steady state
are an infinitesimal imaginary value and self energy elements which includes influence of electrodes. For the steady state
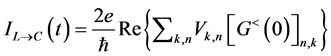 . (7)
. (7)
Similarly we calculate for ILL, IRR, ICC, ICR, etc.
Thus
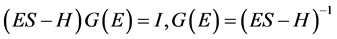 (8)
(8)
Here S is the overlap matrix and I stands for identity operator. We describe overlap matrix is close to identity matrix, thus matrix calculation
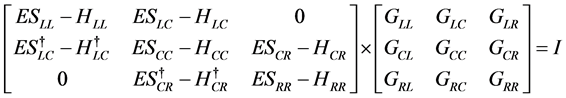 (9)
(9)
The solution of GCC is
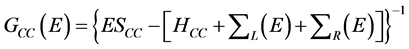 (10)
(10)
where, 



Tr means the trace analyzed. Thus using above equation we get transmission function of the systems. The electron transport calculations are performed using NEGF combined with DFT within the Landauer formalism [14] implemented in ATOMISTIX TOOLKIT [15] . The I-V characteristics are calculated by,

where e, h, and fL(R) are electron charge, Planck’s constant, and the Fermi distribution functions at left (right) electrode, respectively. T(E, Vb) is the transmission coefficient at energy E and bias voltage Vb. We work with the Perdew-Zunger exchange and correlation functional [16] within the local density approximation. Norm-conserving pseudo potentials are used to describe the coreelectrons for all atoms. We have assumed the diameters SiNWs we used are less than 2 nm, Si-Si bond length is almost equal to bulk Si crystal. The nanowires we adopted had different cross section and in different sizes, and the surface is fully hydrogen terminated to eliminate the contribution of dangling bonds. We first of all calculated electronic band structures of SiNWs using ATOMISTIX TOOLKIT, which is excellently matched with experimental data [17] [18] [19] .
4. Result & Conclusions
On analysis we found that with the increase in diameter of SiNWs the band gap decreases and it is inversely proportional to the diameter of wire i.e.

From Figure 2, we find that gap width varied from 3.5 eV to 0.75 eV, for the variation in cross section area from 0.5 nm2 to 1.5 nm2 for [110] [111] [112] series.
Our results are in agreements and perform the same trends with the experimental results [20] . Here, the size dependence indicates the quantum confinement, for the reason that the movement of electrons was confined in the plane perpendicular to wire axis. Energy band near Fermi energy level was effected since the diameter of wires is small, and the effective mass in confinement plane for [111] is smaller than that for [110] wire, and energy shift is large, which indicates the dependence of energy gap on orientation as well, but Figure 3 indicates slight dependence only.
As impurities and dopants are adsorbed on SiNW surface, so they influence the electronic structure, which causes the change in conductance/transport properties. We calculate band structures for nanowires doped with N, & -OH using ATOMISTIX TOOLKIT. Interestingly, the different dopant adsorbed, clearly resulted in different band structure. Thus, all the results shown in Figure 4 are evidence that the different adsorbents modify the band structures in different ways so, we can use SiNWs as
Figure 2. Band gap of the SiNWs versus the cross-sectional diameter, blue dots is for [110], brown for [111], and green for experimental data taken from reference [17] for [111].
Figure 3. Band gap of the SiNWs versus the cross-sectional area, blue dots is for [111], brown for [112].

Figure 4. Diagram for electronic band structure (a) SiNW, (b) SiNW with N doped, (c) SiNW with -OH group.
sensors by tuning the band gaps through controlling surface density of dopants/surface treatments.
Cite this paper
Lamba, V.K. and Garg, O.P. (2016) Designing Sensors Using Nano-Junctions. Journal of Applied Mathematics and Physics, 4, 2247-2253. http://dx.doi.org/10.4236/jamp.2016.412217
References
- 1. Cui, Y. and Lieber, C.M. (2001) Functional Nanoscale Electronic Devices Assembled Using Silicon Nanowire Building Blocks. Science, 291, 851-853.
https://doi.org/10.1126/science.291.5505.851 - 2. Craighead, H.G. (2000) Nanoelectromechanical Systems. Science, 290, 1532-1535.
https://doi.org/10.1126/science.290.5496.1532 - 3. Paulose, M., Grimes, C.A. Varghese, O.K. and Dickey, E.C. (2002) Self-Assembled Fabrication of Aluminum-Silicon Nanowire Networks. Applied Physics Letters, 81, 153-155.
https://doi.org/10.1063/1.1492005 - 4. Dequesnes, M., Rotkin, S. and Aluru, N.R. (2002) Calculation of Pull-In Voltagesfor Carbon-Nano-Tube-Based Nano-Electro-Mechanical Switches. Nanotechnology, 13, 120-131.
- 5. Tang, Z., Xu, Y., Li, G. and Aluru, N.R. (2005) Physical Models for Coupled Electromechanical Analysis of Silicon Nano-Electro-Mechanical Systems. Journal of Applied Physics, 97, 114304.
https://doi.org/10.1063/1.1897483 - 6. Tadmor, E.B., Ortiz, M. and Philips, R. (1995) Quasicontinuum Analysis of Defects in Solids. Philosophical Magazine A, 73, 1529-1563.
https://doi.org/10.1080/01418619608243000 - 7. Tang, Z. and Aluru, N.R. (2008) Multiscale Mechanical Analysis of Silicon Nanostructures by Combined Finite Temperature Models. Computer Methods in Applied Mechanics and Engineering, 197, 3215-3224.
https://doi.org/10.1016/j.cma.2007.11.018 - 8. Tang, Z., Zhao, H., Li, G. and Aluru, N.R. (2006) Finite-Temperature Quasi-Continuum Method for Multiscale Analysis of Silicon Nanostructures. Physical Review B, 74, Article ID: 064110.
https://doi.org/10.1103/PhysRevB.74.064110 - 9. Medic (2003) Two Dimensional Device Simulation Program, 2003. Synopsis, Version 2003.
- 10. Nair, P.R. and Alam, M.A. (2006) Simulation of Nanowire Bio Sensors. Proceedings of 64th Device Research Conference, 183-184.
https://doi.org/10.1109/DRC.2006.305048 - 11. Nogueira, F., Castro, A. and Marques, M.A.L. (2003) A Tutorial on Density Functional Theory. In: Fiolhais, C., Nogueira, F. and Marques, M.A.L., Eds., A Primer in Density Functional Theory, Springer, Berlin, 218-256.
- 12. Stern, E., Klemic, J.F., Routenberg, D.A., Wyrembak, P.N., Turner-Evans, D.B., Hamilton, A.D., LaVan, D.A., Fahmy, T.M. and Reed, M.A., (2007) Label-Free Immunodetection with CMOS-Compatible Semiconducting Nanowires. Nature, 445, 519-523.
https://doi.org/10.1038/nature05498 - 13. Wang, J., Palecek, D., Nielsen, P.E., Rivas, G., Cai, X., Shiraishi, H., Dontha, N., Luo, D. and Farias, P.A.M. (1996) Peptide Nucleic Acid Probes for Sequence-Specific DNA Biosensors. Journal of the American Chemical Society, 118, 7667-7670.
https://doi.org/10.1021/ja9608050 - 14. Nair, P.R. and Alam, M.A. (2006) Performance Limits of Nanobiosensors. Applied Physics Letters, 88, Article ID: 233120.
- 15. Datta, S. (1995) Electronic Transport in Mesoscopic Systems. Cambridge University Press, Cambridge.
https://doi.org/10.1017/CBO9780511805776 - 16. AtomistixToolKit Version 2.0. Atomistix A/S.
www.atomistix.com - 17. Gonze, X., Beuken, J.M., Caracas, R., Detraux, F., Fuchs, M., Rignanese, G.-M., Sindic, L., Verstaete, M., Zerah, G., Jollet, F., Torrent, M., Roy, A., Mikami, M. and Allen, D.C. (2002) First-Principles Computation of Material Properties: The ABINIT Software Project. Computational Materials Science, 25, 478-492.
https://doi.org/10.1016/S0927-0256(02)00325-7 - 18. Perdew, J.P. and Zunger, A. (1981) Self-Interaction Correction to Density-Functional Approximations for Many-Electron Systems. Physical Review B, 23, 5048-5079.
https://doi.org/10.1103/PhysRevB.23.5048 - 19. Li, C.P., Lee, C.S., Ma, X.L., Wang, N., Zhang, R.Q. and Lee, S.T. (2003) Growth Direction and Cross-Sectional Study of Silicon Nanowires. Advanced Materials, 15, 607-609.
https://doi.org/10.1002/adma.200304409 - 20. Li, D., Wu, Y., Kim, P., Shi, L., Yang, P. and Majumdar, A. (2003) Thermal Conductivity of Individual Silicon Nanowires. Applied Physics Letters, 83, 2934-2936.
https://doi.org/10.1063/1.1616981



