American Journal of Computational Mathematics
Vol.06 No.03(2016), Article ID:70824,8 pages
10.4236/ajcm.2016.63028
Computer Simulation of Transition Regimes of Solitons in Four-Photon Resonant Parametric Processes in Case of Two-Photon Resonance
Vladimir Feshchenko1, Galina Feshchenko2
1Dawson College, Montreal, Canada
2Vanier College, Montreal, Canada
Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 30 July 2016; accepted 20 September 2016; published 23 September 2016
ABSTRACT
The transition regimes of solitons in four-photon resonant processes in the case of two-photon absorption of the fundamental radiation are numerically investigated. The standard system of equations for the amplitudes of probability of finding the system in state with certain energy is used to derive the expression for the induced polarization in the nonlinear medium. As for the equations for the amplitudes of the optical pulses, the general case is considered in which both the amplitudes and phases are space-time dependent. We focus on the finite difference methods and the case of simultaneously propagating solitons at all frequencies of the interacting waves (simultons). The obtained results indicate that upon certain threshold conditions all interacting pulses become the solitons of Lorentzian shape. The numerical analysis has also shown that the soliton amplitudes significantly depend on the ratio between the nonlinear polarizability at the fundamental frequency 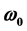 and that of combination of
and that of combination of 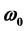 and the trigger-field frequency
and the trigger-field frequency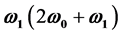 . In the second part of the paper, we apply the method of phase planes to show that at typical values of parameters, the solitons are stable.
. In the second part of the paper, we apply the method of phase planes to show that at typical values of parameters, the solitons are stable.
Keywords:
Solitons, Transition Regime, Stability

1. Introduction
Solitons or self-reinforcing solitary waves can emerge spontaneously in a physical system in which some energy is fed in, for instance as thermal energy or by an excitation with an electromagnetic wave, even if the excitation does not match exactly the soliton solution. Therefore, if a system possesses the necessary properties to allow the existence of solitons, it is highly likely that any large excitation will indeed lead to their formation [1] - [3] . The field of solitons and related nonlinear phenomena has been substantially advanced and enriched by research and discoveries in nonlinear optics [4] - [7] .
In our previous research [8] , we established the possibility of the existence of simultons (simultaneously propagating solitons at different frequencies) in the case of nonstationary Raman scattering with excitation of polar optical phonons under the conditions of the interaction of ultrashort pulses of exciting and Stokes radiation in nonlinear crystals. The relevance of this study is connected both with the fact that one can extract additional information on the optical characteristics of matter, and with the possibility of obtaining of ultrashort pulses.
The second topical problem in modern nonlinear optics is the production of coherent and frequency-tunable radiation in the far ultraviolet (UV) and infrared (IR). In these spectral areas, solid materials have broad absorption bands and this narrows down the application of nonlinear crystals for the generation of electromagnetic radiation. Possible ways of overcoming those difficulties are related with the utilization of nonlinear phenomena in gases and metal vapors. The resonant four-photon interaction (RFPI) in the case of two-photon resonance is one of them. Among the advantages of gases are the presence of narrow resonances and possibility of continuous variation of density, width of spectral line, length of the medium, etc. [9] - [11] . Ultrashort pulse propagation in the case of two-photon resonance was first examined in [12] where the two-photon self-induced transparency effect was predicted. This prediction was subsequently confirmed experimentally [13] and by numerical studies [14] . Third-harmonic generation (THG) in media exhibiting resonance behaviour has also attracted considerable attention [15] - [21] . However, RFPIs that are not frequency degenerate are of no less interest; they can be used to transfer the tuning of radiation from one range to another [22] [23] .
The present paper is devoted to the computer simulation of transition regimes of RFPI solitons in the case of two-photon resonance. The basic equations describing this process are given in Section 2 [12] [24] . The results of computer simulation are shown in Section 3. The stability of solitons is considered in Section 4.
2. Fundamental Principle
Let us assume that two optical pulses with frequencies 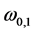 propagate in the nonlinear medium at the angles
propagate in the nonlinear medium at the angles 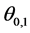 with respect to the z-axis. The value of
with respect to the z-axis. The value of 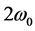 is close to the frequency of resonant transition between levels 2 and 1 in the medium
is close to the frequency of resonant transition between levels 2 and 1 in the medium . The nonlinear interaction between
. The nonlinear interaction between 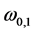 and the medium results in parametric generation of
and the medium results in parametric generation of 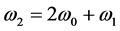 and
and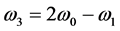 . The values of
. The values of 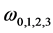 are considered to be in the transparent range of frequencies. We also assume that all electromagnetic waves have the same polarizations.
are considered to be in the transparent range of frequencies. We also assume that all electromagnetic waves have the same polarizations.
To find the system of equations that governs the processes of propagation of optical pulses with frequencies 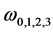 in the medium we take the standard system of equations for the amplitudes of probability
in the medium we take the standard system of equations for the amplitudes of probability 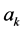 of finding the system in state with energy
of finding the system in state with energy 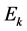 [25]
[25]
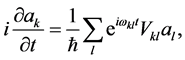 (1)
(1)
where 



We next use (1) and the theory of perturbations [26] to find 

order

To obtain the system of equations for 


where 


The expression for the polarization induced by the superposition of nonlinear waves is defined by

We introduce (3) into (7) and find that the expression for the induced polarization becomes

where 


The system of Equations (4) and (5) can now be rewritten in terms of 



where 






To make the system (8) - (10) complete we add Maxwell’s equations for the all real “slowly-varying amplitudes” 









where 













3. Transition Regime
We carried out the computer simulation of the system (8) - (10) and (11) - (18) for the following parameters of electromagnetic radiation and medium (gases): 






4. Stability
To investigate the stability of solitons we perform the summation of the Equations (12) (14) (16) and (18) for phases 
















Figure 1. The formation of the soliton at frequency
Figure 2. The formation of the soliton at frequency
where 










The behaviour of the latter system is analyzed in terms of phase planes. As an example, Figure 5 shows the phase plane of the following system

Figure 3. The generation of the soliton at frequency
Figure 4. The generation of the soliton at frequency

at 11 different initial conditions for
5. Conclusion
The space-time evolution of the optical pulses by using the computer simulation of transition regimes of four- photon resonant parametric processes in case of two-photon resonance is investigated. The computer simulation was based on application of the finite difference methods to the system of nonlinear equations modeling the foregoing interactions. It is shown that at certain boundary conditions (those result from the “area theorem” (see, e.g. [30] )) the incoming laser pulses at frequencies 

Figure 5. The phase plane of the system (21) and (22) (



or




Cite this paper
M. Arif,J. Hussain,I. Hussain,S. Kumar,G. Bhati,Vladimir Feshchenko,Galina Feshchenko, (2016) Computer Simulation of Transition Regimes of Solitons in Four-Photon Resonant Parametric Processes in Case of Two-Photon Resonance. American Journal of Computational Mathematics,06,267-274. doi: 10.4236/ajcm.2016.63028
References
- 1. Ayoob, S. and Gupta, A.K. (2006) Fluoride in Drinking Water: A Review on the Status and Stress Effects. Critical reviews in Environmental Science and Technology, 36, 433-487.
http://dx.doi.org/10.1080/10643380600678112 - 2. Arif, M., Hussain, J., Hussain, I. and Neyol, S. (2011) Fluoride Contamination of Ground Water of Merta Block in Nagaur District, Rajasthan, India. The Conference of Advance in Environmental Chemistry (AEC), Aizwal, 146-148.
- 3. Arif, M., Hussain, I., Hussain, J., Sharma, S. and Kumar, S. (2012) Fluoride in the Drinking Water of Nagaur Tehsil of Nagaur District, Rajasthan, India. Bulletin of Environmental Contamination and Toxicology, 88, 870-875.
http://dx.doi.org/10.1007/s00128-012-0572-4 - 4. Hussain, J., Sharma, K.C. and Hussain, I. (2005) Fluoride Distribution in Groundwater of Banera Tehsil in Bhilwara District, Rajasthan. Asian Journal of Chemistry, 17, 457-461.
- 5. Hussain, J., Shrama, K.C., Arif, M. and Hussain, I. (2007) Fluoride Distribution and Modelling Using Best Subset Procedure in Nagour District of Central Rajasthan, India. The 27th Conference of the International Society for Fluoride Research (ISFR XXVII), Beijing.
- 6. Hussain, I., Arif, M. and Hussain, J. (2011) Fluoride Contamination in Drinking Water in Rural Habitations of Central Rajasthan, India. Environmental Monitoring and Assessment, 184, 5151-5158.
http://dx.doi.org/10.1007/s10661-011-2329-7 - 7. Sharma, K.C., Arif, M., Hussain, I. and Hussain, J. (2007) Observation on Fluoride Contamination in Groundwater of District Bhilwara, Rajasthan and a Proposal for a Low Cost Defluoridation Technique. The 27th Conference of the International Society for Fluoride Research (ISFR XXVII), Beijing.
- 8. Choubisa S.L., Choubisa L. and Choubisa D.K. (2001) Endemic Fluorosis in Rajasthan. Indian Journal of Environmental Health, 43, 177-189.
- 9. EPA (1997) Public Health Global for Fluoride in Drinking Water. Pesticide and Environmental Toxicology. Section Office of Environmental Health Hazard Assessment, California Environmental Protection Agency.
- 10. Ramanaiah, S.V., Venkatamohan, S., Rajkumar, B. and Sarma, P.N. (2006) Monitoring of Fluoride Concentration in Groundwater of Prakasham District in India: Correlation with Physico-Chemical Parameters. Journal of Environmental Science and Engineering, 48, 129-134.
- 11. WHO (2006) Fluoride in Drinking Water. IWA Publishing, London, 144.
- 12. Athavale, R.N. and R.K. Das (1999) Beware! Fluorosis Is Zeroing in on You. Down to Earth, 8, 24-25.
- 13. Susheela, A.K. (1999) Fluorosis Management Programme in India. Current Science, 77, 1250-1256.
- 14. Chakraborti, D., Chanda, C.R., Samanta, G., Chowdhury, U.K., Mukherjel, S.C. and Pal, A.B. (2000) Fluorosis in Assam, India. Current Science, 78, 1421-1423.
- 15. Muralidharan, D., Nair, A.P. and Satyanarayana, U. (2002) Fluoride in Shallow Aquifers in Rajgarh Tehsil of Churu District, Rajasthan—An Arid Environment. Current Science, 83, 699-702.
- 16. Pillai, K.S. and Stanley, V.A. (2002) Implications of Fluoride—An Endless Uncertainty. Journal of Environmental Biology, 23, 81-97.
- 17. Arif, M., Joshi, S. and Kumar, S. (2012) A Study of Fluoride Contaminated Ground Water in Uniara Tehsil, District-Tonk, Rajasthan, India. India Water Week.
- 18. Arif, M., Hussain, J., Hussain, I. and Kumar, S. (2013) An Assessment of Fluoride Concentration in Groundwater and Risk on Health of North Part of Nagaur District, Rajasthan, India. World Applied Sciences Journal, 24, 146-153.
- 19. Arif, M., Hussain, J., Hussain, I. and Kumar, S. (2013) An Investigation of Fluoride Distribution in Ladnu Block of Nagaur District, Central Rajasthan. World Applied Sciences Journal, 26, 1610-1616.
- 20. Arif, M., Yadav, B.S. and Garg, A. (2013) An Investigation of Fluoride Concentration in Drinking Water of Sanganer Tehsil, Jaipur District, Rajasthan, India and Defluoridation from Plant Material. EQA-Environmental Quality, 12, 23-29.
- 21. Shortt, H.E., Mcrobert, G.R., Barnard, T.W. and Mannadinayer, A.S. (1937) Endemic Fluorosis in Madras Presidency, India. Indian Journal of Medical Research, 25, 553-561.
- 22. WHO (2004) Guidelines for Drinking Water Quality. World Health Organization, Geneva, 515.
- 23. BIS (2003) Indian Standard Specifications for Drinking Water. Bureau of Indian Standards, New Delhi, India: IS: 10500, 10.
- 24. Census (2001) District Nagaur, Rajasthan, Government of Rajasthan.
- 25. Robinson, W.O. and Edington, G. (1946) Fluorine in Soils. Soil Science, 61, 341-353.
http://dx.doi.org/10.1097/00010694-194605000-00001 - 26. Jacks, G., Bhattacharya, P., Chaudhary, V. and Singh, K.P. (2005) Controls on the Genesis of High-Fluoride Ground Waters in India. Applied Geochemistry, 20, 221-228.
http://dx.doi.org/10.1016/j.apgeochem.2004.07.002 - 27. APHA (1991) Standard Methods for the Examination of Water and Wastewater. 17th Edition, American Public Health Association, Washington DC.
- 28. Dean, H.T. (1942) The Investigation of Physiological Effects by the Epidemiological Method. American Association for the Advancement of Science, 19, 23-33.
- 29. Olsson, B. (1979) Dental Finding in High Fluoride Areas. Epidemiology, 7, 51-56.
- 30. Dauxois, T. and Peyrard, M. (2010) Physics of Solitons. Cambridge University Press, Cambridge.
- 31. Williams, M.C. (2013) Solitons: Interactions, Theoretical, and Experimental Challenges and Perspectives. Nova Science Publishers Inc., New York.
- 32. Manevich, L.I. and Simmons, V.V. (2008) Solitons in Micromolecular Systems. Nova Science Publishers Inc., New York.
- 33. Dzedolik, I.V. (2016) Solitons and Nonlinear Waves of Photon-Polaritons and Plasmon-Polaritons. Nova Science Publishers, New York.
- 34. Boyd, R. (2008) Nonlinear Optics. 3rd Edition, Academic Press, Waltham.
- 35. Kivshar, Y. and Agrawal, G. (2003) Optical Solitons: From Fiber to Photonic Crystals. Academic Press, Waltham.
- 36. Abdullaev, F., Darmanyan, S. and Khabibullaev, P. (2013) Optical Solitons. Springer Publishing, New York.
- 37. Feshchenko, G. and Feshchenko, V. (2015) Computer Simulation of Transition Regimes of Solitons in Stimulated Raman Scattering with Excitation of Polar Optical Phonons. American Journal of Computational Mathematics, 5, 336-344.
http://dx.doi.org/10.4236/ajcm.2015.53031 - 38. De Melo, N.R. and Vianna, S.S. (2014) Two-Photon Resonant Forward Four-Wave Mixing in Rubidium Vapor Involving Rydberg States. Journal of the Optical Society of America B, 31, 1735-1740.
http://dx.doi.org/10.1364/JOSAB.31.001735 - 39. Namboodiri, V., Namboodiri, M., Flachenecker, G. and Materny, A. (2010) Two-Photon Resonances in Femtosecond Time-Resolved Four-Wave Mixing Spectroscopy: Beta-Carotene. The Journal of Chemical Physics, 133, Article ID: 054503.
http://dx.doi.org/10.1063/1.3466750 - 40. Smith, A.V., Alford, W.J. and Madley, G.R. (1988) Optimization of Two-Photon Resonant Four-Wave Mixing: Application to 130.2-nm Generation in Mercury Vapor. Journal of the Optical Society of America B, 5, 1503-1519.
http://dx.doi.org/10.1364/JOSAB.5.001503 - 41. Belenov, E.M. and Poluektov, I.A. (1969) Coherence Effects in the Propagation of an Ultrashort Light Pulse in a Medium with Two-Photon Resonance Absorption. Soviet Physics—JETP, 29, 754-756.
- 42. Tan-no, N., Yokoto, K. and Inaba, H. (1972) Two-Photon Self-Induced Transparency of Different Frequency Optical Short Pulses in Potassium. Physical Review Letters, 29, 1211-1213.
http://dx.doi.org/10.1103/PhysRevLett.29.1211 - 43. Tan-no, N., Yokoto, K. and Inaba, H. (1975) Two-Photon Self-Induced Transparency in a Resonant Medium. II Transient Pulse Behaviour. Journal of Physics B: Atomic and Molecular Physics, 8, 349-357.
http://dx.doi.org/10.1088/0022-3700/8/3/005 - 44. Wang, C.C. and Davis Jr., L.I. (1975) Saturation of Two-Photon Transitions in Thallium Vapor. Physical Review Letters, 35, 650-652.
http://dx.doi.org/10.1103/PhysRevLett.35.650 - 45. Ward, J.F. and Smith, A.V. (1975) Saturation of Two-Photon Optical Processes in Cesium Vapor. Physical Review Letters, 35, 653-655.
http://dx.doi.org/10.1103/PhysRevLett.35.653 - 46. Leung, K.M., Ward, J.F. and Orr, B.J. (1974) Two-Photon Resonant Optical Third-Harmonic Generation in Cesium Vapor. Physical Review A, 9, 2440.
http://dx.doi.org/10.1103/PhysRevA.9.2440 - 47. Poluéktov, I.A. (1977) Third Harmonic Generation by Two-Photon Interaction of High-Power Coherent Light Pulses with Resonant Media. Soviet Journal of Quantum Electronics, 7, 364-365.
- 48. Anikin, V.I., Drabovich, K.N. and Dubovick, A.N. (1977) Coherence Effects in Optical-Frequency Summation under Two-Photon Resonance Conditions. Journal of Experimental and Theoretical Physics, 45, 906-911.
- 49. Maimistov, A.I., Manykin, E.A. and Khodulev, L.B. (1979) Propagation of Short Optical Pulses during Third-Harmonic Generation in the Case of Two-Photon Resonance. Journal of Experimental and Theoretical Physics, 49, 431-435.
- 50. Elgin, J.N. and New, G.H.C. (1978) Theory of Two-Photon Resonant Up-Conversion with Ultrashort Pulses. I. Third Harmonic Generation. Journal of Physics B: Atomic and Molecular Physics, 11, 3439-3451.
http://dx.doi.org/10.1088/0022-3700/11/19/019 - 51. Matsuoka, M., Nakatsuka, H. and Okada, J. (1975) Free-Precision Decay of Two-Photon-Induced Coherence in Ca Vapor. Physical Review A, 12, 1062-1065.
http://dx.doi.org/10.1103/PhysRevA.12.1062 - 52. Butylkin, V.S., Grigor’yan, V.S. and Zhabotinskii, M.E. (1980) Four-Wave Interaction of Ultrashort Light Pulses Propagating Successively in a Medium. Journal of Experimental and Theoretical Physics, 51, 832-838.
- 53. Takatsuji, M. (1975) Theory of Coherent Two-Photon Resonance. Physical Review A, 11, 619-624.
http://dx.doi.org/10.1103/PhysRevA.11.619 - 54. Sakurai, J.J. and Napolitano, J. (2013) Modern Quantum Mechanics. 2nd Edition, Pearson, New York.
- 55. Davydov, A.S. and Haar, D. (2016) Quantum Mechanics. 2nd Edition, Science Direct, Amsterdam.
- 56. Butylkin, V.S., Kaplan, A.E., Khronopulo, Y.G. and Yakubovitch, E.I. (2012) Resonant Nonlinear Interactions of Light with Matter. Springer Science & Business Media, New York.
- 57. Makhviladze, T.M. and Sarychev, M.E. (1976) Soliton Regimes of Stimulated Raman Scattering. Journal of Experimental and Theoretical Physics, 44, 471-477.
- 58. Barantzov, V.I., Popov, A.K. and Tartakovskii, G.Kh. (1974) Possibility of Four-Photon Parametric Generation of Light in Gases. Journal of Experimental and Theoretical Physics, 40, 449-456.
- 59. Marchevskii, F.N., Strizhevskii, V.L. and Feshchenko, V.P. (1985) Solitons Formation in Resonant Parametric Processes in Gases. Bulletin of the Russian Academy of Sciences: Physics, 49, 606-610.



























