American Journal of Anal yt ical Chemistry, 2011, 2, 953-961
doi:10.4236/ajac.2011.28111 Published Online December 2011 (http://www.SciRP.org/journal/ajac)
Some Physicochemical and Thermal Studies on Organic
Analog of a Nonmetal-Nonmetal Monotectic Alloy;
2-Cyanoacetamide–4-chloronitrobenzene System
Manjeet Singh, Rama Nand Rai, Uma Shankar Rai*
Department of Chemistry, Centre of Advanced Study, Banaras Hindu University, Varanasi, India
Received June 20, 2011; revised August 3, 2011; accepted August 15, 2011
Abstract
The phase equilibrium data on organic analog of the nonmetal-nonmetal system, involving 2-cyanoacetamide
(CA)―4-chloronitrobenzene (CNB), show the formation of a monotectic (0.10 mole fraction of CNB) and a
eutectic (0.98 mole fraction of CNB) with a large miscibility gap starting from 0.10 mole fraction of CNB of
monotectic (M) and ending at 0.92 mole fraction of CNB of monotectic horizontal (Mh); the upper consolute
temperature Tc being 63˚C above the monotectic horizontal at 118˚C and eutectic temperature is at 85˚C. The
values of enthalpy of fusion of the pure components, the eutectic and the monotectic were determined by the
differential scanning calorimetry (Mettler DSC-4000 system). Using these data, the size of the critical radius,
interfacial energy, excess thermodynamic functions, entropy of fusion, and enthalpy of mixing were calcu-
lated. The solid-liquid interfacial energy data confirm the applicability of the Cahn wetting condition. While
growth data obey the Hillig-Turnbull equation, the microstructural investigations give typical characteristic
features of the eutectic and the monotectic of the system.
Keywords: Phase Diagram, Thermal Properties, Eutectic Composite, Monotectic Alloys, Interfacial Energy,
Microstructure, Solvent Free Synthesis
1. Introduction
It is well known that metal eutectics and intermetallic
compounds show their properties entirely different from
their parent compounds. The studies on mechanism of
solidification behaviour of polyphase alloys, particularly
monotectic alloy, are of potential importance for forma-
tion of self lubricating alloys and various other industrial
applications [1-4]. Although plenty of investigations are
in process to get metal eutectics and their intermetallic
compounds for different applications, these systems are
not suitable for detailed work due to high transformation
temperature, difficulties involved in their purification,
opacity, limited choice of materials and wide difference
in the density of the two components involved [5-8]. Due
to low transformation temperature, ease of purification,
transparency, wider choice of materials and minimized
convection effects on solidification, organic systems are
being used as model systems for detailed investigation of
the parameters which control the mechanism of solidifi-
cation which decides the properties of materials. The last
two decades have witnessed ample physicochemical in-
vestigations on organic materials for non-linear optical
and different electronic applications [9-11].
The eutectic reaction is characterized by the isother-
mal decomposition of liquid eutectic into two solids and
the monotectic reaction is associated with the breaking of
a monotectic liquid, at the invariant temperature, into a
solid and another liquid phase. The eutectic reactions
have been examined in detail during the last four decades
and their products are currently in wide applications. The
freezing behaviour of monotectic is more complicated
but interesting [12]. The main problem arises due to a
wide freezing range and large density difference between
two liquid phases. These results in low cast quality and
de-mixing of liquid phases and have delayed the progress
and potential use of monotectic as industrial material. A
critical scanning of the exiting literature has revealed that
the segregation can be influenced and relaxed by vigor-
ous stirring, chill casting and micro-gravity condition.
In general, there are two models which explain the so-
lidification behaviour of monotectic alloys. These are 1)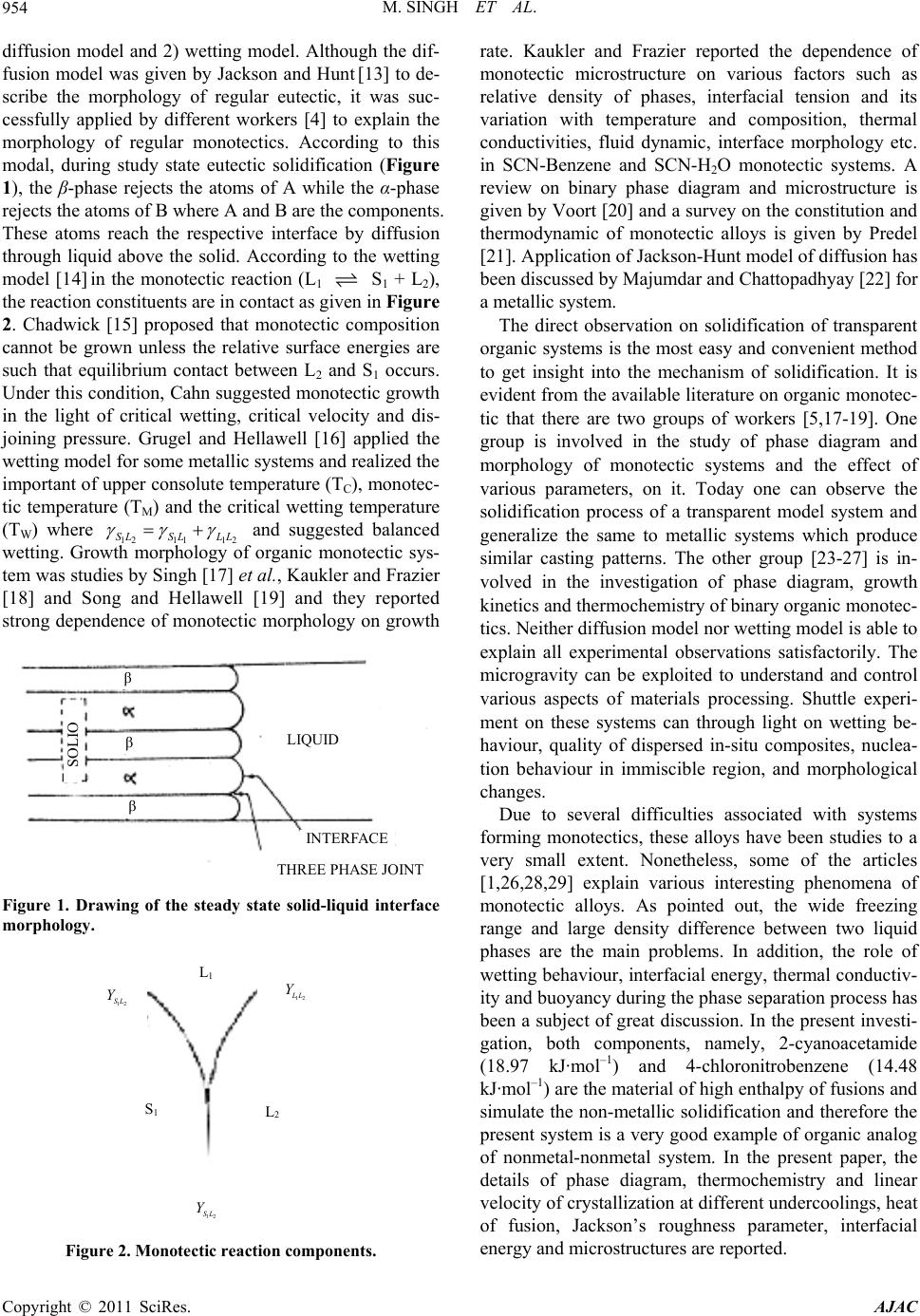

M. SINGH ET AL. 954
diffusion model and 2) wetting model. Although the dif-
fusion model was given by Jackson and Hunt [13] to de-
scribe the morphology of regular eutectic, it was suc-
cessfully applied by different workers [4] to explain the
morphology of regular monotectics. According to this
modal, during study state eutectic solidification (Figure
1), the β-phase rejects the atoms of A while the α-phase
rejects the atoms of B where A and B are the components.
These atoms reach the respective interface by diffusion
through liquid above the solid. According to the wetting
model [14] in the monotectic reaction (L1 S
1 + L2),
the reaction constituents are in contact as given in Figure
2. Chadwick [15] proposed that monotectic composition
cannot be grown unless the relative surface energies are
such that equilibrium contact between L2 and S1 occurs.
Under this condition, Cahn suggested monotectic growth
in the light of critical wetting, critical velocity and dis-
joining pressure. Grugel and Hellawell [16] applied the
wetting model for some metallic systems and realized the
important of upper consolute temperature (TC), monotec-
tic temperature (TM) and the critical wetting temperature
(TW) where 1211 12
SLSL LL


  and suggested balanced
wetting. Growth morphology of organic monotectic sys-
tem was studies by Singh [17] et al., Kaukler and Frazier
[18] and Song and Hellawell [19] and they reported
strong dependence of monotectic morphology on growth
LIQUID
INTE RFACE
β
THREE PHASE JOINT
β
β
SOLIO
Figure 1. Drawing of the steady state solid-liquid interface
morphology.
L
2
S
1
L
1
12
SL
Y 12
L
L
Y
12
SL
Y
Figure 2. Monotectic reaction components.
rate. Kaukler and Frazier reported the dependence of
monotectic microstructure on various factors such as
relative density of phases, interfacial tension and its
variation with temperature and composition, thermal
conductivities, fluid dynamic, interface morphology etc.
in SCN-Benzene and SCN-H2O monotectic systems. A
review on binary phase diagram and microstructure is
given by Voort [20] and a survey on the constitution and
thermodynamic of monotectic alloys is given by Predel
[21]. Application of Jackson-Hunt model of diffusion has
been discussed by Majumdar and Chattopadhyay [22] for
a metallic system.
The direct observation on solidification of transparent
organic systems is the most easy and convenient method
to get insight into the mechanism of solidification. It is
evident from the available literature on organic monotec-
tic that there are two groups of workers [5,17-19]. One
group is involved in the study of phase diagram and
morphology of monotectic systems and the effect of
various parameters, on it. Today one can observe the
solidification process of a transparent model system and
generalize the same to metallic systems which produce
similar casting patterns. The other group [23-27] is in-
volved in the investigation of phase diagram, growth
kinetics and thermochemistry of binary organic monotec-
tics. Neither diffusion model nor wetting model is able to
explain all experimental observations satisfactorily. The
microgravity can be exploited to understand and control
various aspects of materials processing. Shuttle experi-
ment on these systems can through light on wetting be-
haviour, quality of dispersed in-situ composites, nuclea-
tion behaviour in immiscible region, and morphological
changes.
Due to several difficulties associated with systems
forming monotectics, these alloys have been studies to a
very small extent. Nonetheless, some of the articles
[1,26,28,29] explain various interesting phenomena of
monotectic alloys. As pointed out, the wide freezing
range and large density difference between two liquid
phases are the main problems. In addition, the role of
wetting behaviour, interfacial energy, thermal conductiv-
ity and buoyancy during the phase separation process has
been a subject of great discussion. In the present investi-
gation, both components, namely, 2-cyanoacetamide
(18.97 kJ·mol–1) and 4-chloronitrobenzene (14.48
kJ·mol–1) are the material of high enthalpy of fusions and
simulate the non-metallic solidification and therefore the
present system is a very good example of organic analog
of nonmetal-nonmetal system. In the present paper, the
details of phase diagram, thermochemistry and linear
velocity of crystallization at different undercoolings, heat
of fusion, Jackson’s roughness parameter, interfacial
energy and microstructures are reported.

M. SINGH ET AL.955
2. Experimental
2.1. Materials and Purification
2-Cyanoacetamide (Aldrich, Germany) was purified by
crystallization from de-ionized water while 4-chloroni-
trobenzene (Aldrich, Germany) was purified by crystal-
lization from ethanol. The melting temperatures of CA
and CNB were found to be 121.0˚C and 86.0˚C, respec-
tively, which are consistent to their reported values
[30,31].
2.2. Phase Diagram
The phase diagram of CA-CNB system was determined
by recording the melting point temperature of mixtures
and plotting a curve in composition on the X-axis and
their respective melting temperature on the Y-axis. In
this method [32,33] mixtures of two components cover-
ing the entire range of compositions were prepared and
taken in test tubes and after sealing the mouth of the test
tubes these mixtures were homogenized by repeating the
process of melting followed by chilling in ice cooled
water 4 times. The melting/complete miscible tempera-
tures of different composition were determined using a
melting point apparatus (Toshniwal) attached with a pre-
cision thermometer associated with an accuracy of 
0.5˚C. During the melting point determination of each
composition, the heating rates were kept 2˚C - 3˚C/min.
However, cooling rate during homogenization was very
fast because the test tube containing melts were taken
directly into ice cold water.
2.3. Enthalpy of Fusion
The heat of fusion of the pure components, the eutec-
tic and the monotectic were determined [34] by dif-
ferential scanning calorimeter (Mettler DSC-4000
system). Indium and zinc samples were used to cali-
brate the DSC unit. The amount of test sample and
heating rate were about 7 mg and 5˚C min–1, respec-
tively. The values of enthalpy of fusion are reproduci-
ble within 1.0%.
2.4. Growth Kinetics
The growth kinetics of CA, CNB and their eutectic and
monotectic were studied [33,34] by measuring the rate of
movement of the solid-liquid interface at different un-
dercoolings in a U-shape capillary tube of Jena glass of
150 mm horizontal portion and 5 mm internal diameter.
Molten samples of pure components, eutectic and
monotectic were separately taken in the capillary, and
placed in a silicone oil bath. The temperature of the oil
bath was maintained using microprocessor temperature
controller of accuracy 0.1˚C. At a particular temperature,
below the melting point of the sample, a seed crystal of
the same composition was added to start the nucleation
and the rate of movement of the solid-liquid interface was
measured using a traveling microscope and a stop watch.
2.5. Microstructure
Microstructures of the pure components, the eutectic and
the monotectic were recorded [32] by placing a drop of
molten compound on a hot glass slide. To avoid the in-
clusion of the impurities from the atmosphere and forma-
tion of bubbles, a cover slip was glided over the melt and
it was allowed to cool to get a super cooled liquid. In
order to facilitate the heterogeneous nucleation, the
poly-crystal of the same composition (seed crystal) was
added with simultaneous sliding away the slide from the
heat source to favor the unidirectional temperature gra-
dient. The unidirectionally solidified sample on glass
slide was then placed on the platform of an optical mi-
croscope (Leitz Labourlux D). The different regions of
the glass slide were viewed and photographs of interest-
ing region were recorded choosing suitable magnifica-
tion using a camera attached with the microscope.
3. Results and discussions
3.1. Phase Diagram
The melting points of pure compounds CA and CNB are
represented in the extreme left and right side of the dia-
gram at 121˚C and 86˚C, respectively. The melting point
of CA decreases with addition of CNB up to M (the
monotectic point), after which, even a slight addition of
CNB causes the appearance of two immiscible layers
(Figure 3). In this figure the immiscibility region is
shown by the area L1 + L2 bounded by the curve MCMh.
The points shown by black circles on the curve represent
the complete melting/miscibility temperatures above
which the liquids appear as a single homogeneous liquid L.
The point C at the top of the curve is the critical point or
consolute point and the corresponding temperature
(181˚C) is known as critical solution temperature (Tc).
The miscibility temperature starts increasing after M,
attains its maximum point at C, and thereafter decreases
till it attains the monotectic horizontal (Mh). The misci-
bility curve is still continued in the region (S + L2) that lies
between the eutectic and monotectic horizontal lines and
ends at the point E, the eutectic point. The area (L1 + L2)
may be regarded as to be made up of an infinite number of
tie lines which connect the two liquid phases L1 and L2 at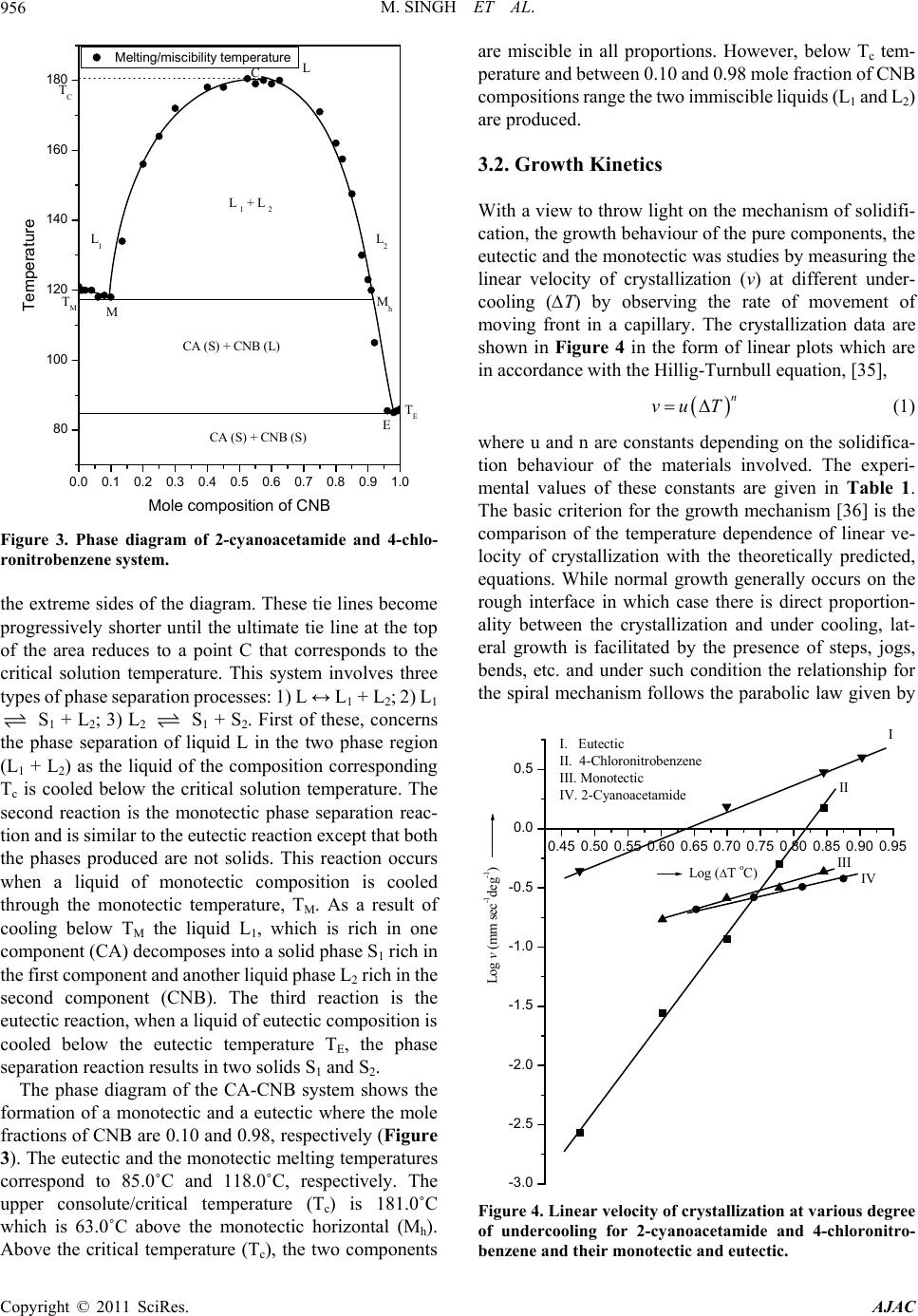

M. SINGH ET AL. 956
0.0 0.1 0.2 0.3 0.4 0.50.6 0.7 0.8 0.9 1.0
80
100
120
140
160
180 C
CA (S) + CNB (S)
CA (S) + CNB (L)
L2
L1
L
L 1 + L 2
E
MMh
TE
TM
TC
Temperature
Mole composition of CNB
Melting/miscibility temperature
Figure 3. Phase diagram of 2-cyanoacetamide and 4-chlo-
ronitrobenzene system.
the extreme sides of the diagram. These tie lines become
progressively shorter until the ultimate tie line at the top
of the area reduces to a point C that corresponds to the
critical solution temperature. This system involves three
types of phase separation processes: 1) L ↔ L1 + L2; 2) L1
S
1 + L2; 3) L2 S 1 + S2. First of these, concerns
the phase separation of liquid L in the two phase region
(L1 + L2) as the liquid of the composition corresponding
Tc is cooled below the critical solution temperature. The
second reaction is the monotectic phase separation reac-
tion and is similar to the eutectic reaction except that both
the phases produced are not solids. This reaction occurs
when a liquid of monotectic composition is cooled
through the monotectic temperature, TM. As a result of
cooling below TM the liquid L1, which is rich in one
component (CA) decomposes into a solid phase S1 rich in
the first component and another liquid phase L2 rich in the
second component (CNB). The third reaction is the
eutectic reaction, when a liquid of eutectic composition is
cooled below the eutectic temperature TE, the phase
separation reaction results in two solids S1 and S2.
 
The phase diagram of the CA-CNB system shows the
formation of a monotectic and a eutectic where the mole
fractions of CNB are 0.10 and 0.98, respectively (Figure
3). The eutectic and the monotectic melting temperatures
correspond to 85.0˚C and 118.0˚C, respectively. The
upper consolute/critical temperature (Tc) is 181.0˚C
which is 63.0˚C above the monotectic horizontal (Mh).
Above the critical temperature (Tc), the two components
are miscible in all proportions. However, below Tc tem-
perature and between 0.10 and 0.98 mole fraction of CNB
compositions range the two immiscible liquids (L1 and L2)
are produced.
3.2. Growth Kinetics
With a view to throw light on the mechanism of solidifi-
cation, the growth behaviour of the pure components, the
eutectic and the monotectic was studies by measuring the
linear velocity of crystallization (v) at different under-
cooling (∆T) by observing the rate of movement of
moving front in a capillary. The crystallization data are
shown in Figure 4 in the form of linear plots which are
in accordance with the Hillig-Turnbull equation, [35],

n
vu T (1)
where u and n are constants depending on the solidifica-
tion behaviour of the materials involved. The experi-
mental values of these constants are given in Table 1.
The basic criterion for the growth mechanism [36] is the
comparison of the temperature dependence of linear ve-
locity of crystallization with the theoretically predicted,
equations. While normal growth generally occurs on the
rough interface in which case there is direct proportion-
ality between the crystallization and under cooling, lat-
eral growth is facilitated by the presence of steps, jogs,
bends, etc. and under such condition the relationship for
the spiral mechanism follows the parabolic law given by
0.45 0.50 0.55 0.60 0.650.70 0.75 0.80 0.85 0.900.95
-3.0
-2.5
-2.0
-1.5
-1.0
-0.5
0.0
0.5
Log (T oC)
I. Eutectic
II. 4-Chloronitrobenzene
III. Monotectic
IV. 2-Cyanoacetamide
IV
III
II
I
Log v (mm sec-1deg-1)
Figure 4. Linear velocity of crystallization at various degree
of undercooling for 2-cyanoacetamide and 4-chloronitro-
benzene and their monotectic and eutectic.

M. SINGH ET AL.957
Equation (1). While in the case of the eutectic and the
monotectic, there is square relationship following para-
bolic law between linear growth velocity and undercool-
ing, in the case of CA there is direct proportionality be-
tween the growth velocity and undercooling. In the case
of CNB the growth velocity is very high in comparison
to that of CA (Table 1). These findings may be ex-
plained by the mechanism given by Winegard et al. [37]
where the crystallisation of eutectic/monotectic begins
with the formation of the nucleus of one of the phases.
This phase grows until the surrounding liquid becomes
rich in the other component and a stage is reached when
the second component starts nucleating. Now there are
two possibilities; either the two initial crystals grow
side-by-side or there may be alternate nucleation of the
two phases. The deviation of n values from 2, observed
in some cases, is due to difference in temperature of bath
and temperature of growing interface. From the values of
u (Table 1) it can be concluded that growth velocity of
eutectic lies between those of the parent components.
However, for monotectic it is higher than one of the par-
ent components. These findings suggest that the two
phases of monotectic and eutectic solidify by the side-
by-side growth mechanism.
3.3. Thermochemistry
The knowledge of enthalpy of fusion values of the pure
components, the eutectic and the monotectic are impor-
tant in understanding the mechanism of solidification,
structure of eutectic melt and the nature of interaction
between two components forming the eutectic and the
monotectic. In addition, different thermodynamic quanti-
ties such as entropy of fusion, interfacial energy, en-
thalpy of mixing, excess thermodynamic functions and
Jackson’s roughness parameter can be calculated from
the entropy of fusion data. The values of enthalpy of fu-
sion of the pure components, the eutectic and the
monotectic, determined by the DSC method, are reported
in Table 2. For comparison, the value of enthalpy of
fusion of the eutectic calculated by the mixture law [14]
is also included in the same table. The value of enthalpy
of mixing which is the difference of experimental and the
Table 1. Values of n and u for pure components, monotectic
and eutectic.
Material n u (mm·sec–1·deg–1)
CA 1.17 0.035
CNB 7.40 8.4 × 10–7
Monotectic 1.61 0.018
Eutectic 2.24 0.031
Table 2. Heat of fusion, entropy of fusion and roughness
parameter of CA, CNB and their monotectic and eutectic.
Materials
Heat of
fusion
(kJ·mol–1)
Heat of
mixing
(kJ·mol–1)
Entropy of
fusion
(J·mol–1·K–1)
Roughness
parameter
(  )
CA 18.97 48.2 5.8
CNB 14.48 40.3 4.9
Monotectic
(Exp.) 16.48 42.1 5.1
Eutectic
(Exp.)
(Cal.)
13.94
14.57 -0.63 38.9 4.7
calculated values of the enthalpy of fusion is found to be
–0.63 kJ·mol–1. As such, three types of structures are
suggested [38]; quasi-eutectic for mixH > 0, clustering
of molecules for mixH < 0 and molecular solution for
mixH = 0. In the present system the negative value of
mixH for the eutectic suggests the formation of cluster of
molecules in the binary melt of the eutectic [39].
The entropy of fusion (fusS) values, for different ma-
terials have been calculated by dividing the enthalpy of
fusion by their corresponding absolute melting tempera-
tures (Table 2). The value of entropy of fusion of eutec-
tic being less than that of monotectic suggests that en-
tropy is more effective in melting of the monotectic. The
entropy of fusion value of eutectic being less than those
of components suggest that entropy is more effective in
melting of pure components than that of the eutectic. A
measure of deviation from ideal behaviour can be best
expressed in terms of excess thermodynamic functions,
namely, excess free energy (gE), excess enthalpy (hE),
and excess entropy (sE) which give a more quantitative
idea about the nature of molecular interactions. The ex-
cess thermodynamic function (YE) is defined as the dif-
ference between the thermodynamic functions of mixing
for a real system and the corresponding values for an
ideal system at the same temperature and pressure. Thus,


E
mix mix
YYrealY ideal 

(2)
where Y is any thermodynamic function. The excess
thermodynamic functions could be calculated [32,40,41]
using the following equations and the values are given in
Table 3:
E
11 2 2
ln ln
l
gRTxx l





 (3)
E2 12
12
ln ln
ll
hRTx x
TT




 



(4)
E12
11221 2
ln ln
ln ln
ll
ll
sRx xxTxT
TT






 



(5)


where ln l
i

, xi and ln l
i
T


 are activity coefficient in

M. SINGH ET AL. 958
Table 3. Excess thermodynamic functions for the eutectic.
Material gE (kJ·mol–1) hE (k·J·mol–1) sE (J·mol–1·K–1)
CA-CNB eutectic 0.2176 49.527 0.1377
liquid state, the mole fraction and variation of log of ac-
tivity coefficient in liquid state as function of tempera-
ture of the component i.
It is evident from Equations (3)-(5) that activity coef-
ficient and its variation with temperature are needed to
calculate the excess functions. Activity coefficient


l
i

could be evaluated [23,32] by using the equation,

11
ln fus i
l
ii
f
us i
H
xRTT



 





(6)
where i
x
,
f
us i
H
, i and T
f
us are mole fraction,
enthalpy of fusion, melting temperature of component i
and melting temperature of eutectic, respectively. The
variation of activity coefficient with temperature could
be calculated by differentiating Equation (6) with respect
to temperature,
T
2
ln l
fus i
i
i
Hi
x
T RT




xT
(7)
i
x
T in this expression can be evaluated by consid-
ering two points around the eutectic. The positive values
of excess free energy indicate that the interaction be-
tween the like molecules (CA-CA and CNB-CNB) are
stronger than the interaction between the unlike (CA-
CNB) molecule [40].
The solid-liquid interfacial tension affects the enthalpy
of fusion value and plays an important role in determin-
ing the kinetics of phase transformation. When liquid is
cooled below its melting temperature, the melt does not
solidify spontaneously because under equilibrium condi-
tion, it contains number of clusters of molecules of dif-
ferent sizes. As long as the clusters are well below the
critical size [41], they cannot grow to form crystals and,
therefore, no solid would result. Also during growth, the
radius of critical nucleus gets influenced by undercooling
as well as the interfacial energy. The interfacial energy
(
 ) is given by
 
23 13
.fus
Am
CH
NV


 (8)
where NA is the Avogadro Number, Vm is the molar
volume, and parameter C lies between 0.30 to 0.35.
The calculated values of interfacial energy using
Equation (8) are given in Table 4. The literature [28,29]
during the past two decades is replete with various attempts
to understand and to explain the process of solidification of
monotectic alloys. The role of wetting behaviour in a
Table 4. Interfacial energy of 2-cyanoacetamide and 4-
chloronitrobenzene and their eutectic and monotectic.
Parameter Interfacial energy (ergs·cm–1)
2
SL

(CNB) 27.9735
1
SL

(CA) 51.480
12
L
L

(CA-CNB) 3.5716
 E (CA-CNB) 28.445
phase separation process is of immense importance. In
view of this, the applicability of Cahn’s wetting condi-
tion has been tested in the present case. The values of
interfacial energy (Table 4), in present case, show ap-
plicability of Cahn wetting condition by satisfying the
relation,
211
SLSLL L
 
2
(9)
where  is the interfacial energy between the faces de-
noted by the subscripts. The interfacial energy between
two liquids, 12
L
L

, has been calculated using the equa-
tion [42],

12121 2
2
L LSLSLSLSL
   (10)
To calculate the size of critical nucleus (r*) and the in-
fluence of undercooling on it, the following equation was
used:
2
*·
fus
fus
T
r
H
T



 (11)
where Tfus, fusH and T are melting temperature of
eutectic, heat of fusion and degree of undercooling, re-
spectively. The computed values of the size of critical
nucleus at different undercoolings using the Equations (8)
and (11) are given in Table 5. The size of critical nu-
cleus decreases with increase in undercooling. Thus, high
undercooling favors the formation of critical nucleus of
smaller size. This may be ascribed to the increase in the
amplitude of molecular vibration at higher temperature.
3.4. Microstructure
In polyphase materials the microstructure gives informa-
tion about shape and size of the crystallites, which play a
significant role in deciding the mechanical, electrical,
magnetic and optical properties of materials. According
to Hunt and Jackson [43] the type of growth from a melt
depends upon the interface roughness (  ) defined by

=

fus H/RT (12)
where  is a crystallographic factor which is generally
equal to or less than one. The values of  are reported in
Table 2. If  > 2 the interface is quite smooth and the

M. SINGH ET AL.959
Table 5. Critical radius of 2-cyanoacetamide and 4-chlo-
ronitrobenzene their eutectic and monotectic.
Undercooling Critical radius × 10–8 (cm)
ΔT (˚C) CA CNB Monotectic Eutectic
3.0 4.623 4.870
4.0 3.468 0.3709
4.5 4.757
5.0 2.774 0.2967 2.922
5.5 3.892
6.0 2.311 0.2473
6.5 3.293
7.0 1.981 0.2119 2.087
7.5 2.851
8.0 1.826
(a)
crystal develops with a faceted morphology. On the other
hand, if  < 2, the interface is rough and many sites are
continuously available and the crystal develops with a
non-faceted morphology. In the present system, the val-
ues of  being greater than 2 in all the cases suggest that
the phases grow with faceted morphology.
(b)
Microstructure of Monotectic and Eutectic
The microstructures of eutectic and monotectic are given
in Figures 5(a)-(b), respectively. In general, the optical
microphotograph of the eutectic is of lamellar nature
[44,45]. The discontinued lamellae in the figure are due
to miscibility of the solid-liquid interface. The broken
lamellar, elongated drops and spherical drops are due to
higher thermal gradient in the liquid in front of the solid
liquid interface. In general, from the energy considera-
tion, there is tendency to form spheres. If the time of
formation of spheres is more than the freezing time, the
elongated sphere will be observed. On the other hand if
time of formation of sphere is less than the time of
freezing, the sphere will be observed. The values of in-
terfacial energy given in Table 4 suggest that the wetting
condition can be successfully applied to the present sys-
tem where 2112
SLSLL L

  . While the value of 2
SL

and 1
SL

are calculated using Equation (8), the value of
12
L
L

is calculated using the Equation (10), [32].
The microstructure of monotectic is given in Figure
5(b), where faceted growth has been observed. The mi-
nor component of the monotectic has been shown in the
major component as thin lines in the microstructure. The
study on interfacial energy reveals the applicability of
Cahn wetting condition and indicates that both phases
are wetting to each other.
Figure 5. Directionally solidify optical microphotograph of
2-cyanoacetamide―4-chloronitrobenzene eutectic (a) and
monotectic (b).
4. Conclusions
The phase diagram between 2-cyanoacetamide and
4-chloronitrobenzene shows the formation of a monotec-
tic and a eutectic with 0.10 and 0.98 mole fractions of
CNB, respectively. The diagram shows that the upper
consolute temperature is 63˚C above the monotectic
horizontal. The growth kinetics suggests that the growth
data obey the Hillig-Turnbull equation for each material,
and the size of critical nucleus depends on the under-
coolings. The enthalpy of mixing was found to be nega-
tive suggesting there by formation of clusters of the ma-
terials. The interfacial energies are correlated by the rela-
tion 2112
SLSLL L, which confirms that the Cahn’s
wetting condition is applicable to the present system. The
microstructural investigations show lamellar growth
morphology for the eutectic and faceted morphology for
the monotectic.
 
5. Acknowledgements
The authors would like to thank the Head, Department of

M. SINGH ET AL. 960
Chemistry, B.H.U., Varanasi, for providing the infra-
structure facilities.
6. References
[1] D. M. Herlach, R. F. Cochrane, I. Egry, H. J. Fecht and A.
L. Greer, “Containerless Processing in the Study of Me-
tallic Melts and Their Solidification,” International Ma-
terials Reviews, Vol. 38, No. 6, 1993, pp. 273-347.
[2] H. Tang, L. C. Wrobel and Z. Fan, “Hydrodynamic
Analysis of Binary Immiscible Metallurgical Flow in a
Novel Mixing Process: Rheomixing,” Applied Physics A:
Materials Science & Processing, Vol. 81, No. 3, 2005, pp.
549-559. doi:10.1007/s00339-004-2638-6
[3] R. Trivedi and W. Kurz, “Dendritic Growth,” International
Materials Reviews, Vol. 39, No. 2, 1994, pp. 49-74.
[4] B. Majumdar and K. Chattopadhyay, “Aligned Monotec-
tic Growth in Unidirectionally Solidified Zn-Bi Alloys,”
Metallurgical and Materials Transactions A: Physical
Metallurgy and Materials Science, Vol. 31A, No. 7, 2000,
pp. 1833-1842. doi:10.1007/s11661-006-0245-1
[5] M. E. Glicksman, N. B. Singh and M. Chopra, “Gravita-
tional Effects in Dendritic Growth,” Manufacturing in
Space, Vol. 11, 1983, pp. 207-218.
[6] H. Yasuda, I. Ohnaka, Y. Matsunaga and Y. Shiohara,
“In-Situ Observation of Peritectic Growth with Faceted
Interface,” Journal of Crystal Growth, Vol. 158, No. 1-2,
1996, pp. 128-135. doi:10.1016/0022-0248(95)00420-3
[7] K. Pigon and A. Krajewska, “Phase Diagrams in the Bi-
nary Systems of 2,4,7-Rinitrofluoren-9-one with Aro-
matic and Heteroaromatic Compounds. II, Thermody-
namic Analysis,” Thermochim Acta, Vol. 58, No. 3, 1982,
pp. 299-309. doi:10.1016/0040-6031(82)87104-9
[8] S. Akbulut, Y. Ocak, U. Boyiik, K. Keslioglu and N.
Marash, “Measurement of Solid-Liquid Interfacial En-
ergy in the Pyrene Succinonitrile Monotectic System,”
Journal of Physics: Condensed Matter, Vol. 18, No. 37,
2006, pp. 8403-8412. doi:10.1088/0953-8984/18/37/001
[9] J. P. Farges, “Organic Conductors,” Marcel Dekker, New
York, 1994.
[10] P. Gunter, “Nonlinear Optical Effects and Materials,”
Springer-Verlag, Berlin, 2000.
[11] N. B. Singh, T. Henningsen, R. H. Hopkins, R. Mazelsky,
R. D. Hamacher, E. P. Supertzi, F. K. Hopkins, D. E.
Zelmon and O. P. Singh, “Nonlinear Optical Characteris-
tics of Binary Organic System,” Journal of Crystal
Growth, Vol. 128, No. 1-4, 1993, pp. 976-980.
doi:10.1016/S0022-0248(07)80081-9
[12] M. A. Savas, H. Erturan and S. Altintas, “Effects of
Squeeze Casting on the Properties of Zn-Bi Monotectic
Alloy,” Metallurgical and Materials Transactions A:
Physical Metallurgy and Materials Science, Vol. 28A,
No. 7, 1997, pp. 1509-1515.
doi:10.1007/s11661-997-0213-4
[13] K. A. Jackson and J. D. Hunt, “Lamellar and Rod Eutec-
tic Growth,” Transactions of the Metallurgical Society of
AIME, Vol. 236, 1966, pp. 1129-1142.
[14] J. W. Cahn, “Monotectic Composite Growth,” Metallur-
gical Transactions A: Physical Metallurgy and Materials
Science, Vol. 10A, No. 1, 1979, pp. 119-121.
doi:10.1007/BF02686415
[15] G. A. Chadwick, “Mettalography of Phase Transforma-
tion,” Butterworths, London, 1972.
[16] R. N. Grugel and A. Hellawell, “Alloy Solidification in
Systems Containing a Liquid Miscibility Gap,” Metallur-
gical Transactions A: Physical Metallurgy and Materials
Science, Vol. 12, No. 4, 1981, pp. 669-681.
doi:10.1007/BF02649742
[17] N. B. Singh, U. S. Rai and O. P. Singh, “Chemistry of
Eutectic and Monotectic; Phenanthrene-Succinonitrile
System,” Journal of Crystal Growth, Vol. 71, No. 2, 1985,
pp. 353-360. doi:10.1016/0022-0248(85)90091-0
[18] W. F. Kaukler and D. O. Frzier, “Crystallization Micro-
structure in Transparent Monotectic Alloys,” Nature
(London, United Kingdom), Vol. 323, 1986, pp. 50-52.
doi:10.1038/323050a0
[19] H. Song and A. Hellawell, “The Growth of Tubular or
Vermicular Structures in Organic Monotectic Systems,”
Metallurgical Transactions A: Physical Metallurgy and
Materials Science, Vol. 20A, No. 1, 1989, pp. 171-177.
doi:10.1007/BF02647504
[20] G. F. V. Voort, “Binary Phase Diagrams and Microstruc-
tures,” Materials Characterization, Vol. 41, No. 2, 1998,
pp. 69-79.
[21] B. Predel, “Constitution and Thermodynamics of
Monotectic Alloys―A Survey,” Journal of Phase Equi-
libria, Vol. 18, No. 4, 1997, pp. 327-337.
[22] B. Majumdar and K. Chattopadhyay, “The Rayleigh In-
stability and the Origin of Rows of Droplets in the
Monotectic Microstructure of Zinc-Bismuth Alloys,”
Metallurgical and Materials Transactions A: Physical
Metallurgy and Materials Science, Vol. 27A, No. 7, 1996,
pp. 2053-2057. doi:10.1007/BF02651956
[23] P. Gupta, T. Agrawal, S. S. Das and N. B. Singh, “Sol-
vent Free Reactions, Reactions of Nitrophenols in
8-Hydroxyquinoline-Benzoic Acid Eutectic Melt,” Jour-
nal of Thermal Analysis and Calorimetry, Vol. 104, No. 3,
2011, pp. 1167-1176.
[24] J. W. Rice, J. Fu and E. M. Suuberg, “Anthracene +
Pyrene Solid Mixtures: Eutectic and Azeotropic Charac-
ter,” Journal of Chemical & Engineering Data, Vol. 55,
No. 9, 2010, pp. 3598-3605. doi:10.1021/je100208e
[25] C. A. Peters, K. H. Wammer and C. D. Knightes, “Mul-
ticomponent NAPL Solidification Thermodynamics,”
Transport in Porous Media, Vol. 38, No. 1-2, 2000, pp.
57-77. doi:10.1023/A:1006615301396
[26] R. N. Rai, S. R. Mudunuri, R. S. B. Reddi, V. S. A.
Kumar Satuluri, S. Ganesamoorthy and P. K. Gupta,
“Crystal Growth and Nonlinear Optical Studies of
M-Dinitrobenzene Doped Urea,” Journal of Crystal
Growth, Vol. 321, No. 1, 2011, pp. 72-77.
doi:10.1016/j.jcrysgro.2011.02.019
[27] S. Kant, R. S. B. Reddi and R. N. Rai, “Solid-Liquid
Equilibrium, Thermal, Crystallization and Microstruc-

M. SINGH ET AL. 961
tural Studies of Organic Monotectic Alloy,” Fluid Phase
Equilibria, Vol. 291, No. 1, 2010, pp. 71-75.
doi:10.1016/j.fluid.2009.12.015
[28] B. Derby and J. J. Favier, “A Criterion for the Determina-
tion of Monotectic Structure,” Acta Metallurgica, Vol. 31,
No. 7, 1983, pp. 1123-1130.
doi:10.1016/0001-6160(83)90208-0
[29] A. Ecker, D. O. Frazier and J. I. D. Alexander, “Fluid
Flow in Solidifying Monotectic Alloys,” Metallurgical
Transactions A: Physical Metallurgy and Materials Sci-
ence, Vol. 20A, No. 11, 1989, pp. 2517-2527.
doi:10.1007/BF02666686
[30] J. A. Dean, “Lange’s Handbook of Chemistry,”
McGraw-Hill, New York, 1985.
[31] U. S. Rai and R. N. Rai, “Studies on Physicochemical
Properties of the Eutectic and Monotectic in the Urea-P.
Chloronitrobenzene System,” Journal of Crystal Growth,
Vol. 169, No. 3, 1996, pp. 563-569.
doi:10.1016/S0022-0248(96)00448-4
[32] S. Chaubey, K. S. Dubey and P. R. Rao, “Alumi-
num-Cadmium Binary Alloy Phase Diagram,” Journal of
Alloy Phase Diagram, Vol. 6, 1990, pp. 153-157.
[33] R. N. Rai, “Phase Diagram, Optical, Nonlinear Optical,
and Physicochemical Studies of the Organic Monotectic
System: Pentachloropyridine-Succinonitrile,” Journal of
Materials Research, Vol. 99, No. 5, 2004, pp. 1348-1355.
doi:10.1557/JMR.2004.0181
[34] U. S. Rai and R. N. Rai, “Physical Chemistry of Organic
Eutectics,” Journal of Thermal Analysis and Calorimetry,
Vol. 53, No. 3, 1998, pp. 883-893.
doi:10.1023/A:1010190402954
[35] W. B. Hillig and D. Turnbull, “Theory of Crystal Growth
in Undercooled Pure Liquids,” Journal of Chemical
Physics, Vol. 24, No. 4, 1956, p. 914.
doi:10.1063/1.1742646
[36] D. A. Porter and K. E. Easterling, “Phase Transformation
in Metals and Aollys,” Vokingham (U. K) co. Ltd.,
Reading, 1982.
[37] W. C. Winegard, S. Majka, B. M. Thall and B. Chalmers,
“Eutectic Solidification in Metals,” Canadian Journal of
Chemistry, Vol. 29, No. 4, 1951, pp. 320-327.
doi:10.1139/v51-037
[38] R. N. Rai and U. S. Rai, “Solid-Liquid Equilibrium and
Thermochemical Properties of Organic Eutectic in a
Monotectic System,” Thermochimica Acta, Vol. 363, No.
1-2, 2000, pp. 23-28.
doi:10.1016/S0040-6031(00)00625-0
[39] U. S. Rai and R. N. Rai, “Physical Chemistry of the Or-
ganic Analog of Metal-Metal Eutectic and Monotectic
Alloys,” Journal of Crystal Growth, Vol. 191, No. 1-2,
1998, pp. 234-242. doi:10.1016/S0022-0248(98)00105-5
[40] N. Singh, N. B. Singh, U. S. Rai and O. P. Singh, “Struc-
ture of Eutectic Melts; Binding Organic Systems,” Ther-
mochimica Acta, Vol. 95, No. 1, 1985, pp. 291-293.
doi:10.1016/0040-6031(85)80059-9
[41] J. W. Christian, “The Theory of Phase Transformation in
Metals and Alloys,” Pergamon Press, Oxford, 1965.
[42] R. Good, “Generalization of Theory for Estimation of
Interfacial Energies,” Industrial & Engineering Chemis-
try, Vol. 62, No. 3, 1970, pp. 54-78.
doi:10.1021/ie50723a009
[43] J. D. Hunt and K. A. Jackson, “Binary Eutectic Solidifi-
cation,” Transactions of the Metallurgical Society of
AIME, Vol. 236, No. 6, 1966, pp. 843-852.
[44] U. S. Rai and P. Panday, “Solidification and Thermal
Behaviour of Binary Organic Eutectic and Monotectic; Suc-
cinonitrile-Pyrene System,” Journal of Crystal Growth, Vol.
249, No. 1-2, 2003, pp. 301-308.
doi:10.1016/S0022-0248(02)01906-1
[45] R. N. Rai, U. S. Rai and K. B. R. Varma, “Thermal, Mis-
cibility Gap and Microstructural Studies of Organic Ana-
log of Metal-Nonmetal System: P-Dibromobenzene-Suc-
cinonitrile,” Thermochimica Acta, Vol. 387, No. 2, 2002,
pp. 101-107. doi:10.1016/S0040-6031(01)00833-4