Optics and Photonics Journal, 2011, 1, 189-196
doi:10.4236/opj.2011.14030 Published Online December 2011 (http://www.SciRP.org/journal/opj)
The Design and Experiment Studies of Optical Adapter
with Integrated Dust Protection and Shutter Design
Samuel I-En Lin
Department of Power Mechanical Engineering National Formosa University, Chinese Taipei
Received August 10, 2011; revised September 9, 2011; accepted September 21, 2011
Abstract
High power lasers (> +21dBm) have gradually become the common solution for signal transmitting systems
including regional cable television, Fiber To The Home (FTTH), and gigabit passive optical networks (G-
PONs) due to their ability to generate signals that can be transmitted over long distances. However, if protec-
tive design is not implemented in the facility at the client side, users may be exposed to health hazards such
as eye damage from these high-power lasers. High-power optical adapters with laser shutter use metal masks
to prevent eye exposure to direct laser beams. They have progressively replaced conventional optical adapt-
ers and entered the market mainstream. Our study uses the Elastic-Plastic theory together with parametric
design to investigate the effect of geometry on the initial spring-back angle of a laser shutter. Once the force
stabilizes, the angles of the initial spring back are found to be the same as the simulated results for several
attempts. In our study, it is observed that factors including the thickness of the metal masking plate, the ini-
tial design angle, the stiffness of the material and the boundary conditions have significant influence on the
spring back angle. These can be used as references in design control.
Keywords: High Power Leaser, Elastic-Plastic Theory, Laser Shutter, Optical Adapter
1. Introduction
In 1966, researchers Kao and Hockham [1] proposed the
theory of fiber optics and presented its potential applica-
tion. Over time, the quality of optical communication
improved extensively, and the bandwidth for communi-
cation increased considerably. To date, fiber optics has
become an essential medium of communication. High
power light sources (> +21dBm) are used in transmitting
data in fiber optic networks, and are additionally used in
cable television. The common wavelengths used in to-
day’s high power telecom lasers (1310 nm, 1550 nm, etc.)
fall in the infrared, outside the visible portion of the
spectrum. When these high power laser beams are emit-
ted from the fiber optical terminal, human eyes are there-
fore unable to recognize the hazard [2-4]. It is therefore
required to install protective terminal units (for example,
laser shutter) to prevent accidental ocular exposure to the
high power laser beams. Integrated laser shutter have
many benefits [5], as their geometric structure is fully
compatible with IEC/TIA specifications, and their phy-
sical dimensions can be fully compatible with any front
panel board on the market. In addition to their suitability
for automated production, their cost is very low. Metal
plates have the ability to return to their original position
when bent within the region of elastic deformation. The
functionality of integrated laser shutter relies on this
property. Figure 1 illustrates the sample application of
an integrated laser shutter on an SC-type adaptor. The
same structure can be applied in various optical commu-
nication modules. Functions for preventing dust can also
be included [6].
To ensure the unit can withstand repeated blocking
and unblocking, the design analysis and stress analysis of
the metal masking plates are very important factors. After
a period of operation time, if a plate malfunctions and is
unable to be restored to the design angle, laser beams may
leak out and present a hazard, and dust could also enter
the laser module which in turn reduces the laser life time.
Tekaslan [7] studied the material property of stamped
stainless steels, investigating the effects of different plate
thicknesses and horizontal angles on the spring back an-
gles. They found that a greater plate thickness and a lar-
ger horizontal angle produce a larger spring back angle.
Chang and Hsu [8] stamped and deformed stainless steel
plates with a thickness of 0.1 mm (JIS SUS 301). When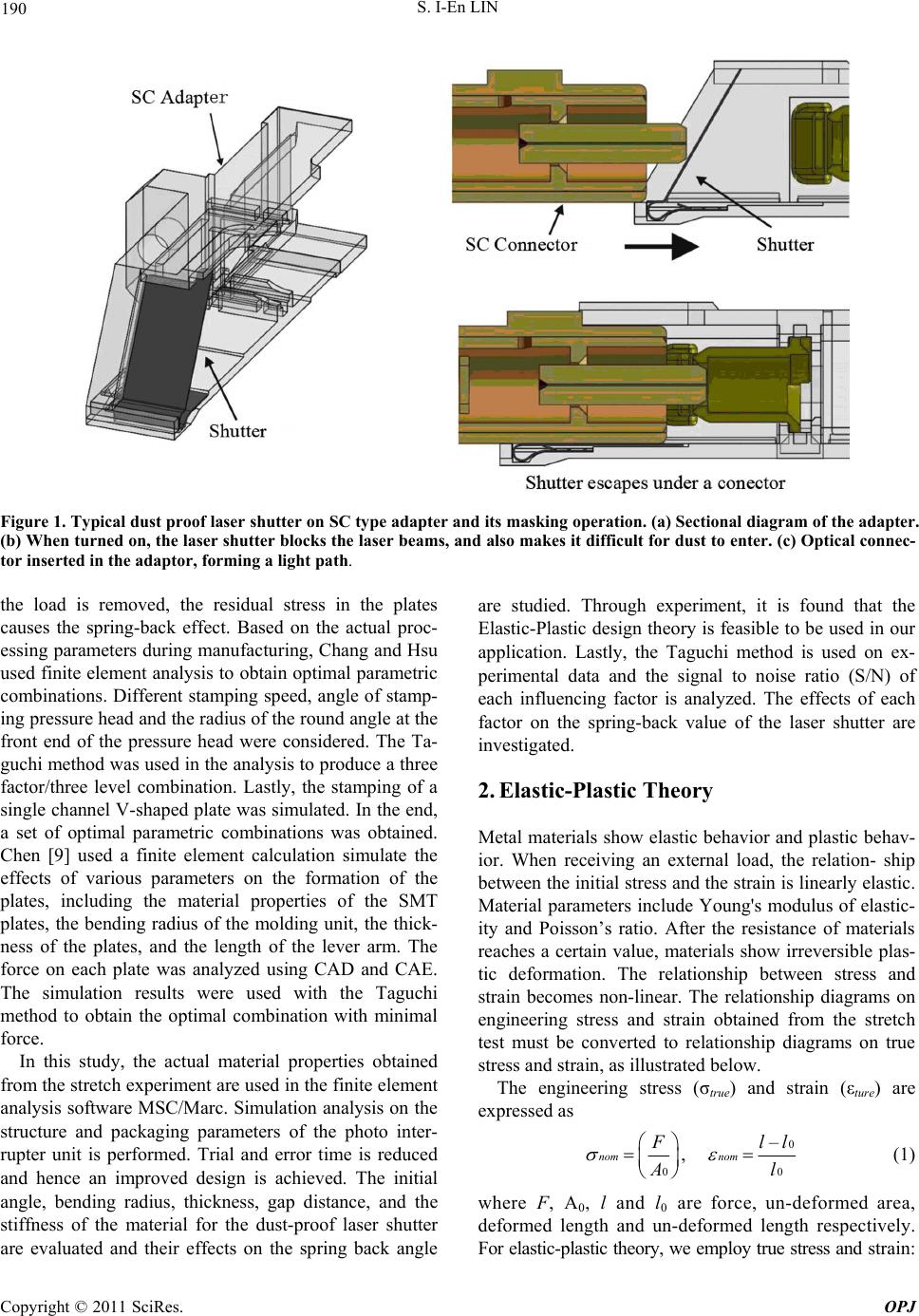

S. I-En LIN
190
Figure 1. Typical dust proof lase r shutter on SC type adapter and its masking operation. (a) Sectional diagram of the adapter.
(b) When turned on, the laser shutter blocks the laser beams, and also makes it difficult for dust to enter. (c) Optical connec-
tor inserted in the adaptor, forming a light path.
the load is removed, the residual stress in the plates
causes the spring-back effect. Based on the actual proc-
essing parameters during manufacturing, Chang and Hsu
used finite element analysis to obtain optimal parametric
combinations. Different stamping speed, angle of stamp-
ing pressure head and the radius of the round angle at the
front end of the pressure head were considered. The Ta-
guchi method was used in the analysis to produce a three
factor/three level combination. Lastly, the stamping of a
single channel V-shaped plate was simulated. In the end,
a set of optimal parametric combinations was obtained.
Chen [9] used a finite element calculation simulate the
effects of various parameters on the formation of the
plates, including the material properties of the SMT
plates, the bending radius of the molding unit, the thick-
ness of the plates, and the length of the lever arm. The
force on each plate was analyzed using CAD and CAE.
The simulation results were used with the Taguchi
method to obtain the optimal combination with minimal
force.
In this study, the actual material properties obtained
from the stretch experiment are used in the finite element
analysis software MSC/Marc. Simulation analysis on the
structure and packaging parameters of the photo inter-
rupter unit is performed. Trial and error time is reduced
and hence an improved design is achieved. The initial
angle, bending radius, thickness, gap distance, and the
stiffness of the material for the dust-proof laser shutter
are evaluated and their effects on the spring back angle
are studied. Through experiment, it is found that the
Elastic-Plastic design theory is feasible to be used in our
application. Lastly, the Taguchi method is used on ex-
perimental data and the signal to noise ratio (S/N) of
each influencing factor is analyzed. The effects of each
factor on the spring-back value of the laser shutter are
investigated.
2. Elastic-Plastic Theory
Metal materials show elastic behavior and plastic behav-
ior. When receiving an external load, the relation- ship
between the initial stress and the strain is linearly elastic.
Material parameters include Young's modulus of elastic-
ity and Poisson’s ratio. After the resistance of materials
reaches a certain value, materials show irreversible plas-
tic deformation. The relationship between stress and
strain becomes non-linear. The relationship diagrams on
engineering stress and strain obtained from the stretch
test must be converted to relationship diagrams on true
stress and strain, as illustrated below.
The engineering stress (σtrue) and strain (εture) are
expressed as
0
00
–
,nom nom
F
ll
A
l





(1)
where F, A0, l and l0 are force, un-deformed area,
deformed length and un-deformed length respectively.
For elastic-plastic theory, we employ true stress and strain:

S. I-En LIN 191
 
1, 1true nomnomtruenomln     (2)
For material configuration in MSC/Marc, if the data
from the stretch test is directly entered for the material
property, this method is known as the direct input of
experimental data. In our study, the test data points en-
tered are different. The engineering stress and engineer-
ing strain obtained from stretch test are converted to true
stress (σtrue) and true strain (εture) within the plastic range
(Equation 2).
In addition to entering the relationship between true
stress and true strain, the elastic-plastic theory can
determine the power law elastic-plastic behavior. The
relationship is expressed as:

m
true true
K   (3)
where K is the true stress when the true strain is equal to
1-also known as the strength constant-and m is the stiff-
ness index. Take the log of the equation to obtain the
values for K and m using the least squares method. For
multiple groups of data, the average value may be taken to
obtain an average strength constant and average strain
stiffness index. Due to the fact that MSC/Marc requires
the input parameters to be the true stress and true strain
within the plastic region, equation in [10] is used:
–tp true
Y
K

  (4)
We rearrange (3) to:
B
Y
ln K
m
tp tp Ae












(5)
The yield strength σY, strength constant K, stiffness
index m, and power law model parameters A and B can
all be obtained from experiment [11]. In MSC/Marc, we
can configure two independent material variables (true
strains within the temperature range and plastic range).
The total stress obtained from the experiment is re-
presented by two independent functions. During the si-
mulation, to study the effects of temperature change on
displacement, it is easier to directly input experimental
data. If temperature factors are not considered, the diffe-
rence between using the power law model or directly using
experimental data is unnoticeable. In our study, the mate-
rial properties are based on data obtained directly from
the experiment.
3. Simulation Model and Experiment Setup
The simulation built for this investigation is mainly used
to study the effects of different parameters on the dis-
placement of the laser shutter. The interactions between
these controlling parameters are not included in the
scope of this study.
3.1. Model Construction and Limitations
Numerous design limitations exist for the integrated laser
shutter since the original adapter modules must comply
with regulations including IEC/TIA. The laser shutter
component is only an accessory whose function is to
block laser beams from leaking when the adapter is being
unplugged. To achieve an accurate simulation the models
are rendered in 3D. The optical fiber connector is con-
sidered as a rigid body. When plugging in the connector,
it presses onto the laser shutter component. When un-
plugging the connector, the laser shutter component re-
turns to certain angle (known as the spring-back angle) to
block the laser beams in time (Figure 2). The spring
back angle (θ) must be large enough to be able to block
the laser beams completely and to fully seal to prevent
dust from entering. The spring-back angle is therefore a
very important figure in the design.
Quasi-static analysis is used in our study. The degrees
of freedom for the boundary conditions for the bottom
part of the laser shutter component are fixed (Figure 2).
We make the following basic assumptions in the simulation:
a) It is a quasi-static process. The movement of the
adapter is slow and we ignore the effects of strain
rate. The fiber optical adapter and fiber optical con-
nectors are totally rigid bodies.
b) The masking plates are made of homogenous and
isotropic materials which obey the yield criteria of
von Mises. Materials with lattices oriented in the same
direction are used in the simulation as well as real
sample production.
Figure 2. Simulated laser shutter. The optical connector
and adaptor are treated as rigid bodies. The optical con-
nector moves to the right and presses on the laser shutter,
then returns to the initial position. This is to measure the
spring-back angle. T is the thickness of the shutter plate
and D is the control space of shutter base-plate.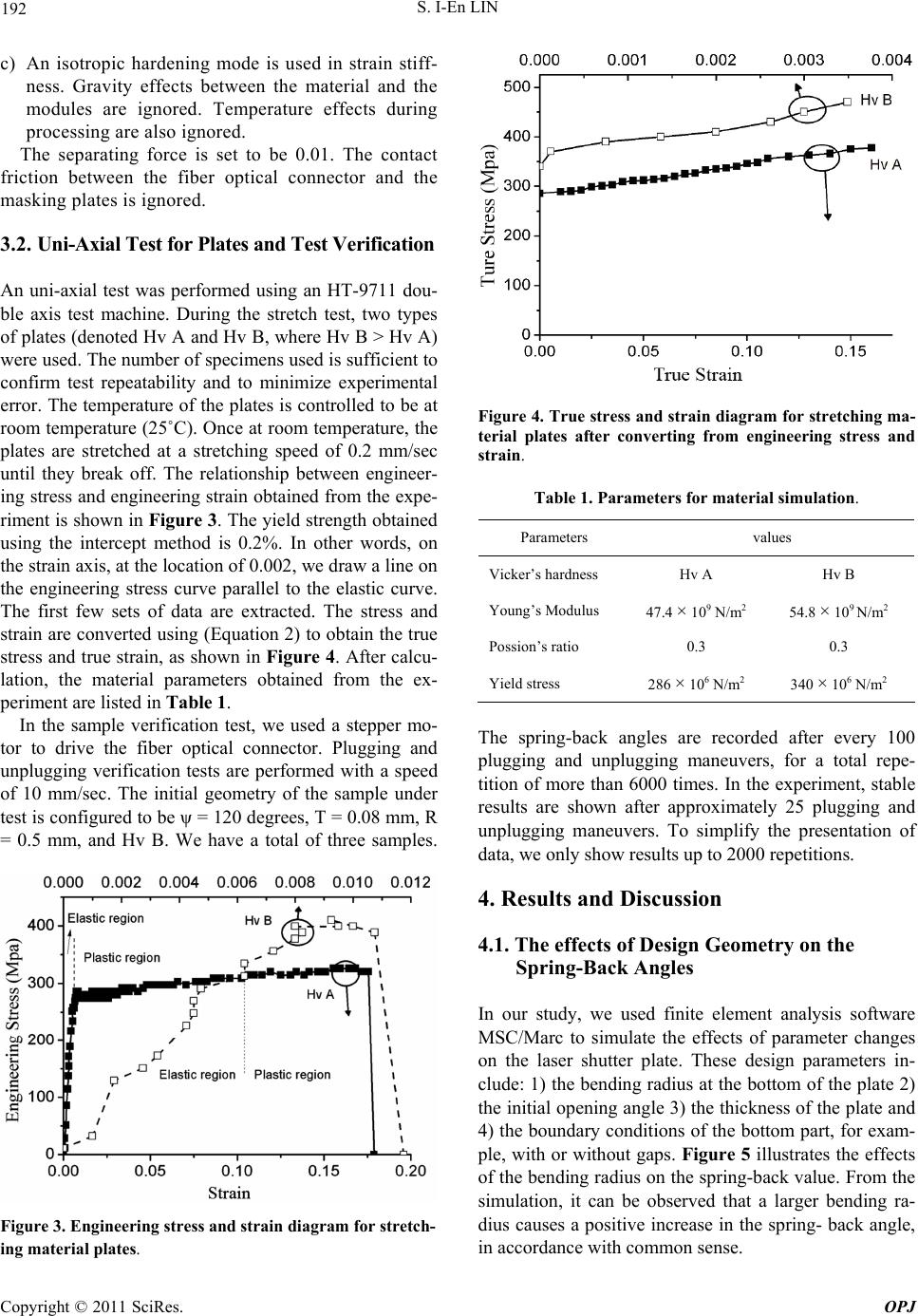

S. I-En LIN
192
c) An isotropic hardening mode is used in strain stiff-
ness. Gravity effects between the material and the
modules are ignored. Temperature effects during
processing are also ignored.
The separating force is set to be 0.01. The contact
friction between the fiber optical connector and the
masking plates is ignored.
3.2. Uni-Axial Test for Plates and Test Verification
An uni-axial test was performed using an HT-9711 dou-
ble axis test machine. During the stretch test, two types
of plates (denoted Hv A and Hv B, where Hv B > Hv A)
were used. The number of specimens used is sufficient to
confirm test repeatability and to minimize experimental
error. The temperature of the plates is controlled to be at
room temperature (25˚C). Once at room temperature, the
plates are stretched at a stretching speed of 0.2 mm/sec
until they break off. The relationship between engineer-
ing stress and engineering strain obtained from the expe-
riment is shown in Figure 3. The yield strength obtained
using the intercept method is 0.2%. In other words, on
the strain axis, at the location of 0.002, we draw a line on
the engineering stress curve parallel to the elastic curve.
The first few sets of data are extracted. The stress and
strain are converted using (Equation 2) to obtain the true
stress and true strain, as shown in Figure 4. After calcu-
lation, the material parameters obtained from the ex-
periment are listed in Table 1.
In the sample verification test, we used a stepper mo-
tor to drive the fiber optical connector. Plugging and
unplugging verification tests are performed with a speed
of 10 mm/sec. The initial geometry of the sample under
test is configured to be ψ = 120 degrees, T = 0.08 mm, R
= 0.5 mm, and Hv B. We have a total of three samples.
Figure 3. Engineering stress and strain diagram for stretch-
ing material plates.
Figure 4. True stress and strain diagram for stretching ma-
terial plates after converting from engineering stress and
strain.
Table 1. Parameters for material simulation.
Parameters values
Vicker’s hardness Hv A Hv B
Young’s Modulus 47.4 × 109 N/m2 54.8 × 109 N/m2
Possion’s ratio 0.3 0.3
Yield stress 286 × 106 N/m2 340 × 106 N/m2
The spring-back angles are recorded after every 100
plugging and unplugging maneuvers, for a total repe-
tition of more than 6000 times. In the experiment, stable
results are shown after approximately 25 plugging and
unplugging maneuvers. To simplify the presentation of
data, we only show results up to 2000 repetitions.
4. Results and Discussion
4.1. The effects of Design Geometry on the
Spring-Back Angles
In our study, we used finite element analysis software
MSC/Marc to simulate the effects of parameter changes
on the laser shutter plate. These design parameters in-
clude: 1) the bending radius at the bottom of the plate 2)
the initial opening angle 3) the thickness of the plate and
4) the boundary conditions of the bottom part, for exam-
ple, with or without gaps. Figure 5 illustrates the effects
of the bending radius on the spring-back value. From the
simulation, it can be observed that a larger bending ra-
dius causes a positive increase in the spring- back angle,
in accordance with common sense.

S. I-En LIN 193
Figure 5. The effects of bending radius at the bottom of the
laser shutter on the spring-back angle.
The effects of initial opening angles (ψ) for two mater-
ials with different hardness on the spring-back angle are
illustrated in Figure 6. In the figure, it can be observed
that, for the same materials with different stiffnesses, the
initial opening angle does affect the spring-back angle. In
addition, compared to materials with lower stiffness (Hv
A), materials with higher stiffness (Hv B) have greater
effects on the spring-back angle. For fixed geometric and
material parameters, the effects of these two thicknesses
on the spring-back angles are analyzed, as shown in
Figure 7. A thinner thickness has better effects on the
spring-back angles of the masking plate. This completely
agrees with the real life experience for common spring-
back analysis. The fixture method for the base of the
laser shutter component does affect the manufactur- ing
assembly method. If we aim to have total enclosure,
every component must be sealed together without gaps.
This would introduce difficulties during the packaging
process. Greater gaps ease the automation process during
manufacturing, but we must consider the effects on the
spring-back angles. Analyzed results in Figure 8 illus-
trate that under ideal conditions where all components
are fixed (Type-I), the spring-back angle is at its opti-
mum. In Type-II, packaging gaps exist at the base.
Greater gaps produce smaller spring-back angles. During
the design process, it is required to have a solution where
both concerns are considered.
4.2. Effects of Changes in Material Property on
the Spring-Back Angles
Properties like material ductility often depend on
processing temperature; this in turn affects the spring-
back value. In our study, two materials with different
stiffness are used for comparison, where Hv B has 32%
higher stiffness than Hv A, and more yield stress, as
Figure 6. The effects of the initial angle of the laser shutter
on the spring-back angle.
Figure 7. The effects of the thickness the laser shutter on
the spring-back angle.
Figure 8. The effects of boundary conditions on the spring-
back angle.

S. I-En LIN
194
obtained from the data from the stretching test. In Figure
9, we have compared materials with the same geometric
conditions but with different stiffness. Due to the fact
that the material of which Hv B is composed demon-
strates better resistance to deformation, analyzed results
show that it has a greater spring-back angle. However, it
is not suitable to only aim for higher stiffness, as the lat-
tice could break if the stress is concentrated at the bend-
ing area, and in turn cause the entire masking purpose to
malfunction. It is necessary to perform experiments (re-
peated plugging and unplugging) to verify its operational
life time. Through analysis, it can be observed that Hv B
shows better results than Hv A, and therefore only Hv B
materials are used for the repeated plugging and unplug-
ging experiment (Figure 10). Under the condition where
T=0.08mm, after stabilization, the spring-back angles are
measured. Data from simulation agrees with the data
obtained from the experiment. This confirms that the
elastic-plastic theory can be applied in the design of laser
shutter components.
Figure 9. The effects of material stiffness on the spring-
back angle.
Figure 10. Comparing spring-back angle from experiment
and simulation for sample Hv B.
4.3. Taguchi method
The Taguchi analysis method is used in order to optimize
the design. We first analyze the properties of important
parameters to determine the number of factors and levels,
and also to judge if interaction effects exist. We then
select the suitable orthogonal arrays to perform factor
allocation which are later to be used as a baseline for
system design and execution. In the process of paramet-
ric design, the main aim is to determine the optimum
combination for factors and levels. This is based on the
value of S/N. Among all combinations, there is always
one with the largest S/N value; this combination simul-
taneously optimizes all factors. Through analysis, it can
be seen that if we control the behavior of a factor at a
certain level within a particular region, a larger S/N
value means a smaller degree of variation in quality, and
we will then be one step closer to the ideal goal.
Based on the analyzed results mentioned above, we
determine the important factors to be (1) the bending
radius at the base of the plate (2) the initial opening angle
and (3) the thickness of the plate. Levels for each factor
are listed in Table 2. The corresponding orthogonal ta-
bles L9(33) are constructed as illustrated in Table 3.
In this table, each factor/level combination appears
three times. This method is very direct and very eco-
nomical. Experiments involving many factors can be
carried outsimultaneously. Using the repeatability of the
experiment, each influential factor in the orthogonal ta-
ble can be examined to determine an effective design
while limiting the number of experiments.
Based on the parameter values for the factors, the
maximum average S/N ratio for each factor level is de-
termined. For materials with stiffness Hv A and Hv B,
we analyze their S/N ratio separately. For Hv A, Figure
11 shows the factor diagram for the “larger the better”
(LTB) characteristic. In Table 2, the combination for the
level of the optimal LTB factor is A2 = 0.4, B1 = 100,
C2 = Type-I 0.08. The orthogonal table in Table 3 L9(33)
shows the optimal combination is the fourth group, and it
also has a better value for spring-back angle. For Hv B,
Table 2. Taguchi controlling factor levels.
Geometric ParametersR ψ B
Factors A B C
Level 1 0.3 100 Type-i
Level 2 0.4 120 Type-ii 0.08
Level 3 0.5 140 Type-ii 0.1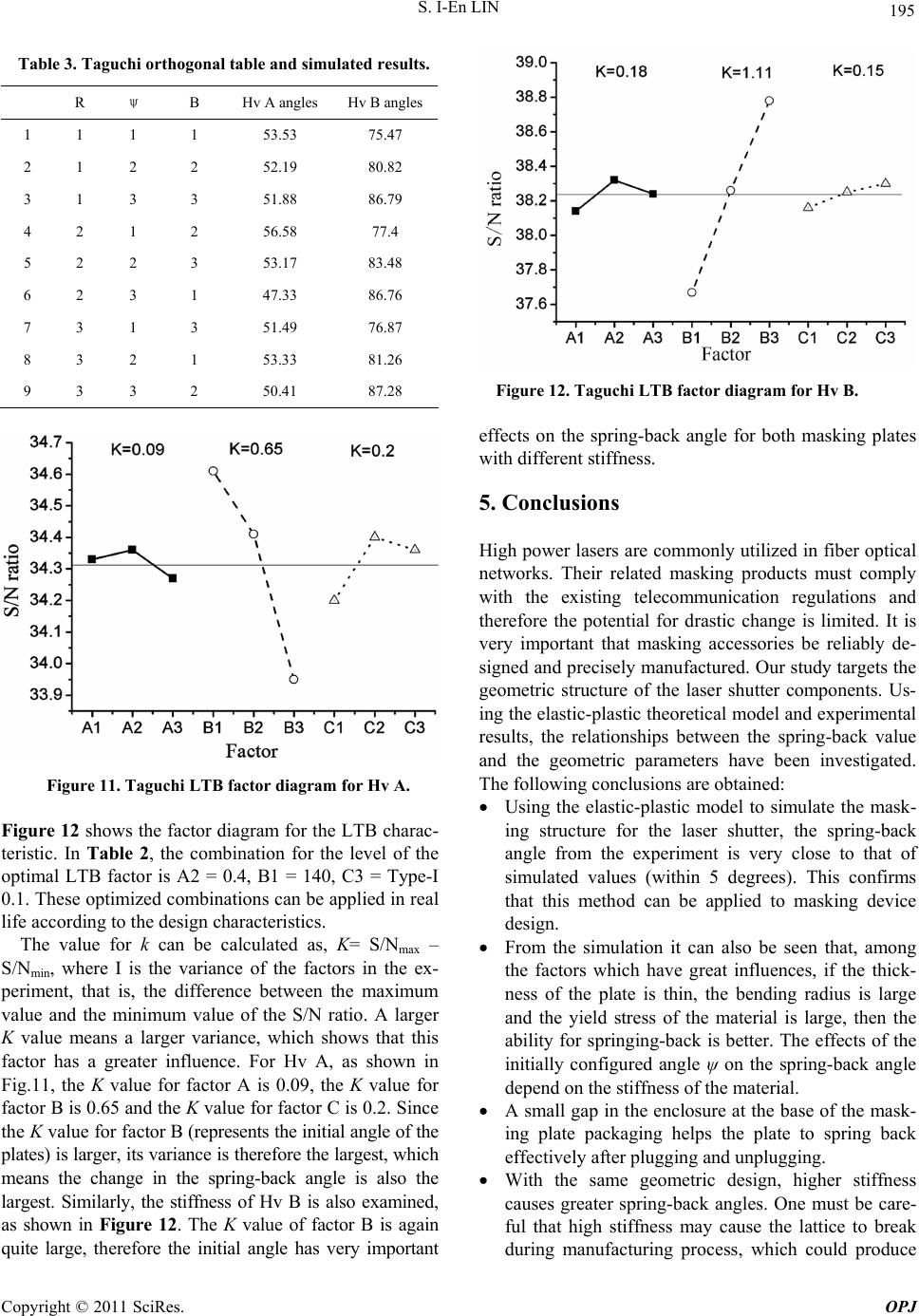

S. I-En LIN 195
Table 3. Taguchi orthogonal table and simulated results.
R ψ B Hv A angles Hv B angles
1 1 1 1 53.53 75.47
2 1 2 2 52.19 80.82
3 1 3 3 51.88 86.79
4 2 1 2 56.58 77.4
5 2 2 3 53.17 83.48
6 2 3 1 47.33 86.76
7 3 1 3 51.49 76.87
8 3 2 1 53.33 81.26
9 3 3 2 50.41 87.28
Figure 11. Taguchi LTB factor diagram for Hv A.
Figure 12 shows the factor diagram for the LTB charac-
teristic. In Table 2, the combination for the level of the
optimal LTB factor is A2 = 0.4, B1 = 140, C3 = Type-I
0.1. These optimized combinations can be applied in real
life according to the design characteristics.
The value for k can be calculated as, K= S/Nmax –
S/Nmin, where I is the variance of the factors in the ex-
periment, that is, the difference between the maximum
value and the minimum value of the S/N ratio. A larger
K value means a larger variance, which shows that this
factor has a greater influence. For Hv A, as shown in
Fig.11, the K value for factor A is 0.09, the K value for
factor B is 0.65 and the K value for factor C is 0.2. Since
the K value for factor B (represents the initial angle of the
plates) is larger, its variance is therefore the largest, which
means the change in the spring-back angle is also the
largest. Similarly, the stiffness of Hv B is also examined,
as shown in Figure 12. The K value of factor B is again
quite large, therefore the initial angle has very important
Figure 12. Taguchi LTB factor diagram for Hv B.
effects on the spring-back angle for both masking plates
with different stiffness.
5. Conclusions
High power lasers are commonly utilized in fiber optical
networks. Their related masking products must comply
with the existing telecommunication regulations and
therefore the potential for drastic change is limited. It is
very important that masking accessories be reliably de-
signed and precisely manufactured. Our study targets the
geometric structure of the laser shutter components. Us-
ing the elastic-plastic theoretical model and experimental
results, the relationships between the spring-back value
and the geometric parameters have been investigated.
The following conclusions are obtained:
 Using the elastic-plastic model to simulate the mask-
ing structure for the laser shutter, the spring-back
angle from the experiment is very close to that of
simulated values (within 5 degrees). This confirms
that this method can be applied to masking device
design.
 From the simulation it can also be seen that, among
the factors which have great influences, if the thick-
ness of the plate is thin, the bending radius is large
and the yield stress of the material is large, then the
ability for springing-back is better. The effects of the
initially configured angle ψ on the spring-back angle
depend on the stiffness of the material.
 A small gap in the enclosure at the base of the mask-
ing plate packaging helps the plate to spring back
effectively after plugging and unplugging.
 With the same geometric design, higher stiffness
causes greater spring-back angles. One must be care-
ful that high stiffness may cause the lattice to break
during manufacturing process, which could produce

S. I-En LIN
196
small gaps. Reliability tests are recommended for ve-
rification purposes.
6. References
[1] K. C. Kao and G. A. Hockham, “Dielectric-Fibre Surface
Waveguides For Optical frequencies,” Proc, IEE, Vol.
133, No. 7, 1966, pp.1151-1158
[2] S. I. E. Lin, “Dust Cap for Fiber Optic Adapter,” US
Patent No. 0310795, 2008.
[3] S. I E. Lin, Fiber optic adapter with integrated shutter, US
patent, 2009/0028507.
[4] S. I. E. Lin, “Dust Shutter for Optical Adapter,” US Pat-
ent No. 0101758, 2008.
[5] “SANWA Electronic Co. Laser Shutter,” Japanese Patent
No. 2008-225133 A, 25 September 2008.
[6] Fitel Product catalog, MPO Adapter with Shutter Design,
Furukawa Electronics, Japan, 2010.
[7] Ö. Tekaslan, N. Gerger and U. Şeker, “Determination of
Spring-Back of Stainless Steel Sheet Metal in ‘V’ Bend-
ing Dies,” Materials and Design, Vol. 29, No. 5 ,2008, pp.
1043-1050. doi:10.1016/j.matdes.2007.04.004
[8] C. C. Chang and F. M. Hsu, “Effects of Punch Radius
and Offset on the Spring Back of Micro Right Angle
Bending,” Master dissertation, National Kaohsiung Uni-
versity of Applied Sciences, Taiwan, 2007.
[9] W. Y. Chiou and M. H. Chen, “The Optimal Design and
Stress Analysis for SMT Spring Finger,” Master disserta-
tion, National Kaohsiung University of Applied Sciences,
Taiwan, 2010.
[10] “MSC\Marc 2010 User Manual,” MSC Software Corpo-
ration, USA, 2010.
[11] Y. Ueda, K. Lida, M. Saito and A. Okamoto, “Finite
Element Model and Residual Stress Calculation for
Multi-Pass Welded Joint between a Sheet Metal and the
penetrating Pipe,” Modeling of Casting, Welding and
Advanced Solidification Processes-V, 1991, pp. 219-227.
[12] W. K. Dai, “A Study on Stent Plastic Forming,” Master
dissertation, National Sun Yat-sen University, Taiwan,
2004.
[13] D. Fratila and C. Caizar, “Application of Taguchi Method
to Selection of Optimal Lubrication and Cutting Condi-
tions in Face Milling of AlMg3,” Journal of Cleaner Pro-
duction, Vol. 19, No. 6-7, 2011, pp. 640-645.
doi:10.1016/j.jclepro.2010.12.007
[14] F. O. Sonmez and A. Demir, “Analytical Relations between
Hardness and Strain for Cold Formed Parts,” Journal of
Materials Processing Technology, Vol. 186, No. 1- 3,
2007, pp. 163-173. doi:10.1016/j.jmatprotec.2006.12.031