 Journal of High Energy Physics, Gravitation and Cosmology, 2019, 5, 167-180 http://www.scirp.org/journal/jhepgc ISSN Online: 2380-4335 ISSN Print: 2380-4327 10.4236/jhepgc.2019.51009 Dec. 20, 2018 167 Journal of High Energy Physics, Gravitation Broken Charges Associated with Classical Spacetime Symmetries under Canonical Transformation in Real Scalar Field Theory Susobhan Mandal Department of Physical Sciences, Indian Institute of Science Education and Research Kolkata, Mohanpur, India We know from Noether’s theorem that there is a conserved charge continuous symmetry. In General Relativity, Killing vectors describe spacetime symmetries and to each such Killing vector field, conserved charge through stress- energy tensor of matter which is mentioned in the article. In this article, I show that under simple set of canonical trans- formation of most general class of Bogoliubov transformation between crea- tion, annihilation operators, those charges associated with spacetime symme- tries are broken. To do that, I look at stress- energy tensor of real scalar field theory (as an example) in curved spacetime and show how it changes under simple canonical transformation whic h is enough to justify our claim. Since doing Bogoliubov transformation is equivalent to coordinate transformation which according to Einstein’s equivalence principle is equivalent to turn on effect of gravity, therefore, we can say that under the effect o charges are broken. Keywords Noether’s Theorem, Killing Vector Field, Einstein’s Equivalence, Broken Charges 1. Introduction In [1], I have shown that internal symmetry like U(1) symmetry of matter field under canonical transformation [2] [3] breaks down. We all know that doing Bogoliubov transformation [4] is equivalent to turning on the effect of gravity by going into different frame. And Killing vector fields [5] [6] of a spacetime decides the symmetries of that spacetime. We also know that stress-energy tensor [5] [6] of matter fields in curved spacetime is covariantly conserved [6]. Mandal, S. (2019 ) Broken Charges Associated with Classical Spacetime Symmetries under Canonical Transformation in Real Scalar Field Theory Physics , Gravit , , 167-180. //doi.org/10.4236/jhepgc.2019.51009 November 5, 2018 December 17, 2018 December 20, 2018 9 by author and Research Publishing Inc. This work is licensed under the Creative Commons Attribution International 4.0). http://creativecommons.org/licenses/by/4.0/  S. Mandal 10.4236/jhepgc.2019.51009 168 Journal of High Energy Physics, G Therefore, let be the stress-energy tensor of the matter field and be a killing vector field of a spacetime we consider then one can show that is a covariantly conserved current [6] ( ) () () 1 0 2 TTT µνµνµν µνµνµννµ ξξξξ ∇=∇+∇+∇= (1) where we have used the symmetry property of stress-energy tensor under contravariant indices, Killing equation [5] [6] [7] and covariantly conserved property of stress-energy tensor. Therefore, we can say that from stress-energy tensor we can construct the generators of spacetime symmetry transformation which is defined on spacelike hypersurfaces. In this article, I will show that under Bogoliubov transformation, generator (conserved charge) is broken [8] [9]. Here also I use the concept that in thermodynamic limit or infinite volume limit Bogoliubov tranformation creates two inequivalent representations of two disjoint Fock space which is often used in quantum many body systems. Because of such inequivalent disjoint vector spaces, the operators both in original form and ones after transformation have their own separate domain to act on states. 2. Breakdown of Translation Invariance of Minkowski Spacetime under Canonical Transformation Here I am going to discuss my main motivation behind this work. Let’s consider a massive real scalar field theory in Minkowski spacetime with field decomposition ( ) () 3 † 3 ˆ d ˆˆ ee 2 π 2 ikxikx x k aa φ ω −⋅⋅ =+ ∫ kk k (2) therefore is equivalent to doing following transformation ˆˆ e ˆˆ e ika ika aa aa ⋅ −⋅ → → kk kk †† (3) The Hamiltonian of the matter field becomes (4) which is invariant under following transformation ˆˆ e ˆˆ e ika ika aa aa ⋅ −⋅ → → kk kk †† (5) where is a 4-vector, which we would expect because spacetime translation is Killing vector fields in Minkowski spacetime. Under the canonical transformation mentioned in [1] () † ˆˆˆ coshsinhcaa θθθ − =− kkkkk (6) the above Hamiltonian becomes  S. Mandal 10.4236/jhepgc.2019.51009 169 Journal of High Energy Physics, G () ( ) 3 3 3 22 322 ˆ ˆ ˆ d ˆ ˆ ˆ ˆ dcoshsinhcoshsinh ˆ ˆˆ ˆ ˆˆ ˆ ˆ dsinhcosh coshsinh ˆ ˆ ˆ ˆˆ dcoshsinhsinhcosh k Hkaa kcccc kcccccccc kccccc ε εθθθθ εθθθθ εθθθθ −− −−−− −− = =++ =+++ +++ ∫ ∫ ∫ ∫ kkk kkkkkkkk kkkkkkkkkkkkk kkkkkkkkkk † † † †† † † † ( ) ˆ c − k † † (7) where we have used the inverse transformation ( ) ( ) † ˆ ˆ ˆ coshsinh ˆ ˆ ˆ coshsinh ˆ ˆ ˆ coshsinh ˆˆ ˆ coshsinh caa caa acc acc θθθ θθθ θθ θθ − − − − =− =− =+ =+ kkkkk kkkkk kkkkk kkkkk † † † † † (8) Note that because of the last term in the transformed Hamiltonian it breaks the invariance under the tranformation †† ˆ ˆ e ˆˆ e ika ika cc cc ⋅ −⋅ → → kk kk (9) If one notes carefully he/she would find that for this case only the time translational symmetry breaks but the spatial translation symmetry is maintained. But this is just an example. If we rather take more general transformation ()() 3 ˆˆˆ d,,akcc αβ ′′ ′′′ =+ ∫ kkk kkkk † (10) such that ()() 22 3 d,,1,k αβ ′′′ −=∀ ∫ kkkkk (11) Then Hamiltonian becomes highly non-local () () () () ()() () ()()() ( )() 11 22 12 1212 12 333** 1211 22 333* 1212 ** 1212 * 12 ˆ ˆˆ ddd,, ˆˆ ,, ˆˆ ddd,, ˆ ˆˆˆ ,,,, ˆˆ ,, Hkkkcc cc kkkcc cccc cc εαβ αβ εαα βββα αβ =+ ×+ = ++ + ∫ ∫ kkk kk kkk kkkk kk kkkk kkkk kkkk kkkkkkkk kkkk † † † † †† (12) This expression undoubtedly suggests that the above non-local Hamiltonian is not invariant under the tranformation . † ˆˆ e ˆˆ e ika ika cc cc −⋅ → → kk kk † (13) which shows that under most general Bogoliubov transformation spacetime translation symmetry of Minkowski spacetime breaks down. As an example we want to mention cosmological particle creation example shown in [10] where these Bogoliubov coeffecients are calculated for conformal spacetime with following metric 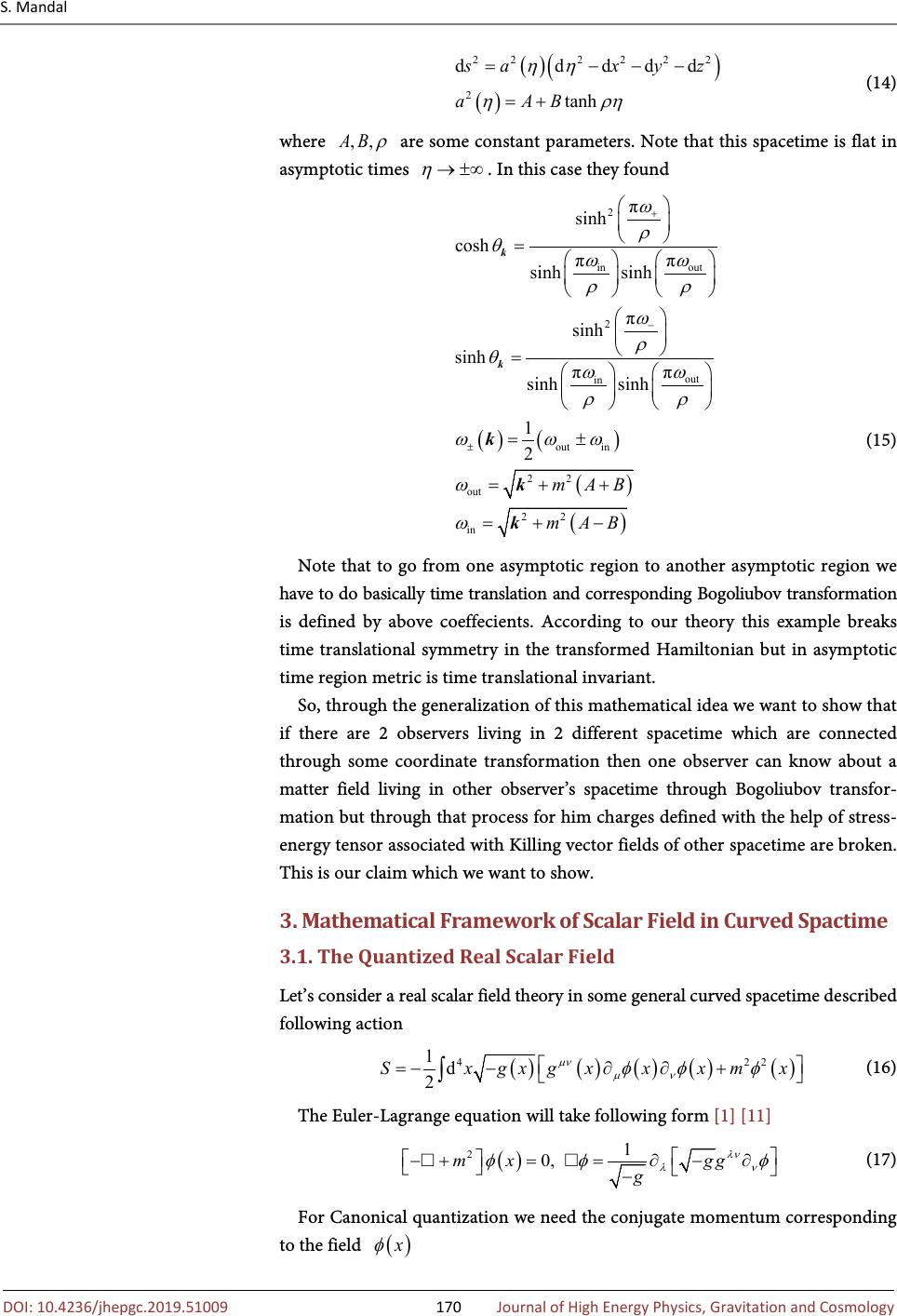 S. Mandal 10.4236/jhepgc.2019.51009 170 Journal of High Energy Physics, G () () () 222222 2 dd ddd tanh saxyz aAB ηη ηρη =−−− =+ (14) where are some constant parameters. Note that this spacetime is flat in asymptotic times . In this case they found () () () () 2 out in 2 out in outin 22 out 22 in π sinh cosh π π sinhsinh π sinh sinh π π sinhsinh 1 2 mAB mAB ω ρ θ ω ω ρρ ω ρ θ ω ω ρρ ωωω ω ω + − ± = = =± =++ =+− k k k k k (15) Note that to go from one asymptotic region to another asymptotic region we have to do basically time translation and corresponding Bogoliubov transformation is defined by above coeffecients. According to our theory this example breaks time translational symmetry in the transformed Hamiltonian but in asymptotic time region metric is time translational invariant. So, through the generalization of this mathematical idea we want to show that if there are 2 observers living in 2 different spacetime which are connected through some coordinate transformation then one observer can know about a matter field living in other observer’s spacetime through Bogoliubov transfor- mation but through that process for him charges defined with the help of stress- energy tensor associated with Killing vector fields of other spacetime are broken. This is our claim which we want to show. 3. Mathematical Framework of Scalar Field in Curved Spactime 3.1. The Quantized Real Scalar Field Let’s consider a real scalar field theory in some general curved spacetime described following action ()()()()() 422 1 d 2 Sxgxgxxxmx µν µν φφφ =−−∂∂+ ∫ (16) The Euler-Lagrange equation will take following form [1] [11] () 2 1 0,mxgg g λν λν φφφ −+==∂−∂ − (17) For Canonical quantization we need the conjugate momentum corresponding to the field  S. Mandal 10.4236/jhepgc.2019.51009 171 Journal of High Energy Physics, G (18) Using this information one can easily write down the Hamiltonian density ( ) 2 0022 00 1 2 ij ij gggm φφφφφ =Π∂−=−−∂+∂∂+ (19) and in other way we can write the Hamiltonian as [] 333 000 11 ddd 22 Hxxx φφφ ==Π∂=Π∂−∂Π ∫∫∫ (20) which can be shown through little bit of algebra. Now note that the last formula give us ( )( ) () 0 ˆ ˆ ˆ ,,,tHtit φφ =∂ xx (21) which we get through the quantization prescription ()() () () ()()()() 3 ˆ ˆ ,,, ˆˆ ˆˆ ,, ,0,,, tti tttt φδ φφ ′′ Π=− ′′ ==ΠΠ xxxx xxxx (22) 3.2. Stress-Energy Tensor Einstein-Hilbert action is following ()( ) 4 matter d 16π x SgxRxS =−+ ∫ (23) with Einstein-equation as the Euler-Lagrange equation (24) where is Ricci tensor and R is Ricci scalar. And the stress-energy tensor is given by (25) which in our case becomes () 2 22 11 22 Tggm µνµνµνµν φφφφ =∂∂−∂− (26) Now note that conservation of stress-energy tensor comes from the fact that matter action is invariant under spacetime diffeomorphisms ( ) () () 4 matter 4 ;;; surfaceterm 0d 1 d 2 0 S Sgxx gx gTxgT gTgT gT T αβ αβ αβαβ αββαβα αβαβ αβαβ αβ αβ αβ α δ δδ δ ξξξ ξξ ξ == =−−+=−− =−∇−+−∇ =−∇ ⇒∇= ∫ ∫∫ ∫∫ ∫ (27)  S. Mandal 10.4236/jhepgc.2019.51009 172 Journal of High Energy Physics, G where is a Killing vector field of the spacetime. 3.3. Fock Space Let us consider a complete set of mode solutions of the Klein-Gordon equation, with being a set of labels which distinguish independent solutions. The modes are normalised with respect to the inner product defined in following way ()() 3*0 ,d,, V fgigxftxgtx =−−∂ ∫ (28) such that solutions are orthonormalized in following manner ** ** ,,, ,0, jjjjjjjj jjjj ffff ffff δδ ′′′′ ′′ ==− == (29) And one can also show that this definition of inner product is time-inde- pendent [12]. Assuming that the inner product is well defined, the corresponding comple- teness relation can be written as: ()( )() () () () 3 0*0* ,,,, jjjj j i ftftftft g δ ′′′ ∂−∂=−− − ∑ xxxxxx (30) Now we write field operator in terms of mode decomposition ()()( ) * ˆ ˆˆ jjjj j xafxafx φ =+ ∑ † (31) Second quantisation promotes to an operator, called the field operator, obeying the commutation relation mentioned earlier. Consequently, the coeffi- cients and obey the following algebra: † †† ˆˆ , ˆˆˆˆ , 0, jjjj jjjj aa aaaa δ ′′ ′′ = == (32) And vacuum state is defined such that (33) Applying products of creation operators to the vacuum state creates multiparticle states, which form a basis of the Fock space () † 12 3 1 2 1 ˆ 0 ! i n n nj S i jjja n σ σ ∈ = = ∑ ∏ (34) The identity operator can be written in terms of the basis vectors 1 1212 1 00 n nn njj jjjjjj ∞ = =+ ∑∑∑ (35) In terms of one-particle operators, the stress-energy tensor can be written in following way  S. Mandal 10.4236/jhepgc.2019.51009 173 Journal of High Energy Physics, G () () ()() () () ** , ** 2 ˆ ˆˆˆˆ ,, ˆˆˆˆ ,, 11 , 22 jkjkjkjk jk jkjkjkjk jkjkjkjk Taaffaaff aaffaaff ffffggffgmff µνµνµν µνµν αβ µνµνµναβµν =+ ++ =∇∇−∇∇− ∑ †† †† (36) Looking at the first 2 terms in stress-energy tensor operator one might think it already breaks U(1) symmetry but it actually not. To know that we have to go further. Let us specialise further to mode solutions of the Klein-Gordon equation which satisfy the eigenvalue equations ** ** , , tjjjtjjj tt jjjjjj iffiff iffiff ωω ωω ∂=∂=− −∂=−∂=− (37) An integration of these equations shows that . Hence, can be interpreted as the frequency of the mode j. Under the assumptions, the norma- lisation conditions and the completeness relation take the form: () ()() () ()() ()()()() () () 3* 3 3 ** d,, d,,0 1 ,,,, jjjjjj jjjj jjjjj j gxftft gxftft ftftftft g ωωδ ωω ωδ ′′′ ′′ ′′ +−= −−= ′ +=− − ∫ ∫ ∑ xx xx xxxxxx (38) which requires ( i.e. , instead of the eigenvalue of the Hamiltonian). Thus, the norm and frequency of a mode can have opposite signs if , forcing modes with negative frequency in the set of particle modes which we don’t want. Using the definition of conjugate momentum and Hamiltonian one can derive [13] ††† 11 ˆ ˆ ˆˆˆˆˆ 22 jjjjjjjj jj Haaaaaa ωω =+=+ ∑∑ (39) Above equation shows that particles for which make negative con- tributions to the total Hamiltonian of the system, while particles for which vanishes do not contribute. 3.4. Finite Temperature Field Theory The concept of temperature is implemented by considering a quantum state containing a thermal distribution of particle states, with the Hamiltonian operator playing the role of energy. The expectation value of an operator in a thermal state at a finite inverse temperature is defined as 1 ˆˆ 11 0,, ˆ 11 ˆˆ Tree Tre n HH nn njj H AAjjjj ββ β β ∞ −− = − == = ∑∑ (40) It is now hard to show that [14] [15]  S. Mandal 10.4236/jhepgc.2019.51009 174 Journal of High Energy Physics, G † ††† ˆ ˆ ˆˆ ˆˆˆˆ ,,0 e11e jj jjjj jjjjjjjj aaaaaaaa βωβω β βββ δδ ′′ ′′′′ − ==== −− (41) Notice because of invariance of the Hamiltonian, in thermal expectation values of stress-energy tensor first 2 terms won’t contribute and therefore we will get ()() ** 1 ˆ ::=,, e1 j jjjj j Tffff µνµνµν βω β ′′ + − ∑ (42) but those term will contribute when we are considering . Just because such 2 terms are present in stress-energy operator does not implies U (1) symmetry breaking but if it presents in generator of certain transformation then only we say U (1) symmetry is breaking. Since generator of time-translation is which is same as hamiltonian operator therefore we are sure that U (1) symmetry is not broken therefore charge is conserved. On the other hand if we find does not contain those 2 terms then charge associated with it does not commute with generators of U(1) action. 4. Canonical Transformation Under following canonical canonical transformation † †† ˆˆˆ coshsinh ˆˆˆ coshsinh jjjjj jjjjj acc acc θθ θθ =+ =+ (43) Then in similar way as in [1] where we use finite temperature field theory concepts from [16] [17] [18] [19] we can write partition function under above transformation in following path integral manner () () () () () () ()() ()() () , 22 0 e 1 ,dcoshsinh sinhcosh E S Ejjjjj j jjjjjjj S φφ β τ φφ φφτφτθθξφτ ξθθφτφτ φτφτ = =∂−+ −+ ∫ ∑ ∫ (44) which can be written in matrix representation in following way (denoting , , where is the che- mical potential) ( ) ()( ) () ( ) () () () ()() () () () () () 0 3 1 ,d 1 2 1 2 d 1 2 1 2 Ejj j jjjj j j jjjj jnjn n njjjj jn jn jjnjj S k i i β τ τ φφτφτ φτ ξχξη φτ φτ ξηξχ βφωφω ωξχξη φω φω ξηωξχ = ∂− ×× ∂− =− − ×× − −− ∑ ∫ ∑ ∫ (45) 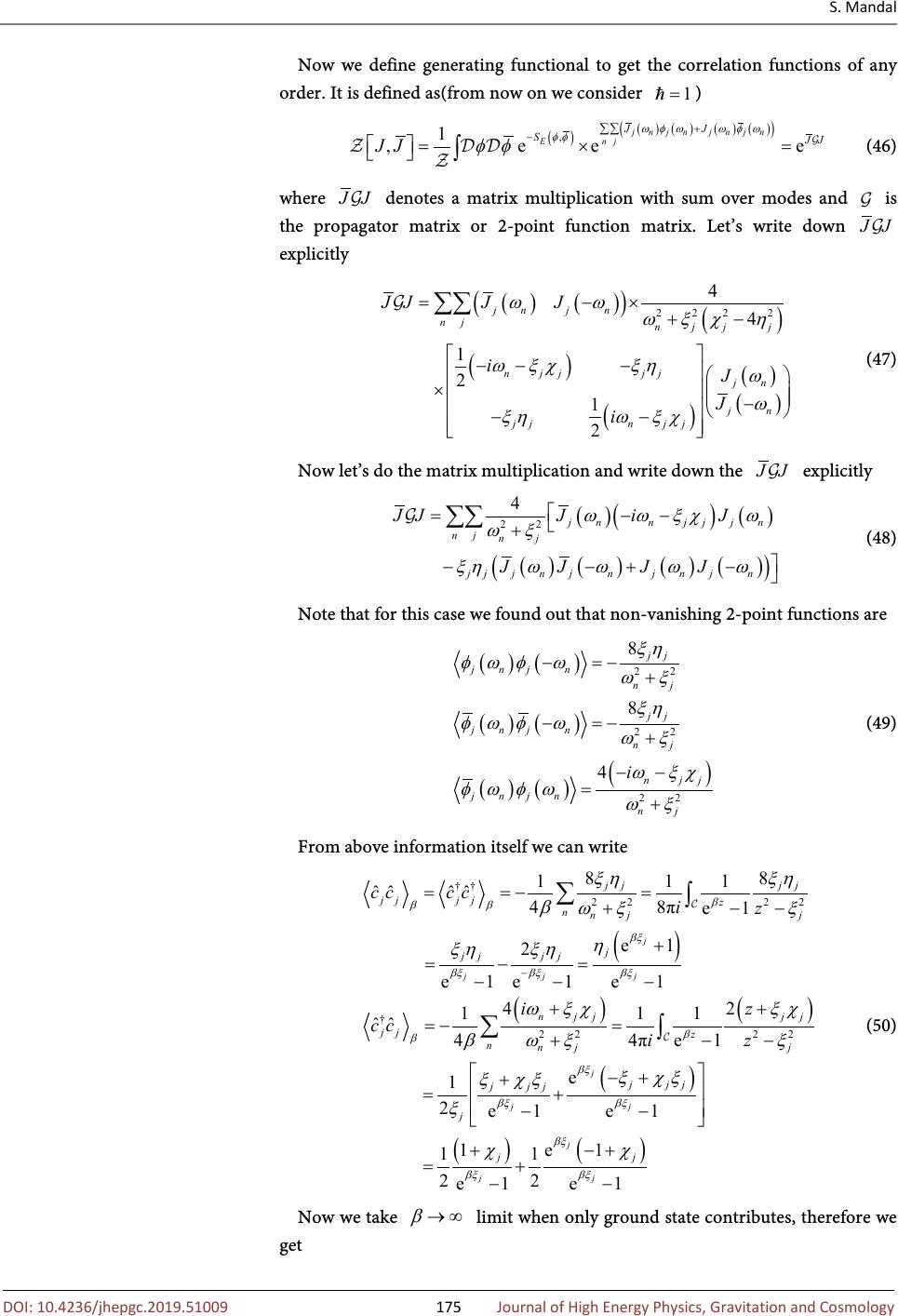 S. Mandal 10.4236/jhepgc.2019.51009 175 Journal of High Energy Physics, G Now we define generating functional to get the correlation functions of any order. It is defined as(from now on we consider ) () ()()()() () , 1 ,eee jnjnjnjn E nj JJ S JJ JJ ωφωωφω φφ φφ + − ∑∑ =×= ∫ (46) where denotes a matrix multiplication with sum over modes and is the propagator matrix or 2-point function matrix. Let’s write down explicitly ()() () () () () () () 2222 4 4 1 2 1 2 jnjn nj njjj njjjj jn jn jjnjj JJJJ i J J i ωω ωξχη ωξχξη ω ω ξηωξχ =−× +− −−− × − −− ∑∑ (47) Now let’s do the matrix multiplication and write down the explicitly () () () () ()()() () 22 4 jnnjjjn nj nj jjjnjnjnjn JJJiJ JJJJ ωωξχω ωξ ξηωωωω =−− + −−+− ∑∑ (48) Note that for this case we found out that non-vanishing 2-point functions are () () ( ) () ( )() () 22 22 22 8 8 4 jj jnjn nj jj jnjn nj njj jnjn nj i ξη φωφω ωξ ξη φωφω ωξ ωξχ φωφω ωξ −=− + −=− + −− = + (49) From above information itself we can write () () () () †† 2222 † 2222 88 111 ˆˆˆˆ 48π e1 e1 2 e1e1e1 42 111 ˆˆ 44π e1 e 1 2 e1e1 j jjj j jj jjjj jjjj z n njj j jjjj njjjj jj z n njj jjj jjj j cccc i z iz cc i z β β β βξ βξβξβξ β β βξ βξβξ ξηξη β ωξξ η ξηξη ωξχξχ β ωξξ ξχξ ξχξ ξ − ==−= +−− + =−= −−− ++ =−= +−− −+ + =+ −− ∑ ∫ ∑ ∫ () () 1e1 11 22 e1e1 j jj jj βξ βξβξ χχ +−+ =+ −− (50) Now we take limit when only ground state contributes, therefore we get  S. Mandal 10.4236/jhepgc.2019.51009 176 Journal of High Energy Physics, G ( ) †† † 2 †2 1 ˆˆ ˆ ˆ sinh2 2 1 ˆˆ 1 sinh 2 ˆ ˆ sinh1 jjjjjj jjjj jjj cccc cc cc ηθ χθ θ === =−= ⇒=+ (51) Note that above results are vacuum expectation values because at limit only ground state contributes inside trace operation in partition function. Above result clearly shows that ground state of the hamiltonian under Bogo- liubov transformation is no longer a state which is annihilated by annihilation operators and also ground state does not maintain U(1) invariance property which we also mentioned in [1]. Now recall that before doing Bogoliubov transformation (52) whereas after doing the transformation we will have following stress-energy tensor operator () () ()() () () ** , ** , ** ˆ ˆˆˆˆ ::,, ˆˆˆˆ ,, ˆˆˆˆ ,coshcoshsinhsinh ˆˆˆˆ coshsinhsinhcosh ,coshcos jkjkjkjk jk jkjkkjjk jkjkjkjkjk jk jkjkjkjk jkj Taaffaaff aaffaaff ffcccc cccc ff µν µνµν µνµν µν µν θθθθ θθθθ θ =+ ++ =+ ++ + ∑ ∑ †† †† †† †† ˆˆˆˆ hsinhsinh kjkjkjk cccc θθθ + †† () () † * * ˆˆˆ ˆ coshsinhsinhcosh ˆˆˆˆ ,coshcoshsinhsinh ˆˆˆˆ coshsinhsinhcosh ˆˆˆˆ ,coshcoshsinhsinh ˆ coshsinh jkjkjkjk jkjkjkjkjk jkjkjkjk jkjkjkjkjk jk cccc ffcccc cccc ffcccc µν µν θθθθ θθθθ θθθθ θθθθ θθ ++ ++ ++ ++ + † † † †† †† ˆˆˆ sinhcosh jkjkjk cccc θθ + †† (53) Now we are going to calculate which w.r.t new vacuum state becomes () () ** ˆ ˆˆˆˆ ::,coshcoshsinhsinh ˆˆˆˆ coshsinhsinhcosh ˆˆˆˆ ,coshcoshsinhsinh ˆˆˆˆ coshsinhsinhcosh jjjjjjjjjj j jjjjjjjj jjjjjjjjjj jjjjjjjj Tffcccc cccc ffcccc cccc µνµν µν θθθθ θθθθ θθθθ θθθθ =+ ++ ++ ++ ∑ †† †† †† †† () () * * ˆˆˆˆ ,coshcoshsinhsinh ˆˆˆ ˆ coshsinhsinhcosh ˆˆ ˆˆ ,coshcoshsinhsinh ˆ ˆˆˆ coshsinhsinhcosh jjjjjjjjjj jjjjjjjj jjjjjjjjjj jjjjjjjj ffcccc cccc ffcccc cccc µν µν θθθθ θθθθ θθθθ θθθθ ++ ++ ++ ++ †† †† †† †† (54)  S. Mandal 10.4236/jhepgc.2019.51009 177 Journal of High Energy Physics, G Note that exact answer may depend on the values of () ()()() **** ,,,,,,, jjjjjjjj ffffffff µνµνµνµν but † †† † ˆ ˆ ˆˆ ˆˆ ˆ ˆ ,,, jjjjjjjj cccccccc are all positive definite. And also note that () () ** ˆ ˆˆˆˆ ::,coshcoshsinhsinh ˆˆˆˆ coshsinhsinhcosh ˆˆˆˆ ,coshcoshsinhsinh ˆˆˆˆ coshsinhsinhcosh jjjjjjjjjj j jjjjjjjj jjjjjjjjjj jjjjjjjj Tffcccc cccc ffcccc cccc µµ µµ µ µ θθθθ θθθθ θθθθ θθθθ =+ ++ ++ ++ ∑ †† †† †† †† () () * * ˆˆˆˆ ,coshcoshsinhsinh ˆˆˆˆ coshsinhsinhcosh ˆˆˆˆ ,coshcoshsinhsinh ˆˆˆˆ coshsinhsinhcosh jjjjjjjjjj jjjjjjjj jjjjjjjjjj jjjjjjjj ffcccc cccc ffcccc cccc µ µ µ µ θθθθ θθθθ θθθθ θθθθ ++ ++ ++ ++ †† †† †† †† (55) And note that () ()() () 22 2 **2 **2*2 , ,, , jjj jjjjj jjj ffmf ffffmf ffmf µ µ µµ µµ µ µ =− ==− =− (56) therefore, we can definitely say that which means all terms of are not zero, which shows that generators associated with spacetime symmetries which I defined earlier are broken which is what observer finds after doing Bogoliubov transformation to know about characteristics of matter in different frame. On the other-hand if we start with the second frame from the matter action level itself without doing Bogoliubov transformation then as usual we will have Hamiltonian of following form () †† 1 ˆˆˆˆ ˆ 2 lllll l Hbbbb=Ω+ ∑ (57) which is invariant under U(1) action and therefore from partition function we can have following thermal expectation values †† †† ˆˆˆˆ , e11e ˆˆˆˆ 0 jj jjjj jjjj jjjj bbbb bbbb ββ ββ ββ δδ ′′ ′′ Ω−Ω ′′ == −− == (58) Note that in limit when ground state contribution is maximum we will have † † †† ˆˆˆˆ 0, ˆˆˆˆ 0 jjjjjj jjjj bbbb bbbb δ ′′′ ′′ == == (59)  S. Mandal 10.4236/jhepgc.2019.51009 178 Journal of High Energy Physics, G And with the stress-energy tensor being () ( ) ()() ** , ** ˆˆˆˆ ˆ ,, ˆˆˆˆ ,, jkjkjkjk jk jkjkjkjk Tbbggbbgg bbggbbgg µνµνµν µνµν =+ ++ ∑ † † †† (60) where are two independent solutions of Klein-Gordon equation corresponding to matter field equation in target curved spacetime where we did go through Bogoliubov transformation. With the above result it is easy to check now that in this case indeed (61) which shows generators with corresponding spacetime symmetries are not broken which we should expect because classically spacetime has some Killing vector fields which defined the corresponding symmetries of the spacetime. 5. Conclusion In the beginning of this article, I emphasized on the fact that in thermodynamic limit although we have 2 disjoint vector spaces but we still can do the canonical transformation. And we also restrict ourself to new Fock space because after taking infinite volume limit we can’t get back to the old Fock space. In this article, I am able to show a inconsistency between Bogoliubov transformation and breaking of charges associated with classical spacetime symmetries through stress-energy tensor of matter. Although breaking of such charges in quantum field theory is not problematic but we show there are two different descriptions, one is Bogoliubov transformation through which we can find the properties of matter field in coordinate transformed spacetime (target spacetime) and other is action description of matter field imposed on the target spacetime itself from the beginning. And this also raises the question of validity of particle production phenomenon [20]-[29] which is a highly debatable matter. In the paper [30] (and others with similar approaches [31] [32] [33] [34]), the author shows that applying the rigorous algebraic approach to QFT, the derivation of the Unruh effect usually done by almost everyone is incorrect, and that the Unruh effect doesn’t exist. More than that, as far as I know, the Unruh effect hasn’t been yet observed therefore, in the end of the day, the Unruh effect has no any expe- rimental observation that would point towards its correctness. Acknowledgements The author wants to thank Dr. Golam Mortuza Hossain for giving him oppor- tunity to work on this topic independently. The author would also like to thank CSIR to support this work through JRF fellowship. Conflicts of Interest The author declares no conflicts of interest regarding the publication of this pa- per.  S. Mandal 10.4236/jhepgc.2019.51009 179 Journal of High Energy Physics, G References [1] Mandal, S.U. (2018) Symmetry Breaking under Canonical Transformation in Real Scalar Field Theory. [2] Perelomov, A.M. (1986) Generalized Coherent States and Their Applications of Modern Methods of Plant Analysis. Springer-Verlag, Berlin. [3] Umezawa, H., Matsumoto, H. and Tachiki, M. (1982) Thermo Field Dynamics and Condensed States. [4] Sato, H.-T. and Suzuki, H. (1994) On Bogoliubov Transformation of Scalar Wave Functions in de Sitter Space. Modern Physics Letters A , 9, 3673-3684. https://doi.org/10.1142/S0217732394003518 [5] Das, A. and De Benedictis, A. (2012) The General Theory of Relativity: A Mathe- matical Exposition. Springer-Verlag, New York. [6] Steven, W. (1972) Gravitation and Cosmology: Principles and Applications of the General Theory of Relativity. Wiley, Hoboken. [7] Sigbjorn, H.Y.G. (2007) Einstein’s General Theory of Relativity. Springer, Berlin. [8] Goldstone, J., Salam, A. and Weinberg, S. (1962) Broken Symmetries. Physical Re- view , 127, 965-970. https://doi.org/10.1103/PhysRev.127.965 [9] Schakel, A.M.J. (1998) Boulevard of Broken Symmetries. [10] Birrell, N.D. and Davies, P.C.W. (1984) Quantum Fields in Curved Space of Cam- bridge Monographs on Mathematical Physics. CUP Edition, Cambridge University Press, Cambridge. [11] Ford, L.H. (1997) Quantum Field Theory in Curved Space-Time. Particles and Fields. Proceedings , 9 th Jorge Andre Swieca Summer School , Campos do Jordao, 16-28 February 1997, 345-388. [12] Parker, L. and Toms, D. (2009) Quantum Field Theory in Curved Spacetime: Quan- tized Fields and Gravity of Cambridge Monographs on Mathematical Physics. Cambridge University Press, Cambridge. [13] Ambrus, V.E. (2014) Dirac Fermions on Rotating Space-Times. PhD Thesis, Uni- versity of Sheffield. [14] Arovas, D. (2013) Lecture Notes on Thermodynamics and Statistical Mechanics (A Work in Progress). [15] Vilenkin, A. (1980) Quantum Field Theory at Finite Temperature in a Rotating System. Physical Review D , 21, 2260-2269. https://doi.org/10.1103/PhysRevD.21.2260 [16] Das, A. (1997) Finite Temperature Field Theory. [17] Kapusta, J.I. (1989) Finite-Temperature Field Theory of Cambridge Monographs on Mathematical Physics. Cambridge University Press, Cambridge. [18] Laine, M. and Vuorinen, A. (2016) Basics of Thermal Field Theory. Lecture Notes in Physics, Vol. 925, Springer, Berlin. https://doi.org/10.1007/978-3-319-31933-9 [19] Yang, Y. (2011) An Introduction to Thermal Field Theory. Imperial College, Lon- don. [20] Ahmadzadegan, A. (2017) Probing the Unruh and Hawking Effects Using Un- ruh-DeWitt Detectors. PhD Thesis, U. Waterloo (Main). [21] Birrell, N.D. and Davies, P.C.W. (1980) Massive Particle Production in Anisotropic Space-Times. Journal of Physics A : Mathematical and General , 13, 2109. https://doi.org/10.1088/0305-4470/13/6/032  S. Mandal 10.4236/jhepgc.2019.51009 180 Journal of High Energy Physics, G [22] Biswas, S., Guha, J. and Sarkar, N.G. (1995) Particle Production in de Sitter Space. Classical and Quantum Gravity , 12, 1591. https://doi.org/10.1088/0264-9381/12/7/005 [23] Mo Chitre, D. and Hartle, J.B. (1977) Path-Integral Quantization and Cosmological Particle Production: An Example. Physical Review D , 16, 251. https://doi.org/10.1103/PhysRevD.16.251 [24] Crispino, L.C.B., Higuchi, A. and Matsas, G.E.A. (2008) The Unruh Effect and Its Applications. Reviews of Modern Physics , 80, 787-838. https://doi.org/10.1103/RevModPhys.80.787 [25] Davies, P.C.W. (1975) Scalar Production in Schwarzschild and Rindler Metrics. Journal of Physics A : Mathematical and General , 8, 609. https://doi.org/10.1088/0305-4470/8/4/022 [26] Degner, A. and Verch, R. (2010) Cosmological Particle Creation in States of Low Energy. Journal of Mathematical Physics , 51, 022302. https://doi.org/10.1063/1.3271106 [27] Duru, I.H. and Ünal, N. (1986) Particle Production in Expanding Universes with Path Integrals. Physical Review D , 34, 959. https://doi.org/10.1103/PhysRevD.34.959 [28] Frieman, J.A. (1989) Particle Creation in Inhomogeneous Spacetimes. Physical Re- view D , 39, 389-398. https://doi.org/10.1103/PhysRevD.39.389 [29] Winitzki, S. (2005) Cosmological Particle Production and the Precision of the WKB Approximation. Physical Review D , 72, Article ID: 104011. https://doi.org/10.1103/PhysRevD.72.104011 [30] Buchholz, D. and Verch, R. (2015) Macroscopic Aspects of the Unruh Effect. Clas- sical and Quantum Gravity , 32, 245004. https://doi.org/10.1088/0264-9381/32/24/245004 [31] Blasone, M., Lambiase, G. and Luciano, G.G. (2017) Nonthermal Signature of the Unruh Effect in Field Mixing. Physical Review , D96, 025023. https://doi.org/10.1103/PhysRevD.96.025023 [32] Ford, G.W. and O’Connell, R.F. (2006) Is There Unruh Radiation? Physics Letters , A350, 17-26. https://doi.org/10.1016/j.physleta.2005.09.068 [33] Nikolic, H. (2001) Inappropriateness of the Rindler Quantization. Modern Physics Letters A , 16, 579-581. https://doi.org/10.1142/S0217732301003681 [34] Rosu, H.C. (2001) Hawking Like Effects and Unruh Like Effects: Toward Experi- ments? Gravitation and Cosmology , 7, 1-17.
|