Open Journal of Physical Chemistry, 2011, 1, 131-140
doi:10.4236/ojpc.2011.13018 Published Online November 2011 (http://www.SciRP.org/journal/ojpc)
131
A Theoretical Study of Binary and Ternary
Hydride-Bonded Complexes N(Beh2)···X with N = 1 or 2
and X = K+ or Ca+2
Boaz Galdino de Oliveira1*, Regiane de Cássia Maritan Ugulino de Araújo2
1Instituto de Ciências Ambientais e Desenvolvimento Sustentável, Universidade Federal da Bahia, Barreiras, Brazil
2Departamento de Química, Universidade Federal da Paraíba, João Pessoa, Brazil
Received June 5, 2011; revised August 12, 2011; accepted September 15, 2011
Abstract
A theoretical study of hydride bonds formed between beryllium hydride and alkaline earth metal cations is
presented. B3LYP/6-311++G(d,p) calculations were used for determining the optimized geometries of the
BeH2···K+, BeH2···Ca+2, BeH2···K+···BeH2, and BeH2···Ca+2···BeH2 hydride-bonded complexes, where among
them the first are binaries, whereas the last ones are ternaries with the calcium (Ca+2) and potassium (K+)
ions mediating the interactions with the beryllium hydride (BeH2). A detailed structural analysis were per-
formed, by which the yielded profiles are in good agreement with results of the infrared vibrational spectrum,
mainly in regards to the existence of red-shifted modes followed by enlarged absorption intensity ratios of
the B-H bonds of the binary complexes. The capability of either donating or accepting of protons among
BeH2, K+, and Ca+2, is currently treated in conformity with Lewis’s acid/base theory, but is also interpreted
through the application of the Quantum Theory of Atoms in Molecules (QTAIM), whose formalism consents
in the molecular modeling of concentrations and depletions of charge density ruled by the Laplacian shapes,
charge transference fluxes, as well as by the local virial theorem of the electronic density with quantification
of the kinetic and potential energies along the bonds and interactions.
Keywords: Hydride Bonds, Vibrational Infrared Spectrum, B3LYP, QTAIM
1. Introduction
In nature, a lot of systems are formed due to a wide
chemical diversity, as intrinsically present in biological
organisms, chemical processes, physical phenomena, and
ray diffractions in spectroscopy analysis, where all these
systems and events produced by them are governed by
any kind of molecular contact [1]. In fact, the stable con-
tact between a site highly electronegative (lone-electron
pairs or unsaturated bonds) and proton donors (Lewis
acids) is a typical scheme of hydrogen bonds, which is
considered the cornerstone for the intermolecular inter-
actions [2], although there are other types very interest-
ing in their own ways. Agostic interactions [3,4], halogen
bonds [5-7], dihydrogen bonds [8,9] or even π stacking
[10,11] are one of the unconventional interactions known
beyond the hydrogen bond profile. In opposition to the
hydrogen bonds [12], there is an additional interaction
so-called hydride bond deriving from the contact be-
tween two positive centers, and it can be identified as
Y+δ-H–δ···X+δ [13]. The apparent negative character of
H–δ is manifested due to the electropositivity of Y+δ and
X+δ, and thereby, H–δ behaves as intermolecular mediator.
Notwithstanding the positive character of X+δ be partial,
it can be a cationic species what leads to a most efficient
or stronger interaction with H–δ. Thus, the great insight is
dedicated to the negativity of H–δ, whose origin arises
due to its bond with the highest electropositive atom Y+δ.
Recently, Yáñez et al. [14] have reported a theoretical
study with analysis of electronic and spectroscopic pa-
rameters of intermolecular complexes formed at light of
“Beryllium Bonds”, whose main features are the non-
linear configurations and interactions with lone-electron
pairs of the Lewis base. Before of this, however,
Grabowski [15] has already shown the ability of the be-
ryllium hydride (BeH2) as proton-accepting molecule
upon the formation of linear dihydrogen bonds, and fur-
ther, Grabowski et al. [13] have also documented evi-

B. G. de OLIVEIRA ET AL.
132
dences that BeH2 also yields stable hydride bonds with
cations derived from alkaline metals, such as Li+, Na+
and Mg+2. In an emphatic conclusion, hydrides bonds are
not covalent interactions, and besides, no significant
similarity in comparison with classical hydrogen bonds is
known [16]. Based in this context, we present a theo-
retical study of hydride complexes formed by BeH2 and
cations derived from the alkaline earth elements, such as
potassium (K+) and calcium (Ca+2), and thereby the
BeH2···K+ and BeH2···Ca+2 binary hydride complexes, as
well as the BeH2···K+···BeH2 and BeH2···Ca+2···BeH2 ter-
nary ones are the systems to be investigated here. In a
direct qualitative comparison with other systems [17,18],
we expect that our study becomes a guide of forward
arguments in order to discuss more carefully the forma-
tion of the hydride bonds.
2. Criteria for Choice of the Theoretical
Level
In general, one of the main objectives of the theoretical
studies of intermolecular systems is devoted to a maxi-
mum reproducibility regarding the available experimen-
tal data [19,20], where bond lengths and the infrared
stretch frequencies are often the most examined parame-
ters [21]. However, the great clog is the accounting of
the London’s dispersion forces, but in association with
the Hartree-Fock architecture, a lot of schematic works
were elaborated with the purpose to quantify the disper-
sion in order to obtain accurate structures of intermo-
lecular complexes [22,23]. On the other hand, it is
widely established the efficiency of some computational
approaches in studies of bound complexes, either MP2 or
any other Post-Hartree-Fock method [24], or even by
applying the hybrid functionals derived from the Density
Functional Theory (DFT) [25]. In this scenery, the
B3LYP functional is one of the most popular codes with
large applications in several studies of hydrogen
bonded-complexes [26-31], and due to this, we elect it to
be used in this current work.
Moreover, it is not only through the classical quantum
calculations that the molecular parameters of the
n(BeH2)···X (with n = 1 or 2 and X = K+ or Ca+2) hydride
complexes will be determined. In fact, the Quantum
Theory of Atoms in Molecules (QTAIM) [32-34] dis-
poses of a great capability for investigating the nature of
the hydride bonds Be+δ-H–δ···K+δ and Be+δ-H–δ···Ca+δ.
This our affirmation is based on the QTAIM faculty to
locate Bond Critical Points (BCP) along the Bond Path
(BP) between each nuclear pairing interactions, wherein
the computation of the electronic density amounts is de-
veloped, and thus, it can be discussed the hydride bond
strength. Nevertheless, it is also granted to QTAIM the
co-valent assignments of the hydride bonds, as well as
the remaining ones, Be-H. This reasoning is developed at
light of QTAIM topological parameters, such as the
Laplacian 2ρ(r) of the electronic density ρ(r) [35,36],
and other components of the local density: local kinetic
energy density G(r) and local potential energy density
U(r) [37,38]. In QTAIM, the Laplacian content provides
a link between the form of the electronic density and the
quantum mechanical formalism, whose result is the in-
terpretation of 2ρ(r) in conformity with the local equa-
tion of the virial theorem:
 
2
22
4rr
GU
m

 

r
r
(1)
or
  rr
H
GU

 (2)
One direct comparison between these equations was
suggested by Cremer and Kraka [39,40], where G(r) and
U(r) represent the kinetic and potential energy densities,
respectively. Once U(r) is always negative whereas G(r)
in turn is positive, H(r) and 2ρ(r) determine which of
these terms (kinetic or potential) are dominant on the
virial theorem, i.e., 2ρ(r) < 0 denotes that ρ(r) is locally
concentrated with great contribution of U(r), as observed
in covalent bonds or even π sites [41]. On the other hand,
2ρ(r) > 0 is obtained when electronic density vacuities
are modeled, and in these cases, the intermolecular in-
teractions can be efficiently analyzed. In QTAIM, there
is a slight and fundamental difference in the conception
of U(r) with regard to U(Ω). Well, U(r) is the pure poten-
tial energy of the electronic density at any r point of the
molecular surface, whose statement is also valid to G(r).
However, when the virial theorem for all forces act on
the surfaces of an atom in a molecule, it is yielded U(Ω).
This is a suitable spatial condition for molecular model-
ing not only to electronic density, but also applied to the
atomic charges computation:
()
i
ii r
qZ dr 



(3)
As can be seen, qi is an atomic charge partition with
no dependence of the theoretical level, but closely related
to the electronic density [42]. In studies of intermolecular
systems, surely the quantification of the atomic charges
and thereby the measurement of the charge transfer is
very useful, and in practice, it can be used to justify a lot
of molecular parameters, such as polarizability or even
vibrational chemical shifts. In these insights presented
hitherto about the QTAIM, our goal in this work is con-
centrated in the molecular topology of the n(BeH2) ···X
hydride complexes, but also motivated by the covalent

133 B. G. de OLIVEIRA ET AL.
interpretation of the Be-H bonds and mainly, but spe-
cially difficult, in the hydride bonds Be+δ-H–δ···X+δ.
3. Computacional Details
The optimized geometries of the n(BeH2)···X (with n = 1
or 2 and X = K+ or Ca+2) hydride complexes were deter-
mined by the GAUSSIAN 98 W [43] quantum packages
in which all calculations were carried out at the
B3LYP/6-311++G(d,p) level of theory. The QTAIM
calculations were also performed by the GAUSSIAN 98
W [44], although some additional computations were
developed by the AIMAll 11.05.16 suite of codes [45],
more properly by its implementations named as
AIMStudio and AIMQB subparts. After completing all
calculations, the values of the hydride bond energies (ΔE)
were obtained in conformity with the supermolecule ap-
proach [46], by which is stated that ΔE = Ecomplex – Eiso-
lated molecules. Furthermore, these ΔE values were refined
with mandatory corrections, where the Boys and Ber-
nardi’s counterpoise based on the Basis Sets Superposi-
tion Error (BSSE) [47], as well as the contribution of the
Zero Point Vibrational Energies (ZPE) [48] also were
included. In the end, the corrected hydride bond energy
(ΔEC) is obtained as follows: ΔEC = ΔE + BSSE + ΔZPE.
4. Results and Discussion
4.1. Structural Parameters
The optimized geometries of the hydride-bonded com-
plexes, BeH2···K+ (I) and BeH2···Ca+2 (II) binaries, as
well as the BeH2···K+···BeH2 (III) and BeH···Ca+2···BeH2
(IV) ternaries, all of them are illustrated in Figure 1. As
cationic acceptor, the structure of the BeH2 molecule is
modified after the complexation. Note that, by taking
into account Be+δ-H–δ bond length value of 1.3267 Å, an
enhancement tendency is verified, whose values are
1.3485, 1.3656, 1.3464 and 1.3560 Å to (I), (II), (III)
and (IV), respectively. Well, these enhanced values are
those directly affected by the hydride bonds Be+δ-
H–δ···K+ and Be+δ-H–δ···Ca+2, whereas the H-Be opposite
bonds are slightly reduced.
It can be seen that elongation of the H–Be bonds is the
more intense complexation effect, and at this point, the
distance of the hydride bonds H–δ···X also should be in-
cluded in this statement. The decrease and increase of the
H–δ···X lengths reflect on the BeH2 structure. Are then
contrary effects, because whereas the shortest distances
of Be+δ-H–δ···X promotes the increasing of Be+δ-H–δ, and
H-Be+δ in turn reduces. Due to this, Grabowski et al. [13]
have declared that Be+δ-H–δ acts as Lewis base after the
complexation with cationic species. On the other view-
Figure 1. Optimized geometries of the hydride-bonded
complexes, BeH2···K+ (I) and BeH2···Ca+2 (II) binaries, as
well as the BeH2···K+···BeH2 (III) and BeH2···Ca+2···BeH2 (IV)
ternaries. All these geometries were obtained at the B3LYP/
6-311++G(d,p) level of theory.
point, H-Be+δ is enlarged at long bonding distances. In
other words, at prior the bonding distances determine the
interaction strength as follows: (II) > (IV) > (I) > (III).
Therefore, it can be concluded initially that calcium
produces the strongly bound hydride complexes.
In an additional brief comment about the hydride dis-
tances, we would like to use a fundamental criterion
widely used in chemical bonds and interactions studies:
the van der Waals [49] covalent radii, as well as the ionic
radii estimated by Goldschmidt [50] and Pauling [51].
By definition, covalent radius is the dimensional measure
of an uncharged atom that composes a covalent bond,
whereas ionic radius is the measure of atom size as ion
within a crystal structure. However, the values of the
hydride distances in this current work computed at the
B3LYP/6-311++G(d,p) level are not exempt of criticism.
In structural viewpoint, only the complexes formed by
potassium can be considered bonded because the dis-
tance values of 2.5478 Å (I) and both 2.5796 Å (III) are
shortest than the sums of the covalent (H = 1.2000 Å)
and ionic (K+ = 1.3800 Å) tabulated radii. Complexes (II)
and (IV), formed by calcium, are not geometrically
bonded because their hydride distances of 2.3156 Å and
both 2.3284 Å are longest than 2.2000 Å (H = 1.2000 Å
and Ca+2 = 1.000 Å). At prior, this can reveal interesting
features on the electronic structure of the hydride com-
plexes, precisely, meaning an accumulation of charge
density within the intersystem region. However, this is a
question to be answered hereafter.
4.2. Infrared Stretching Frequencies and
Absorption Intensities
The interpretation of the vibrational modes of intermo-

B. G. de OLIVEIRA ET AL.
134
lecular systems is seen as routine procedure. This is a
secure way to affirm that a minimum of potential energy
surface was found due to the absence of imaginary fre-
quencies [52-54]. About this, our systems studied in this
work were characterized with no imaginary frequency.
The values of the harmonic stretching frequencies (υ)
and absorption intensities (A) of the hydride-bonded
complexes (I), (II), (III), and (IV) are organized in Ta-
ble 1. In the binary complexes (I) and (II), their
red-shifts of –9 cm–1 and –59 cm–1 accompanied by the
absorption intensities of 197.9 km·mol–1 and 632.9
km·mol-1 related to the Be+δ-H–δ oscillator are the most
important spectroscopic events [55].
Note that, the non-polarity of BeH2 makes it inactive
in the infrared region, but the absorption intensities
above presented emerge due to the formation of the hy-
dride complexes. Contrary to this, the inactivity of the
Be+δ-H–δ is not altered by the formation of the (II I ) and
(IV) systems, although the variation of –5.1 cm–1 and
–17.2 cm–1 in the stretch frequencies indicate the forma-
tion of the red-shifting hydride bonds, as already docu-
mented by Yáñez et al. [14]. Furthermore, the stretch
frequencies of the Be+δ-H–δ displaced to downward val-
ues corroborates with the reduction of their bond length,
as discussed in structural analysis. At this step of exami-
nation, it can be assumed that structural alterations on
BeH2 cause vibrational displacements on its stretch fre-
quencies, and as far as the structure is deformed, in spec-
troscopy analysis is more detectable, i.e., the red-shift
effects are quite clear and distinguishable.
About the new vibrational modes, the stretch frequen-
cies of 178.4 cm–1 and 216.4 cm–1 of (I) and (II) are ac-
tive in infrared spectrum due to their absorption intensi-
ties of 7.2 km·mol–1 and 13.6 km·mol–1. In comparison
with (III) and (IV), the affirmation that inactive absorp-
tions are caused by the intermolecular (hydride bonds)
distances is not cautious, i.e., this event seems not to be
caused by the interaction strength. Meaningfully, the
Table 1. Values of the red-shift effects, absorption intensity
ratios, new vibrational frequencies and absorption intensi-
ties at the B3LYP/6-311++G(d,p) level of theory.
Hydride-bonded complexes
Parameters
(I) (II) (III) (IV)


Be H




 –9 –59.6 –5.1 –17.2




,
,
A
Be H C
A
Be H m






197.9 632.9 0.0 0.0

Be HH




 
  178.4 216.4 149.0 179.9


ABe HH

  
  7.2 13.6 0.0 0.0
*Values of

and A are given in cm–1 and km·mol–1, respectively.
relative weakest new vibrational modes were observed in
(III) and (IV), what also lead us to consider the vibra-
tional activity of these systems in our discussion. So, as
is well-known that the absorption intensity ratios is the
mainstream to characterize the formation of bound sys-
tems, here our criteria are the detection of the red-shift
stretch effects on the BeH2 molecule when (III) and (IV)
are formed.
4.3. Bonding Energies and Polarizability
The stability and bond strength of an intermolecular sys-
tem is well analyzed at light of the bond energies com-
puted through the B3LYP/6-311++G(d,p) approach. In
Table 2 are gathered all ΔEC values calculated for the (I),
(II), (III) and (IV) systems, and besides, the BSSE and
ZPE corrections for these energies are also listed in this
same computational level. Firstly, one interesting point
are the negative values of the hydride bond energies,
which are in range between the –29.11 kJ·mol–1 (III) and
–47.06 kJ·mol–1 (II), what indicate high stability related
to the binary complexes. However, the ternary com-
plexes are not weakly bound, for instance (IV) is stabi-
lized by means of a high bonding energy of –42.66
kJ·mol–1. It should be mentioned that these bonding en-
ergies were corrected by the BSSE and ZPE contribu-
tions, and in this context, it is noteworthy to comment
that small BSSE values contributed to the correction of
ΔE [56,57]. In a careful analysis, as is well-known that
density functional calculations yields much smaller
BSSE amounts, it become required the application of
complete basis sets.
Moreover, it can be seen that negative BSSE values
were computed, what is not usual. This must occur be-
cause the energy of the cations (K+ and Ca+2) are much
more negatives, whereas the beryllium hydride is less
negative in the presence of the wave function of the
cations above cited. Moreover, the long hydride dis-
tances should affect the counterpoise calculation on the
overlap wave functions, what can lead to uncommon
results similar in nature to those found in this current
work. In Table 2 are also listed the values of dipole
moment enhancements (Δμ). With similar procedures to
those performed for the hydride bonding energies, the Δμ
values are determined by subtracting the complex dipole
(μcomplex) minus its monomers (μisolated molecules) [58,59].
Nevertheless, the specialized literature informs “...how
much stronger bonded the intermolecular system more
enhanced its dipole moment...”, of course motivated by
great structural perturbations on the molecular electronic
distribution. Here, to our knowledge, this conclusion is
not applied because the isolated molecules (BeH2, K+
and Ca+2) are non polar. In other words, the computed Δμ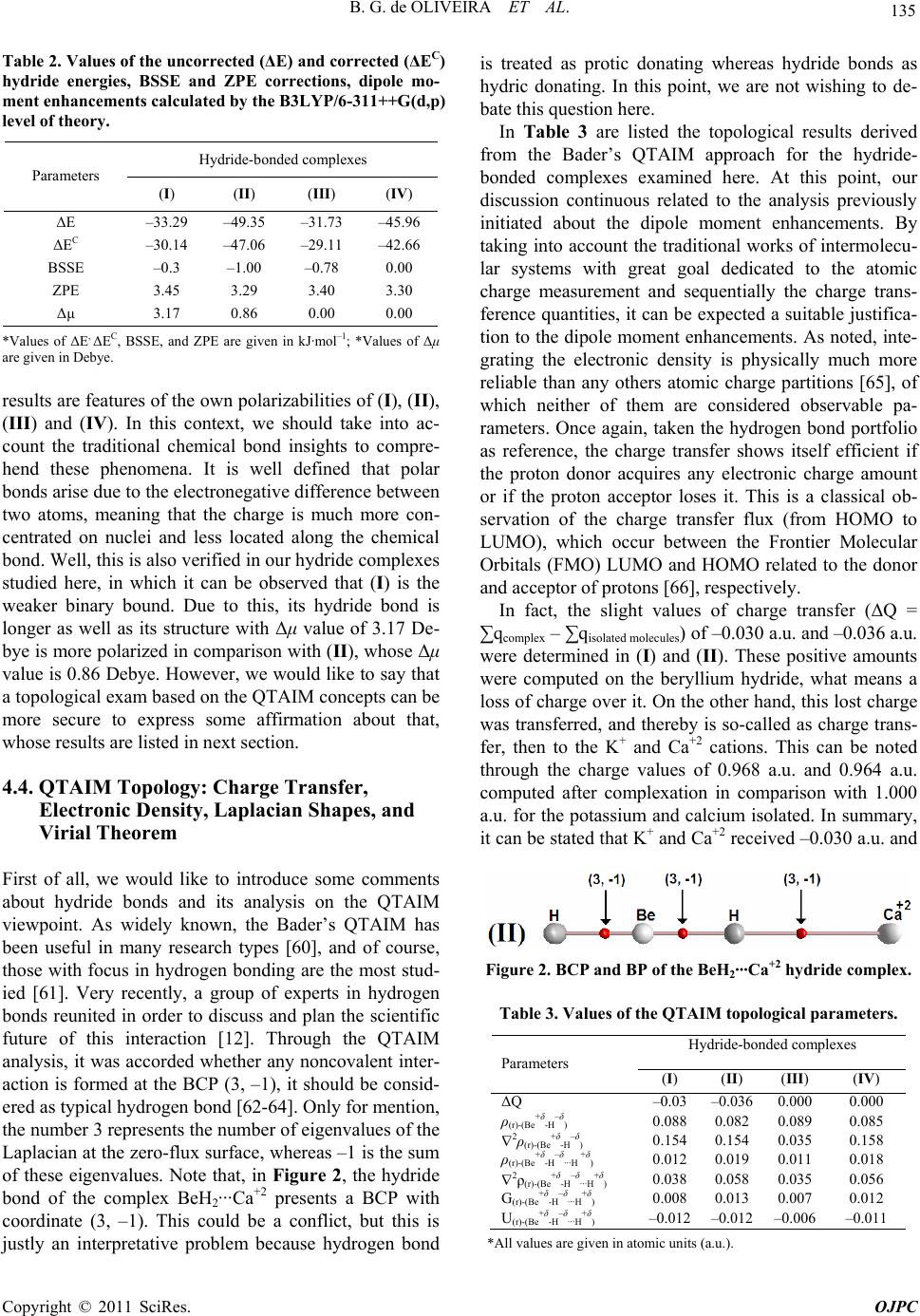

135 B. G. de OLIVEIRA ET AL.
Table 2. Values of the uncorrected (ΔE) and corrected (ΔEC)
hydride energies, BSSE and ZPE corrections, dipole mo-
ment enhancements calculated by the B3LYP/6-311++G(d,p)
level of theory.
Hydride-bonded complexes
Parameters
(I) (II) (III) (IV)
ΔE –33.29 –49.35 –31.73 –45.96
ΔEC –30.14 –47.06 –29.11 –42.66
BSSE –0.3 –1.00 –0.78 0.00
ZPE 3.45 3.29 3.40 3.30
Δμ 3.17 0.86 0.00 0.00
*Values of ΔE, ΔEC, BSSE, and ZPE are given in kJ·mol–1; *Values of Δμ
are given in Debye.
results are features of the own polarizabilities of (I), (II),
(III) and (IV). In this context, we should take into ac-
count the traditional chemical bond insights to compre-
hend these phenomena. It is well defined that polar
bonds arise due to the electronegative difference between
two atoms, meaning that the charge is much more con-
centrated on nuclei and less located along the chemical
bond. Well, this is also verified in our hydride complexes
studied here, in which it can be observed that (I) is the
weaker binary bound. Due to this, its hydride bond is
longer as well as its structure with Δμ value of 3.17 De-
bye is more polarized in comparison with (II), whose Δμ
value is 0.86 Debye. However, we would like to say that
a topological exam based on the QTAIM concepts can be
more secure to express some affirmation about that,
whose results are listed in next section.
4.4. QTAIM Topology: Charge Transfer,
Electronic Density, Laplacian Shapes, and
Virial Theorem
First of all, we would like to introduce some comments
about hydride bonds and its analysis on the QTAIM
viewpoint. As widely known, the Bader’s QTAIM has
been useful in many research types [60], and of course,
those with focus in hydrogen bonding are the most stud-
ied [61]. Very recently, a group of experts in hydrogen
bonds reunited in order to discuss and plan the scientific
future of this interaction [12]. Through the QTAIM
analysis, it was accorded whether any noncovalent inter-
action is formed at the BCP (3, –1), it should be consid-
ered as typical hydrogen bond [62-64]. Only for mention,
the number 3 represents the number of eigenvalues of the
Laplacian at the zero-flux surface, whereas –1 is the sum
of these eigenvalues. Note that, in Figure 2, the hydride
bond of the complex BeH2···Ca+2 presents a BCP with
coordinate (3, –1). This could be a conflict, but this is
justly an interpretative problem because hydrogen bond
is treated as protic donating whereas hydride bonds as
hydric donating. In this point, we are not wishing to de-
bate this question here.
In Table 3 are listed the topological results derived
from the Bader’s QTAIM approach for the hydride-
bonded complexes examined here. At this point, our
discussion continuous related to the analysis previously
initiated about the dipole moment enhancements. By
taking into account the traditional works of intermolecu-
lar systems with great goal dedicated to the atomic
charge measurement and sequentially the charge trans-
ference quantities, it can be expected a suitable justifica-
tion to the dipole moment enhancements. As noted, inte-
grating the electronic density is physically much more
reliable than any others atomic charge partitions [65], of
which neither of them are considered observable pa-
rameters. Once again, taken the hydrogen bond portfolio
as reference, the charge transfer shows itself efficient if
the proton donor acquires any electronic charge amount
or if the proton acceptor loses it. This is a classical ob-
servation of the charge transfer flux (from HOMO to
LUMO), which occur between the Frontier Molecular
Orbitals (FMO) LUMO and HOMO related to the donor
and acceptor of protons [66], respectively.
In fact, the slight values of charge transfer (ΔQ =
∑qcomplex – ∑qisolated molecules) of –0.030 a.u. and –0.036 a.u.
were determined in (I) and (II). These positive amounts
were computed on the beryllium hydride, what means a
loss of charge over it. On the other hand, this lost charge
was transferred, and thereby is so-called as charge trans-
fer, then to the K+ and Ca+2 cations. This can be noted
through the charge values of 0.968 a.u. and 0.964 a.u.
computed after complexation in comparison with 1.000
a.u. for the potassium and calcium isolated. In summary,
it can be stated that K+ and Ca+2 received –0.030 a.u. and
Figure 2. BCP and BP of the BeH2···Ca+2 hydride complex.
Table 3. Values of the QTAIM topological parameters.
Hydride-bonded complexes
Parameters (I) (II) (III) (IV)
ΔQ –0.03–0.036 0.000 0.000
ρ(r)-(Be+δ-H–δ) 0.0880.082 0.089 0.085
2ρ(r)-(Be+δ-H–δ) 0.1540.154 0.035 0.158
ρ(r)-(Be+δ-H–δ···H +δ) 0.0120.019 0.011 0.018
2ρ(r)-(Be+δ-H–δ···H+δ) 0.0380.058 0.035 0.056
G(r)-(Be+δ-H–δ···H+δ) 0.0080.013 0.007 0.012
U(r)-(Be+δ-H–δ···H+δ) –0.012–0.012 –0.006 –0.011
*All values are given in atomic units (a.u.).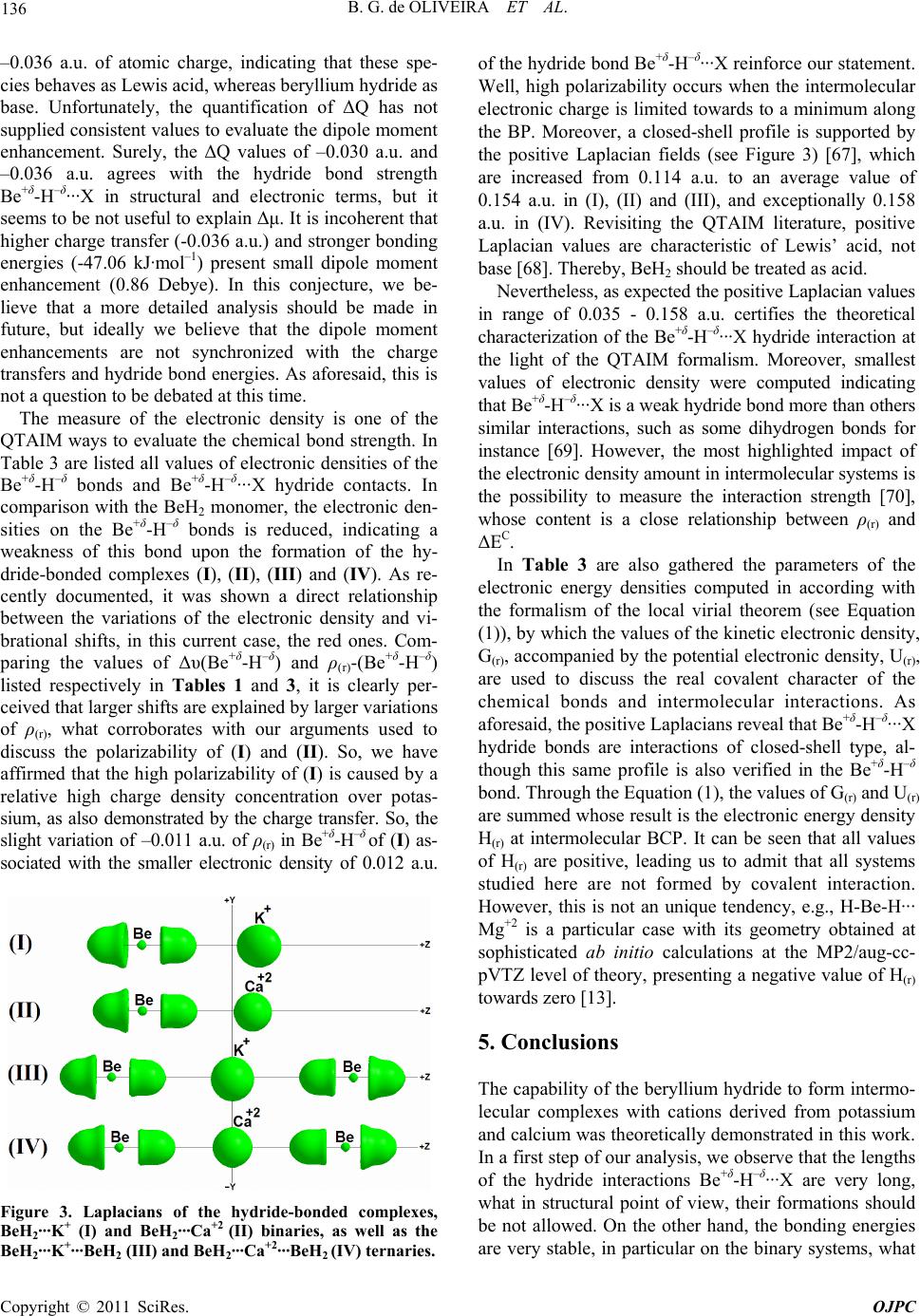

B. G. de OLIVEIRA ET AL.
136
–0.036 a.u. of atomic charge, indicating that these spe-
cies behaves as Lewis acid, whereas beryllium hydride as
base. Unfortunately, the quantification of ΔQ has not
supplied consistent values to evaluate the dipole moment
enhancement. Surely, the ΔQ values of –0.030 a.u. and
–0.036 a.u. agrees with the hydride bond strength
Be+δ-H–δ···X in structural and electronic terms, but it
seems to be not useful to explain Δμ. It is incoherent that
higher charge transfer (-0.036 a.u.) and stronger bonding
energies (-47.06 kJ·mol–1) present small dipole moment
enhancement (0.86 Debye). In this conjecture, we be-
lieve that a more detailed analysis should be made in
future, but ideally we believe that the dipole moment
enhancements are not synchronized with the charge
transfers and hydride bond energies. As aforesaid, this is
not a question to be debated at this time.
The measure of the electronic density is one of the
QTAIM ways to evaluate the chemical bond strength. In
Table 3 are listed all values of electronic densities of the
Be+δ-H–δ bonds and Be+δ-H–δ···X hydride contacts. In
comparison with the BeH2 monomer, the electronic den-
sities on the Be+δ-H–δ bonds is reduced, indicating a
weakness of this bond upon the formation of the hy-
dride-bonded complexes (I), (II), (III) and (IV). As re-
cently documented, it was shown a direct relationship
between the variations of the electronic density and vi-
brational shifts, in this current case, the red ones. Com-
paring the values of Δυ(Be+δ-H–δ) and ρ(r)-(Be+δ-H–δ)
listed respectively in Tables 1 and 3, it is clearly per-
ceived that larger shifts are explained by larger variations
of ρ(r), what corroborates with our arguments used to
discuss the polarizability of (I) and (II). So, we have
affirmed that the high polarizability of (I) is caused by a
relative high charge density concentration over potas-
sium, as also demonstrated by the charge transfer. So, the
slight variation of –0.011 a.u. of ρ(r) in Be+δ-H–δ of (I) as-
sociated with the smaller electronic density of 0.012 a.u.
Figure 3. Laplacians of the hydride-bonded complexes,
BeH2···K+ (I) and BeH2···Ca+2 (II) binaries, as well as the
BeH2···K+···BeH2 (III) and BeH2···Ca+2···BeH2 (IV) ternaries.
of the hydride bond Be+δ-H–δ···X reinforce our statement.
Well, high polarizability occurs when the intermolecular
electronic charge is limited towards to a minimum along
the BP. Moreover, a closed-shell profile is supported by
the positive Laplacian fields (see Figure 3) [67], which
are increased from 0.114 a.u. to an average value of
0.154 a.u. in (I), (II) and (III), and exceptionally 0.158
a.u. in (IV). Revisiting the QTAIM literature, positive
Laplacian values are characteristic of Lewis’ acid, not
base [68]. Thereby, BeH2 should be treated as acid.
Nevertheless, as expected the positive Laplacian values
in range of 0.035 - 0.158 a.u. certifies the theoretical
characterization of the Be+δ-H–δ···X hydride interaction at
the light of the QTAIM formalism. Moreover, smallest
values of electronic density were computed indicating
that Be+δ-H–δ···X is a weak hydride bond more than others
similar interactions, such as some dihydrogen bonds for
instance [69]. However, the most highlighted impact of
the electronic density amount in intermolecular systems is
the possibility to measure the interaction strength [70],
whose content is a close relationship between ρ(r) and
ΔEC.
In Table 3 are also gathered the parameters of the
electronic energy densities computed in according with
the formalism of the local virial theorem (see Equation
(1)), by which the values of the kinetic electronic density,
G(r), accompanied by the potential electronic density, U(r),
are used to discuss the real covalent character of the
chemical bonds and intermolecular interactions. As
aforesaid, the positive Laplacians reveal that Be+δ-H–δ···X
hydride bonds are interactions of closed-shell type, al-
though this same profile is also verified in the Be+δ-H–δ
bond. Through the Equation (1), the values of G(r) and U(r)
are summed whose result is the electronic energy density
H(r) at intermolecular BCP. It can be seen that all values
of H(r) are positive, leading us to admit that all systems
studied here are not formed by covalent interaction.
However, this is not an unique tendency, e.g., H-Be-H···
Mg+2 is a particular case with its geometry obtained at
sophisticated ab initio calculations at the MP2/aug-cc-
pVTZ level of theory, presenting a negative value of H(r)
towards zero [13].
5. Conclusions
The capability of the beryllium hydride to form intermo-
lecular complexes with cations derived from potassium
and calcium was theoretically demonstrated in this work.
In a first step of our analysis, we observe that the lengths
of the hydride interactions Be+δ-H–δ···X are very long,
what in structural point of view, their formations should
be not allowed. On the other hand, the bonding energies
are very stable, in particular on the binary systems, what

137 B. G. de OLIVEIRA ET AL.
justifies their formations and stabilities, although it must
be highlighted that a symmetric charge distribution was
observed on the ternary. Either in binary or in ternary,
the cation Ca+2 yields more stable complexes, although
the dipole moment variation does not justify this state-
ment. Because the ternary complexes are non-polar, their
polarizabilities are nulls, but in binary not. Our expecta-
tion was motivated to obtain stronger bound systems
formed by high polarizability. However, it is not oc-
curred. Independent of this, the computation of the
charge transferences also reinforce our ideas that the
high polarizability of BeH2···K+ is derived from the elec-
tronic charge accumulated on the nuclei (H–δ and K+)
with residues of charge density distributed along the hy-
dride interaction. In regards to the infrared stretch fre-
quencies and absorption intensities, besides the red-shifts
on the Be-H bonds but, the fact by which their oscillators
are inactive in regards to the ternary complexes deserves
great attention once these are the most important criteria
used in the characterization of intermolecular systems.
Due to this spectroscopic event, our analysis was con-
centrated in the interpretation of the new vibrational
modes of the hydride bonds Be+δ-H–δ···X. By taking into
the account the topological QTAIM analysis, it was veri-
fied that only closed-shell interactions compose the
structure of the n(BeH2)···X hydride complexes. Beyond
that, the local virial theorem aided us to conclude that
covalent features were not observed in both Be+δ-H–δ···X
hydride bonds or even in the Be-H ones. In a general
conclusion, the hydride complexes studied here can be
considered closed-shell clusters as a whole.
6. Acknowledgements
CNPq and CAPES Brazilian Funding Agencies.
7. References
[1] B. Kojić-Prodić and K. Molćanov, “The Nature of Hy-
drogen Bond: New Insights into Old Theories,” Acta
Chimica Slovenica, Vol. 55, No. 4, 2008, pp. 692-708.
[2] S. J. Grabowski, “Hydrogen Bonding―New Insights,”
Springer, Amsterdam, 2006.
[3] M. Brookhart, M. L. H. Green and G. Parkin, “Agostic
Interactions in Transition Metal Compounds,” Proceed-
ings of the National Academy of Sciences of the United
States of America, Vol. 104, No. 17, 2007, pp. 6908-6914.
doi:10.1073/pnas.0610747104
[4] L. C. Dorsey and F. P. Gabbaï, “R3C-H→SiFR3 Agostic
Interaction,” Organometallics, Vol. 27, No.13, 2008, pp.
3065-3069. doi:10.1021/om8002619
[5] S. K. Ritter, “Halogen Bonding Begins to Fly,” Chemical
& Engineering News, Vol. 87, No. 38, 2009, pp. 39-42.
doi:10.1021/cen-v087n038.p039
[6] N. J. M. Amezaga, S. C. Pamies, N. M. Peruchena and G.
L. Sosa, “Halogen Bonding: A Study Based on the Elec-
tronic Charge Density,” Journal of Physical Chemistry B,
Vol. 114, No. 17, 2010, pp. 552-562.
doi:10.1021/jp907550k
[7] B. G. Oliveira, R. C. M. U. Araújo, E. S. Leite and M. N.
Ramos, “A Theoretical Analysis of Topography and Mo-
lecular Parameters of the CFCl3···O3 Complex: Linear
and Bifurcate Halogen-Oxygen Bonding Interactions,”
International Journal of Quantum Chemistry, Vol. 111,
No. 1, 2011, pp. 111-116. doi:10.1002/qua.22397
[8] B. G. Oliveira and M. L. A. A. Vasconcellos, “A B3LYP
and QTAIM Study of a New Proton Donor for Dihydro-
gen Bonds: The Case of the C2H5+···nBeH2 Complexes (n
= 1 or 2),” Journal of Structural Chemistry, Vol. 20, No.
5, 2009, pp. 897-902. doi:10.1007/s11224-009-9489-x
[9] B. G. Oliveira and M. L. A. A. Vasconcellos, “The Ethyl
Cation as Proton Donor for Dihydrogen Bonds in the
mC2H5+···nMgH2 (m = 1 or 2 and n = 1 or 2) Complex: A
Theoretical Study,” Inorganic Chemistry Communica-
tions, Vol. 12, No. 11, 2009, pp. 1142-1144.
doi:10.1016/j.inoche.2009.09.010
[10] S. L. Capim, S. R. Santana, B. G. Oliveira, G. B. Rocha
and M. L. A. A. Vasconcellos, “Revisiting the Origin of
the Preferential π-π Stacking Conformation of the
(+)-8-Phenylmenthyl Acrylate,” Journal of the Brazilian
Chemical Society, Vol. 21, No. 9, 2010, pp. 1718-1726.
doi:10.1590/S0103-50532010000900018
[11] S. Zdravković and M. V. Satarić, “Stacking Interaction in
DNA Molecule,” Journal of Computational and Theo-
retical Nanoscience, Vol. 7, No. 10, 2010, pp. 2031-2035.
doi:10.1166/jctn.2010.1580
[12] G. R. Desiraju, “A Bond by Any Other Name,” Ange-
wandte Chemie International Edition, Vol. 50, No. 1,
2010, pp. 2-10.
[13] S. J. Grabowski, W. A. Sokalski and J. Leszczynski,
“Hydride Bonding―Ab initio Studies of BeH2···Li+,
BeH2···Na+ and BeH2···Mg2+ Model Systems,” Chemical
Physics Letters, Vol. 422, No. 4-6, 2006, pp. 334-339.
doi:10.1016/j.cplett.2006.01.120
[14] M. Yáñez, P. Sanz, O. Mó, I. Alkorta and J. Elguero,
“Beryllium Bonds, Do They Exist?” Journal of Chemical
Theory and Computation, Vol. 5, No. 10, 2009, pp.
2763-2771. doi:10.1021/ct900364y
[15] S. J. Grabowski, “BeH2 as a Proton-Accepting Molecule
for Dihydrogen Bonded Systems―ab initio Study,”
Journal of Molecular Structure, Vol. 553, No. 1-3, 2000,
pp. 151-156. doi:10.1016/S0022-2860(00)00576-7
[16] S. J. Grabowski, “What Is the Covalency of Hydrogen
Bonding?” Chemical Reviews, Vol. 111, No. 4, 2011, pp.
2597-2625. doi:10.1021/cr800346f
[17] B. G. Oliveira, R. C. M. U. Araújo and M. N. Ramos,
“Multiple Proton Donors on BeH2···2HCl Trimolecular
Dihydrogen-Bonded Complex: Some Theoretical In-
sights,” Journal of Structural Chemistry, Vol. 19, No. 4,
2008, pp. 665-670. doi:10.1007/s11224-008-9344-5
[18] B. G. Oliveira, R. C. M. U. Araújo, J. J. Silva and M. N.

B. G. de OLIVEIRA ET AL.
138
Ramos, “A Theoretical Study of Three and Four Proton
Donors on Linear HX···BeH2···HX and Bifurcate
BeH2···2HX Trimolecular Dihydrogen-Bonded Com-
plexes with X = CN and NC,” Journal of Structural
Chemistry, Vol. 21, No. 1, 2010, pp. 221-228.
doi:10.1007/s11224-009-9567-0
[19] B. Illien, K. Evain, M. Berthelot and C. Laurence, “An
Experimental and Theoretical Study of the Preferred Hy-
drogen Bonding Site of Methyl Isothiocyanate,” Journal
of Physical Organic Chemistry, Vol. 16, No. 9, 2003, pp.
608-614. doi:10.1002/poc.652
[20] G. Litwinienko, G. A. DiLabio and K. U. Ingold, “A
Theoretical and Experimental Investigation of Some Un-
usual Intermolecular Hydrogen-Bond IR Bands— Ap-
pearances Can Be Deceptive,” Canadian Journal of
Chemistry, Vol. 84, No. 10, 2006, pp. 1371-1379.
doi:10.1139/v06-097
[21] B. G. Oliveira, R. C. M. U. Araújo, A. B. Carvalho and
M. N. Ramos, “A Chemometrical Study of Intermolecu-
lar Properties of Hydrogen-Bonded Complexes Formed
by C2H4O···HX and C2H5N···HX with X = F, CN, NC and
CCH,” Journal of Molecular Modeling, Vol. 15, No. 4,
2009, pp. 421-432. doi:10.1007/s00894-008-0422-9
[22] B. G. Oliveira, R. C. M. U. Araújo, A. B. Carvalho, M. N.
Ramos, M. Z. Hernandes and K. R Cavalcante, “A Theo-
retical Study of the Solvent Effects in Ethylene Oxide:
Hydrofluoric Acid Complex Using Continuum and New
Discrete Models,” Journal of Molecular Structure
(THEOCHEM), Vol. 802, No. 1-3, 2007, pp. 91-97.
doi:10.1016/j.theochem.2006.09.002
[23] M. L. A. A. Vasconcellos, B. G. Oliveira and L. F. C. C.
Leite, “The Acidity of Analogous Ammonium Cations: A
Description of the Solvent Effect through the Attainment
of Hydration Clusters Using the AGOA Methodology,”
Journal of Molecular Structure (THEOCHEM), Vol. 860,
No. 1-3, 2008, pp. 13-17.
doi:10.1016/j.theochem.2008.03.023
[24] A. Y. Li, “Theoretical Investigation of Hydrogen Bonds
between CO and HNF2, H2NF and HNO,” Journal of
Physical Chemistry A, Vol. 110, No. 37, 2006, pp.
10805-10821. doi:10.1021/jp062291p
[25] M. Kamiya, T. Tsuneda and K. Hirao, “A Density Func-
tional Study of van der Waals Interactions,” Journal of
Chemical Physics, Vol. 117, No. 13, 2002, pp. 6010-6014.
doi:10.1063/1.1501132
[26] M. Lozynski, D. Rusinska-Roszak and H. -G. Mack,
“Hydrogen Bonding and Density Functional Calculations:
The B3LYP Approach as the Shortest Way to MP2 Re-
sults,” Journal of Physical Chemistry A, Vol. 102, No. 17,
1998, pp. 2899-2903. doi:10.1021/jp973142x
[27] B. G. Oliveira, R. C. M. U. Araújo, A. B. Carvalho and
M. N. Ramos, “A Theoretical Study about the
Non-Linearity of the Hydrogen Bonding in the
C2H4O-C2H2 and C2H4S-C2H2 Heterocyclic Systems,”
Química Nova, Vol. 30, No. 5, 2007, pp. 1167-1170.
doi:10.1590/S0100-40422007000500022
[28] J. Tirado-Rives and W. L. Jorgensen, “Performance of
B3LYP Density Functional Methods for a Large Set of
Organic Molecules,” Journal of Chemical Theory and
Computation, Vol. 4, No. 2, 2008, pp. 297-306.
doi:10.1021/ct700248k
[29] S. Kolboe and S. Svelle, “Does an Ethene/Benzenium Ion
Complex Exist? A Discrepancy between B3LYP and
MP2 Predictions,” Journal of Physical Chemistry A, Vol.
112, No. 29, 2008, pp. 6399-6400.
doi:10.1021/jp8027879
[30] B. G. Oliveira, R. C. M. U. Araújo, A. B. Carvalho and
M. N. Ramos, “The Molecular Properties of Heterocyclic
and Homocyclic Hydrogen-Bonded Complexes Evalu-
ated by DFT Calculations and AIM Densities,” J. Mol.
Model., Vol. 15, No 2, 2009, pp. 123-131.
doi:10.1007/s00894-008-0380-2
[31] B. G. Oliveira, R. C. M. U. Araújo, A. B. Carvalho and
M. N. Ramos, “Small Heterocyclics as Hydrogen Bond
Acceptors and Donors: the Case of the C2H3XS··· NH3
Complexes (X = H, F and CH3),” Journal of Structural
Chemistry, Vol. 20, No. 4, 2009, pp. 663-670.
doi:10.1007/s11224-009-9458-4
[32] R. F. W. Bader, “Atoms in Molecules. A Quantum The-
ory,” Oxford University Press, Oxford, 1990.
[33] P. L. A. Popelier, “Atoms in Molecules. An Introduc-
tion,” Prentice Hall, London, 2000.
[34] C. F. Matta and R. J. Boyd, “The Quantum Theory of
Atoms in Molecules: From Solid State to DNA and Drug
Design,” Wiley-VCH, Weinham, 2007.
[35] R. F. W. Bader, “Atoms in Molecules,” Acc. Chem. Res.,
Vol. 18, No. 1, 1985, pp. 9-15.
doi:10.1021/ar00109a003
[36] R. F. W. Bader, “A Quantum Theory of Molecular
Structure and Its Applications,” Chemical Reviews, Vol.
91, No. 5, 1991, pp. 893-928. doi:10.1021/cr00005a013
[37] R. F. W. Bader, P. J. MacDougall and C. D. H. Lau,
“Bonded and Nonbonded Charge Concentrations and
their Relation to Molecular Geometry and Reactivity,”
Journal of the American Chemical Society, Vol. 106, No.
6, 1984, pp. 1594-1605. doi:10.1021/ja00318a009
[38] R. F. W. Bader and P. M. Beddall, “Virial Field Rela-
tionship for Molecular Charge Distributions and the Spa-
tial Partitioning of Molecular Properties,” Journal of
Chemical Physics, Vol. 56, No. 7, 1972, pp. 3320-3330.
doi:10.1063/1.1677699
[39] D. Cremer and E. Kraka, “Theoretical Determination of
Molecular Structure and Conformation. 15. Three-
Membered Rings: Bent Bonds, Ring Strain, and Surface
Deocalization,” Journal of the American Chemical Soci-
ety, Vol. 107, No. 13, 1985, pp. 380-3810.
doi:10.1021/ja00299a009
[40] D. Cremer and E. Kraka, “Theoretical Determination of
Molecular Structure and Conformation. 16. Substituted
Cyclopropanes―An Electron Density Model of Sub-
stituent―Ring Interactions,” Journal of the American
Chemical Society, Vol. 107, No. 13, 1985, pp. 3811-3819.
doi:10.1021/ja00299a010
[41] F. Biegler-König, R. F. W. Bader and T. H. Tang, “Cal-

139 B. G. de OLIVEIRA ET AL.
culation of the Average Properties of Atoms in Molecules.
II,” Journal of Computational Chemistry, Vol. 3, No.,
1982, pp. 317-328.
[42] B. G. Oliveira, R. C. M. U. Araújo, A. B. Carvalho, E. F.
Lima, W. L. V. Silva, M. N. Ramos and A. M. Tavares,
“The Hydrogen Bond in the Acetylene-2(HF) Complex:
A Theoretical Study about Intramolecular and Unusual
π···H Interactions Using DFT and AIM Calculations,”
Journal of Molecular Structure (THEOCHEM), Vol. 775,
No. 1-3, 2006, pp. 39-45.
doi:10.1016/j.theochem.2006.06.028
[43] M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuse-
ria, M. A. Robb, J. R. Cheeseman, V. G. Zakrzewski, J. A.
Montgomery, Jr., R. E. Stratmann, J. C. Burant, S. Dap-
prich, J. M. Millam, A. D. Daniels, K. N. Kudin, M. C.
Strain, O. Farkas, J. Tomasi, V. Barone, M. Cossi, R.
Cammi, B. Mennucci, C. Pomelli, C. Adamo, S. Clifford,
J. Ochterski, G. A. Petersson, P. Y. Ayala, Q. Cui, K.
Morokuma, N. Rega, P. Salvador, J. J. Dannenberg, D. K.
Malick, A. D. Rabuck, K. Raghavachari, J. B. Foresman,
J. Cioslowski, J. V. Ortiz, A. G. Baboul, B. B. Stefanov,
G. Liu, A. Liashenko, P. Piskorz, I. Komaromi, R.
Gomperts, R. L. Martin, D. J. Fox, T. Keith, M. A.
Al-Laham, C. Y. Peng, A. Nanayakkara, M. Challacombe,
P. M. W. Gill, B. Johnson, W. Chen, M. W. Wong, J. L.
Andres, C. Gonzalez, M. Head-Gordon, E. S. Replogle
and J. A. Pople, “Revision A.11.2,” Gaussian, Inc., Pitts-
burgh, 2001.
[44] B. B. Stefanov and J. Cioslowski, “An Efficient Ap-
proach to Calculation of Zero-Flux Atomic Surfaces and
Generation of Atomic Integration Data,” Journal of
Computational Chemistry, Vol. 16, No. 11, 1995, pp.
1394-1404. doi:10.1002/jcc.540161108
[45] T. A. Keith, T. K. Gristmill Software, Overland Park KS,
AIMAll (Version 11.05.16), 2011.
http://aim.tkgristmill.com/
[46] F. B. van Duijneveldt and J. N. Murrell, “Some Calcula-
tions on the Hydrogen Bond,” Journal of Chemical
Physics, Vol. 46, No. 5, 1967, pp. 1759-1768.
doi:10.1063/1.1840932
[47] S. B. Boys and F. Bernardi, “The Calculation of Small
Molecular Interaction by the Differences of Separate To-
tal Energies: Some Procedures with Reduced Errors,”
Molecular Physics, Vol. 19, No. 4, 1970, pp. 553-566.
doi:10.1080/00268977000101561
[48] D. A. McQuarrie, “Statistical Thermodynamics,” Harper
and Row, New York, 1973.
[49] R. S. Rowland and R. Taylor, “Intermolecular Non-
bonded Contact Distances in Organic Crystal Structures:
Comparison with Distances Expected from van der Waals
Radii,” The Journal of Physical Chemistry, Vol. 100, No.
18, 1996, pp. 7384-7391. doi:10.1021/jp953141+
[50] V. M. Goldschmidt, “Geochemische Verteilungsgesetze
der Elemente,” Skrifter Norske Videnskaps, Akad, Oslo,
1926.
[51] L. Pauling, “The Nature of the Chemical Bond,” 3rd Edi-
tion, Cornell University Press, Ithaca, 1960.
[52] B. G. Oliveira and L. F. C. C. Leite, “A Quantum
Chemical Study of Red-Shift and Blue-Shift Hydrogen
Bonds in Bimolecular and Trimolecular Methylhydra-
zine-Hydrate Complexes,” Journal of Molecular Struc-
ture (THEOCHEM), Vol. 915, No. 1-3, 2009, pp. 38-42.
doi:10.1016/j.theochem.2009.08.011
[53] B. G. Oliveira, R. C. M. U. Araújo and M. N. Ramos, “A
Theoretical Study of Blue-Shifting Hydrogen Bonds in π
Weakly Bound Complexes,” Journal of Molecular
Structure (THEOCHEM), Vol. 908, No. 1-3, 2009, pp.
79-83.
[54] B. G. Oliveira, M. C. A. Lima, I. R. Pitta, S. L. Galdino
and M. Z. Hernandes, “A Theoretical Study of
Red-Shifting and Blue-Shifting Hydrogen Bonds Occur-
ring between Imidazolidine Derivatives and PEG/PVP
Polymers,” Journal of Molecular Modeling, Vol. 16, No.
1, 2010, pp. 119-127. doi:10.1007/s00894-009-0525-y
[55] B. G. Oliveira, R. C. M. U. Araújo and M. N. Ramos,
“The (H–δ···H+δ) Charge Transfer and the Evaluation of
the Harmonic Molecular Properties of Dihydro-
gen-Bonded Complexes Formed by BeH2···HX with X =
F, Cl, CN, and CCH,” Journal of Structural Chemistry,
Vol. 19, No. 2, 2008, pp. 185-189.
doi:10.1007/s11224-007-9269-4
[56] B. G. Oliveira, E. M. Duarte, R. C. M. U. Araújo, M. N.
Ramos and A. B. Carvalho, “A Theoretical Study of
Nonlinearity in Heterocyclic Hydrogen-Bonded Com-
plexes,” Spectrochimica Acta Part A, Vol. 61, No. 3,
2005, pp. 491-494. doi:10.1016/j.saa.2004.04.023
[57] B. G. Oliveira, E. C. S. Santos, E. M. Duarte, R. C. M. U.
Araújo, M. N. Ramos and A. B. Carvalho, “An MP2 and
DFT Study of Heterocyclic Hydrogen Complexes
CnHmY-HX with n = 2, m = 4 or 5, Y = O, S or N and X
= F or Cl,” Spectrochimica Acta Part A, Vol. 60, No. 8-9,
2004, pp. 1883-1887. doi:10.1016/j.saa.2003.10.006
[58] R. C. M. U. Araújo, J. B. P. Silva and M. N. Ramos, “An
ab Initio Study of Hydrogen Complexes of the X-H···π
Type between Acetylene and HF or HCl,” Spectro-
chimica Acta Part A, Vol. 51, No. 5, 1995, pp. 821-830.
doi:10.1016/0584-8539(94)00194-G
[59] R. C. M. U. Araújo and M. N. Ramos, “An ab Initio
Study of the Molecular Properties of the Acetylene-HX
Hydrogen Complexes,” Journal of Molecular Structure
(THEOCHEM), Vol. 366, No. 3, 1996, pp. 233-240.
doi:10.1016/0166-1280(96)04536-8
[60] E. B. A. Filho, E. Ventura, S. A. do Monte, B. G. Oliveira,
C. G. L. Junior, G. B. Rocha and M. L. A. A. Vasconcel-
los, “Synthesis and Conformational Study of a New Class
of Highly Bioactive Compounds,” Chemical Physics Let-
ters, Vol. 449, No. 4-6, 2007, pp. 336-340.
doi:10.1016/j.cplett.2007.10.080
[61] B. G. Oliveira, R. C. M. U. Araújo, F. S. Pereira, E. F.
Lima, W. L. V. Silva, A. B. Carvalho and M. N. Ramos,
“A Theoretical Study of Molecular Properties of
C2H4···2HF, C2H2···2HF and C3H6···2HF Trimolecular
Hydrogen-Bonded Complexes,” Química Nova, Vol. 31,
No. 7, 2008, pp. 1673-1688.
doi:10.1590/S0100-40422008000700014

B. G. de OLIVEIRA ET AL.
140
[62] B. G. Oliveira, R. C. M. U. Araujo, A. B. Carvalho and
M. N. Ramos, “An Energetic Quantification of In-
ter-Intramolecular Interactions in the C2H2-2HF and
C2H4O-2HF Trimolecular Hydrogen Bonded Complexes:
DFT Calculations and AIM Topological Parameters,”
Chemical Physics Letters, Vol. 433, No. 4-6, 2007, pp.
390-394. doi:10.1016/j.cplett.2006.11.029
[63] B. G. Oliveira, M. L. A. A. Vasconcellos, R. R. Olinda
and E. B. A. Filho, “Uncommon Hydrogen Bonds be-
tween a Non-Classical Ethyl Cation and π Hydrocarbons:
A Preliminary Study,” Journal of Structural Chemistry,
Vol. 20, No. 1, 2009, pp. 81-90.
doi:10.1007/s11224-008-9401-0
[64] B. G. Oliveira and M. L. A. A. Vasconcellos, “Hydrogen
Bonds in Alcohols: Water Complexes: A Theoretical
Study about New Intramolecular Interactions via
CHELPG and AIM Calculations,” Journal of Molecular
Structure (THEOCHEM), Vol. 774, No. 1-3, 2006, pp.
83-88. doi:10.1016/j.theochem.2006.06.018
[65] B. G. Oliveira and R. C. M. U. Araújo, “Relationship
between Charge Transfer and Intermolecular Interactions
in Heterocyclic Hydrogen-Bonded Complexes,” Química
Nova, Vol. 30, No. 4, 2007, pp. 791-796.
doi:10.1590/S0100-40422007000400007
[66] E. Ramos-Cordoba, D. S. Lambrecht and M.
Head-Gordon, “Charge-Transfer and the Hydrogen Bond:
Spectroscopic and Structural Implications from Elec-
tronic Structure Calculations,” Faraday Discuss, 2011,
Advance Article. DOI: 10.1039/C1FD00004G.
[67] P. L. A. Popelier, “On the Full Topology of the Laplacian
of the Electron Density,” Coordination Chemistry Re-
views, Vol. 197, No. 1, 2000, pp. 169-189.
doi:10.1016/S0010-8545(99)00189-7
[68] R. F. W. Bader, J. Hernández-Trujillo and J. Cortés-
Guzmán, “Chemical Bonding: From Lewis to Atoms in
Molecules,” Journal of Computational Chemistry, Vol.
28, No. 1, 2006, pp. 4-14. doi:10.1002/jcc.20528
[69] S. J. Grabowski, T. L. Robinson and J. Leszczynski,
“Strong Dihydrogen Bonds―ab Initio and Atoms in
Molecules Study,” Chemical Physics Letters, Vol. 386,
No. 1-3, 2006, pp. 44-48.
[70] B. G. Oliveira, F. S. Pereira, R. C. M. U. Araújo and M.
N. Ramos, “The Hydrogen Bond Strength: New Propos-
als to Evaluate the Intermolecular Interaction Using DFT
Calculations and the AIM Theory,” Chemical Physics
Letters, Vol. 427, No. 1-3, 2006, pp. 181-184.
doi:10.1016/j.cplett.2006.06.019