Open Journal of Physical Chemistry, 2011, 1, 118-123
doi:10.4236/ojpc.2011.13016 Published Online November 2011 (http://www.SciRP.org/journal/ojpc)
Buffer Standards for Physiological pH of the Buffer
N-(2-Acetamido)-2-aminoethanesulfonic Acid
from 5˚C to 55˚C
Lakshmi N. Roy, Rabindra N. Roy*, Zachary M. Downs, Blake M. Bodendorfer, Jaime A. Veliz,
Jessica M. Stegner, Isaac B. Henson, Joshua T. Wollen
Hoffman Department of Chemistry, Drury University, Springfield, USA
Received August 23, 2011; revised September 27, 2011; accepted November 1, 2011
Abstract
Electromotive force (emf) measurements of the Cell Pt(s), H2(g)|ACES(m1) + NaACES(m2) + NaCl (m3)|
AgCl, Ag(s) have been carried out from 5˚C to 55˚C. The agreement of pH values between two calculated
(extended Debye-Hückel and liquid junction correction) is very good. Two buffer solutions without the chlo-
ride ion and seven buffer solutions with NaCl, at an ionic strength (I = 0.16 mol·kg–1) similar to that of
physiological fluids, have been studied. The pH values for these buffer solutions have been evaluated in the
temperature range of 5˚C to 55˚C using the extended Debye-Hückel equation of the Bates-Guggenheim con-
vention. Values of the residual liquid junction potential (δEj) between the ACES solutions and the saturated
KCl calomel electrode solution have been estimated at 25˚C and 37˚C from the previously determined Ej val-
ues using the flowing junction cell to determine the operational pH values at 25˚C and 37˚C. These ACES
buffer solutions are recommended as secondary standard reference solutions for pH measurements in the
range of physiological application at I = 0.16 mol·kg–1.
Keywords: Buffer, Emf, pH, Zwitterionic
1. Introduction
The zwitterionic amino acid buffer solutions recom-
mended by Good et al. [1,2] have been studied in the
authors’ laboratory for the purpose of pH measurement
control in the physiological range of pH. Concerning the
current investigation, the authors’ goal is to provide pH
values for the ACES, which is depicted by the following
structure:
N
O
H2N S
O
OOH
H
N-(2-acetamido)-2-aminoethanesulfonic acid
ACES
This phosphate buffer [3,4] is comprised of KH2PO4
(0.008695 mol·kg–1) and Na2HPO4 (0.03043 mol·kg–1).
This buffer solution is widely used as the primary refer-
ence standard for routine laboratory measurements.
Some of the limitations have been previously reported
[5-8].
The zwitterionic buffer compound ACES may form
complexes with cations such as Mg+2 and Ca+2. At a high
NaCl concentration, the complex formation is mini-
mized.
Wu et al. [7] have studied MOPSO using two point pH
calibration measurements. Roy et al. [9] studied pK2 and
pH values of 3-(N-morpholino)propanesulfonic acid
(MOPS) from 5˚C to 55˚C.
The following two solutions of ACES were studied
without the presence of the chloride ion: (a) ACES (0.02
mol·k g–1) + NaACES (0.06 mol·kg–1); (b) ACES (0.04
mol·k g–1) + NaACES (0.08 mol·kg–1).
The following seven solutions were also studied but
contain the chloride ion yielding an ionic strength close
to that of blood plasma (I = 0.16 mol·kg–1): (c) ACES
(0.08 mol·kg–1) + NaACES (0.08 mol·kg–1) + NaCl (0.08
mol·k g–1); (d) ACES (0.06 mol·kg–1) + NaACES (0.06
mol·k g–1) + NaCl (0.10 mol·kg–1); (e) ACES (0.04
mol·k g–1) + NaACES (0.04 mol·kg–1) + NaCl (0.12
mol·k g–1); (f) ACES (0.03 mol·kg–1) + NaACES (0.03
mol·k g–1) + NaCl (0.13 mol·kg–1); (g) ACES (0.03

119 L. N. ROY ET AL.
mol·k g–1) + NaACES (0.09 mol·kg–1) + NaCl (0.07
mol·k g–1); (h) ACES (0.02 mol·kg–1) + NaACES (0.08
mol·k g–1) + NaCl (0.08 mol·kg–1); (i) ACES (0.02
mol·k g–1) + NaACES (0.10 mol·kg–1) + NaCl (0.06
mol·k g–1).
2. Experimental Section
The ACES was obtained from Research Organics (Cle-
veland, OH) and was crystallized further. The method
and the assay have been reported elsewhere [8,9]. The
analyses averaged 99.97% with a standard deviation of
0.05%. Buffer were prepared from the weighed amount
of the ACES solid, ACS reagent grade NaCl, a standard
NaOH solution (for preparation of NaACES), and CO2-
free doubly distilled water. Buoyancy corrections were
made for all masses.
The cell design, method of preparation of hydrogen
electrodes, hydrogen gas purification, silver-silver chlo-
ride electrodes, solution preparation, temperature meas-
urements, and emf techniques have been described [8,10,
11].
3. Methods and Results
The emf values required for the pH(s) calculations are
given in Table 1 for cell A. Cell A contains two solutions
without the chloride ion and seven solutions with Cl–.
The cell voltage values have been corrected to a hydro-
gen pressure of 1 atm. At 25˚C, the emf values are aver-
aged from readings taken twice at the beginning and the
middle. The uncertainty lies within 0.02 mV on the av-
erage in.
pH of the ACES Buffer. The Bates et al. [7,8,11-13]
method has been used to evaluate the conventional stan-
dard pH values for solutions (a) to (i). For accurate cal-
culations of pH for nine buffer solutions, the following
cell A is used:
Pt(s), H2(g), 101.325 kPa|ACES (m1)
+ NaACES (m2) + NaCl(m3)|AgCl(s), Ag(s) (A)
where m1, m2 and m3 denote the molalities of the respec-
tive species at 1 atm = 101.325 kPa. Cell A is known as
the Harned-type cell.
Cell B, the flowing junction cell, was used for the
evaluation of the liquid junction potential:
Pt(s), H2(g), 101.325 kPa|ACES(m1) + NaACES(m2)
+ NaCl(m3)||KCl(satd), Hg2Cl2(s), Hg(l) (B)
where the abbreviations “s” “l” and “g” imply the solid,
liquid, and gaseous states, respectively.
For cell C, a primary standard phosphate buffer solu-
tion, NBS/NIST certified, was used. The diagram of cell
C is shown below:
Pt(s), H2(g), 101.325 kPa|KH2PO4
+ Na2HPO4||KCl(satd), Hg2Cl2(s), Hg(l) (C)
The SCE values of the saturated calomel electrode
were taken as –0.2415 and –0.2335 at 25˚C and 37˚C,
respectively [14]. The residual liquid junction potential
δEj values of the buffer solution of ACES were calcu-
lated from cells B and C using the following equation:
E
δEj = E + – k˚pH (1)
SCE
E

where k = 0.059156 and pH = 7.415 at 25˚C and k =
0.061538 and pH = 7.395 at 37˚C. The pH values are that
of the standard phosphate buffer solution and buffer so-
lution of ACES obtained using the extended De-
bye-Hückel equation. The operational definition of pH,
denoted as pH(x), can be calculated by use of the subse-
quent equation
pH(x) = pH(s) + (Ex – Es + δEj)/k (2)
where “x” refers to the unknown buffer, “s” is the
NBS/NIST standard phosphate buffer solution of known
pH, and
 Ej = Ej(s) – Ej(x).
To calculate the pH(s) values for the buffer solutions
under investigation, calculations were made to determine
acidity function, p(aH

Cl), in the temperature range of
25˚C and 37˚C. These calculations were made using emf
values listed in Table 1, the molality of the chloride ion,
and the standard electrode potential of the silver-silver
chloride electrode (E˚). The values of p(aH

Cl) were ob-
tained from the equation below:
() log
H
Cl Cl
EE
pa m
k


 (3)
where “k” is the Nernst slope.
The values of p(aH 
Cl) were plotted against the mo-
lality of the chloride ion. A linear line was obtained. The
intercept is the p(aH 
Cl)˚ value at mCl¯ = 0. The p(aH

Cl)
values for the seven buffer solutions containing Cl¯ are
entered in Tables 2 and 3 from 5˚C to 55˚C.
Conventional pH(s) values for solutions without liquid
junction and absence a chloride ion were determined
using the equation:
o
()() log
H
Cl Cl
pHsp a

  (4)
where the single-ion activity coefficient, o
Cl

, can be
estimated based on corrections. A previous publication
outlines the method used for obtaining this figure [9].
The pH values obtained from the liquid junction cell are
indicated by pH whereas the “conventional” pH calcu-
lated from Equations (5) and (6) are designated as pH(s).
The Bates-Guggenheim convention [15,16], is expressed
y the equation: b

L. N. ROY ET AL.
120
Table 1. Cell Potential of Cell A (in Volts): Pt(s); H2(g), 101.35 kPa|ACES(m1), NaACES(m2), NaCl(m3)|AgCl(s), Ag
(s).
m1 m2 m3 T/˚C
mol· kg–1 5 10 15 20 25 30 35 37 40 45 50 55
0.02 0.06 0.005
0.02 0.06 0.010
0.02 0.06 0.015
0.02 0.06 0.020
0.04 0.08 0.005
0.04 0.08 0.010
0.04 0.08 0.015
0.04 0.08 0.020
0.08 0.08 0.080
0.06 0.06 0.100
0.04 0.04 0.120
0.03 0.03 0.130
0.03 0.09 0.070
0.02 0.08 0.080
0.02 0.10 0.060
0.79035
0.7736
0.76380
0.75659
0.77395
0.75930
0.75154
0.74645
0.69577
0.69083
0.68684
0.68510
0.72884
0.73082
0.75064
0.79189
0.77487
0.76467
0.75741
0.77491
0.75990
0.75176
0.74651
0.69532
0.69030
0.69629
0.68453
0.72906
0.73107
0.75128
0.79332
0.77591
0.76539
0.75803
0.77581
0.76029
0.75188
0.74629
0.69475
0.68972
0.68565
0.68391
0.72911
0.73119
0.75170
0.79440
0.77671
0.76607
0.75848
0.77641
0.76038
0.75143
0.74557
0.69403
0.68869
0.68498
0.68322
0.72905
0.73119
0.75202
0.79541
0.77739
0.76655
0.75880
0.77666
0.76004
0.75064
0.74427
0.69331
0.68805
0.68379
0.68195
0.72980
0.73109
0.75210
0.79654
0.77819
0.76717
0.75921
0.77766
0.76140
0.75253
0.74672
0.69244
0.68710
0.68281
0.68093
0.72878
0.73086
0.75213
0.79731
0.77868
0.76745
0.75943
0.77828
0.76166
0.75276
0.74680
0.69146
0.68600
0.68160
0.67968
0.72846
0.73068
0.75174
0.79779
0.77898
0.76763
0.75949
0.77844
0.76190
0.75301
0.74714
0.69104
0.68558
0.68126
0.67935
0.72827
0.73047
0.75196
0.79807
0.77914
0.76773
0.75957
0.77855
0.76266
0.75411
0.74884
0.69035
0.68487
0.68052
0.67860
0.72792
0.73020
0.75184
0.79866
0.77941
0.76794
0.75956
0.77884
0.76262
0.75418
0.74891
0.68924
0.68373
0.67922
0.67726
0.72743
0.72970
0.75169
0.79921
0.77968
0.76790
0.75948
0.77917
0.76292
0.75449
0.74955
0.68815
0.68257
0.67805
0.67601
0.72674
0.72912
0.75128
0.79961
0.77980
0.76781
0.75941
0.77911
0.76266
0.75428
0.74911
0.68797
0.68193
0.67702
0.67486
0.72590
0.72814
0.75082
am = 1 mol·kg–1.
Table 2. p(aHγCl)˚ of ACES + NaACES buffer solutions from 5˚C to 55˚C obtained by extrapolation for chloride-free solutions,
p(aHγCl) of ACES + NaACES buffer solutions from 5˚C to 55˚C, computed using Equations (4)-(6)a.
T (˚C)
0.02 m ACES
+ 0.06 m NaACES
+ 0.00 m NaCl
I = 0.06 m
0.04 m ACES
+ 0.08 m NaACES
+ 0.00 m NaCl
I = 0.08 m
0.08 m ACES
+ 0.08 m NaACES
+ 0.08 m NaCl
I = 0.16 m
0.06 m ACES
+ 0.06 m NaACES
+ 0.10 m NaCl
I = 0.16 m
0.04 m ACES
+ 0.04 m NaACES
+ 0.12 m NaCl
I = 0.16 m
0.03 m ACES
+ 0.03 m NaACES
+ 0.13 m NaCl
I = 0.16 m
5
10
15
20
25
30
35
37
40
45
50
55
7.781
7.679
7.582
7.484
7.391
7.306
7.218
7.189
7.136
7.056
6.980
6.904
7.446
7.341
7.241
7.145
7.050
6.956
6.871
6.836
6.780
6.694
6.145
6.537
7.267
7.159
7.056
6.956
6.863
6.772
6.684
6.650
6.600
6.520
6.446
6.388
7.275
7.167
7.065
6.966
6.871
6.780
6.692
6.659
6.608
6.530
6.455
6.392
7.281
7.175
7.073
6.977
6.878
6.788
6.699
6.668
6.617
6.538
6.464
6.396
7.285
7.178
7.077
6.981
6.882
6.791
6.702
6.671
6.621
6.541
6.467
6.397
Table 3. p(aHγCl) of ACES + NaACES buffer solutions from 5˚C to 55˚C, computed using Equations (4)-(6)a.
T (˚C)
0.03 m ACES
+ 0.09 m NaACES
+ 0.07 m NaCl
I = 0.16 m
0.02 m ACES
+ 0.08 m NaACES
+ 0.08 m NaCl
I = 0.16 m
0.02 m ACES
+ 0.10 m NaACES
+ 0.06 m NaCl
I = 0.16 m
5
10
15
20
25
30
35
37
40
45
50
55
7.809
7.702
7.599
7.500
7.406
7.318
7.231
7.197
7.146
7.067
6.989
6.912
7.902
7.796
7.693
7.595
7.501
7.411
7.325
7.291
7.241
7.161
7.084
7.005
8.136
8.030
7.926
7.828
7.731
7.639
7.544
7.515
7.434
7.384
7.305
7.228
am = 1 mol·kg–1.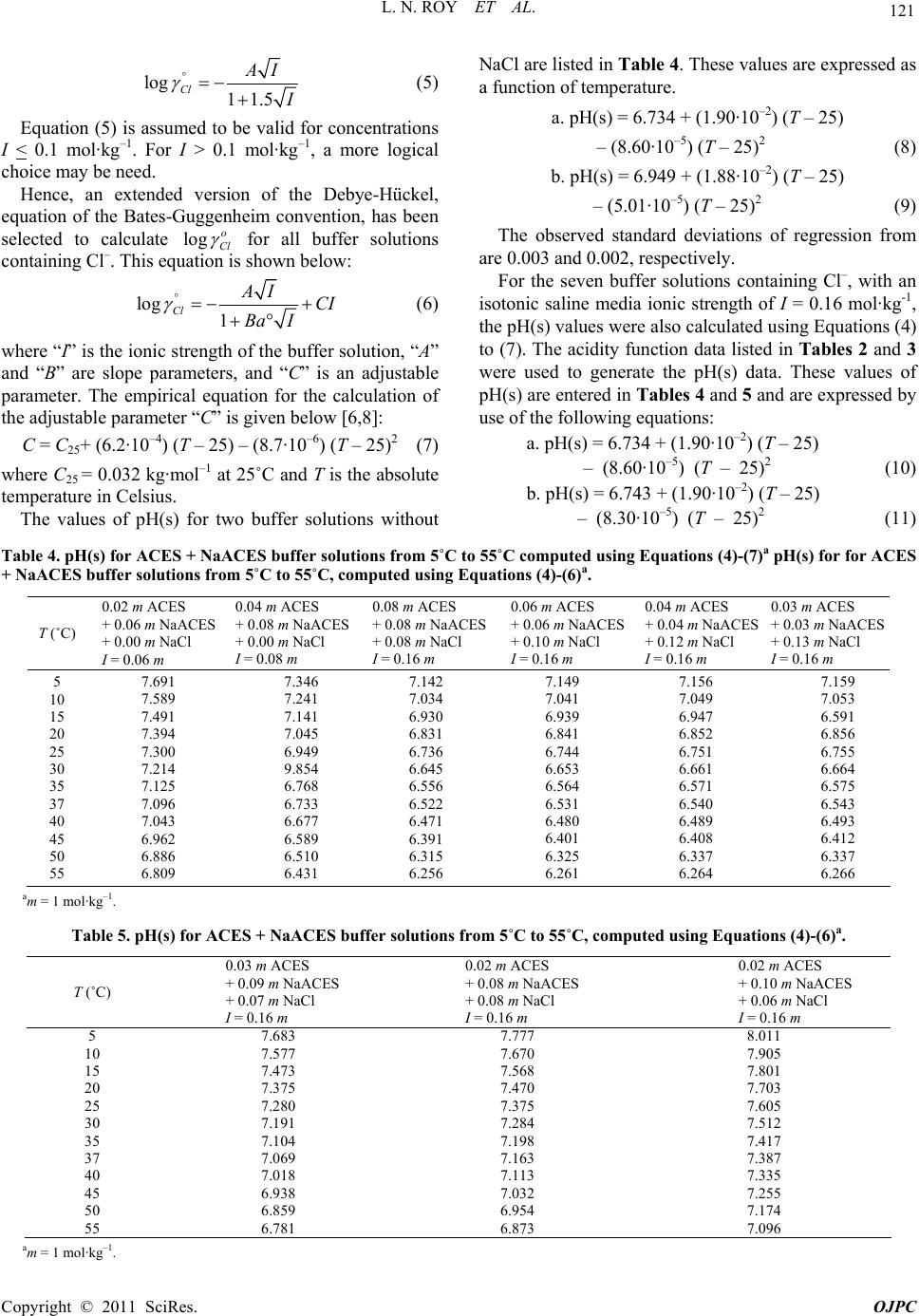

121 L. N. ROY ET AL.
log 11.5
Cl
A
I
I

  (5)
Equation (5) is assumed to be valid for concentrations
I <
0.1 mol·kg–1. For I > 0.1 mol·kg–1, a more logical
choice may be need.
Hence, an extended version of the Debye-Hückel,
equation of the Bates-Guggenheim convention, has been
selected to calculate log o
Cl

for all buffer solutions
containing Cl–. This equation is shown below:
log 1
Cl
AI CI
Ba I

 
 (6)
where “I” is the ionic strength of the buffer solution, “A”
and “B” are slope parameters, and “C” is an adjustable
parameter. The empirical equation for the calculation of
the adjustable parameter “C” is given below [6,8]:
C = C25+ (6.2·10–4) (T – 25) – (8.7·10–6) (T – 25)2 (7)
where C25 = 0.032 kg·mol–1 at 25˚C and T is the absolute
temperature in Celsius.
The values of pH(s) for two buffer solutions without
NaCl are listed in Table 4. These values are expressed as
a function of temperature.
a. pH(s) = 6.734 + (1.90·10–2) (T – 25)
– (8.60·10–5) (T – 25)2 (8)
b. pH(s) = 6.949 + (1.88·10–2) (T – 25)
– (5.01·10–5) (T – 25)2 (9)
The observed standard deviations of regression from
are 0.003 and 0.002, respectively.
For the seven buffer solutions containing Cl–, with an
isotonic saline media ionic strength of I = 0.16 mol·kg-1,
the pH(s) values were also calculated using Equations (4)
to (7). The acidity function data listed in Tabl es 2 and 3
were used to generate the pH(s) data. These values of
pH(s) are entered in Tab l es 4 and 5 and are expressed by
use of the following equations:
a. pH(s) = 6.734 + (1.90·10–2) (T – 25)
– (8.60·10–5) (T – 25)2 (10)
b. pH(s) = 6.743 + (1.90·10–2) (T – 25)
– (8.30·10–5) (T – 25)2 (11)
Table 4. pH(s) for ACES + NaACES buffer solutions from 5˚C to 55˚C computed using Equations (4)-(7)a pH(s) for for ACES
+ NaACES buffer solutions from 5˚C to 55˚C, computed using Equations (4)-(6)a.
T (˚C)
0.02 m ACES
+ 0.06 m NaACES
+ 0.00 m NaCl
I = 0.06 m
0.04 m ACES
+ 0.08 m NaACES
+ 0.00 m NaCl
I = 0.08 m
0.08 m ACES
+ 0.08 m NaACES
+ 0.08 m NaCl
I = 0.16 m
0.06 m ACES
+ 0.06 m NaACES
+ 0.10 m NaCl
I = 0.16 m
0.04 m ACES
+ 0.04 m NaACES
+ 0.12 m NaCl
I = 0.16 m
0.03 m ACES
+ 0.03 m NaACES
+ 0.13 m NaCl
I = 0.16 m
5
10
15
20
25
30
35
37
40
45
50
55
7.691
7.589
7.491
7.394
7.300
7.214
7.125
7.096
7.043
6.962
6.886
6.809
7.346
7.241
7.141
7.045
6.949
9.854
6.768
6.733
6.677
6.589
6.510
6.431
7.142
7.034
6.930
6.831
6.736
6.645
6.556
6.522
6.471
6.391
6.315
6.256
7.149
7.041
6.939
6.841
6.744
6.653
6.564
6.531
6.480
6.401
6.325
6.261
7.156
7.049
6.947
6.852
6.751
6.661
6.571
6.540
6.489
6.408
6.337
6.264
7.159
7.053
6.591
6.856
6.755
6.664
6.575
6.543
6.493
6.412
6.337
6.266
am = 1 mol·kg–1.
Table 5. pH(s) for ACES + NaACES buffer solutions from 5˚C to 55˚C, computed using Equations (4)-(6)a.
T (˚C)
0.03 m ACES
+ 0.09 m NaACES
+ 0.07 m NaCl
I = 0.16 m
0.02 m ACES
+ 0.08 m NaACES
+ 0.08 m NaCl
I = 0.16 m
0.02 m ACES
+ 0.10 m NaACES
+ 0.06 m NaCl
I = 0.16 m
5
10
15
20
25
30
35
37
40
45
50
55
7.683
7.577
7.473
7.375
7.280
7.191
7.104
7.069
7.018
6.938
6.859
6.781
7.777
7.670
7.568
7.470
7.375
7.284
7.198
7.163
7.113
7.032
6.954
6.873
8.011
7.905
7.801
7.703
7.605
7.512
7.417
7.387
7.335
7.255
7.174
7.096
am = 1 mol·kg–1.

L. N. ROY ET AL.
122
Table 6. Emf of Cell B and pH values with δEj correction at 25˚C and 37˚C for ACES buffer.
E/V δEjb/mV Withoutc
δEj corr
Withd
δEj corr
Extendede
D-H eqn.
Withoutc
δEj corr
Withd
δEj corr
Extendede
D-H eqn.
m1 m2 m3 I
25˚C 37˚C 25˚C 37˚C25˚C 37˚C
0.02 0.06 0.00 0.06 0.67559 0.67263 0.3 0.4 7.294 7.299 7.300 7.089 7.095 7.096
0.02 0.08 0.08 0.16 0.67825 0.67498 2.1 2.2 7.338 7.374 7.375 7.127 7.162 7.163
0.03 0.09 0.07 0.16 0.67263 0.66917 2.1 2.2 7.244 7.279 7.280 7.033 7.068 7.069
0.02 0.01 0.06 0.16 0.69186 0.68874 2.1 2.2 7.569 7.604 7.605 7.351 7.386 7.387
Emf of Cell Ca
0.008695 m KH2PO4
+ 0.03043 m Na2HPO4 0.68275 0.69147 2.6 2.9
aCorrected to a hydrogen pressure of 101.325 kPa for physiological phosphate buffer solutions (primary reference standard buffer) at 25˚C and 37˚C; bδEj = E +
– k˚ pH from Equation (1); the pH of the primary standard phosphate buffer solution is 7.415 and 7.395 at 25˚C and 37˚C, respectively; = elec-
trode potential of the saturated calomel electrode = –0.2415 and –0.2335 at 25˚C and 37˚C [14], respectively; units of m, mol·kg–1; cValues obtained from Equa-
tion (2) where δEj = 0 and Table 6; dObtained from Equation (2) and cell potential data from Table 6; eObtained from extended Debye-Hückel (DH) equation of
the Bates-Guggenheim convention.
SCE
E

SCE
E

c. pH(s) = 6.752 + (1.90·10–2) (T – 25)
– (7.80·10–5) (T – 25)2 (12)
d. pH(s) = 6.756 + (1.90·10–2) (T – 25)
– (7.66·10–5) (T – 25)2 (13)
e. pH(s) = 7.282 + (1.86·10–2) (T – 25)
– (6.68·10–5) (T – 25)2 (14)
f. pH(s) = 7.376 + (1.86·10–2) (T – 25)
– (6.57·10–5) (T – 25)2 (15)
g. pH(s) = 7.604 + (1.90·10–2) (T – 25)
– (6.90·10–5) (T – 25)2 (16)
The observed standard deviations of regression from
Equations (10)-(17) are 0.003, 0.002, 0.002, 0.002, 0.002,
0.002 and 0.002, respectively.
4. Discussion and Conclusions
The operational pH values at 25˚C and 37˚C were evalu-
ated from cells with a liquid junction (cells B and C) by
means of the flowing junction cell [6,8]. The emf values
of cells B and C at 25˚C and 37˚C are given in Table 6
for four buffer solutions of ACES. The values of δEj are
also listed in Table 6. The estimated uncertainties are
due to 1) the calculation of logγCl, 2) extrapolation of the
acidity function, 3) emf measurements, and 4) the esti-
mation of δEj. From Table 6, the pH values of four
buffer solutions lie in the range of 7.1 to 7.6. Thus, these
buffer solutions are recommended as useful standards for
pH measurements in the clinical laboratory. The overall
uncertainty of pH is within 0.01 in the experimental tem-
perature range. Work is in progress for the calculation of
pH of the buffer solution using the specific ionic interac-
tion theory of Pitzer [17,18] for the calculation of logγCl.
The main application of these pH data is to establish a
unified pH scale applicable to a wide range of ionic str-
engths for practical pH measurements.
5. Acknowledgements
The authors are grateful for the funding from the Na-
tional Institutes of Health (AREA), under the grant 2-
R15 GM 066866-03 and the diversity supplemental grant
3-R15 GM 066866-03 S1. The authors would also like to
thank Levi Lowther for his dedicated and hard work. The
content of this paper is the sole responsibility of the au-
thors and does not necessarily represent the official
views of the National Institutes of Health or the National
Institutes of General Medical Sciences.
6. References
[1] N. E. Good, G. D. Winget, W. Winter, T. N. Connolly, S.
Izawa and R. M. M. Singh, “Hydrogen Ion Buffers for
Biological Research,” Biochemistry, Vol. 5, No. 2, 1966,
pp. 467-477. doi:10.1021/bi00866a011
[2] W. J. Ferguson, K. L. Braunschweiger, W. R. Braun-
schweiger, J. R. Smith, J. J. McCormick, C. C. Wasmann,
N. P. Jarvis, D. H. Bell and N. E. Good, “Hydrogen Ion
Buffers for Biological Research,” Analytical Biochemis-
try, Vol. 104, 1980, pp. 300-310.
doi:10.1016/0003-2697(80)90079-2
[3] R. N. Roy, J. Bice, J. Greer, J. A. Carlsten, J. Smithson,
W. S. Good, L. N. Roy and K. M. Kuhler, “Buffers for
the Physiological pH Range: Acidic Dissociation Con-
stants of Zwitterionic Compounds (ACES and CHES) in
Water from 5˚C to 55˚C,” Journal of Chemical & Engi-
neering Data, Vol. 42, No. 1, 1997, pp. 41-44.
doi:10.1021/je960279s
[4] V. E. Bower, M. Paabo and R. G. Bates, “A Standard for
the Measurement of the pH of Blood and Other Physio-
logical Media,” Journal of Research of the National Bu-
reau of Standards, Vol. 65A, 1961, pp. 267-270.
[5] R. A. Durst and B. R. Staples, “Tris/Tris HCl: Standard
Buffer for Use in the Physiological pH Range,” Clinical
Chemistry, Vol. 18, 1972, pp. 206-208.

123 L. N. ROY ET AL.
[6] D. Feng, W. F. Koch and Y. C. Wu, “Second Dissocia-
tion Constant and pH of N-(2-Hydroxyethyl) piperazine-
N’-2-ethanesulfonic Acid from 0˚C to 50˚C,” Analytical
Chemistry, Vol. 61, No. 13, 1989, pp. 1400-1405.
doi:10.1021/ac00188a019
[7] Y. C. Wu, P. A. Berezansky, D. Feng and W. F. Koch,
“Second Dissociation Constant of 3-(N-Morpholino)-2-
hydroxypropanesulfonic Acid and pH of Its Buffer Solu-
tions,” Analytical Chemistry, Vol. 65, No. 8, 1993, pp.
1084-1087. doi:10.1021/ac00056a023
[8] R. N. Roy, D. R. Mrad, P. A. Lord, J. A. Carlsten, W. S.
Good, P. Allsup, L. N. Roy, K. M. Kuhler, W. F. Koch
and Y. C. Wu, “Thermodynamics of the Second Disso-
ciation Constant and Standards for pH of 3-(N-Morpho-
lino)propanesulfonic Acid (MOPS) from 5˚C to 55˚C,”
Journal of Solution Chemistry, Vol. 27, No. 1, 1998, pp.
73-87. doi:10.1023/A:1022692629289
[9] R. G. Bates, C. A. Vega and D. R. White Jr., “Standards
for pH Measurements in Isotonic Saline Media of Ionic
Strength I = 0.16,” Analytical Chemistry, Vol. 50, No. 9,
1978, pp. 1295-1300. doi:10.1021/ac50031a026
[10] R. G. Bates, “Determination of pH,” 2nd Edition, Wiley
& Sons, New York, 1973.
[11] R. N. Roy, L. N. Roy, C. E. Denton, S. R. LeNoue, S.
Ashkenazi, M. S. Fuge, C. D. Dunseth, J. L. Durden, C.
N. Roy, A. Bwashi, J. T. Wollen and S. J. DeArmon,
“Buffer Standards for the Physiological pH of 3-[(1,1-Di
methyl-2-hydroxymethyl)-amino]-2-hydroxypropanesulf
onic Acid from 278.15 to 328.15 K,” Journal of Chemi-
cal & Engineering Data, Vol. 54, 2009, pp. 428-435.
[12] L. N. Roy, R. N. Roy, C. E. Denton, S. R. LeNoue, C. N.
Roy, S. Ashkenazi, T. B. Williams, D. R. Church, M. S.
Fuge and K. N. Sreepada, “Second Dissociation Constant
of Bis-[(2-hydroxyethyl)amino]acetic Acid (BICINE) and
pH of Its Buffer Solutions from 5˚C - 55˚C,” Journal of
Solution Chemistry, Vol. 35, No. 4, 2006, pp. 605-624.
doi:10.1007/s10953-005-9009-6
[13] R. N. Roy, L. N. Roy, S. Ashkenazi, J. T. Wollen, C. D.
Dunseth, M. S. Fuge, C. N. Roy, H. M. Hughes, B. T.
Morris and K. L. Cline, “Buffer Standards for pH Meas-
urement of N-(2-Hydroxyethyl)piperazine-N’-2-ethane-
sulfonic Acid (HEPES) for I = 0.16 mol·kg–1 from 5˚C to
55˚C,” Journal of Solution Chemistry, Vol. 38, No. 4,
2009, pp. 449-458. doi:10.1007/s10953-009-9378-3
[14] W. M. Latimer, “Oxidation Potentials,” 2nd Edition,
Prentice-Hall, New York, 1952.
[15] C. A. Vega and R. G. Bates, “Buffers for the Physiologi-
cal pH Range: Thermodynamic Constants of Four Sub-
stituted Aminoethanesulfonic Acids from 5˚C to 50˚C,”
Analytical Chemistry, Vol. 48, 1976, pp. 1293-1296.
[16] R. G. Bates and E. A. Guggenheim, “Report on the Stan-
dardization of pH and Related Terminology,” Pure and
Applied Chemistry, Vol. 1, 1960, pp. 163-168.
doi:10.1351/pac196001010163
[17] K. S. Pitzer and G. J. Mayorga, “Thermodynamics of
Electrolytes. II. Activity and Osmotic Coefficients for
Strong Electrolytes with One or Both Ions Univalent,”
Journal of Physical Chemistry, Vol. 77, No. 19, 1973, pp.
2300-2307. doi:10.1021/j100638a009
[18] M. I. A. Ferra, “A Pitzer Theory Approach to Assignment
of pH to Standard Buffer Solutions,” Electrochimica Acta,
Vol. 16, 1998, pp. 133-142.