 Advances in Pure Mathematics, 2011, 1, 305-314 doi:10.4236/apm.2011.16056 Published Online November 2011 (http://www.SciRP.org/journal/apm) Copyright © 2011 SciRes. APM Uniform Convergence of Extremal Polynomials When Domains Have Corners and Special Cusps on the Boundary Cem Koşar1, Mehmet Kucukaslan2, Fahreddin G. Abdullayev2 1Department of Mat hem at i cs, Facult y of S cience and L iterat ur e , Bitlis Eren University, Bitlis, Turkey 2Department of Mat hem at i cs, Facult y of S cience and L iterat ur e , Mersin University, Mersin, Turkey E-mail: ckosar77@gmail.com, {mkucukaslan, fabdul}@mersin.edu.tr Received May 26, 2011; revised September 13, 2011; accepted Septemb er 22, 2011 Abstract We study the approximation properties of the extremal polynomials in Ap-norm and C-norm. We prove esti- mates for the rate of such convergence of the sequence of the extremal polynomials on domains with corners and special cusps. Keywords: Uniform Approximation, Extremal Polynomials, Conformal Mapping, p-Bieberbach Polynomials 1. Introduction and Main Results 1.1. Statement of Problem and Some Definitions Let be a finite domain bounded by Jordan curve and 0 G G:=LG be an arbitrary fixed point. Let be conformal mapping of onto the disk wz =G 0 0 0, :=Dr 0=0, : <wwr 0=1 with normalization , where 0 is a conformal radius of G with respect to r 0 and let 1 := be an inverse mapping. Let We denote by >0.p 1 p G the set of functions z =0,f analytic in and normalized by such that G 0 f 0=1 1 1:=d< , p p 'z AG pG ffz where d is a two-dimensional Lebesque measure on .G We denote by n of all algebraic polynomials satisfying n n ,deg n Pz P 0=0, n P 0=1. n P Let us consider following extremal problem: 1: nnn AG p PP min (1.1) Using a method similar to the one given in ([1], p. 137), it is seen that there exists an extremal polynomial n Pz furnishing to the problem (1.1), these polyno- mials n Pz are determined uniquely in case of ([1], p. 142). This extremal problem was first considered by Kucukaslan M. and Abdullayev F.G. and they were called the p-Bieberbach polynomial of degree for the pair >1p n 0 ,G in [2], and denoted by ,. np Bz The main goal of this paper is to investigate the approximation rate of convergence to the function ,np Bz in uniform norm for some domains when has some certain singularity, i.e. ,, () := max, np np CG zG c BzBz n (1.2) where :=,> 0,pG the constant is independent of c .n In case of the solution of (1.1) coincides with the well known Bieberbach polynomial =2,p -nth z ,2nn (see, for example, [3,4] ). The appro- ximation properties in the uniform norm of Bz B n Bz on G first was observed by Keldysh in 1939 [3] for the domains with sufficiently smooth boundary. A consi- derable progress in this area has been achieved by Mergelyan [5], Suetin [4], Simonenko [6], Andrievskii [7,8] Gaier [9,10], Abdullayev [11-13], Israfilov [14,15] and the others. In this paper, we are going to consider the case the problem in (1.2). First, we will investigate the approximation rate of to the function >1p ,np Bz in  C. KOŞAR ET AL. 306 1- norm and using well known Simonenko and Andrievskii method (see, for example, [6,7]), the appro- ximation rate of to the function ,np Bz in uniform norm will be obtained. Now we need some definitions: Definition 1.1. ([16], p. 97)The Jordan arc or a curve is called a quasiconformal () arc or curve if there is a quasiconformal mapping L-K1K -K of a region containing such that D L L is line segment or circle. Let L denote the set of all sense-preserving plane homeomorphisms of regions such that DL L is a line segment or circle and let =inf : , LKffFL where f . is the maximal dilatation of such a map- ping Then is L- quasiconformal if and only if If is a <.KL L- quasiconformal, then . LK D gives the global definition of a - qua- siconformal arc or curve consequently. This definition is common in the literature. At the same time, we can consider the domain as the neighborhood of the curve In this case, Definition 1.1 will be called local definition of quasiconformal arc or curve. Through this work we consider the local definition. The local defi- nition has an advantage in determining the coefficients of quasiconformality for some simple arcs or curves. D =,zzs L .L Let us denote 0, mesL natural repre- sentation of := .LG Definition 1.2. ([4]) We say that GC if G has a continuous tangent := zs ,GC for every points . zs Corollary 1.1. ([17]) If then =1K for all >0. Definition 1.3. ([12]) We say that if is expressed as a union of a finite number of ;, 0 0<<2, ,GC :=L -CG arcs, connecting at the points 0, 1 such that is locally smooth at 0 and in the local coordinate system ,,, m zzz L ,z y with origin at , z the following conditions are satisfied: 1j,m , 1) For every z the domain has 1jp ,mG π, j 0< < j2, exterior angle at the corner , z =: . j min 2) For every , z in 1,pj m , y coor- dinate system with origin at z we have 11 12 1 =:,0,zxiyycx xG cx , 21 =: ,0zxiyyxxG C >0 for some constants 12 << <cc ,, i =1,2.i It is clear from the definition that each domain ; GC may have exterior π angles, 0< <2 j , at the points z, , and interior zero angles at which the boundary arcs are touching with 1jp 1-x speed at the points z, . If 1pj m=0 then the domain does not has interior zero angles and G ,0< 2GC < , i.e., .;0CC If =1 then the domain has piecewise smooth boundary with only interior zero angles. We denote the class of domains by G .1;C 1.2. New Results We introduce the following notation. For any and 1<< 2p 0< <2 we set ,,, j pp j and , jp as follows: 11 0 <, 2 42 12 ,:=, <, 222 23 12 1, < 2, 23 p , 3 pp = 1, =2, = 3, j pj pj j 2 2 8 pp 1, 42 4 33 , 42 4 ,:= 23 , 4 1 142, . 2 ppp j j ppj pp p = 1, =2, = 3, =4 j j 2,= 2 11 , =2, 22 ,:=1, =3, 12 1 ,= 12 j j p j p pj p j p 1, 4. Throughout this paper, 12 are positive and 12 ,,,ccc ,, ,, sufficiently small positive constants which in general depend on . G Theorem 1.2. Let and assume that 1< <2p ; GC for some 1 0< 2 and 11 min,, ,pp0< . Then the Bieberbach polynomial satisfies -p ,np Bz ,1np CG Bcn with 1 2 0<<22. p p for each Copyright © 2011 SciRes. APM  C. KOŞAR ET AL.307 Theorem 1.3. Let 62 <<2 6p and assume that ;GC for some 1 < 23 2 . and 22 0<min,,,pp ,np Bz Then the Bie- berbach polynomial satisfies -p ,2np CG Bcn for each with 2 2 0<<2 2. p p Theorem 1.4. Let 3<<2 2p and assume that ;GC for some 2<<2 3 and 3 23 0<min ,, 4 p p . Then the Bieberbach polynomial satisfies -p ,np Bz ,3np CG Bcn for each with 3 2 0<<2 2.p p For 0<<1 we obtain 1 1 max,,=1, 2, 3=,= ipj p and so, we have the following theorem. Theorem 1.5. Let 2 2 14 2 max1,<< 2 222 p and assume that ;GC for some 2<<1 9 and 4 1<, p . Then the Bieberbach poly- -p nomial satisfies ,np Bz ,4 ln np CG Bcnn for each with 4 2 0<<2 2.p p Analogously result can be written for case 1<2. Theorem 1.6. Let 2 2< <21 p and assume that ;GC for some 0<<2 , and 0. Then, for any and arbitrary small 3n>0 . ,5np CG Bcn for each Corollary 1.7. Let and assume that =2p ;GC for some 0< <2 and 11 0<min, 222 . with 1 0< <, p if0< <1 and 12 0<<11 , pp if1<2. Then the Bieberbach polyno- mial ,np Bz satisfies ,26 log nCG Bcnn for each with 1 0<<min,2 . 22 Remark 1.1. 1) Theorems 1.2-1.2, extend the cor- responding results in [3-5, 10,12,13] to case 2p . 2) Corollary 1.2 is extending the one result [10] to domains bounded by a piecewise smooth curve with interior zero angles and in =0 coincides with the corresponding result of this work. 2. Some Auxiliary Results The notation “”, we mean that 1 for a constant , which doesn’t depend on and b. The relation “” indicates that c where are independent of and ab b acb a ,bacb 1 c a23 23 ,cc a.b Let be finite domain bounded by Jordan curve and let G L =wz () be the con- formal mapping of ˆ =w z := extG G onto ˆ=:>1 ww :<1,ww normalized by =, >0 ( 00 ˆˆ =0, >0 ). The level curve (exterior or interior) can be defined for as >0t 1 ˆ :=:=,if<1;=,if> 1,. t Lzzttztt L L Let us denote t and := , tt GintL := t extL ,:=inf :dzLz L . Let be a L- quasiconformal curve and .D Then the region can be chosen to be the region 0 D 0 \, r G ,, G ˆ for a certain number 0 1< depending on 2R and 1 0 R 0 =r . In this case, it is known that the function 1 1 .= .ff 2 is a - quasi- conformal reflection across as shown in ([18], p. 28) by analogously in ([19], p. 75), that is, is a L . 2- quasiconformal mapping leaving points on fixed and L satisfying the conditions \ R GG G 0 \ r G, 0 \ rR GGG G \ for some 0 1< <,RR 0<<1.rr By using the facts in ([16], p. 97) and ([19], p. 76, [20], p. 26) we can find a -CKquasiconformal reflection . across such fies the following L that it satis Copyright © 2011 SciRes. APM  C. KOŞAR ET AL. 308 111 22 1 ,,<<,zzzzzLz 1 1, << , 1 ,<, ,>, z z z zz z zz z (2.1) and Jacobian 2 2 =z J of . satisfied by means of thef a qu D Lemma 2.1. ([18]) Let be a 1.J Therefore, exn theorem otensio asiconformal mapping, without loss of generality we may assume that =, .zzz L- quasiconformal curve; 123 111 0 ;,:, , R LzzG zzzcdzL z ˆ = j wz 231210 ,: ,, = =1,2,3. jj orzzGzzzc dzLwz j Then, ents 1) The statem12 13 zz zz and 12 13 w ww are are w equivalent. So 12 13 zz zz and 1213 ww ww 2) If 12 13 zz,zz then 22 1313 13 1212 12 K wwzz ww wwzz ww and, consequently, for any 33 00 R zLzL 2 121 K ww z 2 212, K z ww where and are fixed cotants. al cu 0 1<< 2R ma 2.2. ([2 1 00 =R t :=L ns Lem 1]) LeG be a quasiconform rve. Then, For every zL 0 there exists an arc 0,z in G joining to z with following p: 1) roperties ,dL z for every If 0,z 2) 12 , is joining the subarc of ,z 0 1 to 2, then 12 1 ,es 2 m very pair 2 0 ,,.z ([7] a -Kqua- for e ) Let be si e aritrary Jan domain and le arc except Lemma 2.3. 1 :=LG mes conformal curve. Then mes , fvery rectifiable arc .G Let G bbord or e 0 :=,, , zL an rectifiab 0L which satisfies the following conditions: 1) mes 0 z for one of its endpoints of its z 12 , 1 2 , for all 12 ,. ng function 2) There exists a monotone increasi t suhat ch t 0 ,dLg z for all . Lemma 2.4. ([22]) Let ;GC for some 0< <2, 0 and ofunc- tion n the arc a measurable f n such that b e give 1 2 0 zf for all .en for all z . Th 21 12 2 21 22 ln1<<2, d2 2<2 1. p AG p p f zp p . Approximation in the 3 - 1 p GNorm Suppose that ;GC f0<<2or some and 0 =z is giveof simput n. For the sake , we assume licity, but witho =1,z loss of generality that =2,m 1 21; 1,1 G and let the local coordinate axes be parallel to OX and OY in the coordinate system; ,z 2:=:,0 .LzzLImz Then 0 z is taken a arbitrary point on 2 L (or on 1 L We recall that the domain 1:=Lz subject t : o , 0 LImz s an hosen directio the cn). ;GC has exteri r π o 0< <2 non zero angle and 1 d of the po type interior zero angle in the neighborhooints 1=1z 2ectively. We can say that the function ˆ =wz domain and =1,z resp for the ;C G satisfies the escribed in conditions d int 1 z Lemma 2 in the neighborhood of po1= . So, we can e Lemma 2 asily get from 1 ˆ 1,z (3.1) for all 22 1 ˆˆ ,1; 1dzL zz 1 :=:1 >.zM zGz On the other hand, using properties of the function z ˆ =w in the neigbourhood of the point 1=1z (see, [7,23]) we obtain 1 ˆˆ 1ln 1zz (3.2) Because each is a ,=1,2 j Lj 1- i quasi- . j mu conformal arc, st be re the quasiconformal flection across . Lsider the Let us con curve 1 112 11 2 :=: =1; 3 cc zxiyyx = 1 212 12 2 :==: =1; 3 cc zxiyy x 1 23 :==:=1 ;zxiyycx 2 24 :==:=1;zxiyycx Copyright © 2011 SciRes. APM  C. KOŞAR ET AL.309 for some where a constnts and from mma 2.2 that 43 <<<,cc Definition 1.3. It is easy to check from Le a1 c 2 c 121 2 ,, j mes i fo Let fficiently large ntural number. For arbitrary r all 12 ,, ,=1,2. i jij =NNR su 0 >nN and a 0< <1, let us choose 1 cn 0 =Rr such =1, 2 such that and points 00 <<rRR , , jjthat they are in the intersection of i zi L and i ts divide These poin Lparts: 11312 21 11 :=,, :=,, RR RR LzzLLzz into four 22 421 12 22 := , :=, RR RR LL z LLzz 1 2 , L z are traversed in the positive direction (counterclockwise). 4132 := RRR L LLLL R and are subarcs of i jR i joining points −1,1 with i z. Lenote t us de 1112 22 1221 := , RR R RL RRLR := Uint and :=\. R UUG We can extend the function to the following way z Uin 2 ,, ():= zzG zr (3.3) 0,, =1,2. R i zUi z Then, , 0, , =,,= ziR iz zG zzzzUi 1,2. (3.4) From the Cauchy-Pompeiu formula ([16], p. 148), we get 11 =d d,. 2ππ zzG iz z U RR Then, using the above notations we obtain 2 ,=1 1 =d 2πLR iz 12 3 4 or, =1 , 1 . R R R LL fL L Since the first part of right hand side in (3.5) is analytic function in G, there exists a polynomial 1, n Pz where 1 deg 1 n Pzn ([24], p. 142), such that 1 2 1d< 2πLR n fc Pz in z . (3.6) Let Then, and from (3 1 0 :=( )d . z nn QzPtt 0=0 n Q .5) and (3.6) we have 1 1 d 2π 1 d, π iR j j ij UR f z iz z (3.5) where 2 2 ,=1 2 1 2π 1 d π nij iR j UR c znz z 1 d j Q z an us take integrals ovef the each de we obtain d letr G opower of si -pth 2 2 ,1 2 d 1 1dd dd. ij p nz G p j pij GR p z nz z GU R zQz (3.7) n-Zygmund inequality ([19], p. 98), we obtain From the Caldero 2ddd, =1, UR p p zi GU R i z 2. So, (3.7) and (3.8) give us (3.8) 2 2 ,=1 () 1 1d d. p j p np AG pij p i UR Qnz (3.9) iR jAG p Copyright © 2011 SciRes. APM 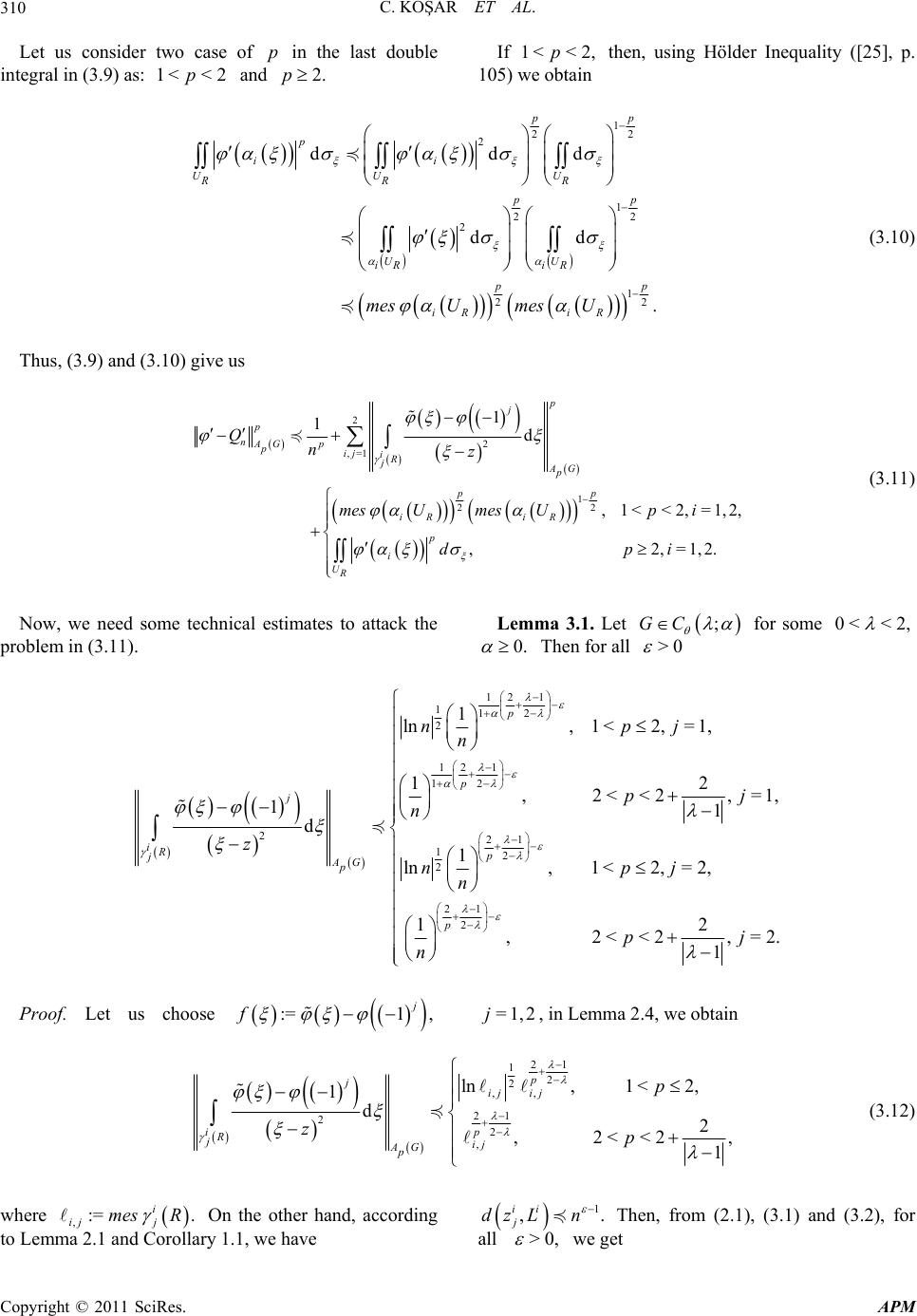 C. KOŞAR ET AL. Copyright © 2011 SciRes. APM 310 Let us consider two case of in the last double integral in (3.9) as: an If then, using Hölder Inequality ([25 10 p d p1< <2p2. 1< <2,p ], p. 5) we obtain 1 22 2d p 1 22 2 1 22 dd dd . p p ii UUU RRR pp UU iR iR p iR iR mesUmesU (3.10) Thus, (3.9) and (3.10) give us 2 2 ,=1 1 22 1 1d ,1<<2, =1,2, ,2 p j p np AG pijiR jAG p pp iR iR p i UR Qnz mesUmesUp i dp (3.11) , =1,2. i Now, we need some technical estimates to attack the problem in (3.11). Lemma 3.1. Let ;GC for some 0< <2, 0. Then for all >0 12 1 112 2 12 1 12 221 21 2 1 ln,1<2,=1, 12 , 2<<2,=1, 11 d 12,=2, 1, p p j p p npj n pj n j n n 1 2 ln iR jAG p zn 2, 1<p 2 2<<2,=2. 1 pj Proof. Let us choose :=1 , j f =1,2j, in Lemma 2.4, we obtain 21 1 2 2 ,, 21 2 2 , ln,1 <2, 1 d2 , 2<<2, 1 p jij ij p iR jij AG p p zp (3.12) here On the other hand, according 1, we have 1 ,. ii j dz Ln all > ,:= . i ij j mes R w to Then, from (2.1), (3.1) and (3.2), for Lemma 2.1 and Corollary 1.0, we get  C. KOŞAR ET AL.311 1 1 1 1 ,1 1,=1 ,, =1 1. 1 ,, =2,= ii j ij ij jii j j dzL jn zdz Ljj n 2 (3.13) Then, the following inequalities are obtained from (3.12) and (3.13) 12 1 112 2 2 ln << 2, 1d np z 11 12 1 1,1 12 ,2 <2. 1 p iRAG p n p n 2 p (3.14) and 2 221 2 1 iRp z 21 12 21 ln,1 <<2, 1d 2 ,2 <2. 1 p AG p np n p n (3.15) Combining (3.14) and (3.15) the proof is completed. □ Now, for sufficiently small 01 0<< , 12 33 VV where we are going to use following notations: 0 1212 22 := R UVVVV j 11 1:= jR V 0 1,:>0, =1,2UDzImzj 2 0 :=1,:<0, =1,2 j jR VUDzImzj 1 30 :=:>0\1,1,, R VUzImz DD 2 30 :=:< 0\1,1,. R VUzImzDD 0 Lemma 3.2. ([26], p. 10) Let ;GC for some 0< <2, 0. ll >0 Then for a 11 13 11 , i ii mes V , i mes V nn and 2 2 1, =1,2 i i mesVi n where 1 := min,. 22 Lemma 3.3. Let ;GC for some 0< <2 , 0 . Then for all >0 min 2 1= R m Ui ,1 , 1, 2. i es n Proof. We have 3 i Now, let us estimate 2 12 =1 = ii iR i i UmesVmes VV 1 i i s V by choosing me =1, >0K and from Lemma 2.1 we have 1 1 2 1 31 1 ,. iii i mesVdzLn 1 1 2 1 11 1 , iii i mesVdzLn On the other hand if we use but except the conformal mapping the method of Gaier in [9] in method, then we obtain min 2,1 2 1. i mes Vn After then using the second and third inequality in the we obtain the desireroof. □ Lemma 3.4. Let first oned p ;GC for some 0< <2 , 0 . Then for all >0 12 1 1 d, UR p p ii n =1,2 where 1 2<2 1 p =min ,2. and Proof. Let ,zG z the point of nearest to :=LG .z Then we have 11 ,zzzz zz and from ([18], Lemma 3) Copyright © 2011 SciRes. APM  C. KOŞAR ET AL. 312 1 00 ,1. oo wwww dwL wrwrwrwrw After this estimation, we get 12 1 21 121 00 1 () 1 d, UU RiR i' U iR p pp w rw n 2 d p w dd pp wrr U iR wh ere :zzL := minr and iR 1 <2 . 1 2p □ By using Lemma 3.1, 3.2, 3.3, 3.4 and (3.11) we get the following results. We need this notation: 12 1 112 2 2 2 1 11 22 2 1 3 1 ln,max,,=1, 2,3. 11 , <,, 0 <. 2 ,:= 11 , <,, <. 23 12 , <,, <<2. 3 p i p n p p np n p n p p n p n 2 i Lemma 3.5. Let and assume that 1< 2p ;GC for some 0<<2 , 0. Then, for and arbitrary smany 3nall >0 1 ,,. np n AG p Bp (3.16) Lemma 3.6. Let 2 2< <21 p and assume that ;GC 1 1 ,12 1 1,0<<1, . 11<2. p np p AG pp n B n (3.17) Proof. The proof of Lemma 3.5-3.6 is similar. So, we give them together. From (3.11) and Lemma 3.1-3.4 we obtain for some 0<<2 , 0. Then, for arbitrary smany 3n andall >0 12 1 112 2 12 1 12 21 12 2 ln n 21 2 1 ln1 <<2,= 1, 1 12 2<2 ,=1, 1 11 <<2,=2, 1 p nAG pp p p npj n Qnpj n pj n n 2 2 1< 2, 211 2<2 ,=2,,2 <2, 11 p p p p pj p n 12 1 ,1 < p n (3.18) Copyright © 2011 SciRes. APM  C. KOŞAR ET AL.313 where 1 := min, 22 , :=min2;1,:=min1, 2 Case 1. Let In this case from (3.18) we get 1< <2.p 2 12 1 112 2 211 ln ,. p pp nAG p n Qn nn p Case 2. Let 21 2<min2 ;2,:=min1,2 11 p 12 1 21 2 11 p pp n Q 1 12 1 1, 0<< 1, 1,1 <2. AG p p p p nn n n Now, let us consider the polynomial . In this case from (3.18) we have 00 := 1. nn n Qz QzQz nn Q It is clear that 00 =0, =1 nn Qz Qz . So, we have 1 1 12 1 1, 1 <<2, 12 , 0<<1,2<2, 1 12 ,1<2,2<2 . 1 p nAG p p p p n Qp n p n So, if we consider the extremal property of the polynomials then we obtain (3.16) and (3.1) respectively. To prove Theorem 1.2-1.6 and Corollary 1.7, we use a sio the one of Andrievskii and Simonenko employed in the proofs of analogous theorems for (see [6,8,10]). simply connected 4.1) in (3.19) to prove Theorems 1.2-1.6. 4. References [1] P. J. Davis, “Interpolation and Approximation,” Blaisdell [2] M. Kucukaslan and F. G. Abdullayeemal Polynomials and Its Approximations Properties,” Novisad Journal of Mathematics, Vol. 39, No2. [3] M. V. Keldysh, “Sur L’approximation en Moyenne Quadratique des Fontions Analtiques,” Matematicheskii Sbornik, Vol. 5, No. 2, 1939, pp. 391-401. [4] P. K. Suetin, “Polynomials Orthogonal over a Region and Bieberbach Polynomial,” American Mathematical Soci- ety, Rohde Island, 1974. [5] S. N. Mergelyan, “Certain Questionsof the Constructive Theory of Functions,” Trudy Matematicheskogo Instituta im. V.A. Steklova R AN, Vol. 37, 1951, pp. 3-91. [6] I. B. Simonenko, “On the Convergence of Bieberbach Polynomials in the Case of Lipschitz Domain,” Mathe- matics of the USSR-Izvestiya, Vol. 13, No. 1, 1979, pp. ,, np n B □ 7 milar method t =2p Lemma 3.7. [2] Let Gbe a domain so that 1 ,np AG p Bn (0,1), =2,3,,n for each and 1 () () 1,> 2, log,= 2, ,0<<2,> nn CGA G p p PP np np 0 r all polynomials of degfo Pz nn Pn and normalized with 0=0. n P Then, ,() np CG .Bn (3.19) It is enough replacing by in (3.16) and (3.17) rstively and e pec 2 =22p p in ([22], Corollary Pub. Company, New York, 1963. v, “New Extr . 2, 2009, pp. 1-1 166-174. doi:10.1070/IM1979v013n01ABEH002017 [7] V. V. Andrievskii, “On the Uniform Convergence of Bieberbach Polynomials in Domains with Piecewise Quasiconformal Boundary,” In: Mappings Theory and Approximation of Function, Naukova Dumka Kiev, 1983, pp. 3-18. ence of Bieberbach Polyno- mials in Domains with Quasiconformal Boundary,” [8] V. V. Andrievskii, “Converg Copyright © 2011 SciRes. APM  C. KOŞAR ET AL. 314 Ukrainian Mathematical Journal, Vol. 35, No. 3, 1983, pp. 233-236. doi:10.1007/BF01092167 [9] D. Gaier, “Polynoimal Approximation of Conformal Maps,” Constructive Approximation, Vol. 14, 1994, pp. 27-40. doi:10.1007/s003659900061 vergen egions with” , 1998, pp doi:10.1007/BF02075463 [10] D. Gaier, “On the Conce of the Bieberbach Poly- nomials in R Corners, Constructive Ap- proximation, Vol. 4. 289-305. [11] F. G. Abdullayev, “On the Convergence of B ins wi Int ev, “Uniform Convergenc ure of Domain in , 2001, pp. 77-101. yev and A. Baki, “On the Convergence of ieberbach Polynomials in Domatherior Zero Angles,” Dokladi Akademii Nauk Ukraine SSR Seria A, Vol. 12, 1989, pp. 3-5. [12] F. G. Abdullaye of the Bieber- bach Polynomials Inside and on the Clos the Complex Plane,” East Journal on Approximations Vol. 7, No. 1, 13] F. G. Abdulla[ Bieberbach Polynomials in Domains with interior zero angles,” Complex Variables: Theory and Applications, Vol. 34, No. 2, 2001, pp. 131-143. [14] D. Israfilov, “Approximation by p-Faber Polynomials in the Weighted Simirnov Class Ep(G,w) and the Bieberbach Polynomials,” Constructive Approximation, Vol. 17, No. 3, 2001, pp. 335-351. doi:10.1007/s003650010030 [15] D. Israfilov, “Uniform Convergence of the Bieberbach Polynomials in Closed Smooth Domains of Bounded Boundary Rotation,” Journal of Approximation Theory, Vol. 125, No. 1, 2003, pp. 116-130. doi:10.1016/j.jat.2003.09.008 al A siconformal Mappings,” ration Pub., Atlanta, , 1997, , Vol. 51, No. 3, /A:1013796308878 [16] O. Lehto and K. I. Virtanen, “Quasiconformal Mapping in the Plane,” Springer Verlag, Berlin, 1973. [17] S. Rickman, “Characterization of Quasiconformrcs,” Annales Academiae Scientiarum Fennicae Mathematica, 1996. [18] F. G. Abdullayev, “On the Orthogonal Polynomials in Domains with Quasiconformal Boundary,” Dissertation, Donetsk (in Russian), 1986. [19] L. V. Ahlfors, “Lectures on Qua Van Nostrand, Princeton, 1996. [20] V. V. Andrievskii, V. I. Belyi and V. K. Dzjadyk, “Con- formal Invariants in Constructive Theory of Functions of Complex Variable,” World Fede Georgia, 1995. [21] F. G. Abdullayev, “Uniform Convergence of Generalized Bieberbach Polynomials in Regions with non-zero an- gles,” Acta Mathematica Hungarica, Vol. 77, No. 3 pp. 223-246. [22] F. G. Abdullayev, “Uniform Convergence of Generalized Bieberbach Polynomials in Regions with Zero Angles,” Czechoslovak Mathematical Journal 2001, pp. 643-660. doi:10.1023 ormal Map- imirnov and N. A. Lebedev, “Functions of Com- and Approximation by Ra- and, 1960. of NAS of Azerbaijan, Vol. 14, No. 22, [23] V. I. Belyi and I. E. Pritsker, “On the Curved Wedge Condition and the Continuity Moduli of Conf ping,” Ukrainian Mathematical Journal, Vol. 45, No. 6, 1993, pp. 837-844. [24] V. I. S plex Variable Constructive Theory,” MIT Press, Cam- bridge, 1968. [25] J. L. Walsh, “Interpolation tional Functions in the Complex Domain,” American Mathematical Society, Rohde Isl [26] F. G. Abdullayev and M. Kucukaslan, “On the Conver- gence of the Fourier Series of Orthonormal Polynmials in the Domain with Piecewise Smooth Boundary,” Pro- ceeding of IMM 2001, pp. 3-13. Copyright © 2011 SciRes. APM
|