Theoretical Economics Letters, 2011, 1, 111-113
doi:10.4236/tel.[phone] Published Online November 2011 (http://www.SciRP.org/journal/tel)
Are Sunspots Stabilizing?
Paul Shea
University of K e nt ucky, Lexington, USA
Received August 18, 2011; revised October 4, 2011; accepted October 12, 2011
Abstract
The reduced form solutions of indeterminate rational expectations models often include extraneous expecta-
tional errors or “sunspots”. Sunspots are usually modeled as independent of the model’s fundamentals, and
are often presumed to result in excess volatility. An alternate approach, however, is to assume that sunspots
include both an overreaction or underreaction to fundamentals, as well as genuine extraneous noise. This
paper uses a simple linear model to formally show how the relationship between sunspots and fundamentals
affects aggregate volatility. Sunspots reduce volatility if 1) they include an undereaction to fundamentals, 2)
the variance of genuine extraneous noise is sufficiently small, and 3) the root that causes indeterminacy is
sufficiently far from one.
Keywords: Sunspots, Indeterminacy, Volatility,
1. Introduction
It is well known that linear rational expectations models
may be indeterminate, implying that a continuum of
equilibria paths exist. Often these equilibria depend on
extraneous expectational errors, known as sunspots. In
their seminal paper examining the general equilibrium
effects of sunspots, Cass and Shell (1983) [1] define a
sunspot as “represent[ing] extrinsic uncertainty, that is,
random phenomena that do no t affect tastes, endowments,
or production possibilities.” Although it is debatable
whether an overreaction or underreaction to fundamen-
tals fits with this definition, it is clear that a sunspot that
includes such a reaction is consistent with a rational ex-
pectations equilibrium under indeterminacy.1 The most
common approach for modeling sunspots is to assume
that they are independent of fundamentals, and to treat
the variance of sunspots as a parameter to be calibrated.2
This is equivalent to assuming that sunspots include an
underreaction to fundamentals.
Benhabib and Farmer (1999) [4] write that sunspots
are of interest because they both add an additional source
of volatility, and because they allow for richer propaga-
tion dynamics. It follows from the former property that
sunspots are typically destab ilizing. In the Real Business
Cycle (RBC) literature, sunspots are therefore often
viewed as a mechanism for reducing the volatility of
productivity shocks needed to match the volatility of
output, and thus reducing the probability of productivity
regressing. Most notably, Farmer and Guo (1994) [5]
show that a RBC model that includes only sunspot
shocks does arguably as well at matching the data as a
standard RBC which includes only fundamentals.3 Like-
wise, a major literature in monetary economics views
ensuring a unique equilibrium, and thus avoiding excess
volatility, as a primary goal of monetary policy.4
This paper uses a simple linear model to show that
sunspots may either reduce or increase volatility relative
to the model’s minimum state variable (MSV) solution. I
decompose sunspots into a linear combination that in-
cludes an overreaction or underreaction to fundamentals,
as well as genuinely extraneous noise. I prove that sun-
spots reduce volatility if: 1) they include a small enough
underreaction to fundamentals, 2) the ratio of the vari-
ance of genuine noise to the variance of fundamental
shocks is sufficiently small, and 3) the root responsible
for indeterminacy is sufficiently far from one.
1Throughout the paper, overreactions and underreactions are defined as
relative to the response to fundamentals that occurs in the model’s
minimum state variable (MSV) solution. McCallum (1983) [2] pro-
p
oses the minimum state variable solution as a selection criteria for
models with multiple equilibria. The MSV solution, by definition the
most parsimonious, does not depend on extraneous noise.
2See Farmer (1999) [3] for a te xtbook treatment of indeterminacy.
3Other RBC models that include sunspots in equilibrium include Ben-
habib and Farmer (1996) [6], Schmitt-Grohe (1997) [7], Schmitt-Grohe
and Uribe (2000) [8], and Shea (2011) [9].
4See Woodford (2003) [10] for an overview of this literature.

P. SHEA
112
2. Model
The precise conditions for sunspo ts to be stabilizing are a
function of the specific model being analyzed. I thus
focus on a simple, univariate linear model to make the
paper’s main point. The main result, however, easily ex-
tends to more complex linear models.
A variable, t, depends on its one-period ahead ex-
pectation as well as a fundamental shock, :
yet


1 e
ttt
yEy  
t
(1)


lim
tt
jEyj 0

  (2)
where tis iid, and mean-zero. If e< 1  , then equilib-
rium is unique and follows:
= e t
yt (3)
If < 1 
, however, then equilibrium is indeterminate
and may be represented as:

111ttt
yye
t




 (4)
where


1tttt
y
Ey 

 is agents’ expectational error.
Equations (1) and (2) hold as long as t

is an iid
mean-zero process. The literature defines t

as a sunspot.
The sunspot may be decomposed into two elements:
ttt
ev

e
v
. t is an overreaction or underreaction to
fundamentals and t is the part of the sunspot that de-
pends on truly extraneous noise. Iterating Equation (4)
backwards yields the following representation of the so-
lution:

ii
i
i1 i0
1
ttt t
yeev 






 i 

(5)
Two special cases merit discussion. First, if ,
then sunspots are entirely genuine extraneous noise that is
unrelated to fundamentals. This is the most common ap-
proach to modeling sunspots.5 Notably, setting
does not eliminate the effects of indeterminacy under this
approach. Instead, this parameterization results in self-
fulfilling perfect foresight where contemporaneous sto-
chastic shocks have no effect in equilibrium. Second,
and results in the MSV solution, iden-
tical to the unique equilibrium under determinacy. For
the remainder of the paper, I define as an
overreaction (relative to the MSV solution) to funda-
mentals, and as an underreaction.
= 0
2= 0
v

1
= 12
= 0
v

< 1
>
Setting has a simple appeal. Under indeter-
minacy, any martingale difference sequence may repre-
sent agents’ self-fulfilling beliefs and is thus consistent
with rational expectations. The fundamental shock is
thus just one of an infinite number of candidates and is
accorded no special consideration under this approach.
= 0
2
v

is treated as a parameter to be calibrated, possibly
by choosing the value that best fits the data as in Farmer
and Guo (1994) [5].
There are, however, two compelling justifications for
assuming that 0

. First, in modern macroeco-
nomics, forward-looking equations such as Equation (1)
usually result from agents solving an optimization prob-
lem. Agents are typically presumed to understand that,
for any


1tt
Ey

et
, they must respond to a one unit in-
crease in by also increasing t by one unit in order
to be optimizing. The fundamental shock is the unique
stochastic process that appears in the structural model
and it is therefore unlikely that its contemporaneous value
does not appear in the solution. For it not to appear, it
must be the case that the direct effect of in Equation
(4) is perfectly offset by its effect on agents’ self-fulfill-
ing beliefs. There typically is no apparent reason for this
knife edge case to occur.
y
et
To demonstrate the second justification for 0

,
consider the following example. t is output in a neo-
classical model where money does not ordinarily matter.
is a fundamental shock to productivity. Although, in
principle, t
y
et

may be either a popular macroeconomic
variable such as a monetary aggregate, or the population
of penguins in Antarctica, it is far more likely that agents
will coordinate their beliefs on the former than the latter.
Suppose that agents, persuaded by decades of monetary
economics, set t

as a monetary aggregate. The monetary
aggregate may itself be a function o f productivity. thus
represents the response of the monetary authority to
productivity while t is the part of the monetar y aggregate
that is independent of the fundamental.

v
Lubik and Schorfheide (2004) [11] provide an exam-
ple of setting 0


2
v
. In that paper, the authors en-
dogenize and


so that the solution under inde-
terminacy and the MSV solution are identical at the
boundary between the determinate and indeterminate
regions. Away from the boundary, however, and

2
v

are treated as parameters to estimate. This ensures
that small changes to the model’s parameters which
switch the solution from indeterminate to determinate, or
vice-versa, do not have large effects on the model’s equi-
librium behavior. It also aids the authors with their main
purpose of empirically estimating a New Keynesian
model where part of the parameter space yields indeter-
minacy.
3. Results and Analysis
It is straightforward to calculate the volatility of
under determinacy and indeterminacy: t
y
5This is also the approach taken by Farmer’s (1999) [3] textbook on
indeterminacy.

det 2
t
Var yv

 (6)

P. SHEA
113


2
det 22
e
22
11
11
in
t
Var y2
v






 





 (7) mon approach to modeling sunspots (), this may
or may not be the case. Another special case is to assume
that sunspots include the same response to fundamentals
as the MSV solution (). In this case, for any
, sunspots only add noise to the system and are
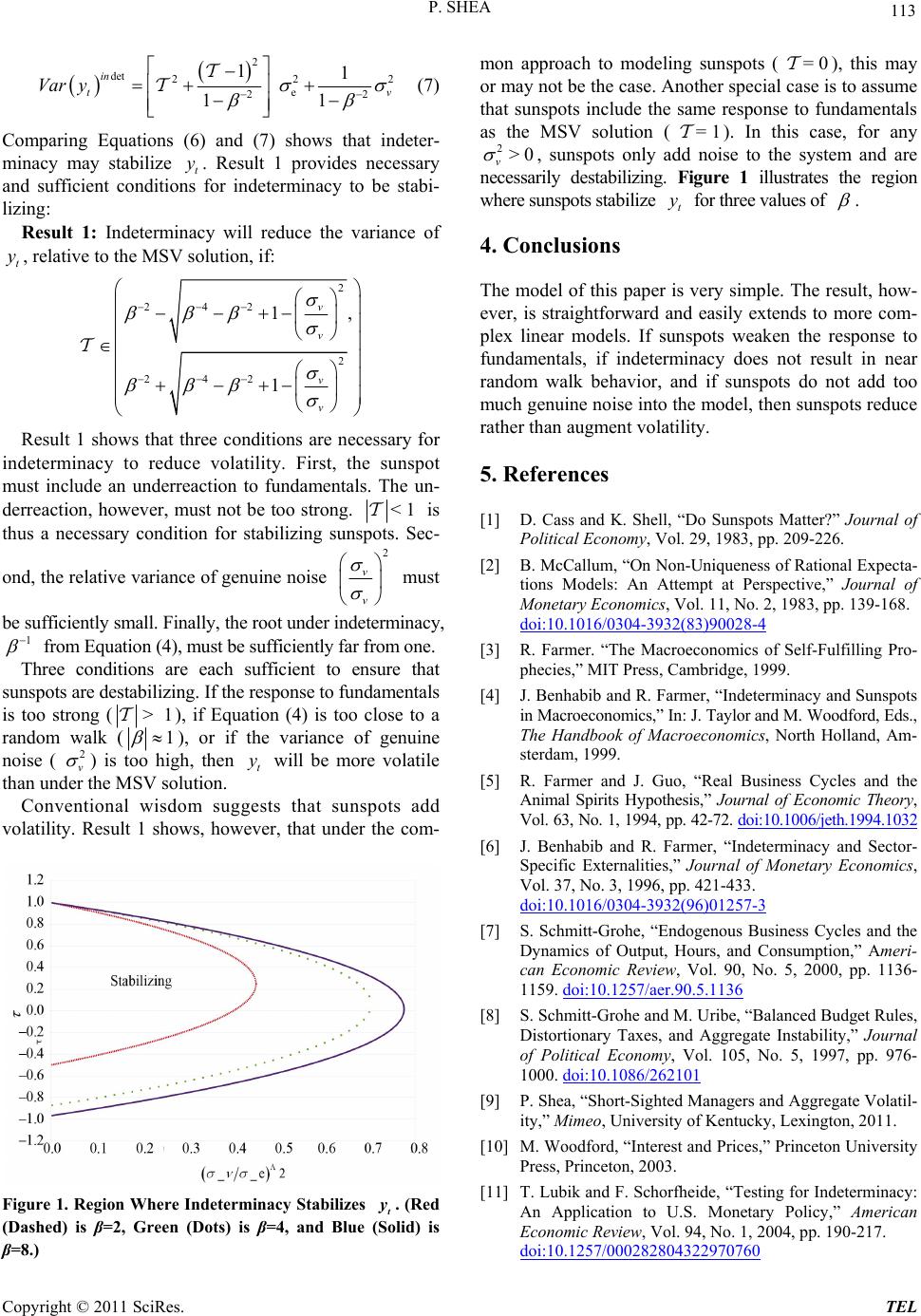
necessarily destabilizing. Figure 1 illustrates the region
where sunspots stabilize for three values of
= 0
= 1
t
y
2>0
v


.
Comparing Equations (6) and (7) shows that indeter-
minacy may stabilize t. Result 1 provides necessary
and sufficient conditions for indeterminacy to be stabi-
lizing:
y
Result 1: Indeterminacy will reduce the variance of
, relative to the MSV solution, if:
t
y4. Conclusions
2
242
2
242
1,
1
v
v
v
v



 



























The model of this paper is very simple. The result, how-
ever, is straightforward and easily extends to more com-
plex linear models. If sunspots weaken the response to
fundamentals, if indeterminacy does not result in near
random walk behavior, and if sunspots do not add too
much genuine noise into the model, then sunspots reduce
rather than augment v olatility.
Result 1 shows that three conditions are necessary for
indeterminacy to reduce volatility. First, the sunspot
must include an underreaction to fundamentals. The un-
derreaction, however, must not be too strong. < 1 is
thus a necessary condition for stabilizing sunspots. Sec-
5. References
[1] D. Cass and K. Shell, “Do Sunspots Matter?” Journal of
Political Economy, Vol. 29, 1983, pp. 209-226.
ond, the relative variance of genuine noise
2
v
v





must [2] B. McCallum, “On Non-Uniqueness of Rational Expecta-
tions Models: An Attempt at Perspective,” Journal of
Monetary Economics, Vol. 11, No. 2, 1983, pp. 139-168.
doi:10.1016/0304-3932(83)90028-4
be sufficiently small. Fi nally, the root under indeterminacy,
1

 from Equation (4), m ust be sufficiently far from one. [3] R. Farmer. “The Macroeconomics of Self-Fulfilling Pro-
phecies,” MIT Press, Cambridge, 1999.
Three conditions are each sufficient to ensure that
sunspots are destabilizing. If the response to fundamentals
is too strong (> 1), if Equation (4) is too close to a
random walk (1  ), or if the variance of genuine
noise (2 v

) is too high, then will be more volatile
than under the MSV solution. t
y
[4] J. Benhabib and R. Farmer, “Indeterminacy and Sunspots
in Macroeconomics,” In: J. Taylor and M. Wo odf ord, Eds.,
The Handbook of Macroeconomics, North Holland, Am-
sterdam, 1999.
[5] R. Farmer and J. Guo, “Real Business Cycles and the
Animal Spirits Hypothesis,” Journal of Economic Theory,
Vol. 63, No. 1, 1994, pp. 42-72. doi:10.1006/jeth.1994.1032
Conventional wisdom suggests that sunspots add
volatility. Result 1 shows, however, that under the com- [6] J. Benhabib and R. Farmer, “Indeterminacy and Sector-
Specific Externalities,” Journal of Monetary Economics,
Vol. 37, No. 3, 1996, pp. 421-433.
doi:10.1016/0304-3932(96)01257-3
[7] S. Schmitt-Grohe, “Endogenous Business Cycles and the
Dynamics of Output, Hours, and Consumption,” Ameri-
can Economic Review, Vol. 90, No. 5, 2000, pp. 1136-
1159. doi:10.1257/aer.90.5.1136
[8] S. Schmitt-Grohe and M. Uribe, “Balanced Budget Rules,
Distortionary Taxes, and Aggregate Instability,” Journal
of Political Economy, Vol. 105, No. 5, 1997, pp. 976-
1000. doi:10.1086/262101
[9] P. Shea, “Short-Sighted Managers and Aggregate Volatil-
ity,” Mimeo, University of Kentucky, Lexington, 2011.
[10] M. Woodford, “Interest and Prices,” Princeton University
Press, Princeton, 2003.
[11] T. Lubik and F. Schorfheide, “Testing for Indeterminacy:
An Application to U.S. Monetary Policy,” American
Economic Review, Vol. 94, No. 1, 2004, pp. 190-217.
doi:10.1257/000282804322970760
Figure 1. Region Where Indeterminacy Stabilizes t
y
. (Red
(Dashed) is β=2, Green (Dots) is β=4, and Blue (Solid) is
β=8.)