Open Access Library Journal
Vol.05 No.04(2018), Article ID:83739,50 pages
10.4236/oalib.1103797
The Relativistic Giant Atom: Evidences and Recommendations
Emad Eldieb
Forensic Sciences Organization, Cairo, Egypt

Copyright © 2018 by author and Open Access Library Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: July 2, 2017; Accepted: April 10, 2018; Published: April 13, 2018
ABSTRACT
In 2017 we published a new theory under the title: physics of the relativistic giant atom [1] , where we suggested an alternative solution for the theory of special relativity. Some points were ambigious. Here in this present article we rewrite the same paper with clarification of the ambigios points, and with adding new ideas. If the original relativity says: the relativistic mass m(v) of a particle increases and its relativistic length decreases with a defined γ factor hence, the hidden meaning of this original solution is appearance of the relativistic linear mass density in the form of: . This rearrangement may allow―under defined conditions―an alternative relativistic solution in the form of creation of new particles counting , each with the same original rest mass mo and the same original rest radius ro. This study searches in the conditions required for this alternative solution as follow: if we wrote the famous Einstein’s equation of the rest mass of a particle (let it, a proton) as: then this form describes the particle electromagnetic energy in a rest state, where the proton doesn’t feel―from interior― its charge and consequently this equation cannot describe repulsive electric energy. If we multiplied the left side of this equation by the number one then the result should be the same right side, and the equation still describes the proton in its rest state. Now if we defined a sphere s, with a quantity of protons p, distributed homogeneously among much more number of neutrons n, and if we have a quantity equal the number one as: (where; is the spacing between particles).
(where rs is the radius of the sphere while c is the speed of the electromagnetic energy and not real particle speed). This nonrelativistic equation is still carrying the same meaning, and it is―still―describing the rest mass equivalent energy of the same particle with the same rest state defined by the new spacing rc (in addition to its inertial description defined by its rest radius ro). The hidden meaning here is condensation of the particles in this new rest state. This last equation realizes two results: first, it describes the particle in its rest state, so the sphere can absorb physically all the repulsive & exclusive energies, and second, it realizes the initial conditions: & (where the Coulomb’s equation form is now fixed on the Einstein’s form) hence, we may conclude an alternative relativistic solution in the form of: This idea led me to put a base for physics of self-replication as application of the relativistic giant charge which is ultra-cold fermions condensate. This state has the relativistic efficiency to make the particles replicate and withdraw all the kinetic energy. When we followed up physics of the giant atom we found complete coincidence between the estimated parameters of its orbit and the astronomic parameters of the solar system. This means that the solar system―in one of its primordial evolutionary stages―was a giant atom like system, and in the same time gives documents for the correctness of physics of the giant atom.
Subject Areas:
Quantum Mechanics
Keywords:
Quantum Physics, Relativistic Mass, Microscopic State, Ultra-Cold Neutrons, Solar System

1. Introduction
In this paper we put the relativistic basis for the giant atom like system [1] . Before we go with the discussion we have to stress on that it may appear in the beginning of this long study that there was contradiction with the current knowledge like the constant speed of light, Pauli’s exclusion principle, and like quantum physics, but as soon as possible we would find that the study completely respects such facts. Also we have to remember all over this study that the final macroscopic state of the microscopic events inside the giant charge is the absolute zero energy except from a bit quantity of energy. We would divide the discussion into two parts: the first would discuss physics of the relativistic giant charge, while the second will discuss the solar system in its primordial evolutionary stage as giant atoms, where we would discover that there is complete coincidence between the estimated parameters of the orbits of the giant atoms and that of the solar system as a document for the past existence of the giant atom system. The first part of this study discusses physics of the giant charge, while the second part discusses physics of this giant atom like system as orbital, and gives evidences for possibility of existence of such system.
Throughout the first part of this study we will find that the relativistic giant charge unit, like the giant proton unit (Compton sphere) having a quantity of elementary particles equal would produce―under a defined physical condition―the alternative relativistic solution in the form of replication by copy and paste to appear as a giant proton (the adult) having quantity of particles equal . The careful mathematic manipulation with relativity would reveal that the relativistic particle mass (inside the giant charge unit) would not increase by its high speed (special relativity will not affect the mass of the elementary particle) but instead, the particles quantity is the one which increases (the substitutive relativistic solution would be in the form of increase the particles count from n into N). This means that the energies of the electric and that of the relativistic gravitational field uG and also of the quantum state would be withdrawn giving finally a macroscopic rest state (the relativistic mass m = the rest mass mo).
Explicitly, the process of self-replication is the relativistic alternative solution. So if you looked for physics of this unit you would convince that the rest state is the macroscopic net state, or you can put the growth action in place of the motion action. This study stands on two physical bases. The first one is to put the coulomb’s form of the electrostatic energy in the form of the famous Einstein’s equation of the rest mass of a particle, and incorporate the reduced Compton’s
wavelength as the spacing between particles where the rest
radius of the particle = ro.
To guarantee that we deal with real matter, and to guarantee that the quantum speed―which is arising from this spacing―is less than the speed of light we mathematically derived this spacing as just a bit above and consequently the quantum speed v just a bit below c. The second idea is to use the effect of the special relativity on the gravitational and electric energy ug & ue and also on the quantum energy uc inside this sphere. To approximate this effect let us imagine a body has constant speed v, and on the same time it moves under the acceleration
, the relativistic gravitational force
while the same body with the same v would hold relativistic electromotive force . Where, the charge e, is invariant. So, although the nonrelativistic Fe is much bigger than the nonrelativistic Fg yet the relativistic FG may get equal to the relativistic electric energy. If such equality happened, suspense would occur.
The study would be clear step by step. The Equations (56)-(68) would clarify the ambiguous points. I advise the reader to record his objections till he reaches to Equation (68). The author stresses on that; we have to be brave and accept the correct mathematic results whatever it may have suspense. The goal is to put the electric repulsive energy―per a proton―inside this sphere, in the Einstein’s rest form. Once this occurs then we get two results. The first is; we have to search for alternative relativistic solution (other than the relativistic mass & length). The second is; we succeeded, macroscopically, to abort―physically―the electric repulsive energy. We―also―can find the equations packet which would abort physically the quantum exclusive energy due to the spacing between the particles.
2. The Compton’s Sphere in a Rest State
From the relativistic relation where , if the speed v of a particle = c then the relativistic mass m would be infinite (refused), and if then, (also is refused).
The Einstein’s famous equation of the rest mass [2] of a subatomic particle like a proton (or neutron…) is defined by
(1)
where, it is positive energy equating the relation between the inertial rest mass mo of the particle and its rest mass equivalent energy Eo (where k stand for the electrostatic constant , e is the value of the elementary charge in coulomb and ro is the classic rest radius of the particle). If we multiplied the left side by the number 1 then the right side should be typically the same. On the same form, if we defined a system (neglect quantum mechanics for a moment) formed of quantity of protons p, distributed homogeneously among
much more number of neutrons n, such that and if we defined the quantity
(2)
where, the relativistic mass here―in this equation―m = the rest mass mo + 0 = mo and consequently (If―and only if―the product inside the big brackets = 1 and consequently, if v = c = speed of the electromagnetic energy e m.e). Let us call this system “Compton’s sphere”. Now let us―also―multiply the electric field contributor of e m.e of the rest particle by the number one so let us define:
(3)
Because of we can define any quantity of p and n so let us―also―define another quantity as:
(4)
Now again, let us―for a moment―neglect the relativistic & quantum effect and let us imagine that we have so big quantity of protons inside this Compton’s sphere that the repulsive electric energy takes the form of:
(5)
We can see this equation as a physical description for the electric potential of the electromagnetic energy of a particle in a rest state (see Appendix) and―also―we can see it as a physical description for the repulsive electric energy for each particle. But we have to notice that: the original relativistic solution describes the kinetic energy while our alternative solution begins from the idea of Equation (2) which describes the particle (definitely, inside our sphere) by non-relativistic mass (see later), so, we can formulate:
The important point―here―is to notice that the number one is not arbitrary mathematic number because it carries a physical meaning, so it could not―here―replaced by any other number. The number one when be multiplied by Equation (1) gives the physical meaning of the energy of a particle in a rest state, and when be multiplied by Equation (3) it gives the physical meaning of the electric potential subdivision of this electromagnetic energy (half of the stored energy) of the particle in the same state. This means that multiplication by other numbers to get the same result is physically wrong. For example, we cannot multiply the left side of the first equation say, by o.1 to get in the other side rest particle with o.1 (mathematics sometimes have to think as a physician). Also we have to notice that the two sides of each of Equation (3) & Equation (5) are synonyms, so to conserve this relation we have to do first; an added deficit quantity in one side have to be compensated by an added equal excess quantity in the same side, and second the same operations have to be hold on the other side of the equation. Also we have to notice that the equations which describe half of the rest particle are physically not independent equations because finally we have to describe a complete particle. So, such equations should be considered as a temporary station just to describe the micro events of the macro-state. Hence, we can do a junction between Einstein’s equation form, which talks about a particle in a “rest state” and coulomb’s equation form which talks finally about “relativistic mass”. Since, this coulomb’s form takes the same form of Einstein’s equation (if we neglected―for a moment―the original relativistic relations), so it is acceptable to carry the two meanings (rest mass & relativistic mass). When the two meanings are present in the same equation, then this may be explained by presence of “alternative” relativistic solution which affects something other than the rest mass. But the thing which acts against this coulomb’s form is that, it carries the meaning of real speed which is―from the side of view of relativity theory―refused. So let us define infinitesimal small quantity with minus sign (deficit) & with positive sign (excess) and let this zero quantity appears in Equation (5) as follow:
(6)
where is the ratio between the two unknowns n and p while μ is another
unknown, all should be defined. Since, we want to study physics of collection of particles in this Compton’s sphere which has the highest possible exclusive (the electric & quantum) acquired energy, then, the particle never get energy more than that defined in Equation (6) and so, this equation could be considered the key for studying this case. But because is forbidden by the special relativity, so we need an infinitesimal cutoff, therefore, Equation (6) appears with the deficit only as:
(7)
This coulomb’s form (kindly, look at the integral form of Equations (41) & (42)) talks about a sphere which neglects each of the relativistic relations & the quantum effect and so it respects only the relativistic rule that
But actually, this acquired speed is a microscopic picture, while the final form of our sphere obeys Equation (3) or Equation (2) so its macroscopic state is the rest state. This means that c2 which appears in (3) does not describe acquired speed but it just to describe the electric potential subdivision of the rest mass. So, is the speed of the electromagnetic energy (not real speed), hence, it is not refused in this sphere (where means particle in a rest state). Therefore, the problem―here―which our system avoids is not but it is (if we neglected, the relativistic relations, hence the distance intervals in the primed and non-primed frames are equal: therefore, the approaching frames can add their velocities). Fortunately, our alternative relativistic solution prevents but by the dynamics of Equation (2) (where the particle appears in the rest state ) or Equation (6) where the particle appears in the rest state .
This problem arises from that; since the gravitational energy (ug) never waste from matter then it would add energy to the energetic function of the system (this ambiguous sentence would be clear in the next sections) which means excess speed over c. Hence, the deficit quantity which appears in the left side of (7) aims to avoid this problem. This is good news, because this means that the deficit in energy equals ug which would help us to define the unknowns. Equation (6) carries the same description of Equation (5) and could be considered its microscopic shape, while (7) was formulated to show and concentrate on this microscopic action. It is clear from the deficit inside Equation (7) that it initiates the relativistic action. But each of the two equations in the above form is still describing the rest mass (no relativistic mass appears) so this form may carry the meaning of the alternative relativistic solution. Since the equation does not speak about external energy (it just describes the potential or its rest energy), so its inertial rest c2 with that cutoff (deficit) appears as real speed. This speed would launch the relativistic action which appears if―and only if―we began from (7). This action―as we will see―would add first; excess quantity equal the deficit, so we go back to Equation (6). And second, it would add the quantity D which is defined by Equation (9) (see next) so we go back to Equation (2) or Equation (1) which is just describing a particle in a rest state which means that the relativistic mass is the same the rest mass. Hence, we are in an urgent need to the alternative relativistic solution. Finally, we would discover that the entire equations of this sphere are not more than description of the energy of a particle in a rest state. Equation (2) could be written mathematically in the form of Equation (6) as:
(8)
Let the second term = D, the excess quantity = b & the deficit = d
(9)
If we delayed the definition of the spacing to a next chapter, then Equation (7) could be considered as a junction between Einstein’s & coulomb’s equation forms, from which we can declare launching physics of the giant atom. In the next chapter we would put in consideration the relativistic effect and then in a following chapter we would consider the quantum & uncertainty effect.
3. The Rest Particle inside a Collection
To conserve the initial conditions (
coulomb’s form on the form; where the initial conditions are
implied in this Einstein’s form. Since, the factor c2 does not imply real speed so this form of equation means abortion of the electric repulsive energy of the coulomb’s form inside the Compton’s sphere. All the micro-events which would appear in this study should be directed physically to realize―finally―the initial condition of the macro-state of Equation (1), that is to say, we will choose―all over the study―the solution which realizes the rest state of the particle inside this sphere. It is the fatal mistake if we forgot this idea. If we want to talk about real speed and to avoid real speed and―also―to initiate the action of physics of the sphere let the speed v of the sphere particles be close to c therefore, let us define where the deficit would appear as:
(10)
This form talks about real speed so the electrostatic energy is so great that the relativistic relations are considerable.
Let us rewrite (6) as:
(11)
And let us in this chapter consider only the contents of the outer brackets of the two sides which are equivalent to (7). The goal here is to see how the relativistic action―due to the deficit―produces the “D” term and also to see the urgent physical need for the alternative relativistic solution. Since the left side of Equation (1) or Equation (3) is a synonym to the right side, so adding a deficit and an excess quantity to the left side should appear―also―in the right side as in Equation (11). Actually (7) is the firing equation of the Compton’s sphere. But since the final macroscopic state would appear in the complete form of Equation (11) or Equation (1) so we will see that the Compton’s sphere is in complete rest state; the deficit quantity was a deficit in the rest mass of the left side of the equation and necessarily, a deficit of the rest energy of the right side, while the compensating excess term is the nonrelativistic gravitational energy which equals the equivalent energy of that deficit. This deficit in the equation is the first block in physics of the giant charge which will enable us to visualize the microstates of this equation. Now let us use the relativistic relations, so let us put
And let,
where; . Let us―also―define another unknown as; ,
(12)
where is constant.
This is the original relativistic solution with the expression of these unknowns. Of course, one of the goals is to derive the value of this speed v (& density of the matter of this sphere) and consequently the values of these unknowns. The physical meaning of the last four equations appears from the next section.
4. Global View
1) Put the free particle described by Equation (1) inside the collection described by Equation (5). This gives a mathematic solution for the collection, but does not give the physical solution. According to the next Equation (14) and Equation (15), we can see relativistic gravitational potential , and hence, the sphere would reach to equilibrium in a certain point. This idea can be the starting point of the physical solution. Now, we can define extreme electric initial speed as Equation (10), and also, we can define nonrelativistic gravitational potential:
And from Equation (12) we can get:
Hence, we understand that we can control and define the arbitrary quantities inside the sphere such that finally we get
And hence, we can understand the physical meaning of Equations (6)-(11).
2) Equations (20)-(27), can discover the mathematics of how each of uG and ue looks as:
, and how they add:
3) This sphere represents a bound system. The bound system has a lower potential energy than the sum of its constituent parts. Its mass must be less than the total mass of its unbound constituents. In a separate section we would define the potential energy which binds the constituents of the sphere. If we looked to this sphere as a large nucleus, then all the energies―of the semi-empirical mass model―vanish, except the asymmetry and the Coulomb terms, in addition to the new term which is extremely considerable here in this very great nucleus. We would see how both, the electric repulsive and the quantum exclusive energies are aborted inside this sphere. The future―as we will see soon―carries something bad for the compound: . This uG will represents something other than central binding energy. Hence, the system as a bound state should create a free ug. This means that the particle mass should lose a little bit.
Since, the rest-particle mass after missing becomes ms (not mo)
By the law of conservation of energy, the energy of the system remains a constant (u = constant):
. This is the intrinsic property of this magic sphere!!!
The cardinal idea is that: The factor that acts upon u to conserve it, is the same factor which converts v2 into c2 or, the deficit in the mass due to the gravitational binding energy
Hence, we can recall equation 2 to understand what the information which it carries. It say’s: if like then, it is―like Equation (1)―zero kinetic energy, such that, the energy which appears is rest energy (zero speed-state). So, the sphere succeeded to abort u: the original relativistic relations vanish, and replaced by alternative relativistic relations defined by Equation (22) and (56).
In above, we labeled the rest energy of the system by the subscript t, to distinguish from the inertial energy of the rest particle. The next diagram summarizes the entire above. The rest particle would be labeled by the brackets { } while the energy of the system would be labeled by the brackets [ ] so no further need to the subscript t.
↓
By the dynamics of conservation of u:
It must be clear that the term b―which appeared in Equation (9) and which appears in other positions of the text―acts on u by the above dynamics (by conservation of energy) not by else. Hence, we can again recall Equations (6)-(9) to understand how the term acts with the conserved term u
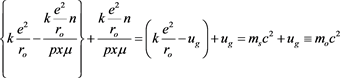
Appearance of the term 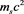 is enough to convince that Equation (2) is realized and u is aborted (it was transformed into rest energy). The two terms
is enough to convince that Equation (2) is realized and u is aborted (it was transformed into rest energy). The two terms  mean that we have got the particle which is defined by Equation (1), but inside a collection. If you want the same free particle―defined by Equation (1)―to appear here, then we can add the sign of equivalency. The confused point here is that, if the relativistic uG had been aborted with its conjugate ue, hence we can ask about how a new ug could be precipitated in the system. The answer you can find it in Section 9.
mean that we have got the particle which is defined by Equation (1), but inside a collection. If you want the same free particle―defined by Equation (1)―to appear here, then we can add the sign of equivalency. The confused point here is that, if the relativistic uG had been aborted with its conjugate ue, hence we can ask about how a new ug could be precipitated in the system. The answer you can find it in Section 9.
It is clear that the quantity  which appears always with the summation
which appears always with the summation 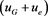 it is not―at all―the same original rest energy, although, it describes the original rest mass. Therefore, we have to distinguish between the inertial (internal) rest energy of the same original rest particle and the rest energy of the system which represents the subject of this article. Where did the kinetic energy go? Here is the answer:
it is not―at all―the same original rest energy, although, it describes the original rest mass. Therefore, we have to distinguish between the inertial (internal) rest energy of the same original rest particle and the rest energy of the system which represents the subject of this article. Where did the kinetic energy go? Here is the answer:
We have two functions: one describes a constant real speed, while the second describes zero speed-state. Both should appear in a general solution. According
to the original relativity, the kinetic energy appears as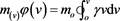 ,
,
while it appears in the alternative solution in the form:
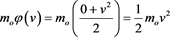 . As you see, in this alternative solution, each of
. As you see, in this alternative solution, each of
the mass and the speed is a constant and is neither a continuous nor a differential function. Hence, we can conclude: the general solution―which adds the two solutions―, represents a particle bouncing forth and backward―inside its defined space―between two points each with infinite potential. We need a discontinuous function to represent a harmonic but not simple motion (not sinusoidal):
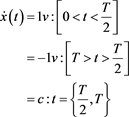
Such that c represents the zero state (rest energy), while .
.
The upper case letter describes the time of the oscillatory motion 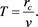
The last solution carries the meaning of 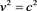 which first, can―by the addition to the original rest energy of the particle (
which first, can―by the addition to the original rest energy of the particle ( )―realize (as we will see) the general solution
)―realize (as we will see) the general solution 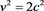 and second, could be considered as the macroscopic state or the boundary condition which should strictly define the first two microscopic sates. Hence, we have:
and second, could be considered as the macroscopic state or the boundary condition which should strictly define the first two microscopic sates. Hence, we have: 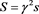 (please look at Section 15 to realize that the relativistic particles number is a temporary stage, and we will return to the initial particles number: a fact which agrees with the familiar knowledge)
(please look at Section 15 to realize that the relativistic particles number is a temporary stage, and we will return to the initial particles number: a fact which agrees with the familiar knowledge)
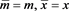
4) From all above, we can summarize: the particle inside this magic sphere appears as a “paper” formed of two faces. The particle itself looks as a point while the remainder of the surrounding is the energy of the system. On a face of the paper is written:
Special relativity―here―and only here, is constrained by Equation (1), therefore; the repulsive and the exclusive energies are aborted and consequently, the original relativistic relations are―also―aborted. This means constant density of matter.
While on the second face is written:
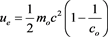
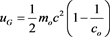
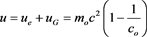
Now, let us divide the sphere into two sub-spheres.
5. The Relativistic Protons Potential Well & the Approximate Solution
Now, let a defined quantity of protons in a defined density of nucleons to allow each proton to gain the above defined speed 





potential energy per a proton 



where; rs is the radius of the sphere & G is the universal gravitational constant. As we saw in the beginningof this section―and to realize Equations (2) & (5)―(we would see later that the magnetic moment energy of the sphere is close to zero, therefore uG would replace it to realize the intuitive mathematic solution) there should be a form of equality (that is to say, we are searching for the conditions required for this equality) appears in the form:

The right side of this equation represents the electric energetic function which should appear―in the Compton’s sphere―in a rest form (one half of a particle in a rest state) while the left side represents the second half of the rest particle (see Appendix).
From the Einstein’s equation form:



is realized only at
(11)―which carries the relativistic meaning―and not perfectly from (1) so we would consider (17) is a proposal realized only at existence of the terms b & D defined in (9), and hence we would deal with the next equations on this base where later on we will study how the excess term is deposited in the equation. Let us now see how the term “D” is added.
Hence, ―on this proposal―we can do algebraic simplifications (abbreviations on the factor co) where finally the equality appears after simplification as:

where; co is the equality factor (defined from Equation (12)) and acts in this equality by a vague process would be determined latter and the negative sign means that the two sides act opposite to each other. The solution in the form of asymmetric addition is;

Let us search for other solution and so go back again.
Let us recall the equality;
Put 

Rewrite (18) in its simplified relativistic form

By substitution of (12) & (20) in the left side and of (4) in the right side of (21)
Since, 

Since, n is the counting of the neutrons, but we want the actual counting of the nucleons (where

The middle side of this equation―like the left―has become positive energy; such that each side is―now―possessing the same magnitude and the same
positive form of 
solution as:

The term D is―now―deposited in the additive form where each term of the left side represents the value of the energy of ue & uG.
This equation does not describe a new created particle but it describes the Compton state energy (per a proton)―definitely―in a rest state, as equivalent to the right side of the equation, so it is a new description (originating from the Compton state) of the rest particle which when be added to its inertial description we get:

Equation (25) describes the total rest states (0 + 0). So the proposed equality (20) has a solution appears simply from (25) and also has a relativistic concept in the form of:

And from (26) it has an original relativistic solution as;

Equation (23) is the simplest form regarding the factor co there for it is the correct form which shows the correct relativistic alternative solution. Equations (23)-(27) act as one packet. Equations (27) & (25) are synonyms where they represent the condition required for existence of the giant charge where such state does not mean 

But this conclusion appears only inside (22) and the equations derived from it. So Equation (23)―which is the simplest form and which engulfed physically the negative sign of the proposal of Equation (20)―is the one which realizes excellently the initial condition which is discovered by Equation (24), so it is the one which carries the alternative relativistic solution. Implicitly, when Equation (20) takes place in (18) and when the symmetric addition takes place in (24) which carries the meaning of 



Equations (20) & (27) realize each other in the special relativity and represent the second block in the giant charge physics.
Now from Equation (23) we can write the original relativistic mass in the form of:

A new terminology can correct any misunderstand. So we can put the reproductive energy or the rest energy of the system in place of the kinetic energy. Dividing the two sides of Equation (22) over p discovers the factor m which appears (independently from Equation (12)) as:

Since; 

where; the capital letters N and P are the “relativistic” number of the neutrons and protons of the giant proton (the adult) while the small letters n and p are the “rest” number of the neutrons and protons of the giant proton unit (the zygote-like unit). Actually, the relativistic dilatation of the particle number is not strange in physics: as the velocity of an observer relative to an emissive surface increases the number of photons (due to time dilatation) encountered by the observer per unit area per unit time (intensity).
6. Physics of Compton’s Sphere
1) Equation (2) describes the particle in a rest state by the energetic functions of the Compton’s sphere (in addition to its inertial energetic functions) so the final result is a rest particle defined by the intrinsic properties of Compton’s sphere (the new rest spacing rc) in addition to its rest radius ro.
2) Since the rest particle is defined by its electric & magnetic fields, and since the superposition of the charges of the particles is the origin of the charges of the collection, so the sphere (collection) has electric flux:

where n (in this & the next equation) is the unit vector in the outward direction of the sphere. And has magnetic flux:

This means no magnetic charges inside the sphere, so we need a flux to replace that zero flux. Hence, the flux of the conservative gravitational energy ug can perform this role. But since the particles are oscillatory moving so this ug would appear as uG (you never forget that Compton’s sphere energy is a copy from Equation (1), so its entire equations should go straight forward with this equation.
The goal in this point was to define so great speed that uG can represent the half of the energy of a rest particle. This was done by choosing defined number of particles within defined density (as we will see in the next sections).
3) Since, we need to realize Equation (2) (the initial conditions) so, we have to do algebraic simplifications on (

where each of the left & the right side is fixed on 
together we can get a description of a rest particle.
4) After all, Equation (22) leaved behind; 

5) If a particle moved in speed v (the motion inside our sphere comes from Equation (10)) then the linear mass density;
So, this may carry a hidden relativistic meaning appearing as creation of new rest particles (If―and only if―we were inside Equation (2)) counting
6) The strategy of Equation (22) is that; since Equation (2) is the final macro state so mo & ro of Equation (22) should be eliminated from co where each side
of the equation is equivalent to
7) We have to notice that the done algebraic simplification makes 
8) Because the term 


from the same equations as; 
the typical potential. Thereafter, we should call it 'the relativistic gravitational rest energy which with its conjugate: 





potential because it is written in the form
you notice that it is a negative term with denominator formed of the real radius of the relativistic sphere (you will see astronomic application in Equations (74)-(76)). The rest energy, all over the article, means the additive conjugate 

9) The particle is now defined (after the additive process) by the rest state (0 + 0), which means no motion and so no relativistic relation. To fire the relativistic relations we defined the Compton rest state of the particle to appear as; 


10) Equation (25) does not mean neither description of two particles nor production of a new particle where (25) is defined by (27). So self repetition means neither transformation of energy into new particle nor creation of particles by collision. But it means―here―alternative relativistic solution.
11) If we treated our sphere as in the semi-empirical model then the above is the coulomb’s term while the others would be discussed step by step.
12) After all, Equation (2) does not add a new particle, but it describes (new) rest energy originating from the Compton’s sphere (per a rest particle) exactly as equation 1 describes the rest mass of the rest particle.
13) Since the electromagnetic energy density describing the rest particles is constant and does not obey the superposition (if we have a proton among a collection of protons inside a sphere, then each proton is still possessing rest energy defined by 
side of the equation―which is describing the rest particle―, is equal
then it would not obey the superposition. If the left side of the equation went straightforward with the conservative electric energy then it had to be multiplied by P not p but since it became describing the rest energy of the particle so p which realizes 
asked about the fate of the electric energy of the ratio 
next explanation. Put 

Although this is contrasting with the current knowledge but let us go on to see that our solution refuses this incorrect speed. Now we want to see the relativistic effect of the speed 

where, the minus sign has a final physical meaning realized by Equations (25) & (27). This means that the alternative relativistic solution returns






This addition―after the physical engulf of the negative sign (which appears with m2 in the original relativistic solution) by Equation (22)―leaves behind the relation 
14) The physical meaning of each of the new extra definition of the rest macro-sate of the particle (inside our sphere) which is defined by rc and the physical meaning of the imaginary factor which is plugged in Equation (21) would be more clear from the next study of the wave function of the particle. The particle inside our sphere is defined by 

Since solution of Schrödinger Equation (1D) for a particle inside infinite potential well takes the form of; 


a) Speaking about the relativistic function and the real speed of the particle then we would have the alternative relativistic relations defined by Equation (28). Therefore;




So the relativistic wave function appears as; 



This solution treats the particle as a point moves really forward from 0 to L and backward in the opposite direction. But finally the particle looks in the mid-point. It is better to say that rc carries here the meaning of the spacing not the rest radius of the particle (The energy density is explained in a next section).
b) The next goal is to show that the zero term of the wave function solution has physical meaning for the particle inside our sphere. So, we would search in how this zero term (of the wave function, 1D) realizes the new extra definition of the rest particle inside our sphere. This extra definition needs describing the relativistic mass by (26) where the imaginary factor appears as; 


physical meaning of this case. If the case satisfies no motion then x is constant and the integration is transformed into simple multiplication.

After normalization of 

The solution tells us that if l is the length of the particle then its expected length is l.
Although it is a trivial solution yet it realizes Equations (26) & (27).
This means that the first term 


15) The last point here is to know how the original relativistic solution takes place in the radial direction. If the motion of the sphere’s particles occurs in all directions in the same time (like explosion or growth) then we can write the invariant event interval 

where the primed terms stand for the moving coordinates. We can formulate the relativistic relations of the motion as follow:




And finally, we can recall the properties of the metric tensor and write: 
Again, because aborting this original solution, then, the alternative one should take place.
After all, we saw a “physical snake” have engulfed the repulsive electric energy which appeared with the relativistic uG as a conjugate pair. Once the initial condition was realized then we need a relativistic solution―other than the original one―which appears as Equation (30). Microscopically, the events inside the Compton’s sphere are fired up by Equation (7) (where the real velocity v = c + deficit) or by the big brackets of Equation (6) the alternative relativistic solution passing through the entire packet of the equations from 7 till 68 and finally it should appear in the form of Equation (1). Since, the macro-state of the relativistic Compton’s sphere is no more than multiplication of equation one by the number one so you will notice that the macro-state of the entire packet of the equations from 1 till 96 (which describes the interior of the giant proton) obeys―macroscopically―the same rule (conservation of density of the states & density of matter, and so it describes forever the rest state).
Actually the sphere gives the solution as growth in the three dimensional space, with the final constant density, from the initial sphere with the initial number of particles n and p―let us call it the giant charge unit (GCU)―till it reaches to the final sphere (let us call it the giant charge GC) with the final number of particles N and P. Or; (GCU) co = (GC). Hence, the goal―in this point―is to define astrophysical meaning for N such that the giant charge is fit to orbit. We need to understand the transformation of energy (actually, ―as we will see―it is not passive transformation but it is relativistic solution) into matter in this alternative solution deeply and not superficially. We need the entire equations packet; we need the particles spacing r to be defined by a bit excess above rc which gives quantum speed defined by a bit quantity below c. Also we need to pick up the relativistic imaginary solution and deal mathematically with it to find that 

7. The Relativistic Neutrons Potential Well (Asymmetry Term)
Let us define the Compton’s (reduced) wave length of a free neutron as; 
And let the momentum to be defined as: 
To define the spacing which defines this
The uncertainty of the reduced Compton’s wave length means transient transformation of matter into energy and vice versa or actually creation and annihilation. This is not our case, where self-replication in our Compton’s sphere
is a relativistic action. This means that if the uncertainty 
spacing 

1) Since the highest energy which anybody can acquire is
then the exclusion quantum energy (symmetry term) of any collection of particles (inside a closed system) never exceeds this highest energy and consequently its spacing never exceeds the above length. In the next discussion we will search in the exact required density which realizes the highest possible energy inside Compton’s sphere where the initial conditions are realized.
This density allows us to use the new expression; Compton’s sphere instead of Fermi sphere. We expect that the density inside the sphere is in this range but the accurate value of the spacing should be defined from the density of the states of the collection. In GCU and because of 
(You noticed that number of nucleons A is given here in our model the symbol n and number of the protons Z takes here the symbol p)
In the Compton’s sphere (on the same way of Fermi sphere) [5] ; Pauli’s exclusion Principle needs to multiply the count of the filled states by two (+/-spin). We need―also―to divide over eight [5] to get only the positive space octant (where

This―for all neutrons―becomes
where n is the quantity of the neutrons of the giant proton unit and V its volume. And finally divide over n to get the average
By putting 
can estimate the average;
This is a comfortable result, but since the accurate value of 





2) This is the accurate spacing which defines the Compton’s sphere (as defined by Equation (10)). You can notice that the major character of this sphere is that the in-between distances are in the range of the reduced Compton’s wave length 


The relativistic gravitational energy



If we write 
multiplication of the constant numerical factors 
(31) then we can rewrite Equation (31) after simplification as follow;

The factor 



where, A & G are constants and ro―here―in these relativistic relations means the rest distance rc (otherwise it means the classic radius of the neutron).
Putting 




Putting 

Adding the two energies of (36) we get

where; uc & uG appears as conjugate pair which when be added together then added to the inertial rest energy they realize the state 

The alternative solution is constrained by; inter-between distance ≈ 3.127 × 10−16 m. This gives (in the original solution) quantum kinetic energy for each neutron ≈ 0.752 × 10−10 joule which when is added symmetrically to its conjugate gives the state of (37). The neutron (like the proton) appears in its potential (or precisely, the inertial) well as a pair of conjugate. We also notice―from Equation (1)―that,
8. The Integral Form of Compton Sphere
The average of the quantum term as estimated before appears as:
By substitution and putting the value of rc we can estimate the average:

But the average of the electric potential energy needs to do the following:

We can do the same manipulation with the average gravitational energy. So the average gravitational and electric energy appears as

This form is not compatible with the inertial form 

where, the question is to find the quantity of the protons p, which realizes this modified integral form. And to appear as

where, the question is to find n which realizes this modified integral form. But we do not have―from the system―enough equations to define all the unknowns of the system. Let us search.
9. Definition of the Giant Proton Unit & Driving Its Unknowns
From the definition of µ and from Equation (29):
where N is the quantity of the neutrons in the giant proton while n & p is the quantity of neutrons & protons in the giant charge unit.
We want to define co as a universal constant. In the second part of the discussion we will estimate N

By dividing each side of Equation (22) over co we can define

And from the meaning of self-replication of the giant proton unit―till it reaches to the giant proton―and recalling Equation (30):


And since,

And since,

From (22) (24) and (48):

By dividing 
By putting the value of n and substitution in (46) we can define co and then from (49) we can define p (from ue).
From defining p & n we can define the ratio
From the defined values of 
At last from (39) we can―also―define―ug.
This arrangement in derivation of the unknowns (where we derive ug after solving the entire unknowns) is necessary (we will explain this latter). Now we can estimate all the unknowns as





It is clear―now―that we cannot realize Equation (2) with speed less than that defined by Equation (54). A lesser one cannot realize Equation (6). It is the
familiar to equate the particle speed by real number: 
but here, physics of the alternative relativistic solution allows equating the squared speed as c minus an infinitesimal cut off. This cut should not allow a lesser squared speed than that of Equation (54). But a bigger one (approaching more to the speed of light) is allowed. This allows―from Equations (23) and (56)―a bigger γ2 (co) which means replication of a bigger sphere (this is the scientific base for a more complicated giant atom as we will see later). Therefore, we can define co to include more complicated giant system:
We have to notice that the protons have the same wavy behavior and so it has―also―uc but this term could be neglected because of the distance between two neighbor protons = 104rc (where n/p as we will see is about ten power twelve). So, the exclusive Pauli’s term of the proton well appears as; 
Because we estimated the above values from outside the system of the giant unit (using, N) so we have to test them. So putting the above values in the equations of the system we find finally:

10. The Deficit & the Excess as a Perfect Solution
The goal here is to show how the term b is delivered from the same alternative relativistic solution which was fired by the starting point (Equation (7)). If this term was found independent from the alternative solution and since the deficit equal the excess then both with each other had to give zero term. The zero term would spoil the deficit and consequently aborts the relativistic action. The relativistic action needs a deficit (not zero term). Then, it looks as if the excess was delivered side by side with the term D and by the same alternative relativistic solution. That is to say; Equation (7) is actually the launching point. First, we can summarize what we got.
We “picked up” the imaginary relativistic solution, 

After that, we introduced this refused solution on the relativistic gravitational energy, and then go on till we reached to the accepted substitutive relativistic solution. So physics of the giant charge could be summarized as a shift from the original solution;

The alternative solution is surely not passive transformation of energy into
matter (not as;
transformation would give one particle for each of the contributors.
The relativistic solution is completely far from this vision. It could be written as

In the original solution 
The first point to motivate physics of the Compton’s sphere is to insure that the total Compton state final energy u, per a particle neither above nor below moc2. Its net value should be exactly moc2. If it was above this limit then this means that 
quantum energy 
or mathematically, 

Since, 

where, ug―with the small letter subscript―is stand for the nonrelativistic gravitational energy (not uG). Equation (58) surely describes (1 & 2) but―till up now it cannot describe our sphere without realization the condition mentioned in (9 & 27) which are not yet realized. Equation (57) is just to say that: to prevent excess speed over c or to prevent the state which is inside the brackets of (58) we have to add―ug or definitely the acquired energy must be not over the energy of (57). But this equation alone doesn’t tell us how does ug precipitate in the system. All the equations which contain a cutoff energy―from Equation (6) and upward―could be understood on this base. In Equation (7)―as example―the deficit 

You can have a population of neutral particles with zero electric energy. And you can have a population of particles with zero short range energy if the in-between distance was far from Fermi range. And you may get a population of matter with zero quantum energy such that Pauli’s Exclusion Principle may disappear in condensate state (like; Cooper’s pair & neutron pair). Moreover the quantum term which appears in Equation (33) depends reversibly on the mass so it disappears at the mass goes to infinite. Definitely, you can forever multiply a wave function―describing a quantum particle―by a non-zero complex constant A, to get―by linear combination―the same state with the same physical concept as:

But you never get matter without gravitational energy. On general: no field is forever synonym to matter except the gravitational field. Since each of the contributors; ue, uc and uG are energies would be added to the particle and since the gravitational field never vanish from matter and since the system supplies each particle with ug then the contributors should have a deficit equal ug otherwise the added ug of the system would add speed to light which is forbidden. This additive ug is the residual energy of the system after withdrawing all the other energies.
Since,

We began with Equation (7) and followed up the microstates of the coulomb’s form where each conjugate pair realized in the approximate solution that the relativistic mass is equal to the rest mass, so up till now we are still maintaining the general structure of (1 & 2) if―and only if―(9 & 27) were realized (or if the term b was deposited). Equation (58) expected presence of ug but it did not precipitate it. So we need to fix (57) on Equation (58) (but without its brackets). Let us see how. Now, since
And since,
Then the conjugate pair u appears as:

Again, since 


Actually this speed (as we will discuss later on) doesn’t need external source of energy outside the system. If we looked to the neutron in its well and if 


This idea should be maintained and never waste. Explicitly, the energy which we observe in a conjugate pair of u is not more than description of the rest energy of the Compton’s sphere per rest particle perfectly as Equation (1) describes the rest mass.
Equation (58) describes the rest energy of the particle of Equation (1) perfectly. And it can also describe the particle of our sphere but only when we do approximation for the relation 
The approximate solution enabled us to fix our system on the form of (1), where (58) described the rest energy as a perfect synonym to the rest mass.
Now we can begin again from the speed which is defined in (10), so let us recall the accurate value of v2 as it is defined in (60) and put it in its original relativistic relation

As in the approximate solution, we can put here 



This is the same radical physical meaning of the proposal of (20) where here it again engulfs the negative sign of the equation in which it was introduced to leave behind a physical meaning. The equation―now―tells us that we have one particle with two different rest masses. The unique solution should be defined by different systems. That is to say the right side of the equation represents the rest mass of the particle (ms) inside the sphere before adding ug while the left side represents (mo) outside the sphere or inside the sphere after adding ug



By simple simplification and multiplying each side by c2 and then substitution from (44) with the value of ug and substitution in (63)

This is the relativistic solution for existence of the nonrelativistic ug in our system. Hence, we can fix (57) on (58) as:
where the contents between the brackets represent the equivalent energy of the rest mass of the particle inside the sphere (the particle with the deficit) while the positive ug is the added gravitational energy so the relativistic mass
Also the energy 

If we put: 


We notice also in the approximate solution that, we used 
Since the deficit in the rest mass of the particle which is defined by Equation (1) is a deficit in the rest energy of the Compton sphere (per a particle) which is defined by Equation (2), so we began from:
This deficit fired up the relativistic solution by the speed which is defined by (10). Then we used the original relativistic technical tools which finally produced the alternative relativistic solution which appeared as: 



where, the content between the brackets appears with its deficit which fired the alternative relativistic solution which delivered all the outside the brackets. Finally we get:
And by the symmetric addition we get:
The last equality was put to show that we are finally in a complete rest state (

Defining the unknowns of the Compton sphere depended on the next idea;
since we began from 

So, the approximate solution ignored the small deference between these quantities where the equation got;
This inconsiderable error which was committed by the approximate solution could not be avoided because each of ms & ug is unknown (but could be derived after defining the other unknowns)
This means that from the quantitative side of view we can put 




Anyway, Equation (79) from which we will derive the quantity N shows that the speed resulting from the gravitational energy is so little that we can neglect md.
The meaning of mo & ms appears well from the normalized wave function,
where the wave number
Appearance of the rest mass as ms in the subsection (13-a) is well perfect because this means c2 of the fields split as; 

We can summarize all the above chapters to give the global picture as follows;
Given a particle let be called P, defined by 
where this equation says; the rest mass is equivalent to the rest energy. The particle in the giant charge committee is defined by rest mass ms & mass deficit md but since the rest mass of this equation is synonym to the rest energy of equation 2 which describes the macroscopic state of our sphere so this mass deficit appears also as rest energy deficit. Hence the particle appears as
This deficit in energy fired out the alternative relativistic solution defined by (56). But the particle with this deficit is not the particle P. Fortunately, the relativistic solution itself is the one which delivered energy excess equal the energy deficit. And again, since the rest energy of the sphere per a particle (defined by Equation (2)) and the rest mass (defined by Equation (1)) are synonyms so we have the same excess in the mass too. Hence the particle appears as

net result is 


the meaning:
As in the semi-empirical mass model the nucleons inside the nucleus has mass deficit which appears as nuclear binding energy also here. The particle inside the Compton sphere has to pay from its pocket to subscribe as a member in this committee. The sphere looks like a giant particle where its vacuum is absolutely energy empty so the particles to live with each other they have to pay from their masses. We have also do stress on that, the system itself by its nonrelativistic equations cannot precipitate ug because each of Einstein & coulomb form talks nothing about it although coulomb’s form (the microstates) expected its existence. The matter also calls it, but the allowed nonrelativistic equations cannot realize it. So the relativistic equations discovered the trivial solution;

But its hidden nontrivial meaning is that; each of the minus & the positive ug & its synonym md is a relativistic solution. The deficit in the rest mass of Equation (1) is equivalent to the deficit in the rest energy of Equation (2) but this deficit by the relativistic action have created final relativistic solution formed of one packet appeared as; reproduction of particles counting 
The kinetic energy appears―by the alternative relativistic solution―in a rest form. This means that the GCU have appeared after all these relativistic micro-states to realize Equations (1) where it means actually that it came from multiplication of Equation (1) by the number one to give the same solution. The energy looks as if it was turned from the region of the kinetic energy and flashed in the region of the reproductive rest energy with the simple conservative momentum & energy solution;

In biology, self-replication takes other form. The chromosome of the cell of the living thing―by metabolism―utilizes the nutrients to construct another chromosome. No creation of particles; neither from energy nor from relativistic action. Number of the input of the particles (of the mother chromosome + of the utilized nutrients) is the same of the output (of the mother chromosome + of the born one). The cell during replication does not gain particles. So the substitutive solution is not clearly suitable here. The dead or even the adult, or accurately the immature or even the old cell cannot replicate even if it was put in a bath of the required nutrients or―accurately―even if it was put in the same biologic medium required for replication. Here, there is no mass deficit and consequently no energy deficit. The chromosome acts as a template and appears like 

This view does stress on that 
11. The Wave Function & the Concept of the Particle Speed
The defined density of matter―inside our sphere―alone can offer quantum speed for the neutron, but it cannot alone define the proton speed which needs defined number of protons with the same defined density. We saw that speed of both particles is defined by Equation (10), but what is the concept of this speed? The energy eigenfunction of the particle inside the potential wells could be written as;
where on normalization:
Since, the momentum operator
And since,
At first glance this conclusion seems strange. After all:
The ± sign provides the explanation: The particle is moving back and forth, and so there should be two momentum eigenfunctions for every energy eigenfunction, corresponding to the two possible directions of motion. The procedure for finding the eigenvalues of a quantum-mechanical operator 
Hence each energy eigenfunction can be expressed as a linear combination of the two wave functions so that
From above we conclude that the particle inside the Compton’s sphere oscillates back and forth with its speed v ≈ c.
From the side of view of special relativity, (if the capital letters stand for the measurements on the moving coordinate) we hold on the synchronous measurement (T = 0) for a body moving to the right:
And for a body moving to the left we would hold:


Since―as we have discussed before―both xo & mo go straight forward in a linear relation so the same result holds with the relativistic mass.
Actually many experimental trials succeeded to trap ultra-cold neutrons in slow speed. High technology succeeded to trap neutrons with speed of 5 meter per second [7] [8] . If we could to get the least possible speed like that of the giant charge then we may get it. But I can suggest that if we had a universe defined by the Equations (45)-(54) or if we had count of neutrons defined by (52) each with
speed 
spontaneously condensate to Compton state.
12. The Solar System as Giant Atoms
Now in this second part of the discussion we would suggest that the solar system in one of its primordial historical evolutionary stage had presented as giant atoms. This suggestion would be tested by doing comparison between our estimations about the parameters of the orbits of the giant atom and that of the solar system. The tested parameters of the solar system would be:
Speed and radius of the orbit of each planet, the elliptical orbits of the planets and also we would define the ratio between the mass of the sun to the total mass of the entire planets. So, our work suggested that the solar system had begun its motion as units of giant atoms. Later on, the atomic model disappeared after it left behind the above mentioned parameters to refer to the past existence of the giant atomic model.
Now the giant atom was formed of giant charges, and the giant charge had existence in the form of Compton state. Existence of such state―as we saw from physics of the giant atom―has overcome the following problems; coulomb’s force which would not allow existence of much charges in a comparative narrow space like the giant charge, Pauli’s exclusion principle; which never allow two typical fermions to exist in the same energy level and the quantum energy density which acts against condensation of matter in such a state. We would see also (after estimating the radius of the giant atom orbit) that the giant charge can orbit as a collection of points in a circle without considerable radiation (not violating Larmor’s equation).
12.1. The Hydrogen Atom Like System [9] [10]
Consider an electron e, with a non-relativistic mass me and a proton p, with mass mp orbit each other and consider μ stand―here―for the reduced mass.
The two body problem could be reduced as one body problem as
If,

If,
The Lagrangian Lg could be written as;
The modulus of the angular momentum vector = L

The ground state 
The solution gives;
The expected radius
The expected velocity
12.2. The Major Atom: A Proposed Inversed Atom
Although it is a proposed system yet it is the mathematic base of the giant atom system. In the giant electron we can hold the same equations of the giant proton.
Since, the proton count p in the giant proton = the electron count e in the
giant electron and since the electron mass 
of the proton and if we substituted in Equation (16)
where, 

proton. Hence we can say that the giant atom is a perfect inversed atom. This chapter is to study the mathematics of this inversed atomic system. So, in the hydrogen atom like―system (h.a.l.s); suppose that we succeeded to bind its
electron through imaginary energy with a number of neutrons =
neutrons. And suppose that this proposed binding energy ≥ the total energy between the proton and its orbiting electron such that the neutrons bound electron can orbit as one body. Once we can create this imaginary major atom we will notice that here would be shift of the center of the masses towards the neutron bound electron. There would be (also) respective relative shift from quantum to classic physics. Let us study this inversed atom (i.a.) side by side with (h.a.l.s.) and let us use for the first the capital letters and for the second the small letters.
We did nothing except binding the electron with 1844 neutrons so;
From (69)
where, Me is mass of the electron which is bound with 1844 neutrons
And where, μ = the inverse of 

From above we can get
The angular momentum L obeys:
where, l is an acquired inversed atom level number.
On substitution we get


This speed is the speed of the orbiting proton in this theoretical inversed atom model. Since the giant atom is inversed atom model so it conserves the same speed.
We can get also
where λ is the wave length of Bohr’s radius.
12.3. The Huge Charge
Here we have to focus on the next equations of the giant atom which supplies us with the physical unit of the orbiting giant charge; let us call it the huge charge. The huge charge which appears in the giant atom equations is the smallest unit of the orbiting charge. It has a mass mu and a matter wave length λu

where λ is the wave length of Bohr’s radius.
Its angular momentum would be in the form;
The circumference S of the giant orbit is the summation of the huge waves;
The orbital could be considered as one dimensional infinite square well of length 2πr or a model for a one-dimensional box. Since that, we have two particles with different states; +1/2 spin & −1/2 spin occupying the same energy level. So that,
You can―also―consider that the factor 2 in the denominator is due to that; the wave length is formulated as “a particle in a box model” such that: 
Since, “in this chapter” n & p is the number of neutrons & protons inside the
giant proton (not inside the Compton sphere) & since, 

where, the factor inside the brackets is constant as defined by Equation (51) (it is the same; in the Compton sphere & in the giant proton) while n is the number of the neutrons inside the giant proton (which we called in Equation (43); N).
12.4. The Mathematic Analysis of the Giant Atom Orbit
The giant proton to orbit the giant electron we need equation in the form of:

where the left side of this relation means the gravitational energy inside the giant proton per a huge proton lying on the most outer layer of the sphere (defined by
the mass
electron and the giant proton in its orbit defined by the orbit radius R per a huge charge. This form simply and directly means that:
Since the spheres are in orbits then the total energy equals half the electric potential energy. If this total attractive electric energy between the sphere A and a huge charge of the outermost layer of the sphere B was just equal or lesser than the gravitational energy which binds this huge charge with its sphere then the sphere would orbit as one body. Obviously, we take a charge from the most outer layer because according to Gaussian’s theorem, although the most outer layer has the maximum flux yet its particles has the minimum gravitational potential so we guarantee that if this particle preserved on its sphere then all the particles of the sphere would do the same. If the left side of the above relation was lesser than the right side then the charges of the giant atom would be attracted as singled particles which would lead to evacuating it from the charges and consequently destroying of the whole electric building. This is very important point which would give radical and dramatic event would be discussed later (during talking about the end of Compton sphere). The hidden important meaning
behind the factor (
mentioned condition―that the huge proton is the unit of the orbit.
In one word this condition (the equality form) guarantees that the sphere would orbit as huge charges units with the least amount of n and consequently of p. But the relation in its inequality form can allow additional n as we will see when we speak about the astronomic giant proton. (Obviously, we can conclude the same required condition if we applied correctly the physical meaning of the escape velocity). Since, ―as we will see―the higher orbitals are built by additive particles to the ground state so this ground state should be built by the least amount of the particles. So, Equation (75) neglected a factor coming from the integration which had to be considered. Suppose that the total electric energy between the giant proton A, & the giant electron B, is just more than the total binding energy inside A, so that A, would be evacuated from its charges. We want to estimate the least amount of the charges which cannot do evacuation such that adding any other amount of the charge―even one charge―would lead to increase the electric potential more than the binding energy so evacuation begins to take place. Since that, we want to prevent addition of extra proton. Therefore, the correct estimation needs to suppose that the protons on the surface of A, are pulled to B, one by one by the least possible power. Since each of the two spheres has curvature then there is a point O, on the surface of A, is the nearest point to B. The electric work done (by B) 


where, the huge mass (
The least amount of the binding energy which is required to prevent evacuation and to enable the sphere to orbit is formulated from (74) & (75) as;

You can see that loss of―even―all the protons in the mind of the left side means loss of mass = 10−12 of the total mass (so it is constant), while that in the right side means 
13. The Giant Atom Orbit
We showed that the giant proton to rotate―by the electric field―in its orbit as one body (or as huge charges but not as individual protons) needs internal gravitational binding energy (the particles by its own gravity bind with each other). From (74) & (76), the least required binding energy has to be in the form

where; 


where: rc is the spacing between particles inside the sphere. Or could be written as

Now, let us re-go to the giant proton unit which needs the ratio
From Equation (70), 

Since it is known that our sun losses gradually some of matter in production of heat. It is known that it lost about seven percent of its natal mass [11] so if the present mass of the sun = 1.99 × 1030 kg & if its natal mass was, A
By the inverse square law, we have ?also―natal orbit speed;


This is the circular giant atom orbit, but the astronomic orbit of the mercury planet is elliptical with eccentricity 
Equation (78) tells us that the orbiting giant proton possess number of particles just enough to overcome the electric attractive energy. If the orbit was elliptical then this energy would be maximal at the perihelion Rp therefore this quantity of the particles has to be enough to overcome this maximal energy. This means that we have to substitute in (78) at the perihelion (minimal distance).
From (77) we can conclude that n2 is proportional with 
to multiply n by
This is a constant factor should not be introduced in any other mathematic operation. It is a factor just to guarantee that the quantity n is ready to overcome the maximal possible electric energy and to orbit as one body. Putting the value of rc and putting the value of the average speed v and by substitution in equation 78 which appears now as;

So, we can get the number of the neutrons n of the orbiting giant proton;

This is the least possible quantity of the particles in the orbiting giant proton whose binding energy can overcome the total electric energy at the nearest point (perihelion). From the first equality of Equation (77) we can conclude that;

where, A is a known constant.
From the second equality of the same equation we can reformulate:
By dividing over (82)
where, B is another known constant.

Or, we can conclude the value of p directly as
By substitution in Equation (73) (or from the above equations)

since, the mean value of the orbital radius of the nearest planet (mercury) equals about 

From (84) and by using the inverse square law we can estimate the real natal orbital radius:


Later on―in a separate discussion―we would see that each a planet was formed of number of these giant charges then in a next stage and after beta decay and formation of atoms the inter-particles distances would increase and consequently the giant charge radius would increase which would lead to inability of the unit to keep its charges which would lead to escape of the charges and consequently attraction and unification of the units to form the planet where Newton’s laws would receive the file of the motion as the same R & v and converse it without modification by the same square inverse law in its gravitational form. Unification is not a simple classic addition but it is quantum overlapping of the waves to maintain the same radius &speed. The law is the additive energy (gravitational plus the electric) is constant. This stability is controlled by the count of the additive spheres. As example if in the initial condition, the gravitational energy was about zero while the electric energy was hundred joule, and we have one giant proton in the orbit, then if this giant proton lost half of its charges then fifty percent (of the final condition) of the units would collect to maintain this initial condition and so on.
14. The Higher Giant State
The giant atom in its ground state is the smallest inversed giant atom whose giant proton can orbit its giant electron. The inversed atom is the mathematic basis of the giant atom. The created (or the unit) giant charge is the physical unit of the giant charge while the huge charge is the physical basis of the giant orbit. So the ground state is formed of (p) of the huge wave length (λu) or p2/n times λ
The higher levels (the complicated giant charge which represent the planets next to mercury planet) could be constructed by the same above basis. We suggest (recalling Equation (54)) that; the higher states of the complicated giant proton have number of protons = p∙d. Where d―in this chapter―means multiplication of (p) of the giant proton by a factor lies between 1 and 10 and so it obeys the relation:

Such that, the product p∙d means protons of the giant proton times d. We suggest for the complicated giant electron the factor D, where D―in this chapter―is a factor obeys the same relation as

Such that D means electrons evacuated giant electron (giant neutron).
From above we can estimate the ratio between mass of the complicated giant electron to that of the complicated giant proton = 1844.

In the giant atom (the ground state) 

In the complicated atom, the system allows that:
The number of the protons in the complicated giant proton = p1 while the electrons of the complicated giant electron = p2 such that:


The equations packet of the higher states:
The motion of the giant proton in a higher state is controlled by;
As a huge proton:
Its angular momentum obeys;

where 
The electric force gives

where 
We can notice that the mass of the huge proton in the complicated giant proton still equals;
As a giant proton:
The electric force gives

where the big bracket is its mass, (

15. The End of Compton State and Reality of the Particles Number
The matter density in condensate like nucleus and neutron star is about 5 × 1014 g/cc (with particle spacing about 2 × 10−15 m). In such high density β decay is forbidden because decay result in excess particles leading to decrease spacing between particles but neutron degenerate refuse―by Pauli’s exclusion―this decrease [14] [15] . Our condensate model differs from neutron star and consequently needs deep insight. First, we don’t now search for how such high density was formed. Let us begin―at least here―from the point of; if such sphere had exist (perhaps in early universe) then the study would only search in physics of such sphere. In the giant charge unit neutron decay is not forbidden but it has no chance. No chance because the time need for GCU to grow into Gc equal 
From the equality mentioned in Equation (21), the giant electron needs number of particles 1840 times as massive as the giant proton, hence, the latter orbits around the first. Hence, we can define the orbit length RS and the orbital speed vS.
In the stage of the neutron decay the familiar atoms are formed, consequently, the next results would be established:
1) rc and v inside the sphere S would vanish and the free charges escape from the sphere.
2) The electrostatic potential between the giant electron and its giant proton vanish.
3) The Newtonian gravitational potential would predominantly receive the file of the orbital motion to conserve RS & vS.
From 1, we can conclude the next results:
The relativistic number of the particles would vanish so, we return to 
The Newtonian gravitational potential of the solar system orbitals needs union of enough number of s (not S) to conserve RS & vS.
From all above, we conclude that we return again to the common physics which states that the charges quantity is conserved (generally, the sum of the baryon or lepton number of all incoming particles is the same as the sum of the baryon numbers of all particles resulting from the operation): we began with number of charges inside the sphere s equal p and then it became relativistic 

16. The Solar System
We know that the solar system is formed of nine planets rotate around the sun. The nearest one to the sun is mercury which has orbit radius = 

Our suggestion states that; each planet had formed (historically) of similar and homologous units. The number of the planet units could be concluded by dividing the planet mass over the mass of the giant proton. Each of these units had behaved as a giant proton. Each planet unit had a sun which had behaved as a giant electron.
The planet unit had rotated―on a base of quantum mechanics―around its sun unit forming a system of “giant atom”.
The old system had finished by union of all the sun units to form one “sun” and union of the identical planet units to form one planet (interference of the identical orbitals). So the different planet units had formed nine planets. So―now―we have one sun and nine planets. We also suggested for the solar system the following:
The units of the same planet had the same R & v therefore after beta decay they pulled to each other and collect in one body mass. Also do the electrons evacuated giant electrons. So we would have one planet orbits its special sun. The units of another higher state have another
Because of the radii of the orbits of the units of the different suns are not so away from each other comparative with the units of the different planets so they collect in one mass that is; one sun and nine planets. Mercury orbit represented the ground state of the solar system motion. The higher planets obey;
17. The Ratio “Ms ÷ ΣMp”
It is the ratio between mass of the sun and the summed masses of all planets.
Our estimation gave;
The astronomic ratio ≈ 600 where the sun natal mass = 2.13 × 1030 kg [11]
Therefore, there is error in our estimation = 1840 ÷ 600 = 3
So we suggest for dealing this error that; there were 3 orbiting giant protons for each giant electron as; (O, y, z), (x, O, z) & (x, y, O).

Three orbiting giant protons for each giant electron should make elliptical orbit (even in the ground state) and give spatial shape as if it was real giant atom.
Union of the three orbits (overlap of their waves) in the Newtonian stage may explain the final shape of the orbit.
18. The Giant Charge Interior
After we knew physics of Compton sphere and how it replicated till it becomes giant charge then this giant charge is the final macroscopic product. Its interior would forget the entire microscopic events and would remember only: the huge
charge (which is formed of one charge and 


gravitational energy per a huge charge so the huge charge is the one which describes this wavy behavior. The huge proton has a classic radius


huge wave hence the momentum p appears as expression of this wave as:
Anyway, each particle of the giant charge (the adult) appears with rest mass = ms and with zero energy except its ug which appears as the residual binding
energy of the system. So it has: 
between the brackets is the mass of the huge charge (notice that, = ms). The ratio

sphere are conserved. Equations (23) & (24) describe the density of energy of the particle inside the Compton’s sphere and also inside the giant charge. This form of the two equations guarantees that v never exceeds c; therefore the entire energies for a proton & a neutron inside the giant charge appear as

where: um is the magnetic energy subdivision. The motion inside the giant sphere is withdrawn and appears here, in the macroscopic version as rest energy except a bit of energy is still alive and represents the net residual energy of the system. The particles by this residual energy possess matter waves. We can estimate the wave length 
Diagrammatic Summary
1)
2) The intuit; 
sphere in a rest state →

3) Any other additional solution should be constrained by this intuit. This equation carries three results. The first is; the squared c does not mean real speed, so the particle is in a rest state without relativistic relations. The second is that; we succeeded to abort―physically―the repulsive electric energy. The third result appears if we wrote c in the next relativistic form;

(↓) (↓)
(Original relativity 

↓
Giant Charge Unit = Compton Sphere ←

4) On the same way, Equations (31)-(38) can abort the exclusive quantum energy due to the spacing between particles of the sphere.
The original relativity should be constrained by the above intuit → alternative relativistic solution;



Larmor’s equation problem:
It is clear that the circular (or the elliptical) orbit of the giant atom differs from the normal atom in which the electron is described by the wave function where it appears as cloud spread all over its standing wave (not orbiting in a circle otherwise it would radiate till finally fall in the nucleus). If we go to the mathematic derivation of Larmor’s equation and as approximation comparison with orbit of a hydrogen atom: if the number of the elementary charges in the giant atom = q and its orbit radius = R, and if A is a constant, and using J.J Thomson treatment with the suitable modification



Results:
1) The density of the giant charge defined by the spacing 
density of the Compton sphere defined by 
density of the micro-states in which the particles condensate & replicate by the relativistic alternative solution.
2) If we multiplied the electric subdivision―of the rest energy of the particle―by one, and if we factorized and analyzed this one―as Equations (5) & (6) and then followed up the relativistic micro-state of Equation (7) we would get the solar system as in Equations (79), (86) & (95).
3) We succeeded to fix the coulomb’s form of the electric energy on the rest energy form as in Equation (3). We also succeeded to do a junction between the classic & the quantum mechanics.
Conclusion:
The giant atom like system is a scientific truth. This is confirmed by what we found as complete coincidence between the parameters of such a system and that of the solar system. Such a system carries the scientific seeds of physics of self-replication.
Cite this paper
Eldieb, E. (2018) The Relativistic Giant Atom: Evidences and Recommendations. Open Access Library Journal, 5: e3797. https://doi.org/10.4236/oalib.1103797
References
- 1. Emad, E. (2017) Physics of the Relativistic Giant Atom. International Journal of Theoretical and Mathematical Physics, 7, 68-89.
- 2. Griffiths, D.J. (1995) Introduction to Quantum Mechanics. Prentice-Hall, Upper Saddle River, New Jersey, 155.
- 3. Garay, L.J. (1995) Quantum Gravity of Minimum Length. International Journal of Modern Physics, 10, 145.
https://doi.org/10.1142/S0217751X95000085 - 4. Bailey, D. (2011) Semi Empirical Nuclear Mass Formula: Strings and Binding Energy. PHY357: Strings & Binding Energy. University of Toronto.
- 5. Doolittle, A. (1982) Density of States and Fermi Energy Concept Alzergin and v. Korepin. Letter in Mathematical Physics, 6, 12-18.
- 6. Heisenberg, W. (1949) The Physical Prin-ciple of Quantum Theory. Dover Publications, Mineola, New York.
- 7. Regal, C.A., Greiner, M. and Jin, D.S. (2004) Fermi Energy. Physical Review Letters, 92, Article ID: 040403.
- 8. Golub, R. and Chordson, D.R. (1991) Ultracold Neutrons. Adam Hilger, Bristol.
- 9. Emad, E. (2015) The Electrostatic Energy in a Rest Form; Evidences and Recommendations. International Research Journal of Pure and Applied Physics, 3, 9-14.
- 10. Emad, E. (2009) The Giant Atom Like System. Advanced Studies in Theoretical Physics, 3, 14-15.
- 11. Kaufman, W.J. (1979) Planets and Moons. W. H. Freeman, San Francisco.
- 12. Simon, J.L. and Bretagnon, P. (1994) Numerical Expression for Formulae & Mean Elements for the Moon & Planets. Astronomy and Astrophysics, 282, 663-683.
- 13. Strom, R.G. and Sprague, A. (2003) Exploring Mercury: The Iron Planet. Praxis Public Ltd., Chichester.
- 14. Baym, G. (1995) Neutron Stars: Observing the Properties of High-Density Nuclear Matter. Nuclear Physics A, 590, 233.
https://doi.org/10.1016/0375-9474(95)00238-V - 15. Dwivedi, V. (2012) Condensates in Neutron Star Interiors. Phys 569: Emergent States of Matter.
http://guava.physics.uiuc.edu/~nigel/courses/569/Essays_Fall2012/Files/dwivedi.pdf - 16. Anderson, J.D., Colombo, G., Esposito, P.B., Lau, E.L., et al. (1987) The Mass Gravity Field and Ephemeris of Mercury. Icarus, 71, 337-349.
https://doi.org/10.1016/0019-1035(87)90033-9 - 17. Konopliv, A.S., Banerdt, W.B. and Sjogren, W.L. (1999) Venus Gravity: 180th Degree and Order Model. Icarus, 139, 3-18.
https://doi.org/10.1006/icar.1999.6086 - 18. Krane, K. (1988) Introductory Nuclear Physics. John Wily & Sons, Hoboken.
- 19. Turtur, C.W. (1985) An Explanation of Electron Mass by the Energy of Its Fields.
https://www2.ostfalia.de/export/sites/default/de/pws/turtur/FundE/English/electron_engl.pdf
Appendix
Actually Lorentz postulated that the intrinsic energy of the charged particle is due to its electromagnetic behavior [19] . So let us estimate the part of the electromagnetic energy due to the electric field and add it to that part due to the motion (spin) of the particle (magnetic energy). Now in each infinitesimal volume we have the electric energy density in the form of;
where ω is the permittivity constant. We would estimate the magnetic field B in z-direction (in S.I. units) postulating a literal circular motion of the charged particle with radius of rotation equals its classic radius


simple form:
where μ is the permeability constant of the space and i is the electric current of the charged particle as:
\The energy part due to spin 
Put 

And the total energy is estimated by adding the two parts of energy
If we applied the above mathematic manipulation in Equation (2) (the giant charge unit) then either in the macroscopic state (rest state) or the microscopic state (
where, 











































































































