Theoretical Economics Letters, 2011, 1, 70-72
doi:10.4236/tel.[phone] Published Online November 2011 (http://www.SciRP.org/journal/tel)
Compensation and the Twin Producer
Gains from Production Quotas
Troy G. Schmitz1, Andrew Schmitz2
1Morrison School of Agribusiness and Resource Man agement, Arizona State University, Mesa, Arizona
2Food and Resource Econom ics, IFAS, University of Florida, Gainesville, USA
Received June 21, 2011; revised August 12, 2011; accepted August 22, 2011
Abstract
The limited theory on production quotas focuses on the impact of introducing quotas when otherwise the
market would be competitive. We develop a model also on the effect of removing quotas, and then consider
the combined effects of both introducing and removing quotas. Under the value of quota approach, the
amount of money spent by the government for the buyout (i.e., value of quota) is equal to the sum of the net
gain to producers when the quota was introduced plus the net gain if it were removed. Compensation is the
key to a quota buyout, as producers have little interest in politically supporting a government compensated
buyout unless they gain by so doing.
Keywords: Compensation, Producer Gains, Production Quotas
1. Introduction
Theoretical studies on production quotas, such as those
by Wallace [1]; Johnson [2]; Rucker, Thurman, and Sum-
ner [3]; and Just, Hueth, and Schmitz [4], focus on the
impact of production quotas compared to a competetive
situation. In addition, Schmitz and Schmitz [5] ana-
lyzed the impacts of removing production quotas with an
empirical application to the removal of the U.S. produc-
tion quota for peanuts. They found that farmer compen-
sation for the buyout was based on the value of quota
approach. Farmers will lobby for the method of payment
that leads to their greatest benefit. Successful political
rent-seeking behavior by farmers will lead to an outcome
from a buyout that favors farmers relative to taxpayers
(Vercammen and Schmitz [6]; Schmitz, Furtan, and Bay-
lis [7]; Schmitz and Schmitz [5]). In this paper, we con-
sider the twin producer gains from both the introduction
and removal of quotas. Under one approach to compen-
sation, producers gain from both the implementation of a
quota and its removal. Under the value of quota approach,
for example, the value of quota at the time of removal
exactly equals the twin producer gains from the quota
(i.e., the gain from introducing the quota plus the gain
from removing it). Quotas when implemented can have a
much larger impact on the welfare of producers than
when they are removed, even though gains are present
when quotas are introduced and are present when they
are removed. In addition, consumers generally have
much more to gain from a production quota buyout than
do producers.
2. Theoretical Model
We present the theoretical discussion of the impact of
production quotas on 1) producer welfare when quotas
are introduced; 2) the effect of removing quotas; and 3)
the combined impact of both introducing and removing
quotas. In our analysis, we consider two periods where in
period 1 the quotas are introduced and in period 2 they
are removed.
2.1. Period 1
Consider Figure 1 where S is the supply schedule for
good x and D is the domestic demand. In the absence of a
quota, the competitive price is p0 and the corresponding
output is q0. Suppose we introduce a production quota
that restricts output to q1: price increases from p0 to p1.
As a result, producers gain (p1p0da – dcb)—a result often
ignored in the discussion of production quotas. Producer
welfare results are derived by comparing the competitive
equilibrium price p0 and quantity q0 with the quota price
p1 and quantity q1.

71 T. G. SCHMITZ ET AL.
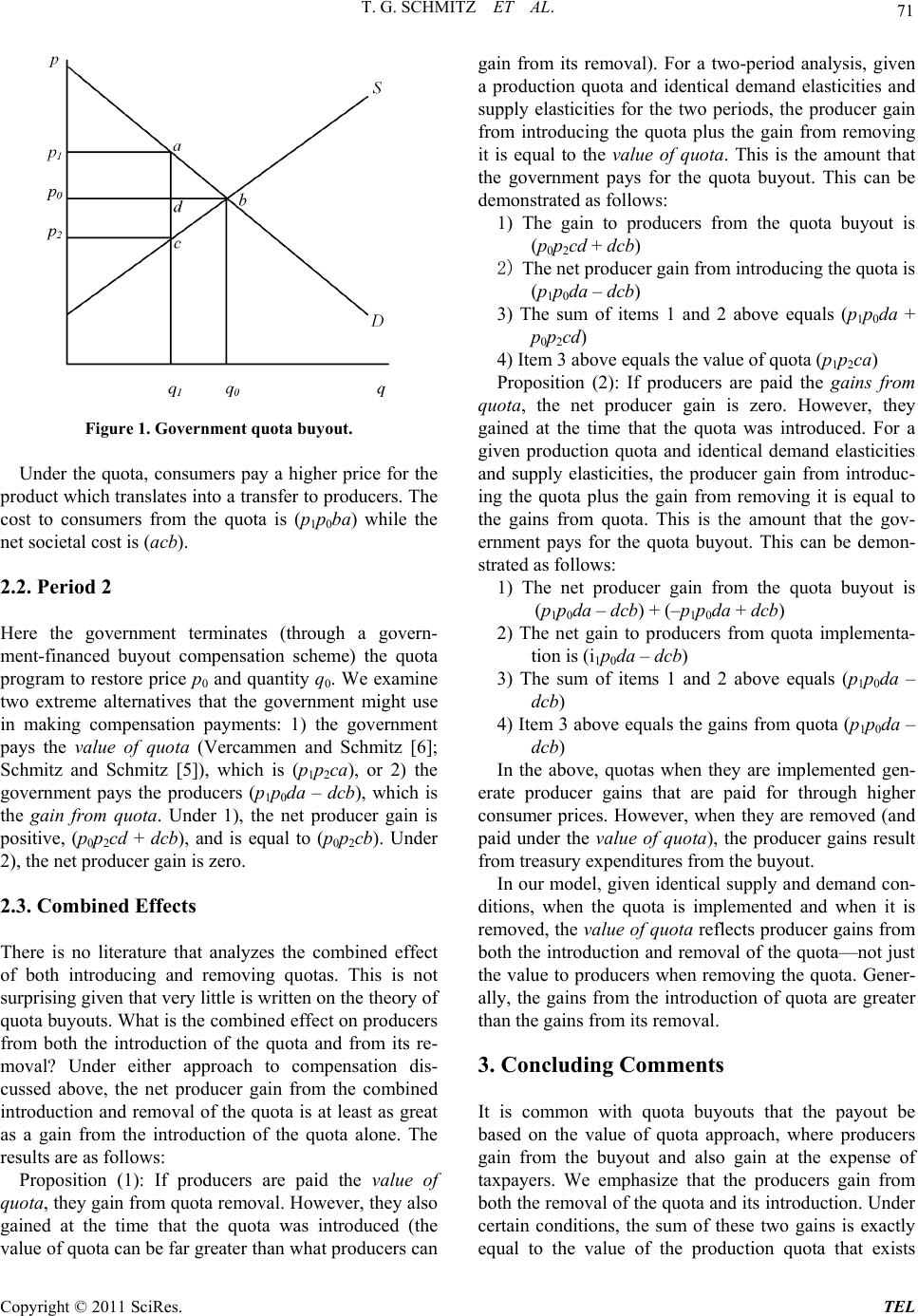
Figure 1. Government quota buyout.
Under the quota, consumers pay a higher price for the
product which translates into a transfer to producers. The
cost to consumers from the quota is (p1p0ba) while the
net societal cost is (acb).
2.2. Period 2
Here the government terminates (through a govern-
ment-financed buyout compensation scheme) the quota
program to restore price p0 and quantity q0. W e examine
two extreme alternatives that the government might use
in making compensation payments: 1) the government
pays the value of quota (Vercammen and Schmitz [6];
Schmitz and Schmitz [5]), which is (p1p2ca), or 2) the
government pays the producers (p1p0da – dcb), which is
the gain from quota. Under 1), the net producer gain is
positive, (p0p2cd + dcb), and is equal to (p0p2cb). Under
2), the net producer gain is zero.
2.3. Combined Effects
There is no literature that analyzes the combined effect
of both introducing and removing quotas. This is not
surprising given that very little is written on th e theory of
quota buyouts. What is the combined effect on producers
from both the introduction of the quota and from its re-
moval? Under either approach to compensation dis-
cussed above, the net producer gain from the combined
introduction and removal of the quota is at least as great
as a gain from the introduction of the quota alone. The
results are as follows:
Proposition (1): If producers are paid the value of
quota, they gain from quota removal. However, they also
gained at the time that the quota was introduced (the
value of quota can be far greater than what produ cers can
gain from its removal). For a two-period analysis, given
a production quota and identical demand elasticities and
supply elasticities for the two periods, the producer gain
from introducing the quota plus the gain from removing
it is equal to the value of quota. This is the amount that
the government pays for the quota buyout. This can be
demonstrated as follows:
1) The gain to producers from the quota buyout is
(p0p2cd + dcb)
2) The net producer gain from introducing the quota is
(p1p0da – dcb)
3) The sum of items 1 and 2 above equals (p1p0da +
p0p2cd)
4) Item 3 above equals the value of quota (p1p2ca)
Proposition (2): If producers are paid the gains from
quota, the net producer gain is zero. However, they
gained at the time that the quota was introduced. For a
given production quota and identical demand elasticities
and supply elasticities, the producer gain from introduc-
ing the quota plus the gain from removing it is equal to
the gains from quota. This is the amount that the gov-
ernment pays for the quota buyout. This can be demon-
strated as follows:
1) The net producer gain from the quota buyout is
(p1p0da – dcb) + (–p1p0da + dcb)
2) The net gain to producers from quota implementa-
tion is (i1p0da – dcb)
3) The sum of items 1 and 2 above equals (p1p0da –
dcb)
4) Item 3 above equals the gains from quota (p1p0da –
dcb)
In the above, quotas when they are implemented gen-
erate producer gains that are paid for through higher
consumer prices. However, when they are removed (and
paid under the value of quota), the producer gains result
from treasury expenditures from the buyout.
In our model, given identical supply and demand con-
ditions, when the quota is implemented and when it is
removed, the value of quota reflects producer gains from
both the introduction and removal of the quota—not just
the value to producers when removing the quota. Gener-
ally, the gains from the introduction of quota are greater
than the gains from its removal.
3. Concluding Comments
It is common with quota buyouts that the payout be
based on the value of quota approach, where producers
gain from the buyout and also gain at the expense of
taxpayers. We emphasize that the producers gain from
both the removal of the quota and its introd uction. Under
certain conditions, the sum of these two gains is exactly
equal to the value of the production quota that exists

T. G. SCHMITZ ET AL.
72
when the quota is removed. Quota values are not a mea-
sure of the net gain to producers from a quota buyout
even though many policy analysts continue to believe so.
When assessing the magnitude of compensation, it is
important to consider both the introduction and removal
of quotas, otherwise one is left with the wrong impress-
sion of the size of the payout based on the value of the
quota. When considering both of the components, the
size of the compensation can be large. But, this result is
not surprising in view of the many packages that have
been put together to compensate losers from a change in
policy (Schmitz and Zerbe [8]).
4. References
[1] T. D. Wallace, “Measures of Social Costs of Agricultural
Programs,” Journal of Farm Economics, Vol. 44, No. 2,
1962, pp. 580-599. doi:10.2307/1235863
[2] P. R. Johnson, “The Social Cost of the Tobacco Pro-
gram,” Journal of Farm Economics, Vol. 47, No. 2, 1965,
pp. 242-255. doi:10.2307/1236572
[3] R. Rucker, W. Thurman and D. Sumner, “Restricting the
Market for Quota: An Analysis of Tobacco Production
Rights with Corroboration from Congressional Testimo-
ny,” Journal of Political Economy, Vol. 103, No. 1, 1995,
pp. 142-175. doi:10.1086/261979
[4] R. E. Just, D. L. Hueth and A. Schmitz, “The Welfare
Economics of Public Policy: A Practical Approach to
Project and Policy Evaluation,” Edward Elgar Publishing,
Cheltenham, 2004.
[5] A. Schmitz and T. G. Schmitz, “Benefit-Cost Analysis:
Distributional Considerations under Producer Quota Bu-
youts,” Journal of Benefit-Cost Analysis, Vol. 1, No. 1,
2010, Article 2.
[6] J. A. Vercammen and A. Schmitz, “Supply Management
and Import Concessions,” Canadian Journal of Econom-
ics, Vol. 25, No. 4, 1992, pp. 957-971.
doi:10.2307/135774
[7] A. Schmitz, H. Furtan and K. Baylis, “Agriculture Policy,
Agribusiness, and Rent-Seeking Behaviour,” University
of Toronto Press, Toronto, 2002.
[8] A. Schmitz and R. O. Zerbe Jr., “Applied Benefit-Cost
Analysis,” Edward Elgar Publishing, Cheltenham, 2008.