P. BOCCOTTI
70
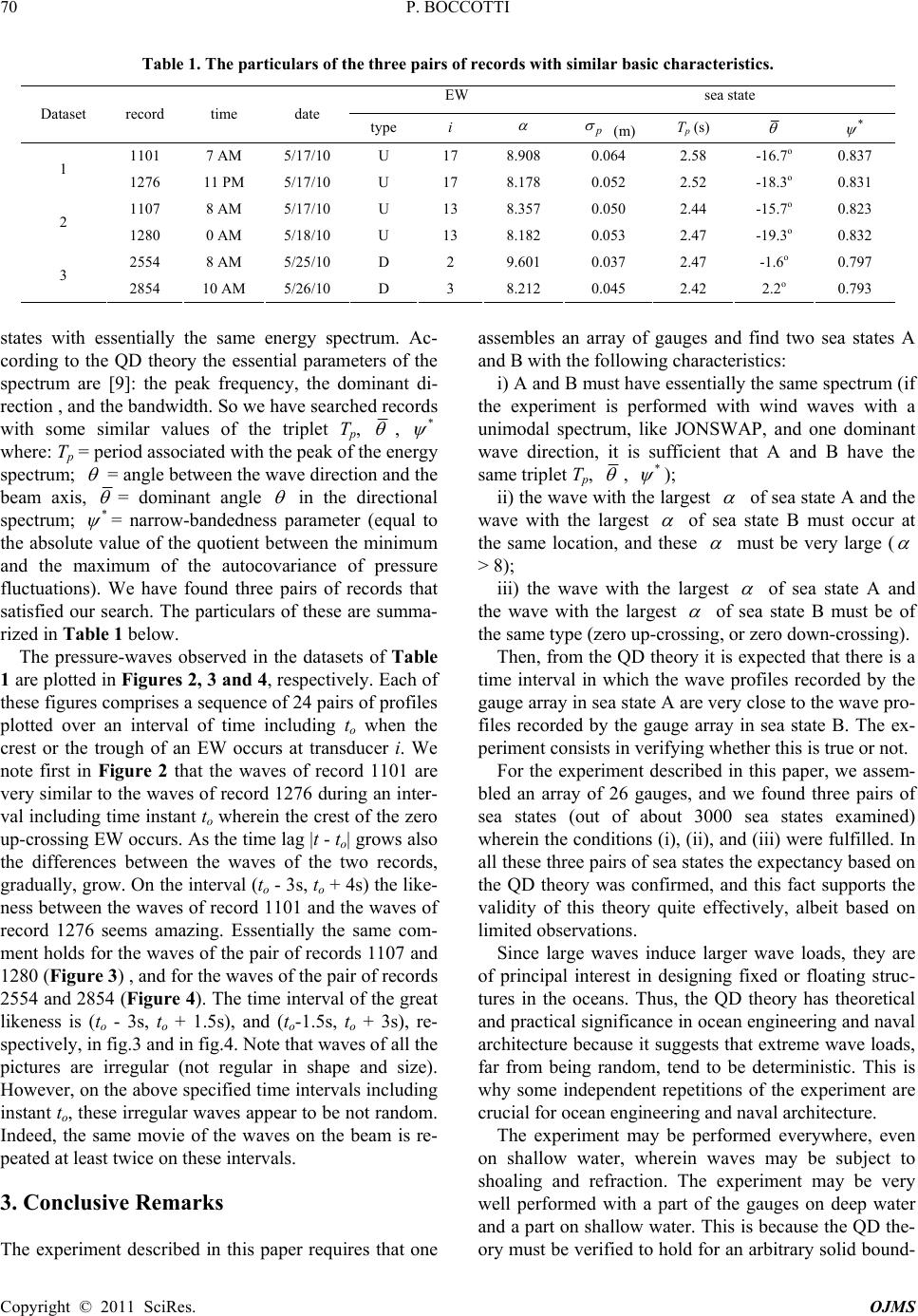
Table 1. The particulars of the three pairs of records with similar basic characteristics.
EW sea state
Dataset record time date
type i
(m)
T
p
(s)
*
1101 7 AM 5/17/10 U 17 8.908 0.064 2.58 -16.7
o
0.837
1 1276 11 PM 5/17/10 U 17 8.178 0.052 2.52 -18.3
o
0.831
1107 8 AM 5/17/10 U 13 8.357 0.050 2.44 -15.7
o
0.823
2 1280 0 AM 5/18/10 U 13 8.182 0.053 2.47 -19.3
o
0.832
2554 8 AM 5/25/10 D 2 9.601 0.037 2.47 -1.6
o
0.797
3 2854 10 AM 5/26/10 D 3 8.212 0.045 2.42 2.2
o
0.793
states with essentially the same energy spectrum. Ac-
cording to the QD theory the essential parameters of the
spectrum are [9]: the peak frequency, the dominant di-
rection , and the bandwidth. So we have searched records
with some similar values of the triplet Tp,
,
*
where: Tp = period associated with the peak of the energy
spectrum;
= angle between the wave direction and the
beam axis,
= dominant angle
in the directional
spectrum;
*
= narrow-bandedness parameter (equal to
the absolute value of the quotient between the minimum
and the maximum of the autocovariance of pressure
fluctuations). We have found three pairs of records that
satisfied our search. The particulars of these are summa-
rized in Table 1 below.
The pressure-waves observed in the datasets of Table
1 are plotted in Figures 2, 3 and 4, respectively. Each of
these figures comprises a sequence of 24 pairs of profiles
plotted over an interval of time including to when the
crest or the trough of an EW occurs at transducer i. We
note first in Figure 2 that the waves of record 1101 are
very similar to the waves of record 1276 during an inter-
val including time instant to wherein the crest of the zero
up-crossing EW occurs. As the time lag |t - to| grows also
the differences between the waves of the two records,
gradually, grow. On the interval (to - 3s, to + 4s) the like-
ness between the waves of record 1101 and the waves of
record 1276 seems amazing. Essentially the same com-
ment holds for the waves of the pair of records 1107 and
1280 (Figure 3) , and for the waves of the pair of records
2554 and 2854 (Figure 4). The time interval of the great
likeness is (to - 3s, to + 1.5s), and (to-1.5s, to + 3s), re-
spectively, in fig.3 and in fig.4. Note that waves of all the
pictures are irregular (not regular in shape and size).
However, on the above specified time intervals including
instant to, these irregular waves appear to be not random.
Indeed, the same movie of the waves on the beam is re-
peated at least twice on these intervals.
3. Conclusive Remarks
The experiment described in this paper requires that one
assembles an array of gauges and find two sea states A
and B with the following characteristics:
i) A and B must have essentially the same spectrum (if
the experiment is performed with wind waves with a
unimodal spectrum, like JONSWAP, and one dominant
wave direction, it is sufficient that A and B have the
same triplet Tp,
,
*
);
ii) the wave with the largest
of sea state A and the
wave with the largest
of sea state B must occur at
the same location, and these
must be very large (
> 8);
iii) the wave with the largest
of sea state A and
the wave with the largest
of sea state B must be of
the same type (zero up-crossing, or zero down-crossing).
Then, from the QD theory it is expected that there is a
time interval in which the wave profiles recorded by the
gauge array in sea state A are very close to the wave pro-
files recorded by the gauge array in sea state B. The ex-
periment consists in verifying whether this is true or not.
For the experiment described in this paper, we assem-
bled an array of 26 gauges, and we found three pairs of
sea states (out of about 3000 sea states examined)
wherein the conditions (i), (ii), and (iii) were fulfilled. In
all these three pairs of sea states the expectancy based on
the QD theory was confirmed, and this fact supports the
validity of this theory quite effectively, albeit based on
limited observations.
Since large waves induce larger wave loads, they are
of principal interest in designing fixed or floating struc-
tures in the oceans. Thus, the QD theory has theoretical
and practical significance in ocean engineering and naval
architecture because it suggests that extreme wave loads,
far from being random, tend to be deterministic. This is
why some independent repetitions of the experiment are
crucial for ocean engineering and naval architecture.
The experiment may be performed everywhere, even
on shallow water, wherein waves may be subject to
shoaling and refraction. The experiment may be very
well performed with a part of the gauges on deep water
and a part on shallow water. This is because the QD the-
ory must be verified to hold for an arbitrary solid bound-
Copyright © 2011 SciRes. OJMS