Journal of Modern Physics
Vol.07 No.14(2016), Article ID:71561,5 pages
10.4236/jmp.2016.714171
The Golden Ratio
Csizmadia Jozsef
Inovator plus Ltd. Kanjiza, Kanjiza, Serbia

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: August 22, 2016; Accepted: October 23, 2016; Published: October 27, 2016
ABSTRACT
The Lorentz transformation (if x = ct) is the same the golden ratio: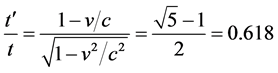 .
.
Keywords:
Lorentz Transformation, Relativity, Golden Ratio

1. Introduction
So, the aim of this work is to derive the golden ratio connected to Lorentz trans- formation. Usually the golden ratio is derived from the a rectangle [1] but book [2] derives the golden ratio from a right-angled triangle. This makes possible to connect Lorentz transformation with the golden ratio. The Lorentz factor formula can be the easiest explained from the right-angled triangle. The formula 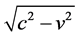 is in fact the formula of one of the right-angled triangle side:
is in fact the formula of one of the right-angled triangle side:
1) 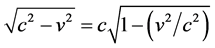
It can be seen from this formula also that “c” is the hypotenuse of the right-angled triangle. At the same time “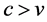 ” is one criterion of Lorentz factor formula as well. That means, that the hypotenuse of the right-angled triangle is “c”, the other sides is “v” and
” is one criterion of Lorentz factor formula as well. That means, that the hypotenuse of the right-angled triangle is “c”, the other sides is “v” and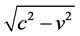 . This allows that from so defined right-angled triangle to explain the formula of Lorentz transformation too and the golden ratio formula as well. For the derivation of the equations high school mathematics is used. That’s the reason why the equations are more transparent and easier to understand because they are simply.
. This allows that from so defined right-angled triangle to explain the formula of Lorentz transformation too and the golden ratio formula as well. For the derivation of the equations high school mathematics is used. That’s the reason why the equations are more transparent and easier to understand because they are simply.
2. We Introduce First the (t'/t) Formula
The derivation of Lorentz transformation can be found at the end of the book marked by [3] in the Appendix I. The derivation of Lorentz transformation equations starts from the fact that we measure two light-rays move face each other, in a standing and in a moving coordinate system. So the travelled distance of the light-ray in the standing coordinate system is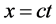 . Naturally in the movable coordinate system the travelled distance of the light-ray is
. Naturally in the movable coordinate system the travelled distance of the light-ray is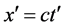 . Here it can be seen that Lorentz’s starting point were a few assumptions, (see [3] Appendix I) and from these simple assumptions got the formula named after him. After that Einstein substituted in the Lorentz trans- formation equation
. Here it can be seen that Lorentz’s starting point were a few assumptions, (see [3] Appendix I) and from these simple assumptions got the formula named after him. After that Einstein substituted in the Lorentz trans- formation equation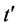 , the equation of
, the equation of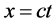 . This derivation can be found in the book marked number [3] on the page 32. Now I show this derivation.
. This derivation can be found in the book marked number [3] on the page 32. Now I show this derivation.
2) So, 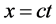 and
and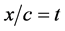 :
:

3) Equation (t'/t):
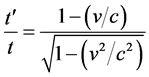
We are going to prove that the equation 3) is the golden ratio.
2.1. Now We Derive the Other Form of the Equation (3)
Before we start with the derivation of the golden ratio, we transform the Equation (3).
4) In further derivation we shall use the following equations from the Appendix I of the book [3] :
5) In further derivation we shall use the following equations from the [4] :
(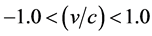 See the Figure 1).
See the Figure 1).
6) From the [4] we take also the following equations:
7) We shall transform the Equation (3) and take the Equation (4) and Equation(5) and Equation (6) into account:
8) So:
The Equation (8) can be found in [5] .
Figure 1. The Equation (8).
2.2. Derivation of the Golden Ratio
We mentioned in the introduction, the formula of golden ratio, we are going to derive it from a right-angled triangle [2] . (Note: Figure 2 can be found in [2] ).
9) See Figure 2:
10) The golden ratio:
11) See Equation (9):
12) Now we transform the Equation (10) so that it should be equal with Equation (8).We will take into account that:
13) Of course, the Equation (12) is identical with Equation (8):
Figure 2. In the book [2] the golden ratio = OE/OA, and
3. Conclusion
It can be seen in the Equation (13) that 
14) The Equation (13):
15) So:
4. Discussion
Naturally the golden ratio often appears in physics. Hardy suggest to use the golden ratio (See E. Naschi work, references [6] . Lorentz transformation equation is the same as suggested by Hardy. It would be good to prove in other way the connection of two equations. That’s the reason why the two equations are not unambiguous:
16) The Hardy equation [6] , and the Equation (13):
17) But still it is interesting the Equation (11) trivial solution:
Cite this paper
Jozsef, C. (2016) The Golden Ratio. Journal of Modern Phy- sics, 7, 1944-1948. http://dx.doi.org/10.4236/jmp.2016.714171
References
- 1. Golden Ratio.
https://en.wikipedia.org/wiki/Golden_ratio - 2. Bronstejn I.N. and Szemengyajev, K.A. (1982) Matematikai zsebkonyv. Translate: Mathematical Pocketbook. Muszaki konyvkiado, Budapest.
- 3. Einstein, A. (1921) Relativity: The Special and the General Theory. METHUEN and CO. LTD., London.
http://www.gutenberg.lib.md.us/3/6/1/1/36114/36114-pdf.pdf - 4. https://en.wikipedia.org/wiki/Lorentz_transformation
- 5. Trasformazione di Lorentz.
https://it.wikipedia.org/wiki/Trasformazione_di_Lorentz - 6. El Naschie, M. (2016) Journal of Modern Physics, 7, 1420-1428.
http://dx.doi.org/10.4236/jmp.2016.712129




















