Applied Mathematics
Vol. 3 No. 7 (2012) , Article ID: 20012 , 4 pages DOI:10.4236/am.2012.37108
New Unique Common Fixed Point Results for Four Mappings with Ф-Contractive Type in 2-Metric Spaces*
Department of Mathematics, College of Science, Yanbian University, Yanji, China
Email: pyj6216@hotmail.com
Received May 15, 2012; revised June 15, 2012; accepted June 22, 2012
Keywords: 2-Metric Space; Class Ф; Cauchy Sequence; Coincidence Point; Unique Common Fixed Point
ABSTRACT
In this paper, some new unique common fixed points for four mappings satisfying Ф-contractive conditions on noncomplete 2-metric spaces are obtained, in which the mappings do not satisfy continuity and commutation. The main results generalize and improve many well-known and corresponding conclusions in the literatures.
1. Introduction
There have appeared many unique common fixed point theorems of mappings with some contractive condition on 2-metric spaces. But most of them held under subsidiary conditions [1-3], for examples: commutativity of mappings or uniform boundness of mappings at some point, and so on. In [4-8], the author obtained similar results for infinite mappings with generalized contractive or quasi-contractive conditions under removing the above subsidiary conditions. These results generalized and improved many same type unique common fixed point theorems.
In this paper, by introducing a new class Ф, we will discuss the existence problem of unique common fixed points for four mappings with Ф-contractive type on noncomplete 2-metric spaces without any subsidiary conditions. The obtained main results in this paper further generalize and improve the corresponding results.
Here, we give some well known concepts and results.
Definition 1.1. ([4]) A 2-metric space  consists of a nonempty set X and a function
consists of a nonempty set X and a function 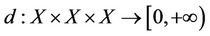 such that
such that
1) for distant elements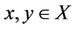 , there exists an
, there exists an 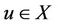 such that
such that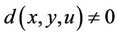 ;
;
2) 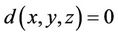 if and only if at least two elements in
if and only if at least two elements in 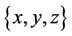 are equal;
are equal;
3)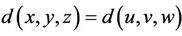 , where
, where 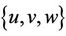 is any permutation of
is any permutation of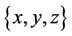 ;
;
4) 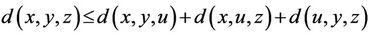 for all
for all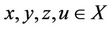 .
.
Definition 1.2. ([4]) A sequence 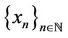 in 2-metric space
in 2-metric space 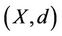 is said to be cauchy sequence, if for each
is said to be cauchy sequence, if for each 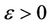 there exists a positive integer
there exists a positive integer 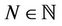 such that
such that 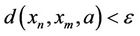 for all
for all 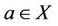 and
and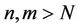 .
.
Definition 1.3. ([4,5]) A sequence 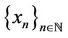 is said to be convergent to
is said to be convergent to , if for each
, if for each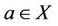 ,
,
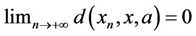 .
.
And write 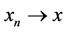 and call x the limit of
and call x the limit of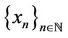 .
.
Definition 1.4. ([4,5]) A 2-metric space 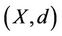 is said to be complete, if every cauchy sequence in X is convergent.
is said to be complete, if every cauchy sequence in X is convergent.
Definition 1.5. ([9,10]) Let f and g be self-maps on a set X. If 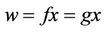 for some
for some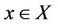 , then x is called a coincidence point of f and g, and w is called a point of coincidence of f and g.
, then x is called a coincidence point of f and g, and w is called a point of coincidence of f and g.
Definition 1.6. ([11]) Two mappings 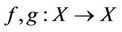 are weakly compatible if, for every
are weakly compatible if, for every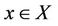 , holds fgx = gfx whenever
, holds fgx = gfx whenever 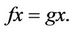
Lemma 1.7. ([6-8]) Let 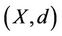 be a 2-metric space and
be a 2-metric space and 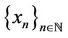 a sequence. If there exists
a sequence. If there exists 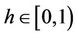 such that
such that 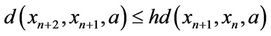 for all
for all 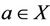 and
and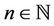 , then
, then 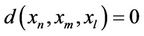 for all
for all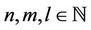 , and
, and
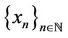 is a cauchy sequence.
is a cauchy sequence.
Lemma 1.8. ([6-8]) If  is a 2-metric space and sequence
is a 2-metric space and sequence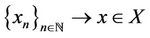 , then
, then
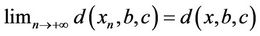
for each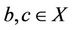 .
.
Lemma 1.9. ([9,10]) Let 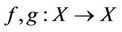 be weakly compatible. If f and g have a unique point of coincidence
be weakly compatible. If f and g have a unique point of coincidence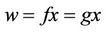 , then w is the unique common fixed point of f and g.
, then w is the unique common fixed point of f and g.
2. Main Results
Denote Ф the set of functions 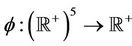 satisfying the following conditions:
satisfying the following conditions: 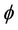 is continuous and increasing in each coordinate variable, and
is continuous and increasing in each coordinate variable, and  and
and  for all
for all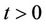 .
.
Examples Let 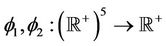 be defined by
be defined by
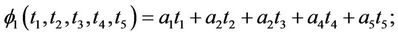
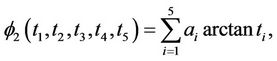
where 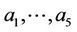 are non-negative real numbers satisfying
are non-negative real numbers satisfying
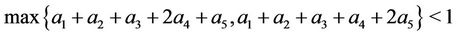 .
.
Then obviously,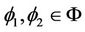 .
.
The following theorem is the main result in this present paper.
Theorem 2.1. Let 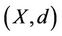 be a 2-metric space, S, T, I,
be a 2-metric space, S, T, I,  four single valued mappings satisfying that
four single valued mappings satisfying that 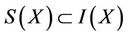 and
and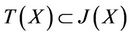 . Suppose that for each
. Suppose that for each ,
,
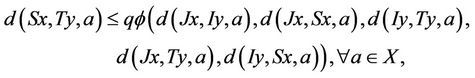 (1)
(1)
where 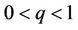 and
and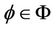 . If one of
. If one of 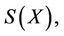
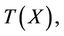
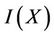 and
and 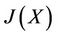 is complete, then T and I, S and J have an unique point of coincidence in X. Further,
is complete, then T and I, S and J have an unique point of coincidence in X. Further, 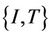 and
and  are weakly compatible respectively, then S, T, I, J have an unique common fixed point in X.
are weakly compatible respectively, then S, T, I, J have an unique common fixed point in X.
Proof Take any element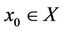 , then in view of the conditions
, then in view of the conditions 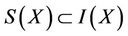 and
and , we can construct two sequences
, we can construct two sequences 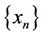 and
and 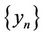 as follows:
as follows:
 (2)
(2)
For any 
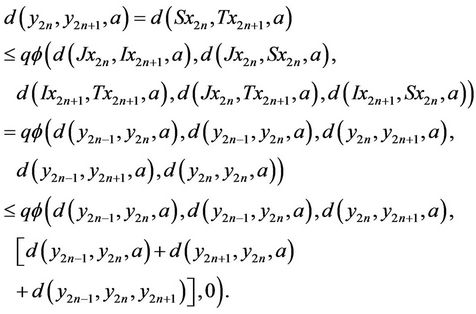 (3)
(3)
If , then by (1) and Ф, we have that
, then by (1) and Ф, we have that
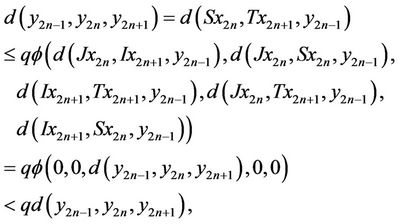 (4)
(4)
which is a contradiction since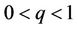 , hence
, hence 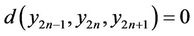 . And therefore, (3) becomes that
. And therefore, (3) becomes that
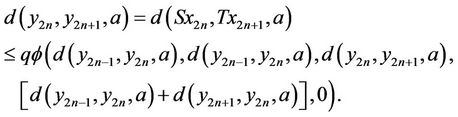 (5)
(5)
If there exists an 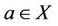 such that
such that
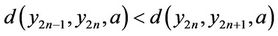 , then (5) becomes
, then (5) becomes
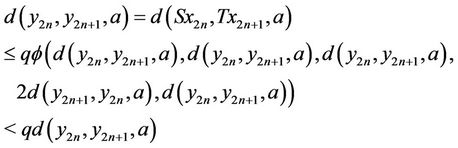
which is a contradiction since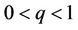 , hence we have that
, hence we have that 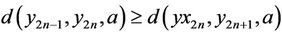 for all
for all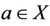 . So by (5) and Ф, we obtain that
. So by (5) and Ф, we obtain that
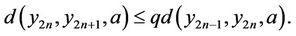 (6)
(6)
Similarly, we can prove that
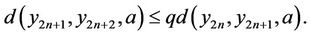 (7)
(7)
Hence we have that
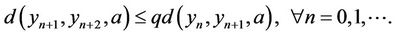 (8)
(8)
So 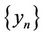 is a Cauchy sequence by Lemma 1.7.
is a Cauchy sequence by Lemma 1.7.
Suppose that  is complete, then there exists
is complete, then there exists  and
and 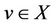 such that
such that
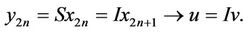 (If
(If 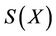 is complete, there exists
is complete, there exists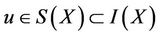 , then the conclusions remains the same.)
, then the conclusions remains the same.)
Since
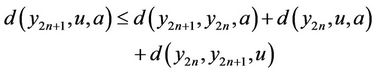
and 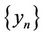 is Cauchy sequence and
is Cauchy sequence and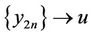 , we obtain that
, we obtain that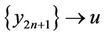 .
.
For any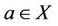 ,
,
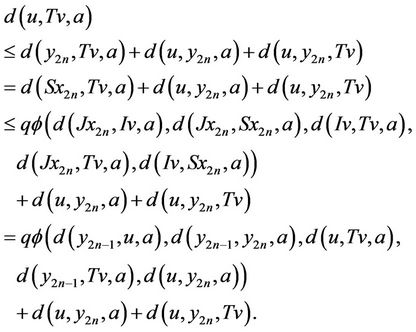
Let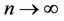 , then the above becomes
, then the above becomes
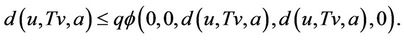
If  for some
for some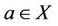 , then we obtain that
, then we obtain that
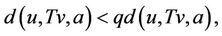
which is a contradiction since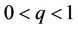 . Hence
. Hence 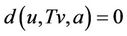 for all
for all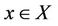 , so
, so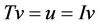 , i.e., u is a point of coincidence of T and I, and v is a coincidence point of T and I.
, i.e., u is a point of coincidence of T and I, and v is a coincidence point of T and I.
On the other hand, since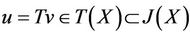 , there exists
, there exists 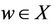 such that
such that 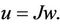 By (1), for any
By (1), for any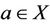 ,
,
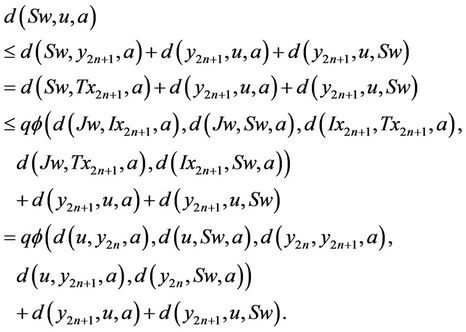
Let , then we obtain that
, then we obtain that
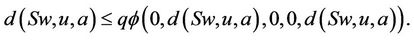
If 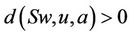 for some
for some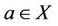 , then the above becomes that
, then the above becomes that
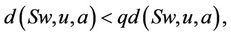
which is a contradiction since 0 < q < 1, so 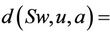 for all
for all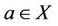 . Hence
. Hence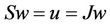 , i.e., u is a point of coincidence of S and J, and w is a coincidence point of S and J.
, i.e., u is a point of coincidence of S and J, and w is a coincidence point of S and J.
If 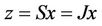 is another point of coincidence of S and J, then there exists
is another point of coincidence of S and J, then there exists 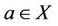 such that
such that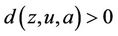 , and we have that
, and we have that
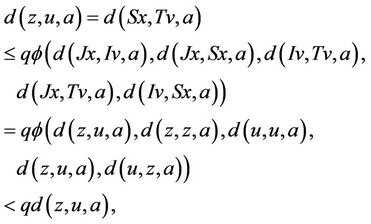
which is a contradiction. So  for all
for all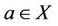 , hence
, hence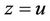 , i.e., u is the unique point of coincidence of S and J. Similarly, we can prove that u is also unique point of coincidence of T and I.
, i.e., u is the unique point of coincidence of S and J. Similarly, we can prove that u is also unique point of coincidence of T and I.
By Lemma 1.9, u is the unique common fixed point 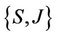 and
and  respectively, hence u is the unique common fixed point of S, T, I, J.
respectively, hence u is the unique common fixed point of S, T, I, J.
If 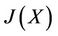 or
or 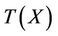 is complete, then we can also use similar method to prove the same conclusion. We omit the part.
is complete, then we can also use similar method to prove the same conclusion. We omit the part.
Here, we give only one of particular forms of theorem 2.1, which itself also generalize and improve many known results.
Theorem 2.2. Let 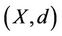 be a 2-metric space, S, T, I,
be a 2-metric space, S, T, I, 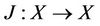 four single valued mappings satisfying that
four single valued mappings satisfying that 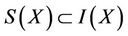 and
and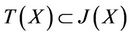 . Suppose that for each
. Suppose that for each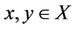 ,
,
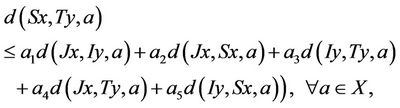
where 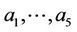 are non-negative real numbers satisfying
are non-negative real numbers satisfying
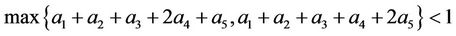 .
.
If one of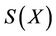 ,
, 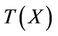 ,
, 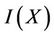 and
and 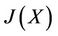 is complete, then T and I, S and J have an unique point of coincidence in X. Further,
is complete, then T and I, S and J have an unique point of coincidence in X. Further,  and
and 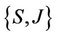 are weakly compatible respectively, then S, T, I, J have an unique common fixed point in X.
are weakly compatible respectively, then S, T, I, J have an unique common fixed point in X.
Proof Take 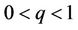 satisfying
satisfying
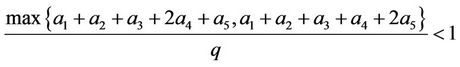
and let
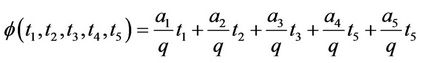 .
.
Then obviously,  , hence q and
, hence q and 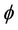 satisfy all conditions of Theorem 2.1, so the conclusion follows from theorem 2.1 Using Theorem 2.1, we give the following contractive or quasi-contractive versions of Theorem 2.1 for two mappings.
satisfy all conditions of Theorem 2.1, so the conclusion follows from theorem 2.1 Using Theorem 2.1, we give the following contractive or quasi-contractive versions of Theorem 2.1 for two mappings.
Corollary 2.3 Let 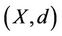 be a 2-metric space,
be a 2-metric space,  two single valued mappings satisfying that for each
two single valued mappings satisfying that for each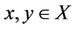 ,
,
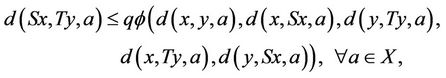
where 0 < q < 1 and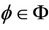 . If one of
. If one of 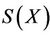 and
and 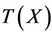 is complete, then T and S have an unique common fixed point in X.
is complete, then T and S have an unique common fixed point in X.
Proof Let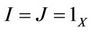 , then by Theorem 2.1, there exist
, then by Theorem 2.1, there exist  such that u is the unique point of coincidence of S and J. But obviously S and J are weakly compatible, so u is the unique fixed point of S by Lemma 1.9. Similarly, u is also unique fixed point of T, hence u is the unique common fixed point of S and T.
such that u is the unique point of coincidence of S and J. But obviously S and J are weakly compatible, so u is the unique fixed point of S by Lemma 1.9. Similarly, u is also unique fixed point of T, hence u is the unique common fixed point of S and T.
Corollary 2.4 Let 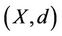 be a complete 2-metric space,
be a complete 2-metric space, 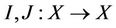 two single valued surjective mappings satisfying for each
two single valued surjective mappings satisfying for each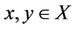 ,
,
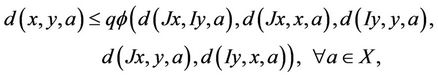
where 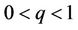 and
and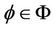 , then I and J have an unique common fixed point in X.
, then I and J have an unique common fixed point in X.
Proof Let , then by Theorem 2.1, there exist
, then by Theorem 2.1, there exist 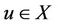 such that u is the unique point of coincidence of S and J. But obviously S and J are weakly compatible, so u is the unique fixed point of J by Lemma 1.9. Similarly, u is also unique fixed point of I, hence u is the unique common fixed point of I and J.
such that u is the unique point of coincidence of S and J. But obviously S and J are weakly compatible, so u is the unique fixed point of J by Lemma 1.9. Similarly, u is also unique fixed point of I, hence u is the unique common fixed point of I and J.
REFERENCES
- Y. J. Piao, G. Z. Jin and B. J. Zhang, “A Family of Selfmaps Having an Unique Common Fixed Point in 2-Metric Spaces,” Journal of Yanbian University (Science Edition), Vol. 28, No. 1, 2002, pp. 1-5.
- H. S. Yang and D. S. Xiong, “A Common Fixed Point Theorem on p-Metric Spaces,” Journal of Yunnan Normal University (Science Edition), Vol. 21, No. 1, 2001, pp. 9-12.
- S. L. Singh,, “Some Contractive Type Principles on 2- Metric Spaces and Applications,” Mathematics Seminar Notes (Kobe University), Vol. 7, No. 1, 1979, pp. 1-11.
- Y. J. Piao and Y. F. Jin, “Unique Common Fixed Point Theorem for a Family of Contractive Type Non-Commuting Selfmaps in 2-Metric Spaces,” Journal of Yanbian University (Science Edition), Vol. 32, No. 1, 2006, pp. 1-3 (in Chinese).
- Y. J. Piao, “A Family of Quasi-Contractive Type NonCommutative Self-Maps Having an Unique Common Fixed Point in 2-Metric Spaces,” Journal of Heilongjiang University (Science Edition), Vol. 23, No. 5, 2006, pp. 655-657 (in Chinese).
- Y. J. Piao, “Unique Common Fixed Point for a Family of Self-Maps with Same Type Contractive Condition in 2- Metric Spaces,” Analysis in Theory and Applications, Vol. 24, No. 4, 2008, pp. 316-320. doi:10.1007/s10496-008-0316-9
- Y. J. Piao, “Unique Common Fixed Point for a Family of Self-Maps with Same Quasi-Contractive Type Condition in 2-Metric Space,” Journal of Nanjing University (Mathematical Biquarterly), Vol. 27, No. 1, 2010, pp. 82-87 (in Chinese).
- Y. J Piao, “Uniqueness of Common Fixed Point for a Family of Mappings with
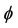 -Contractive Condition in 2- Metric Spaces,” Applied Mathematics, Vol. 3, 2012, pp. 73-77. doi:10.4236/am.2012.31012
-Contractive Condition in 2- Metric Spaces,” Applied Mathematics, Vol. 3, 2012, pp. 73-77. doi:10.4236/am.2012.31012 - M. Abbas and G. Jungck, “Common Fixed Point Results for Noncommuting Mappings without Continuity in Cone Metric Spaces,” Journal of Mathematical Analysis and Applications, Vol. 341, No. 1, 2008, pp. 416-420. doi:10.1016/j.jmaa.2007.09.070
- Y. Han and S. Y. Xu, “New Common Fixed Point Results for Four Maps on Cone Metric Spaces,” Applied Mathematics, Vol. 2, 2011, pp. 1114-1118. doi:10.4236/am.2011.29153
- C. D. Bari and P. Vetro, “
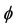 -Pairs and Common Fixed Points in Cone Metric Spaces,” Rendiconti del Circolo Matematico Palermo, Vol. 57, 2008, pp. 279-285.
-Pairs and Common Fixed Points in Cone Metric Spaces,” Rendiconti del Circolo Matematico Palermo, Vol. 57, 2008, pp. 279-285.
NOTES
*This paper is supported by the Foundation of Education Ministry, Jilin Province, China (No: 2011[434]).

