Advances in Pure Mathematics
Vol.06 No.12(2016), Article ID:72165,6 pages
10.4236/apm.2016.612069
The Riemann Hypothesis-Millennium Prize Problem
A. A. Durmagambetov
Karaganda State University, Karagandy, Kazakhstan

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: October 3, 2016; Accepted: November 19, 2016; Published: November 22, 2016
ABSTRACT
This work is dedicated to the promotion of the results C. Muntz obtained modifying zeta functions. The properties of zeta functions are studied; these properties lead to new regularities of zeta functions. The choice of a special type of modified zeta functions allows estimating the Riemann’s zeta function and solving Riemann Problem- Millennium Prize Problem.
Keywords:
Euler, Chebyshev, Dirichlet, Riemann, Hypothesis, Zeta Function, Muntz, Function, Complex Numbers, Regular Function, Integral Function Representation, Millennium Prize Problem

1. Introduction
In this work we are studying the properties of modified zeta functions. Riemann’s zeta function is defined by the Dirichlet’s distribution
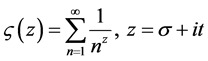 (1)
(1)
absolutely and uniformly converging in any finite region of the complex z-plane, for which 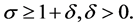 If
If 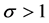 the function is represented by the following Euler product formula
the function is represented by the following Euler product formula
 (2)
(2)
where p is all prime numbers.  was firstly introduced by Euler [1] in 1737, who decomposed it to the Euler product formula (2). Chebyshev [2] , studying the law of prime numbers distribution, had considered this function. However, the most profound properties of the function
was firstly introduced by Euler [1] in 1737, who decomposed it to the Euler product formula (2). Chebyshev [2] , studying the law of prime numbers distribution, had considered this function. However, the most profound properties of the function 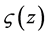 had only been discovered later, when the function had been considered as a function of a complex variable. In 1876 Riemann [3] was the first who showed that:
had only been discovered later, when the function had been considered as a function of a complex variable. In 1876 Riemann [3] was the first who showed that:
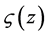 allows analytical continuation on the whole z-plane in the following form
allows analytical continuation on the whole z-plane in the following form
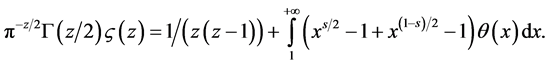 (3)
(3)
where ―gamma function.
―gamma function.
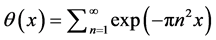 .
.
 is a regular function for all values of z, except z = 1, where it has a simple pole with a deduction equal to 1, and satisfies the following functional equation
is a regular function for all values of z, except z = 1, where it has a simple pole with a deduction equal to 1, and satisfies the following functional equation
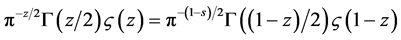 (4)
(4)
This equation is called the Riemann’s functional equation.
The Riemann’s zeta function is the most important subject of study and has a plenty of interesting generalizations. The role of zeta functions in the Number Theory is very significant, and is connected to various fundamental functions in the Number Theory as Mobius function, Liouville function, the function of quantity of number divisors, and the function of quantity of prime number divisors. The detailed theory of zeta functions is showed in [4] . The zeta function spreads to various disciplines and now the function is mostly applied in quantum statistical mechanics and quantum theory of pole [5] [6] [7] . Riemann’s zeta function is often introduced in the formulas of quantum statistics. A well-known example is the Stefan-Boltzman law of a black body’s radiation. The given aspects of the zeta function reveal global necessity of its further investigation.
The most significant contribution to the study of zeta functions is found in the results obtained by Muntz [8] .
Muntz generalized all the results from the studies of zeta functions’ analytical properties. He noticed that all the properties can be integrated in one theory, which is called the Muntz theorem for zeta functions.
Our goal is to use this theorem on the analogs of zeta functions. We are interested in the analytical properties of the following generalizations of zeta functions:
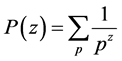 (5)
(5)
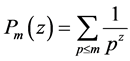 (6)
(6)
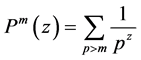 (7)
(7)
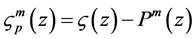 (8)
(8)
where p are prime numbers. The forms of the given function (5)-(8) allow assuming that they possess the same properties as the zeta function (1), but it is not quite obvious, considering
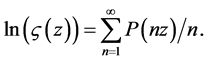 (9)
(9)
we see the necessity of analyzing (5)-(8) functions for a deeper understanding of the properties of zeta functions.
2. Results
These are the well-known results obtained by Muntz for the zeta function.
Theorem 1. Let the function 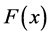 be limited on every finite interval and have an order
be limited on every finite interval and have an order 



Let N be the set of all natural numbers and

Below we will always let m > 3, this limitation is introduced only to simplify the calculations. Considering all the information above let us rewrite
For the function
Theorem 2. Let the function F(x) be limited on every finite interval and have an order






PROOF: According to the theorem conditions we have

After the substitution of variables nx = y we can rewrite

The last steps are true and result from the theorem conditions and Weierstrass theorem of uniform convergence of improper integrals. Let us introduce the functions



According to the theorem conditions we have

Applying the theorem conditions we have

Substituting the variablles of the last part
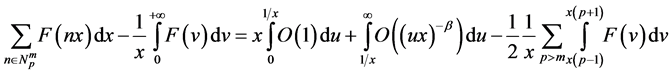
Calculating we obtain the following

According to the result above we obtain

Using the properties of defined integrals and subintegral function positivity, we have


From the result above it follows that

According to the Muntz theorem, we have

Finally, after the substitution of variables we have

From the last equation we obtain the Muntz formula. From which we have the regularity of the function 
Theorem 3. The Riemann’s function has nontrivial zeros only on the line
PROOF: For

Applying the Muntz formula from the theorem 2

estimating by the module

Estimating the zeta function, potentiating, we obtain

According to the theorem 1 

similarly, applying the theorem 2 for 

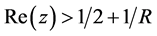


These estimations for 


3. Conclusion
In this work we obtained the estimation of the Riemann’s zeta function logarithm outside of the line 
Acknowledgements
The author thanks S.N. Baibekov for introducing the prime numbers to the proble- matics in the collective article [9] . Without this the work would be impossible.
Cite this paper
Durmagambetov, A.A. (2016) The Riemann Hypothesis-Mil- lennium Prize Problem. Advances in Pure Mathematics, 6, 915-920. http://dx.doi.org/10.4236/apm.2016.612069
References
- 1. Euler, L. (1988) Introduction to Analysis of the Infinite. Springer-Verlag, Berlin.
https:/doi.org/10.1007/978-1-4612-1021-4 - 2. Chebyshev, P.L. (1946) Selected Mathematical Works. Gostekhizdat, Moscow. [In Russian]
- 3. Riemann, G.F.B. (1972) On the Number of Prime Numbers Less than a Given Quantity. Chelsea, New York.
- 4. Titchmarsh, E.C. (1986) The Theory of the Riemann Zeta Function. 2nd Revised (Heath-Brown) Edition, Oxford University Press, Oxford.
- 5. Ray, D. and Singer, I.M. (1971) R-Torsion and the Laplacian on Riemannian Manifolds. Advances in Mathematics, 7, 145-210.
https:/doi.org/10.1016/0001-8708(71)90045-4 - 6. Bost, J.-B. (1987) Fibres determinants, determinants regularises et measures sur les espaces de modules des courbes complexes. Séminaire Bourbaki, 152-153, 113-149.
- 7. Kawagoe, K., Wakayama, M. and Yamasaki, Y. (2008) The q-Analogues of the Riemann zeta, Dirichlet L-Functions, and a Crystal Zeta-Function. Forum Mathematicum, 20, 126.
https:/doi.org/10.1515/FORUM.2008.001 - 8. Müntz, Ch.H. (1992) Beziehungen der Riemannschen ζ-Funktion zu willkurlichen reellen Funktionen. Matematisk Tidsskrift. B, 39-47.
- 9. Baibekov, S.N. and Durmagambetov. A.A. (2016) Infinitely Many Twin Primes.
arXiv:1609.04646 [math.GM]




