Advances in Pure Mathematics
Vol.2 No.5(2012), Article ID:22802,3 pages DOI:10.4236/apm.2012.25048
Some Criteria for the Asymptotic Behavior of a Certain Second Order Nonlinear Perturbed Differential Equation
Department of Mathematics and Computer Science, Izmir University, Izmir, Turkey
Email: aydin.tiryak@izmir.edu.ti
Received March 13, 2012; revised April 26, 2012; accepted May 6, 2012
Keywords: Perturbed differential equations; nonoscillatory solution; asymptotic behavior
ABSTRACT
In this paper we give sufficient conditions so that for every nonoscillatory  solution of
solution of 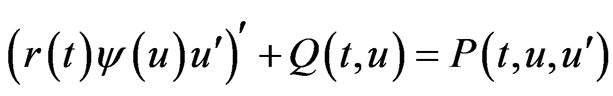 , we have
, we have . Our results contain the some known results in the literature as particular cases.
. Our results contain the some known results in the literature as particular cases.
1. Introduction
This paper is concerned with the problem of asymptotic behavior of the second order nonlinear perturbed differential equation
 (1)
(1)
where
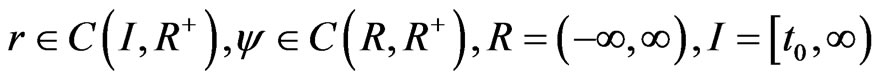 .
.
Throughout the paper according to the results we shall impose the following conditions:
(H1) Let 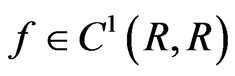 and there exists a constant
and there exists a constant
 such that
such that  and
and 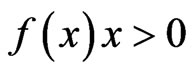 for
for
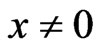 (H2)
(H2) 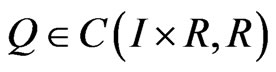 and there exists a continuous function
and there exists a continuous function 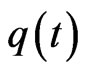 such that
such that 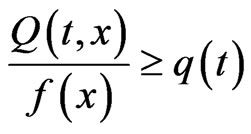 for
for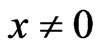 (H3)
(H3) 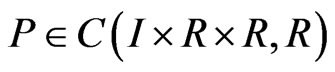 and there exists a continuous function
and there exists a continuous function 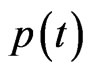 such that
such that 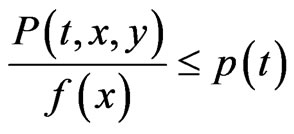 for
for

(H4)  and there exists a continuous function
and there exists a continuous function 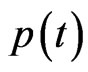 such that
such that 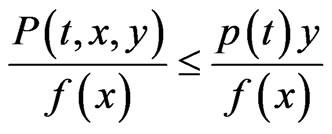 for
for

(H5)  and
and  for every
for every
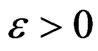 .
.
We shall also restrict our attention only to the solution of the differential equation (1.1) which exist on some ray of the form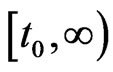 .
.
The oscillatory behavior of the solution of second order ordinary differential equations including the existence of oscillatory and nonoscillatory solutions has been the subject of intensive investigation. This problem has received the attention of many others. See for example, [1-5]. Since the publication of Hammet’s paper in [6] in 1971, the asymptotic behavior of the solution of the ordinary and functional differential equations has been widely discussed in the literature [2,4,7-9].
In this paper we give sufficient conditions so that for every nonoscillatory 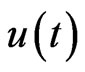 solution of (1), we have
solution of (1), we have
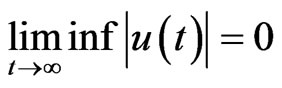 . Our results contain the results in [10]
. Our results contain the results in [10]
as particular cases.
2. Main Results
In this section we prove our main results.
Theorem 2.1. Let conditions (H1), (H2), (H3) and (H5) hold. If there exists a differentiable function 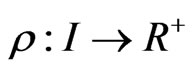 such that
such that
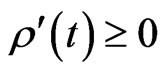 , (2)
, (2)
 (3)
(3)
and
 (4)
(4)
where
 .
.
Then for every nonoscillatory solution  of equation
of equation
(1), we have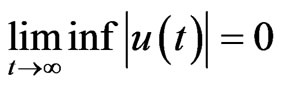 .
.
Proof. Let  be a nonoscillatory solution of (1). We may assume that
be a nonoscillatory solution of (1). We may assume that  for
for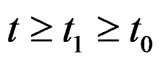 . Define
. Define
 . (5)
. (5)
Differentiating (5) and making use of (1) and from hypothesis (H1), (H2) and (H3), it follows that
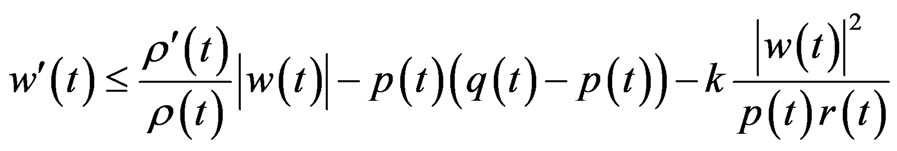 (6)
(6)
By using the inequality
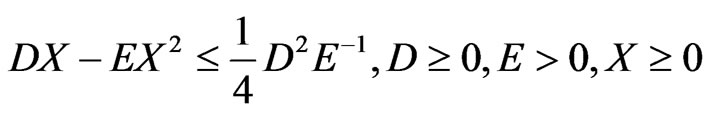 (7)
(7)
we get
 (8)
(8)
Integrating this inequality, from 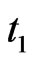 to
to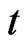 , we get
, we get
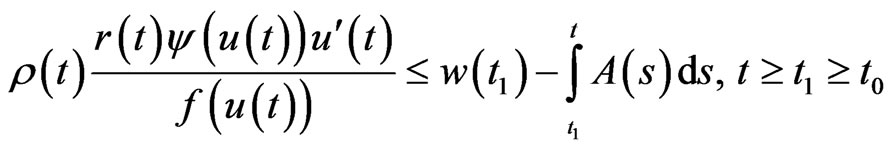 (9)
(9)
Dividing (9) by 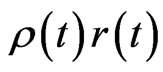 and hence integrating from
and hence integrating from  to
to  we obtain
we obtain
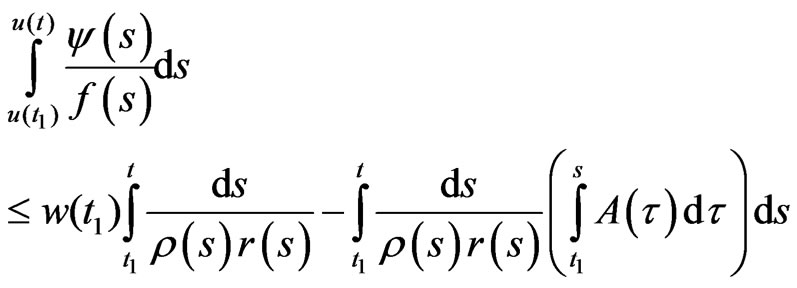 (10)
(10)
Using (4) we get
 (11)
(11)
If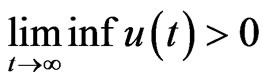 , then there exists a positive constant
, then there exists a positive constant 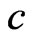 such that
such that 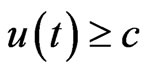 for all
for all 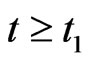 and consequently, by (H5)
and consequently, by (H5)

which contradicts (11). Thus we must have
 . The proof for the case
. The proof for the case 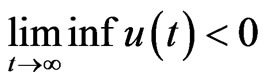
for  is similar and hence is omitted.
is similar and hence is omitted.
We note that when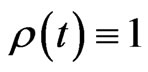 , (H1) condition can be weakened. Indeed from the proof of Theorem 2.1, the following result can obtain easily.
, (H1) condition can be weakened. Indeed from the proof of Theorem 2.1, the following result can obtain easily.
Theorem 2.2. Let conditions (H1), (H2), (H3) and (H5) hold. Suppose that
 ,
, 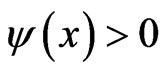 and
and 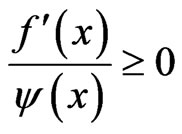 for
for 
and
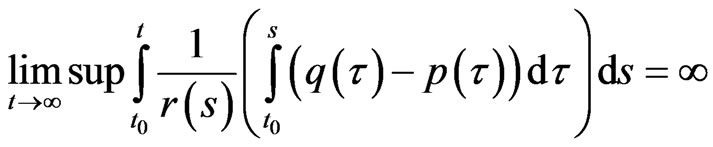 .
.
Then for every solution 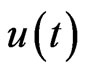 of Equation (1), we have
of Equation (1), we have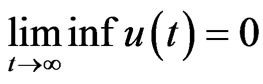 .
.
By taking (H4) instead of (H3) we obtain the following result which can be applied for example to the damped equation
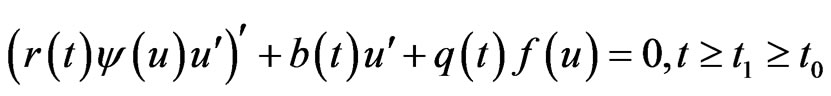 .
.
Theorem 2.3. Let conditions (H1), (H2), (H4) and (H5) hold. Suppose that
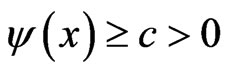 . (12)
. (12)
If there exists a differentiable function  such that (3) holds and,
such that (3) holds and,
 (13)
(13)
where
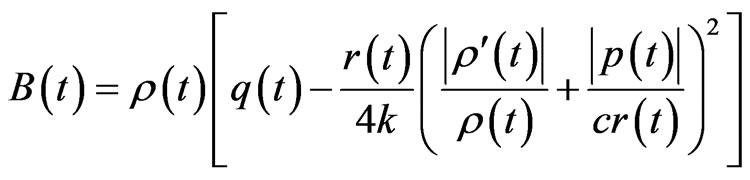
then for every solution 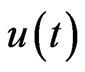 of Equation (1), we have
of Equation (1), we have
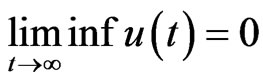 .
.
Proof. Let 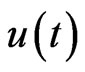 be a nonoscillatory solution of Equation (1). We may assume that
be a nonoscillatory solution of Equation (1). We may assume that 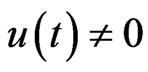 for
for  . Differentiating (5) and making use of (1) and from hypothesis (H1), (H2), (H4) and (12), as in the proof of Theorem 2.1, we can obtain easily that
. Differentiating (5) and making use of (1) and from hypothesis (H1), (H2), (H4) and (12), as in the proof of Theorem 2.1, we can obtain easily that
 (14)
(14)
Integrating this inequality from 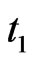 to
to 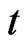 we get
we get
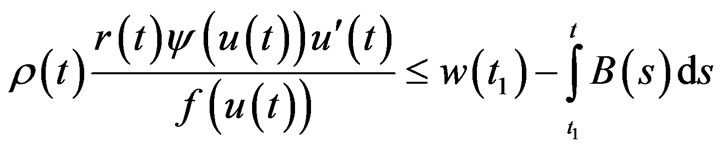 (15)
(15)
Dividing (15) by 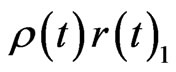 and hence integrating from
and hence integrating from  to
to  we obtain
we obtain
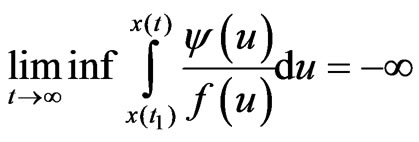
The rest of of the proof is similar to that of Theorem 2.1 and hence is omitted.
Remark 2.1. Grace and Lalli, consider the following equation
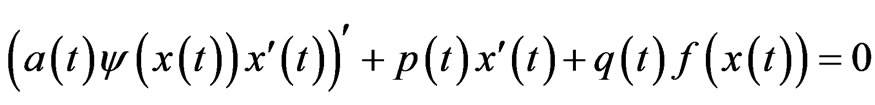
in [10] and give a similar result. But if we compare Theorem 2.3 with Theorem 2 in [10], we observe that they have a condition such as 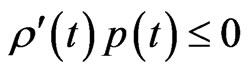 for
for  which impose some restriction on
which impose some restriction on  and
and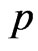 . In our result we remove this condition, so Theorem 2.3 is weaker then Theorem 2 in [10].
. In our result we remove this condition, so Theorem 2.3 is weaker then Theorem 2 in [10].
Remark 2.2. To give similar results for the equation
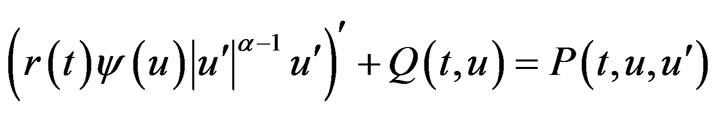
where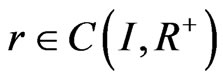 ,
, 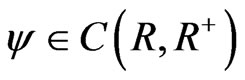 ,
,  ,
,
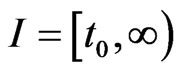 and
and 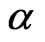 is a positive real number, still remains as an open problem and will be interesting.
is a positive real number, still remains as an open problem and will be interesting.
3. Example
Consider the differential equation of the form
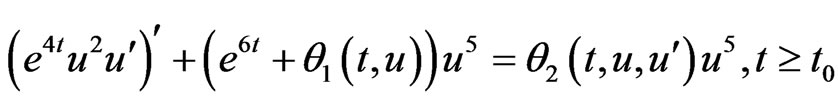
where  and
and  are continuous function such that
are continuous function such that 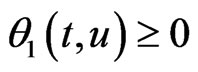 and
and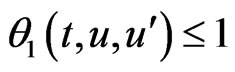 . All conditions of Theorem 2.1 are satisfied. Then every nonoscillatory solutions
. All conditions of Theorem 2.1 are satisfied. Then every nonoscillatory solutions 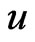 of (16) we have
of (16) we have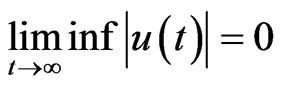 . In particular
. In particular
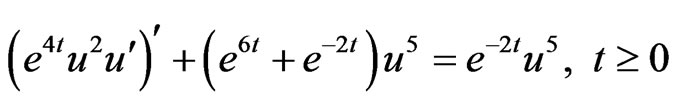
has a solution 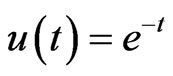 and
and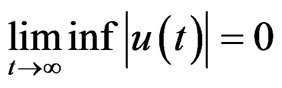 .
.
REFERENCES
- R. P. Agarwal, S. R. Grace and D. O. Regan, “Oscillation Theory for Second Order Linear, Half Linear, Superlinear, Sublinear Dynamic Equations,” Kluwer, Dordecht, 2002. Hdoi:10.1016/0022-247X(91)90185-3
- S. R. Grace, “Oscillatory and Asymptotic Behavior of Certain Functional Differential Equations,” Journal of Mathematical Analysis and Applications, Vol. 62, No. 1, 1991, pp. 177-188.
- M. Remili, “Oscillation Criteria for Second Order Nonlinear Perturbed Differential Equations,” Electronic Journal of Qualitative Theory of Differential Equations, Vol. 25, 2010, pp. 1-11.
- P. Temtek and A. Tiryaki, “Oscillation Criteria for a Certain Second Order Nonlinear Perturbed Differential Equations,” Submitted for Publication, 2012.
- Q. Zhang and L. Wang, “Oscillatory Behavior of Solutions for a Class of Second Order Nonlinear Differential Equation with Perturbation,” Acta Applicandae Mathematicae, Vol. 110, No. 2, 2010, pp. 885-893. Hdoi:10.1007/s10440-009-9483-8
- M. E. Hammet, “Nonoscillation Properties of a Nonlinear Differential Equation,” Proceedings of the American Mathematical Society, Vol. 30, No. 1, 1971, pp. 92-96. Hdoi:10.1090/S0002-9939-1971-0279384-5
- R. P. Agarwal, S. R. Grace and D. O. Regan, “Oscillation Theory for Second Order Dynamic Equations,” Taylor and Francis, London, 2003. Hdoi:10.4324/9780203222898
- Ch. G. Philos, “On the Existence of Nonoscillatory Solutions Tending to Zero at ∞ for Differential Equations with Positive Delays,” Archiv der Mathematik, Vol. 36, No. 1, 1981, pp. 168-170. Hdoi:10.1007/BF01223686
- P. J. Y. Wong and R. P. Agarwal, “Oscillation Theorems and Existence Criteria of a Asymptotically Monotone Solution for Second Order Differential Equations,” Dynamic Systems & Applications, Vol. 4, 1995, pp. 477-496.
- S. R. Grace and B. S. Lalli, “Oscillations in Second Order Differential Equations with Alternating Coefficients,” Periodica Mathematica Hungarica, Vol. 1, 1998, pp. 69-78.

