ABSTRACT

A simple method for measuring RF plasma parameters by means of a DC-biased Langmuir probe is developed. The object of this paper is to ensure the reliability of this method by using the other methods with different principles. First, Langmuir probe current 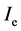response on RF voltage 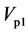superimposed to DC 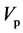biased probe was examined in DC plasmas. Next, probe current response of DC biased probe in RF plasmas was studied and compared with the first experiment. The results were confirmed by using an emissive prove method, an ion acoustic wave method, and a square pulse response method. The method using a simple Langmuir probe is useful and convenient for measuring electron temperature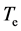, electron density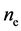, time-averaged space potential, and amplitude of space potential oscillation 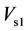in RF plasmas with a frequency of the order of.

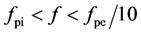Keywords:

Langmuir Probe, Radio Frequency Discharge, RF Plasma, Plasma Parameters

1. Introduction

During the past 50 years, various techniques have been developed to determine plasma parameters in RF discharge plasmas using a Langmuir probe [1] - [5] , an RF-driven probe [6] - [8] , a compensated RF-driven probe [9] - [11] , a tuned probe [12] [13] , and optical method [14] [15] . When an RF signal same as the phase and amplitude of space potential in RF (13.56 MHz) discharge plasmas is applied to a probe, RF potential between the probe and the plasma can be removed. In this case, a characteristic curve similar to a curve of DC discharge plasma is provided by this technique. By using this principle Braithwaite [6] , Paranjpe [13] , and others carried out detailed experiments and reported the experimental results [6] - [13] . However, these techniques are complicated and troublesome for measuring the plasma parameters. For example, the driven probe method needs a phase controller, an attenuator, an oscilloscope, etc., and the tuned probe technique requires a tuning network, a low-path filter, and so forth for obtaining probe current and voltage 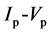characteristics. In addition, these procedures need to lock the probe potential to the phase of oscillation of plasma space potential at each time whenever experimental conditions are altered; namely, gas pressure, electric power, probe position, and so on. Therefore, the methods mentioned above are rather difficult and impractical.

The same electric current flows in a probe circuit in the following two cases. The first case is that the probe potential is constant, and space potential oscillates in the plasma. The second case is that space potential is constant, and probe potential oscillates in the plasma. In other words, both cases are totally equivalent for an electric circuit. In 1963, by using a numerical computation Boschi [3] obtained the time-averaged probe characteristic curves of a DC plasma in which a probe potential vibrated sinusoidally. If the electron distribution function is Maxwellian and the probe voltage 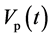in a DC plasma is oscillating sinusoidally with a frequency 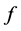and an amplitude 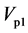around the probe bias voltage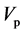, i.e.

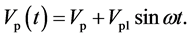(1)

Here, is angular frequency. For probe bias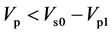, where electrons are always retarded, the electron current density 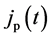flowing into the probe can be expressed as follows [3] [4] :

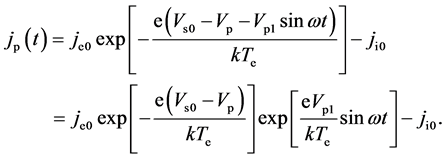(2)

Here, is the space potential of DC plasma. The time-averaged probe characteristic can be expressed as follows:

(3)

where and are saturated currents of electrons and ions for a DC plasma. is the zeroth order modified Bessel function. If is defined by the following equation, i.e.

(4)

Equation (5) can be derived from Equations (1)-(4).

(5)

Equation (5) shows that the time-averaged probe characteristic curve shifts in parallel to more negative value than a case of, so that the electron temperature is constant regardless of the frequency f and the amplitude. In the case of, because the probe characteristic curve was not expressed in a numerical formula, it was derived by using a computer [Figure 2 in Ref. 3]. As a result, following conclusions were derived.

(A) Inflection points appear at two places of on the time-averaged probe characteristic curve.

(B) The floating potential is unrelated to the applied frequency, and moves to the low potential side by as the amplitude becomes large.

(C) The electron temperature is constant regardless of the frequency f and the amplitude.

He obtained the time-averaged probe characteristic curves where the sinusoidal voltage from 10 Hz to 10 MHz was applied to a probe in DC discharge plasma. As a result, the experimental data well agreed with the theory concerning to the items (B) and (C). Both items suggest that electron temperature can be also obtained from a time-averaged characteristic curve of RF discharge plasma. Garscadden [1] also measured how a probe characteristic curve was changed by applying a sinusoidal potential covering from 50 Hz to 500 Hz to the probe. As a result, the curve as it was expected by Equation (5) was provided [see Figure 2 in Ref. 1].

In this paper investigations of the effects of RF potential oscillation on the Langmuir probe characteristic curve are described, and a simple method for interpreting the plasma parameter data is presented. This method is based on using a time-averaged Langmuir probe characteristic, and is very simple, because almost the same probe circuit which is used for DC discharge plasmas can be used.

2. Experiments Using DC Discharge Plasma

2.1. Experimental Device for Applying a Sinusoidal Voltage to a Probe

The experimental device and measurement system for obtaining a probe characteristic curve is shown in Figure 1(a). The discharge chamber, 23 cm in diameter and 50 cm in length, is situated in a stainless steel vacuum chamber of 60 cm in diameter and 100 cm long, which is evacuated to a pressure of 10⁻³ Pa by using a diffusion pump and a rotary pump. A probe tip has a plane circular surface of 3.5 mm in diameter and is spot- welded to a copper wire lead of a 50-Ω semi-rigid coaxial cable which is sleeved within a glass tube. This probe, collecting electrons on both sides, is put at the position of a radius of 3 cm. A magnetic field is added so that the high energy-tail electrons from a cathode of 2 cm in diameter cannot arrive at the probe.

The probe is biased by two dry batteries of 90V in order to prevent 50 Hz signal from spreading over the probe and discharge circuit. In addition to a DC bias voltage, a

sinusoidal voltage with amplitude is applied to the probe by a function generator which gives signals whose frequency f is controlled from 30 kHz to 10 MHz. An argon plasma is generated at a pressure of 0.133 Pa by applying a discharge voltage (=−36 V) to a disc cathode (BaO cathode) with respect to the cylindrical electrode (anode) which is connected to a 50 Ω resistor. For the DC discharge three storage batteries were employed to prevent 50 Hz signal from spreading over the probe and discharge circuit. Before drawing a characteristic curve the probe is cleaned by biasing to −100 V in order to remove surface contamination by Ar ion bombardment. A probe characteristic curve is drawn on an X − Y recorder for measuring plasma parameters. The low-pass filter (LPF) with blocking frequency of 10 kHz is used to allow DC current into the Y terminal of the X − Y recorder.

In order to receive a high frequency signal from plasma exactly next four items [16] [17] are considered.

1) A 50 Ω metal film resistor is put between a discharge tube and the ground. This resistor is used to match the characteristic impedance of the 50-Ω coaxial cable. The signal from plasma is received by this resistor.

2) Three lead storage batteries connected tandemly for DC discharge is put on a wooden desk. This is because the capacitance does not evolve between the batteries and the earth.

3) The power supply (P.S.) for heating the barium oxide (BaO) cathode which diameter is 2 cm is separated from the measurement circuit.

4) Sinusoidal voltage provided by a function generator is applied to a probe using a 50 Ω metal film resistor instead of a coupling transformer [2] .

2.2. Time-Averaged Probe Characteristic Curves

Time-averaged probe characteristics, which have been already reported in Refs. [18] [19] , are shown in Figure 2. All of characteristics shown by dotted lines are the same curves obtained at. From a semi-log plot of their electron currents space potential, electron temperature and electron density are gotten, so that ion plasma frequency is, which is given by for Ar. Time-averaged curves at f = 30 kHz, 0.6 MHz, and 10 MHz for are also shown by solid lines in Figures 2(a)-(c), respectively. For there appear two inflection points on the curve (solid line) at as pointed by long arrows (see also Refs. 3, 4 and 5). In this case, the same from the two curves as expressed by Equation (5) can be obtained. However, for the inflection points become

not clear as pointed by short arrows, and their potentials seem to approach to the potential. For the potentials of these points are completely shifted to as shown in Figure 2(c). These phenomena are different from the results reported in Refs. 3, 4 and 5. On the other hand, one can see that in the regime three time-averaged curves shown solid lines are the same form in all cases in Figure 2. Therefore, it can be confirmed that electron temperature provided from the range of the time-averaged curve (solid line) gives the same value without depending on the applied frequency f as described in the item (C). It is also confirmed that is independent of the amplitude.

2.3. Frequency Dependency of Inflection Points

Figure 3 shows frequency dependency of potential difference between two inflection points observed on a time-averaged curve, as described in Figure 2 for. In the case of, has a constant value as shown in Refs. [1] and [3] . In the case of, however, becomes small as frequency rises. Finally, in the case of, is equal to 0 V. This phenomenon occurs due to the slow motion of ions, being not able to follow the change of the high-frequency electric field within the probe-plasma sheath [20] . The phenomena to occur at frequency more than are different from the theoretical results reported in References [1] - [3] .

Figure 4 shows semi-log plots of time-averaged electron currents shown in Figure 2(c). In the case of, two inflection points appear on the semi-log plot of the time-averaged electron current, as shown by opened circles. The potential of the upper one is equal to the DC plasma space potential. The potential of

the lower one is equal to −1.9 V, which is also equal to. In other words, the potential difference between these points equals the amplitude of the RF voltage applied to the probe. In addition, the electron saturation current at the upper inflection point at is almost equal to that in the case of (dotted line). Further, the same electron temperature of can be estimated from two parallel straight-lines fitting to the semi-log plots in the retarding potential range. Therefore, It was confirmed that Equation (5) could be even applied to the case of the high frequencies more than. Therefore, all plasma parameters including can be measured from the semi-log plots shown by opened circles in Figure 4.

The potential difference between upper and lower inflection points measured from the semi-log plot of the time averaged curves (see Figure 4) is plotted as a function of at, as shown in Figure 5. Both and are normalized by which is also measured from the slope of the semi-log plot of the time averaged curves. Here, cross symbols are obtained from a DC plasma of, and (). Open circles are obtained from a DC plasma of, and (). Closed circles are obtained from a DC plasma of, and (). In all cases, the condition is satisfied. It is found that and are well agreed with each other in a wide voltage range. Therefore, can be measured precisely from.

2.4. Time-Resolved Probe Characteristic Curves

The experimental setup with a sampling convertor for obtaining a time-resolved probe characteristic curve is shown in Figure 1(a). An oscillating current flowing in a probe is inputted into the sampling converter. The characteristic curve at each time phase is drawn on the X − Y recorder by changing the probe voltage with fixing output- phase of the sampling comverter.

Probe characteristic curves at each time phase are shown in Figure 6, where is 6.0 V. Plasma parameters are, , and, re- spectively.Curves in the case of 30 kHz are shown by dotted lines. On the other hand, curves in the case of 10 MHz () are shown by solid lines. Probe voltage is oscillating between and. Instantaneous probe voltages at and are and, respectively, as shown in an inset in Figure 6. and are floating potentials at and in the case of f = 30 kHz. Potential difference is equal to in Figure 6.

There are four features in the probe current shown in Figure 6. First, the probe currents oscillate with the applied voltage in phase. This means that in this probe circuit only conduction current flows, but displacement current does not flow. Second, for, the amplitude of probe current at 10 MHz is larger than that at 30 kHz. This phenomenon will be discussed in section 5. Third, at, oscillating potential difference between the probe and plasma becomes 0 V, so that two characteristic curves shown by a dotted line and a solid line overlap each other. These curves also agree with a curve in the case of. In other words, one can obtain exact plasma parameters mentioned above by using the curves at without any effect from RF electric field. It is also indicated that the time-averaged characteristics curve shown by dotted lines has two inflection points as shown in

Figure 2(a). Fourth, in the retarding range, the probe currents in the cases of 30 kHz and 10 MHz are overrapped each other, indicating that electron tem- perature can be provided from the time-averaged probe characteristic curves shown by solid lines in the retarding potential range. This phenomenon will be also discussed in Secsion 5.

Each curve shown by solid line has an inflection point at, so that the time-averaged characteristic curves shown by solid line has at least one inflection point at [see Figure 2(c)]. RF probe current has the maximum at as shown in Ref. [21] .

3. Experiments Using RF Discharge Plasma

3.1. Experimental Device for Drawing Plasma Characteristics

The experimental device and circuit for obtaining a probe characteristic in RF plasmas with space potential oscillation by using a DC-biased probe is shown in Figure 1(b), where the cylindrical chamber is grounded. RF discharge at 8.2 MHz is carried out. The experiment is performed in a cylindrical chamber of 23 cm in diameter and 50 cm in length with an cylindrical electrode (22 cm in diameter) to which RF power of 200 W is applied via matching unit. Argon is used as a working gas at pressure of 0.133 Pa. Background pressure is 10⁻³ Pa. A tantalum probe same as what is used in Figure 1(a) is placed in the center of the device. It was movable in the axial direction. The output voltage of plasma generator, which is suppressed to a one-tenth by an attenuator, is inputted to a trigger terminal of the sampling convertor.

3.2. Time-Resolved Probe Characteristic Curves in RF Discharge Plasmas

Time-resolved probe characteristic curves are shown by solid lines in Figure 7, where a time-averaged probe curve is shown by a dotted line. Instantaneous voltage of space potential at and are and, respectively, as shown by an inset in Figure 7. Here, is the amplitude of RF space potential. RF potential difference between the probe and plasma vanishes at, so that, , and can be obtained from the characteristic curves at these time phases as mentioned in Figure 6., , and are obtained. The time-averaged probe curve shown by dotted line has only one inflection point at as also shown in Figure 2(c). Time-resolved curves shown by solid lines have also inflection point at. The oscillating probe current for has a large amplitude, similar to the results in Figure 6. This phenomenon will be also discussed in Section 5.

3.3. Semi-Log Plots of Time-Resolved Probe Electron Current I_e

Figure 8 shows semi-log plots of time-resolved electron current of the probe at four time phases; i.e.,. The experimental condition is the same as that in Figure 7. Because an RF electric field does not exist at, the time-resolved curve has only one inflection point at. Plasma parameters mentioned above can be provided from this curve. Because all four straight lines fitting to the semi-log plots in the retarding potential range are parallel, it is confirmed that of this RF discharge plasma is constant, being independent of the time phase.Since frequency of RF potential fluctuation is much lower than electron plasma fre- quency, i.e., (GHz), electrons can easily follow the change of space potential fluctuation and can be isothermalized to a single Maxwellian.

3.4. Semi-Log Plots of Time-Averaged Probe Electron Current I_e

Semi-log plots of the time-averaged electron current and time-resolved electron current at are shown in Figure 9. Because an RF electric field does not exist in the case of, this curve has only one inflection point. Plasma parameters can be provided from this curve, namely, , and. In the case of time-averaged curve, the potential difference 22.0 V between upper and lower inflection points can be thought to give the amplitude of RF space potential oscillation, as shown in Figure 4. The validity of is verified by an emissive probe method described in the following Section 4. The other plasma parameters, , and, which are derived from the time-resolved curve at, can be also obtained from the semi-log plot of the time-averaged characteristic curve.

From this figure, it can be also measureed that defined by Equation (4) is 11.74 V. By substituting and into Equation (4), is obtained. This value is almost equal to. Therefore, it is confirmed that Equation (5) can be also applied to RF plasmas. It should be noted that the inflection point method presented here is quite easy to obtain than a method using Equation (4).

From the technique described above, plasma parameters of RF plasmas can be easily obtained by using almost the same probe circuit as used for DC discharge plasmas, by combining a Microsoft Visual C++ software and a personal computer controlled Source Meter-2400 manufactured by Keithley Instruments. This technique is very convenient and useful for the measurement of plasma parameters of RF plasmas efficiently.

4. Comparison with Other Measurement Methods

As shown in Section 3, plasma parameters of RF discharge plasma were measured easily by a semi-log plot of the time-averaged characteristic curve of Langmuir probe. In order to ensure the reliability of the data provided by the probe method above, it is necessary to compare the plasma parameters with those provided using measurement procedures based on different principles. The comparison experiments were carried out for existence of inflection point at, validity of electron temperature, and the mechanism of electron current enhancement and suppression at and, respectively, in RF plasmas (see Figure 7).

4.1. Inflection Point Measurement with Emission Probe Method

Emission probes were employed to measure the space potential of DC discharge plasma exactly [22] [23] . A few researchers reported the methods for measuring the amplitude of plasma space potential by using the inflection point technique [24] - [26] . Here, emission probe method is employed to confirm the existence of the inflection points at in RF plasmas and to verify the presence of lower inflection point at on the semi-log plot of time-averaged probe characteristic curve in RF plasmas.

The experiment was performed in the RF discharge tube shown in Figure 1(b)., , and are 0.67 Pa, 6.6 eV, , respectively. A conventional emission probe made of tantalum hair pin wire of 0.125 mm in diameter is employed in order to measure the amplitude of the RF fluctuation of space potential. The emission probe is covered with an alumina tube with outer diameter of 2 mm with two holes, except for the probe tip [22] . The filament heating current is maintained by a lead storage battery to prevent 50 Hz signal from spreading over the probe and discharge circuit.

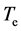
Time-averaged characteristic curves of the emission probe are shown in Figure 10. In the case of probe heating current, the curve similar to that plotted by dotted curve in Figure 7 is obtained. Therefore, the inflection point appears at one point. Although the semi-log plot of this curve is not shown in this figure, time-averaged space potential and amplitude of space potential are ob-

tained from the semi-log plot of the time-averaged electron current. However, in the cases of and 1.12 A there appear three inflection points in both curves as pointed by arrows. The voltage of the middle point is the same as that in the case of. The potential difference between upper and lower points is 40.2 V as shown in Figure 10 and it is almost equal to 39.6 V which is twice of the amplitude of the space potential 19.8 V. Therefore, it is confirmed experimentally that and obtained from the emission probe method well agree with the values provided by the Langmuir probe method shown in Figures 7-9.

4.2. Electron Temperature Measurement with Ion Acoustic Wave Method

Ion acoustic wave method is one of the useful ways for obtaining an electron temperature in RF discharge plasmas [27] - [30] . The plasma generation and the measurement system for ion acoustic wave are shown schematically in Figure 11. They are housed in a stainless steel vacuum chamber with an inner diameter of 60 cm and a length of 100 cm. This reactor, 7 cm in diameter and 12 cm in length, is the same as that shown in Figure 1 of Ref. [31] except that plasma is produced by an RF discharge. A cylindrical

Langmuir probe P (diameter 0.6 mm, length 1.8 mm made of tantalum wire) is spot- welded to a copper core wire of a 50 Ω semi-rigid coaxial-cable (outer diameter 2.2 mm), which is sleeved with a glass tube. The gas pressure is evacuated to a pressure of 10⁻³ Pa by using a diffusion pump and a rotary pump. Argon plasma is generated by RF (25 MHz) discharge.

A grid electrode G located at the outlet of the RF electrode is used for controlling the electron temperature in the downstream region of plasma [32] - [35] . The grid G (16 mesh/in.) is made of 0.29-mm-diameter stainless steel wire and installed on a 4.2-cm- diameter aluminum ring flame which is connected to the earth using capacitors which exhibit low impedance to RF while allowing DC biasing of this electrode [32] . These capacitors are not shown in Figure 11.

Ion acoustic waves are excited by an exciter (Exc.: 20 mesh/in., 2-cm diameter) and detected by a movable detector (Det.: 8 mesh/in., 1.5-cm diameter). Exc. and Det. are made of a grid of the stainless steel wire of 0.29 mm in diameter. The frequency of ion acoustic wave is changed by a low frequency (LF) oscillator between 50 kHz and 300 kHz. Argon pressure is 0.67 Pa. A magnetic field of is applied in order that axial electron density distribution becomes uniform and the ions suffer one-di- mensional compressions in the plane waves [36] [37] . A wave pattern is drawn on the X − Y recorder through a lock-in amplifier.

The dispersion relation given by Equation (6) for ion acoustic waves is derived from a fluid theory under the conditions and.

(6)

Here, and are frequency, wave length, and velocity of the ion acoustic wave, respectively [38] . Using argon gas, in the case of, this expression is referred to Equation (7)

(7)

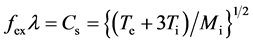
Here, the units of, , and are cm, eV, and kHz, respectively. Therefore, electron temperature can be derived by measuring the wave length of the ion acoustic at the frequency.

Wave patterns at for and −40 V are shown in Figure 12. The wavelength of the ion wave becomes short with a drop of V_G. The wavelengths at and −40 V are 2.0 cm and 1.25 cm, respectively. From this result, the electron temperature at is calculated to be 1.64 eV by substituting and cm in Equation (7). The relations between and in the cases of grid voltage V_G = 40 V, 0 V, and −40 V are shown in Figure 13. The slope of the straight line corresponding to the phase velocity of the ion acoustic wave becomes small with a drop of. This result indicates that electron temperature drops as decreases.

Electron temperatures calculated by the ion acoustic method with Equation (7) and measured by the probe method are shown in Figure 14 as a function of by closed and open circles, respectively. Both electron temperatures are well agreed with each

other. decreases from 4.2 eV to about 0.5 eV by a decrease in. Since the electron temperatures obtained by two methods well fit each other, it is confirmed that electron temperature provided by the probe method is correct.

4.3. Electron Current Variations Using a Square Pulse in DC Discharge Plasmas

Since it was confirmed that probe current response on RF voltage superimposed to DC biased probe in DC plasmas was equivalent to that on DC biased probe in RF plasmas (see Figure 6 and Figure 7), a mechanism on the current enhancement and suppression for at and, respectively, in RF plasmas (see

Figure 7) was investigated by using a square pulse voltage superimposed to DC probe voltage in DC plasma in an experimental apparatus shown in Figure 1(a). Rise time, time width, and amplitude of the square pulse voltage are 0.05 μs, 10 μs, and ±5 V, respectively. The rise time 0.05 μs is equivalent to a quarter period of 5 MHz signal. This pulse voltage is applied to the DC biased probe voltage. Therefore, the voltage of the disc probe changes from to [19] .

When, the characteristic curve of the disc probe is shown in Figure 15(a) under the condition that and B are 0.133 Pa and, respectively. The disc probe is put at the position of a radius of 3 cm so that high energy electrons cannot arrive at this place. Plasma parameters are obtained, i.e., , and, thus. Figure 15(b) shows temporal variations of probe current with initial probe voltage as a parameter. In the case of, the pulsed voltage changes from to (). In this case, electron current attained immediately to the stationary current without any deformation (see bottom trace). On the other hand, when, i.e., the electron current quickly overshoots in the initial response, and such overshooting of the electron current attains the maximum as approaches to. Further, in this case, after overshooting the electron current diminishes and returns to a minimum value, evolving an amplitude oscillation decaying in time. A period of this oscillation is about 1.71 μs, corresponding to the frequency 0.58 MHz which is lower than. From this pulse experiment, it was known that the electron current increases at in Figure 6 and at in Figure 7 is quite similar to the current response at the initial stage when the positive square voltage was applied to the probe.

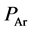
During the initial time response, time-resolved probe measurements are carried out in a DC plasma for measuring the space potential profile in front of the probe. Here,

, , , and under and. Figure 16(a) and Figure 16(b) show axial (z) distribution of (a) space potential and (b) electron current ratio near the disc probe, respectively. Space potentials are obtained by using time-resolved characteristic curves of the cylindrical probe. The voltage of the disc probe changes from 5 V to 15 V in 0.05 μs. The symbols ○, △ and ● correspond to the times when the current becomes the maximum, minimum and steady state values, respectively, as shown by an inlet in Figure 16(a). In the case of the steady state (●), the space potential profile is uniform, even close to the probe position at z = 0. Here, Debye length obtained from and is.

On the other hand, in the case of the current maximum time (○) the space potential simply decreases in the z direction from 15 V to 4 V within a range of 5 mm from z = 0 mm. In other words, the positive probe potential leaks further into the bulk plasma up to z = 5 mm, which is far from the sheath edge () of the probe. Therefore, the probe would collect more electrons from the bulk plasma [39] . Therefore, probe current becomes larger than that in the steady state, as shown by opened circles in Figure 16(b). On the other hand, at the current minimum time (△) the space potential becomes lower than that in the steady state as shown in Figure 16(a), indicating that there appears a potential minimum dip between z = 0 mm to 1 mm. Because of the formation of this potential dip the probe electron current is much suppressed compared to that in the steady state value, as also shown in Figure 16(b) [40] [41] .

At the time pointed by square (□) in Figure 16(a), one can also confirm an appearance of the potential dip in front of the disc probe, resulting in a suppression of electron current, although these data are not shown in Figure 16. This result is closely related to the current suppression at in Figure 6 and at in Figure 7.

5. Discussion

In our experiments using a DC plasma, two phenomena were observed. First, when is higher than, the amplitude of the oscillating probe current for 10 MHz was larger than that for 30 kHz, as shown in Figure 7. Second, instantaneous probe current does not depend on the frequency when is lower than, where electrons in the ion sheath receive a retarding force during the complete cycle. Let us here discuss why these two phenomena occur. It is convenient for the explanations to divide the range of probe voltage into two regions, i.e., and.

5.1. Assumption

In order to explain the two phenomena mentioned above it is necessary to make the following simplified assumptions.

1) Electrons do not collide with particles of neutral gas inside the probe sheath. This requirement is reduced to

. (8)

where is the collision cross section in cm² [4] . When and, Debye length becomes. On the other hand, electron collision mean-free path becomes at. Therefore, when is lower than 20 Pa, sheath thickness of several times becomes much smaller than. On the contrary, when the sheath thickness becomes larger than by an increase of pressure, collisions of electrons in the probe sheath cannot be ignored. In this case, usual probe theory has to be modified by taking the electron collision into account.

2) For the RF frequencies f in the range electrons can completely follow the oscillating electric field in the plasma sheath, but ions cannot follow at all. Here, is electron plasma frequency. Therefore, nomal sheath in the steady state cannot be formed due to the slow movement of ions at all. When the probe bias voltage is changed rapidly in a DC plasma, ions move under a time-averaged electric field, similar to the case at in Figuer 7. As long as RF frequency f is kept in the range, probe characteristics are not changed as known from the experiments in Section 2. Actually, we confirmed the usefulness of this probe analysis even in the RF discharges at frequency of 13.56 MHz ( [32] [33] [35] ).

5.2. In the Case of V_p(t) < V_s0

Temporal variations of potential curves near the probe are drawn schematically in Figure 17(a) when probe voltage oscillates between and around in a DC plasma. In the case of, the inequality is always estab-

lished inside the ion sheath so that the potential curves are expressed by convex curves as shown by dotted lines. The sheath edges also oscillates between and around. The instantaneous probe current is decided by an instantaneous potential difference as given by Equation (2).

When f is 10 MHz, the ions cannot follow the change of RF electric field in the probe sheath, so that during the complete cycle, the density distribution of ion is kept as in the steady state, namely with the same distribution at. When, only electron density decreases in comparison with that at, so that the potential distribution becomes more positive as shown by solid line at. But, the same electron current is obtained as in the case of 30 kHz, because it is decided simply by a potential difference, according to Equation (2). On the other hand, when, only electron density increases in comparison with that at. As the result, the potential distribution becomes more negative compared to that in the case of 30 kHz as shown by solid convex curve at. Even in this case, the same electron current as in the case of 30 kHz is obtained, because it is also decided by a potential difference, according to Equation (2).

In this way it is found that the probe current is independent of the applied frequncy f in the case that is below. Therefore, it is confirmed that the electron temperatue of RF discharge plasma can be obtained from the semi-log plot of time averaged characteristic curve as shown by opened circles in Figure 4.

5.3. In the Case of V_p(t) > V_s0

Potential distributions in the cases of f = 30 kHz and 10 MHz are schematically drawn by dotted and solid lines, respectively, in Figure 17(b). When f = 30 kHz, which is lower than, a conventional probe sheath is formed according to the probe potential oscillation between and around. Simultaneously, the sheath edge also oscillates between and around.

However, when f = 10 MHz, which is higher than, the density distribution of ion is always kept as in the averaged state at. In this case, an abrupt increase in the probe potential from to between and in Figure 6 causes a spread of the effective sheath width, as observed in Figure 16(a), which results in an increase in the electron current, as show in Figure 16(b). This is the reason why the electron current is so much increased at in Figure 6. On the other hand, when the probe voltage rapidly decreases from to between and in Figure 6, electron current is suppressed, as observed in Figure 16(b), by a formation of a negative potential dip, as observed in Figure 16(a). This negative potential dip is also formed in front of a cathode of a diode, namely a virtual cathode [40] [41] . This is the reason why the electron current is so much suppressed at in Figure 6.

5.4. In the Case of RF Discharge Plasma

In the RF discharges, space potential V_s(t) oscillates between and around under the constant probe voltage as schematically shown in Figure 17(c). When the space potential rapidly increases from to between and, such an abrupt drop of acceleration voltage for the electrons causes slowing down of electron speed, which causes a stagnation of electrons near the probe, and a resultant formation of a negative potential dip, as observed in Figure 16(a). Then, the electron current is suppressed, compared to the case of 30 kHz as shown in Figure 7. On the other hand, when the space potential rapidly decreases from to between and, such speed-up electrons causes a relative lack of electrons near the probe, which results in an increase in the space potential, and hence resulting in a spread of the sheath width as shown in Figure 16(a). Then, more electrons are collected by the probe and the probe electron current is enhanced compared to the case of 30 kHz as shown in Figure 7.

It is clarified that when is higher than in RF discharge plasma, RF current with large amplitude flows into the probe as shown in Figure 7. It is also clear that when is lower than, the same RF current as shown in Figure 17(a) flows into the probe because it is decided by a potential difference.

6. Conclusions

Langmuir probe characteristic curve is examined under an influence of relative oscillating potential difference between the probe and the plasma. Sinusoidal potential ranging from 30 kHz to 10 MHz with amplitude from 0 V to 7.5 V is first applied to the probe in a direct-current (DC) discharge plasma. In the case of low frequency, which is very lower than ion plasma frequency, the time-averaged probe characteristic curve has two inflection points at and. On the other hand, in the case of high frequency which is higher than, there appears two inflection points at and on a semi-log plot of time-averaged curve. Upper inflection point coincides with the space potential of DC discharge plasma, which is the same space potential at of the time-resolved curve. Electron saturation current at upper inflection point well coincides with that of. Therefore, electron density can be derived from the electron saturation current at the upper inflection point. Potential difference between upper and lower inflection points on a semi-log plot of the time-averaged curve shows the amplitude. It was also confirmed that the electron temperature is constant regardless of the frequency f and the amplitude.

These results are applied to RF discharge plasma with oscillating space potential to measure the plasma prameters by using a DC-biased Langmuir probe. As a result, it was confirmed that similar probe characteristic could be obtained in RF discharge plasmas. The amplitude of space potential oscillation, obtained from the potential difference between two inflection points on the semi-log plot of the time-averaged curve in RF discharge plasmas, was confirmed by an emissive probe method. The electron temperature in RF plasmas is confirmed by using the ion acoustic wave method. Both electron temperatures are well agreed with each other between 4.2 eV to 0.5 eV. The mechanism for the electron current enhancement and suppression for in RF plasmas is clarified by the square pulse experiment.

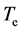
The method using a single Langmuir probe with a semi-log plot of time-averaged curve is useful and convenient for measuring electron temperature, electron density, time-averaged space potential, and amplitude of space potential oscillation in RF plasmas with a frequency of the order of. This technique mitigates a great deal of troublesome measurement of plasma parameters in RF discharge plasmas.

Acknowledgements

Authors are indebted to Prof. Noriyoshi Sato, Tohoku University, for his comments and encouragement.

Cite this paper

Kato, K. and Iizuka, S. (2016) Analysis of Langmuir Probe Characteristics for Measurement of Plasma Parameters in RF Discharge Plasmas. Journal of Applied Mathematics and Physics, 4, 1811-1836. http://dx.doi.org/10.4236/jamp.2016.49185

References