Sociology Mind
2011. Vol.1, No.4, 145-150
Copyright © 2011 SciRes. DOI:10.4236/sm.2011.14018
Altruism, Selfishness and Social Cohesion
Antonio Luigi Paolilli
University of Salento, Lecce, Italy.
Received May 1st, 2011; revised July 8th, 2011; accepted September 6th, 2011.
Recently has been shown that, given certain conditions, altruism can prevail in a population even without the
help of mechanisms as kin selection, reciprocal altruism and group selection. At the light of this hypothesis, it is
shown how altruism and cooperative aptitudes can favor the concentration of a population and how an (incom-
plete) evolution of altruism, caused by group selection, into the Benthamian form, determining the emergence of
sentiments and behaviors, such as a sense of justice, as well as envy and gossip, can lead to the formation of a
regulated society. From this standpoint, altruism leads to group selection, and not the contrary.
Keywords: Altruism, Selfishness, Group Selection, Economic Development
Introduction
The principal theories adopted to explain the emergence of
altruistic behaviors in the human genre are kin selection, recip-
rocal altruism and group selection. Recently has been shown
that, given certain conditions (mainly a rise in marginal outputs
due to the increasingly altruistic aptitudes of agents), altruism
can prevail in a population even without the help of these
mechanisms.
In the light of the latter hypothesis, this work shows that al-
truism and cooperative attitudes can work in favor of popula-
tion concentration and therefore, after the emergence of altru-
ism or at least cooperative attitudes, can trigger group selection.
The latter, in turn, favors an (incomplete) evolution of altruism
into the Benthamian form, bringing out sentiments and behave-
iors, such as a sense of justice, as well as envy and gossip,
which can lead to the formation of a regulated society.
From this standpoint, altruism leads to group selection, and
not the contrary.
The paper is organized as follows.
Section 1 is an overview of the literature about the origin of
altruism. Section 2 is devoted to define the reasons at the basis
of the concentration of a population. In Section 3 a model de-
scribing the concentration process is presented. Section 4 illus-
trates the nature and the genesis of some internal control
mechanisms, already mentioned in Section 2.
The Origin of Altruism: An Overview
The problem of the origin of altruism has been at the centre
of a wide debate.
The principal theories adopted to explain the emergence of
altruistic behaviors in the human genre are kin selection, recip-
rocal altruism and group selection.
The thesis of group selection (Winne-Edwards, 1962) asserts
that altruism involves cooperation and the internal cohesion of
a group, thus favoring its survival (Sober, 1991). This thesis has
been challenged by many evolutionists who think that, as the
unit of reproduction in humankind is not the group but the indi-
vidual, selection might favor those characteristics that maxi-
mize individual utility, thus operating against altruism. (Wil-
liams, 1966), in particular, argues that even if group selection is
theoretically possible, it’s role in nature is insignificant. This
happens because any level of the biological hierarchy requires a
process of natural selection which operates at that level, and
this is a very rare event, due to the fact that, in Williams’s view,
the fundamental unit of selection (the replicator, in Dawkins’
terminology, 1976) is the gene. In fact, in this framework
even individuals which are sexually reproducing organisms
cannot be units of selection because they are not faithfully
replicated.
Evolutionary biologists, therefore, have given greater support
to the thesis of kin selection (or inclusive fitness theory), which
holds that a subject’s altruistic behavior is directed mainly to-
ward his relatives. In this framework (Eberhard, 1975) has
mathematically shown that also small degrees of consanguinity
can constitute the basis for kin selection, and this is true, above
all, with low costs for the benefactors.
Another approach is reciprocal altruism, in which selection
can favor altruism even if it is directed at individuals with no
degree of consanguinity, as long as there is reciprocity. An
important condition to permit this outcome, therefore, is that
cooperation is based on prize-punishment mechanisms. Impor-
tant contributions on this point are the Tit-for-Tat strategy (Ax-
elrod & Hamilton, 1981), the Ultimatum Game, introduced by
(Güth et al., 1982) (see also Güth, 1995; Witt & Yaary, 1992),
and the Gift Exchange Game (Fehr, et al., 1993). Altruism, in
this context, might be the consequence of a rational choice.
However, in one particular game, the Dictator Game, in
which there is a responder who can only accept or refuse the
offer made by a proposer, with no consequences for the latter, it
has been shown that altruism is not absent (Forsythe et al,1994),
and this is in contrast with the concept of altruism as a rational
choice, drawing attention instead to genetic factors. Many
scholars, however, think that genes do not totally explain re-
ciprocal altruism. (Witt, 1985), in particular, places importance
on the capacity to learn, a capacity also referred to by (Lorenz,
1994, 1963), for the animal species in general. (Bergstrom &
Stark, 1993) believe that the behavior of an individual can es-
sentially be determined both by genetic factors and by imitation.
According to the concept of “bounded rationality” elaborated
by (Simon, 1957, 1983, 1992, 1993), there is a gap between the
actual behavior and the predictions of rational actor models. In
other words, individuals are not able to maximize their objec-
tive function if the costs of information collection and process-
1For the limits of our reasoning in conditions of uncertainty, see (Tversky &
Kahneman, 1974), (Cosmides & Tooby, 1996), (Gigerenzer & Hoffrage,
1995
)
.

A. L. PAOLILLI
146
ing are too great, and they therefore have a tendency to act on
advice and to respect norms2.
However, it cannot be denied that cultural factors, and more
generally cognitive factors, are linked to a genetic substratum.
Bowles and (Gintis, 2003), for instance, maintain that culture
and genes are strongly linked in the human species. (Gintis,
2000), in particular, asserts that humans show manifestations of
strong reciprocity, which is a behavior that probably has a ge-
netic component, because it cannot be justified only by cultural
or rational motivations. He has also modeled (Gintis, 2003)
Simon’s explanation of altruism (Simon, 1990), “showing that
altruistic norms can “hitchhike” on the general tendency of
internal norms to be personally fitness-enhancing”.
About the emergence of the genetic predisposition to altruis-
tic behaviors, Sober (Sober & Wilson, 1996), and (Frank, 1998,
2006), bringing group selection in again, have noted that, using
the Price equation (Price, 1970), strategies that are socially
beneficial but that give negative outcomes for the individuals
adopting them can survive if groups dissolve and remix often
enough and if there is a large covariance between traits in asso-
ciated partners. This happens because groups with a high pro-
portion of altruists have higher than average fitness and there-
fore grow faster than other groups, increasing the global fre-
quency of the unselfish gene, even if its frequency falls in every
group.
The multiple level of selection, above all, is then the central
idea of the new group selection approach2, which is based on
the cognition that “there is one theory of natural selection oper-
ating on a nested hierarchy of units, of which inclusive fitness
and game theory are special cases” (Wilson & Sober, 1994). In
this context we also find Bowles and (Choi, 2003) who, using
an evolutionary game theory model, assert that altruism can
emerge if combined with opposing sentiments (like xenopho-
bia), due to the fact that the most cohesive groups tend to pre-
vail in conflicts.
More recently it has been shown, by means of an evolution-
ary model (Paolilli, 2009), that cooperative aptitudes may have
emerged, at least initially, without the help of other mecha-
nisms, and particularly even if the altruists do not discriminate,
during their interactions, between altruists and egoists. In other
words, the altruists, more inclined to cooperation, prevail upon
the egoists, coexist with them or succumb depending on the
value of certain parameters, directly linked to the productivity
of the interacttions among the agents, or also depending on the
initial size of the two populations, without the intervention of
punishment mechanisms.
Altruism and Group Relations
The Paolilli model (Paolilli, 2009), reflecting a Smithian
viewpoint and the x-efficiency theory3, is based on the assump-
tion that the more the empathy between agents grows, the more
intense and numerous trade relations become, thus increasing
the output of the system. Indeed, as the work cited mentions, in
the environmental context examined (the savannah of the first
hominids), trade must be seen as a sharing of work services for
a common purpose, the outcome of which is enjoyed by each
agent according to his own degree of altruism and that of the
other person.
The model cited gives an explanation of the origin and
prevalence of altruism in a population, but has the drawback of
only considering binary economic relations (in pairs). However,
as is implicitly recognized by (Paolilli, 2009) (the “model, natu-
rally, does not exclude the operating of other … mechanisms,
such as group selection, kin selection and reciprocal altruism”),
this approach can be developed. To do this, we must take two
important elements into consideration.
The first element is the possibility that the increase of coop-
erating individuals, at least up to a certain level and for some
activities, generates a more than proportional rise in the out-
comes, thus favoring the appearance of teams composed by n
individuals, with n > 2.
The second element is the cost (also in terms of time or en-
ergy) of the transactions, which, in addition, becomes very
important when there are more than two agents interacting si-
multaneously, due to the exponential increase in possible links
between a growing number of agents. The need to reduce in-
teraction costs could determine two important consequences: 1)
the stabilization of relations, thus favoring the reduction of
uncertainly see (North 1990) and the specialization of actors,
who will act in a stable organization, and 2) the concentration
of the population, to make the relations easier. The same indi-
vidual, however, could act in many relations, in pairs or in
teams composed of more than or far more than two people, for
different activities, and this situation could favor the appear-
ance of complex groups.
The fact that the population is concentrated in stable groups,
which contain teams composed of two individuals for some
activities and more than two individuals for others, will lead to
the appearance of groups characterized of different degrees of
internal cohesion. The degree of cohesion, in fact, will depend
(directly) on the average propensity for altruism of the group
members, and (inversely) on the relational costs. Moreover, the
concentration of population, while favoring relations among the
members of the group, discourages those among members of
different groups. At this point, if two groups are using the same
resources, the most productive one (with lower production costs)
will prevail, even without war dynamics, increasing its size and
spreading its colonies in the space.
The simplest way to reduce the relational costs is to decrease
the number of relations in a team composed of more than two
individuals, structuring them in a nodal way4.
However, as we have seen (Paolilli, 2009), there is no cer-
tainty that in every human group the altruists (or cooperative
subjects) prevail completely, since intermediate situations are
also possible, with the coexistence of altruists and egoists, or at
any rate individuals with different degrees of altruism. If two
groups, competing for the same resources and possessing the
same technological level, are not composed only of altruists,
and if both are also structured with teams characterized by
nodal relations, the most efficient of the two will prevail, i.e.
the group which, besides an aptitude for altruism, also has
mechanisms to control the operation of its members.
2Field (2001) concludes that modularity cognition and the multiple level o
f
selection hold the key to explaining the prevailing of altruism.
3The concept of x-(in)efficiency (Leibenstein, 1966, 1973) has been ex-
tended by (Altman, 1996, 2001, 2005, 2006), who has shown that in a mar-
ket both ethical and non-ethical firms, characterized by different x-effi-
ciency levels, can survive.
4Naturally the nodal structure of relations will be efficient as long as it does
not reach such a level than it overloads the “nodal” subjects.
From this viewpoint, since altruism favors population con-
centration, it leads to group selection, and not the contrary, as is
usually thought. In fact we can claim that the existence itself of
a group always requires, if not actual altruism, at least an apti-
tude for exchanging or cooperating even if it is only for selfish
purposes rather than (or as well as) a predatory attitude.

A. L. PAOLILLI 147
Modeling Population Concentration
A simple formalization will be useful to show how and why
a population can assemble in one place.
Let us consider the existence of a single population p. More-
over, let us suppose that, if the population is dispersed (due to
not being cooperative or only by chance) and without technol-
ogy, every individual needs an area, which we will call place
and which will be the surface unit of the territory (assumed as
homogeneous).
If a population p assembles in a point5, it will experience two
opposing consequences: on the one hand, due to the distance
from the place s and consequent costs, the net output of the
places themselves will diminish, and on the other hand, it will
increase due to the synergies deriving from the concentration of
population. The influence of these synergies will depend on the
average degree of altruism (or cohesion) of the group.
The evolution of a group which has a certain technological
level and a certain efficiency of internal economic and social
relations, can be shown by the following equation:

dp dta kp SAp

(3.1)
It indicates that the population p varies according to a coeffi-
cient k, its actual size p and the available resources a
SA p

. A
is the surface of the (circular, given that we assume the homo-
geneity of the territory) area, expressed in term of places, ex-
ploited by the population assembled at its center. is a
function of the Cobb-Douglas type and represents the amount
of resources available (whose measure unit is the resources
necessary for the survival of an individual). The value of A is
elevated to the exponent α (whose value is less than 1), which
strictly depends on the technological level and will be assumed
as a constant. The fact that α is less than 1 means that, as the
exploitation area grows, its net marginal productivity decreases,
due to the increase of the distance. In fact the concentration of
population determines transport costs, even if only in terms of
time.
a
SA
The synergies are indicated by the coefficient S, whose value,
larger than 1 (S = 1 indicates absence of synergies), is strictly
dependent on their efficiency, in turn linked to the possible
existence of a nodal organization or of control mechanisms6.
A population dispersed on an area A, then, will assemble at
the center of this area only if and it will grow until it
will equal . Also the exploited area A will increase, if the
new places are sufficient to the survival of new individuals. We
can calculate the maximum value of A , i.e. the exploitable area
(A*), making equal to 1 the first derivative of (), with re-
spect to A:
a
SA A
a
SA
a
SA
11SA 

 (3.2)
Then the exploitable area is:
1
1
*AS



 (3.3)
The circular exploitable area, however, is obviously a func-
tion of the radium which, therefore, is *rA
1
1
π
S
r



 (3.4)
Note that r is expressed in terms of places, i.e. its unitary
value is the length of the side of a square whose surface is a
place.
Due to the fact that 2 *
A
pr, we can insert 3.4 in 3.1 and
then, simplifying, we can write:
1
1
dp dtkp SSp


















(3.5)
The equilibrium values of 3.5 are:
. We can
also write it as follows:
0p

,
1
1
pS S





 



(3.5’)
The first is a trivial equilibrium point and entails the extinction
of the group, the second is stable for positive values of k.
Observing 3.5’ we can easily see that, when the values of
parameters S and α (with α < 1) increase, the (potential) popu-
lation p grows more and more quickly (the value of k, instead,
if only positive, is not decisive). It should be noticed that, if S =
1 (for the absence of productive relations or synergies), no con-
centration of population happens: the non trivial equilibrium
value (different from 0) of 3.5, in fact, in this case is equal to αα,
which is less than 1 for α < 1. This indicates the (obvious) ne-
cessity of interpersonal relations and subsequent synergies for
every human (and non human) gathering and, above all, it is
true for every technological level.
We must underline, in fact, that 3.1 can describe every phe-
nomenon of concentration, at any level, from microscopic (cel-
lular) to macroscopic (population) scale. When from the con-
centration of some units derives a growth of disposable re-
sources, due to synergies, if the effect of synergies surpasses
the cost of exploitation of the area which they exploit, the con-
centration happens.
Moreover, in the formula the value of S depends on inter-
personal relations, which are not only of altruistic kind. Even
the egoists may wish to concentrate in a place. It is sufficient, in
fact, that they are available to trade or to cooperate with the
other components of the group rather than to prey them, in or-
der to generate synergies, even if more limited see (Forges
Davanzati & Paolilli, 2004) and (Paolilli, 2009).
In the next section we will show that among humans the rela-
tions, and therefore the concentration of the population, are
driven by a necessary combination of altruism and selfishness.
If two groups, at the same technological level, are so close to
each other to compete on the resources, even a small difference
in the degree of internal cohesion (here expressed by S) is ob-
viously enough to determine a clear prevalence of the most
cohesive group, especially if they are numerically comparable.
In this regard such a behavior has been recently shown by
(Paolilli & Pollice, 2011), even if the model used in that context
is different from that we have here presented (particularly the
distance between the two groups, for the sake of simplicity, is
not explicitly considered).
5For the sake of simplicity we assume that the concentration happens at a
point, rather than on a surface.
6S and α are probably linked by a feedback relation, because it is possi
b
le
that technological progress makes interpersonal relations more efficient,
while it is quite certain that the more intense the latter, the more frequent are
the innovations, which while often being the output of individual effort, are
also favored by culture, evidently linked to a social context.
Group Selection and Its Influence on the Group
The appearance of more complex types of interpersonal rela-
tions (nodal structure, control mechanisms) will lead to differ-
ent degrees of efficiency in the groups, which will influence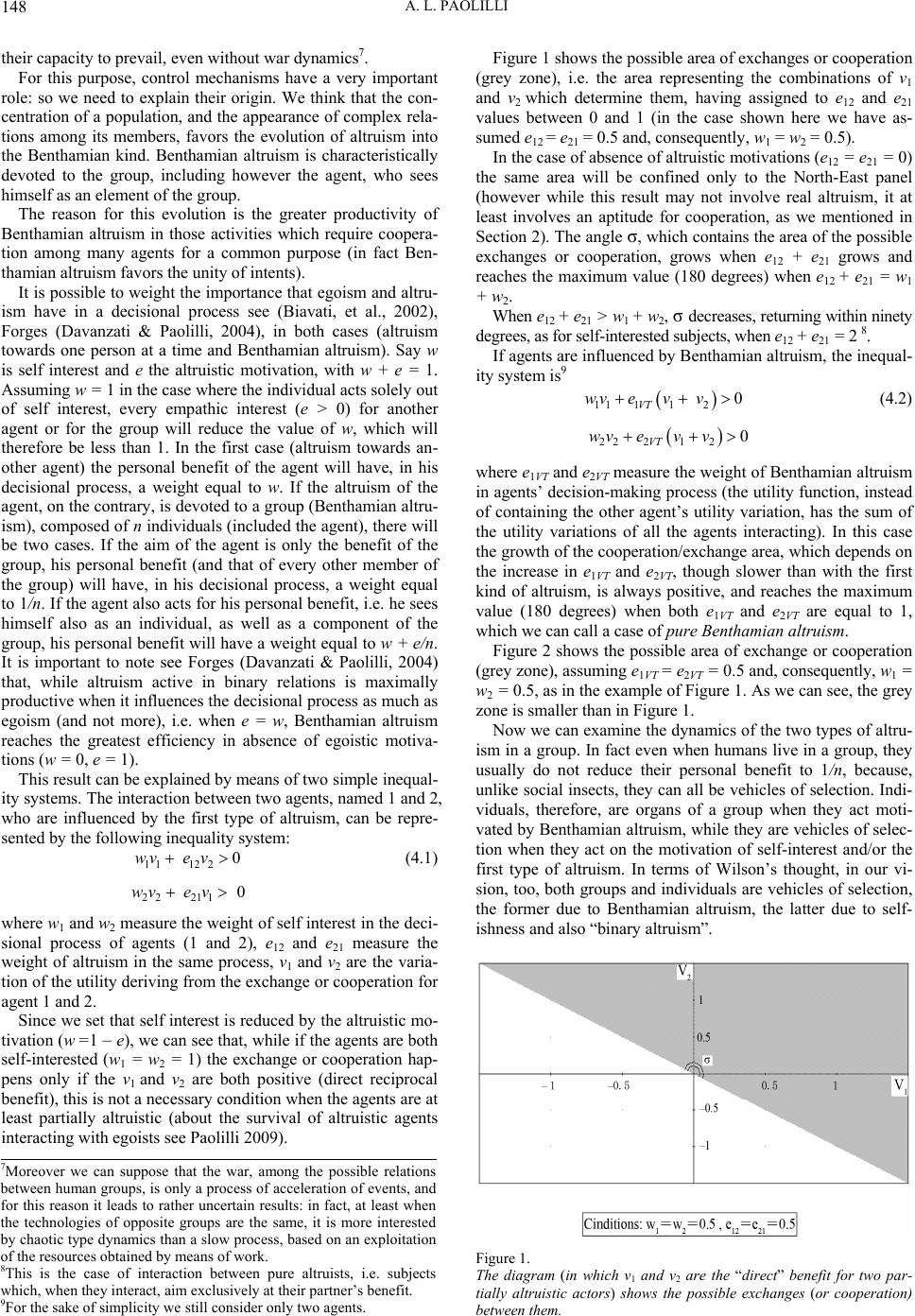

A. L. PAOLILLI
148
their capacity to prevail, even without war dynamics7.
For this purpose, control mechanisms have a very important
role: so we need to explain their origin. We think that the con-
centration of a population, and the appearance of complex rela-
tions among its members, favors the evolution of altruism into
the Benthamian kind. Benthamian altruism is characteristically
devoted to the group, including however the agent, who sees
himself as an element of the group.
The reason for this evolution is the greater productivity of
Benthamian altruism in those activities which require coopera-
tion among many agents for a common purpose (in fact Ben-
thamian altruism favors the unity of intents).
It is possible to weight the importance that egoism and altru-
ism have in a decisional process see (Biavati, et al., 2002),
Forges (Davanzati & Paolilli, 2004), in both cases (altruism
towards one person at a time and Benthamian altruism). Say w
is self interest and e the altruistic motivation, with w + e = 1.
Assuming w = 1 in the case where the individual acts solely out
of self interest, every empathic interest (e > 0) for another
agent or for the group will reduce the value of w, which will
therefore be less than 1. In the first case (altruism towards an-
other agent) the personal benefit of the agent will have, in his
decisional process, a weight equal to w. If the altruism of the
agent, on the contrary, is devoted to a group (Benthamian altru-
ism), composed of n individuals (included the agent), there will
be two cases. If the aim of the agent is only the benefit of the
group, his personal benefit (and that of every other member of
the group) will have, in his decisional process, a weight equal
to 1/n. If the agent also acts for his personal benefit, i.e. he sees
himself also as an individual, as well as a component of the
group, his personal benefit will have a weight equal to w + e/n.
It is important to note see Forges (Davanzati & Paolilli, 2004)
that, while altruism active in binary relations is maximally
productive when it influences the decisional process as much as
egoism (and not more), i.e. when e = w, Benthamian altruism
reaches the greatest efficiency in absence of egoistic motiva-
tions (w = 0, e = 1).
This result can be explained by means of two simple inequal-
ity systems. The interaction between two agents, named 1 and 2,
who are influenced by the first type of altruism, can be repre-
sented by the following inequality system:
1112 2
wve v0
0
(4.1)
22 211
wve v
where w1 and w2 measure the weight of self interest in the deci-
sional process of agents (1 and 2), e12 and e21 measure the
weight of altruism in the same process, v1 and v2 are the varia-
tion of the utility deriving from the exchange or cooperation for
agent 1 and 2.
Since we set that self interest is reduced by the altruistic mo-
tivation (w =1 – e), we can see that, while if the agents are both
self-interested (w1 = w2 = 1) the exchange or cooperation hap-
pens only if the v1 and v2 are both positive (direct reciprocal
benefit), this is not a necessary condition when the agents are at
least partially altruistic (about the survival of altruistic agents
interacting with egoists see Paolilli 2009).
Figure 1 shows the possible area of exchanges or cooperation
(grey zone), i.e. the area representing the combinations of v1
and v2 which determine them, having assigned to e12 and e21
values between 0 and 1 (in the case shown here we have as-
sumed e12 = e21 = 0.5 and, consequently, w1 = w2 = 0.5).
In the case of absence of altruistic motivations (e12 = e21 = 0)
the same area will be confined only to the North-East panel
(however while this result may not involve real altruism, it at
least involves an aptitude for cooperation, as we mentioned in
Section 2). The angle σ, which contains the area of the possible
exchanges or cooperation, grows when e12 + e21 grows and
reaches the maximum value (180 degrees) when e12 + e21 = w1
+ w2.
When e12 + e21 > w1 + w2, σ decreases, returning within ninety
degrees, as for self-interested subjects, when e12 + e21 = 2 8.
If agents are influenced by Benthamian altruism, the inequal-
ity system is9


11 112 VT
wv evv0

 (4.2)


222120 VT
wvev v


where e1VT and e2VT measure the weight of Benthamian altruism
in agents’ decision-making process (the utility function, instead
of containing the other agent’s utility variation, has the sum of
the utility variations of all the agents interacting). In this case
the growth of the cooperation/exchange area, which depends on
the increase in e1VT and e2VT, though slower than with the first
kind of altruism, is always positive, and reaches the maximum
value (180 degrees) when both e1VT and e2VT are equal to 1,
which we can call a case of pure Benthamian altruism.
Figure 2 shows the possible area of exchange or cooperation
(grey zone), assuming e1VT = e2VT = 0.5 and, consequently, w1 =
w2 = 0.5, as in the example of Figure 1. As we can see, the grey
zone is smaller than in Figure 1.
Now we can examine the dynamics of the two types of altru-
ism in a group. In fact even when humans live in a group, they
usually do not reduce their personal benefit to 1/n, because,
unlike social insects, they can all be vehicles of selection. Indi-
viduals, therefore, are organs of a group when they act moti-
vated by Benthamian altruism, while they are vehicles of selec-
tion when they act on the motivation of self-interest and/or the
first type of altruism. In terms of Wilson’s thought, in our vi-
sion, too, both groups and individuals are vehicles of selection,
the former due to Benthamian altruism, the latter due to self-
ishness and also “binary altruism”.
7Moreover we can suppose that the war, among the possible relations
b
etween human groups, is only a process of acceleration of events, and
for this reason it leads to rather uncertain results: in fact, at least when
the technologies of opposite groups are the same, it is more interested
b
y chaotic type dynamics than a slow process, based on an exploitation
of the resources obtained by means of work.
8This is the case of interaction between pure altruists, i.e. subjects
which, when they interact, aim exclusively at their partner’s benefit.
9For the sake of simplicity we still consider only two agents.
Figure 1.
The diagram (in which v1 and v2 are the “direct” benefit for two par-
tially altruistic actors) shows the possible exchanges (or cooperation)
between them.

A. L. PAOLILLI 149
Figure 2.
The diagram shows the possible exchanges (or cooperation) between
two partially Benthamian altruists.
When a hierarchic organization of interpersonal relations
appears, therefore, many binary relations continue to exist. In
modern societies, for example, this happens in friendship and in
the market; the same teams interact in the market by means of
binary relations. In these relations, as we have pointed out, the
presence of egoism, beside altruism, increases effort and there-
fore productivity.
However, if a person who gives to an egoist acts because he
is moved by the first type of altruism, this action, if it is per-
ceived as detrimental to the group, will be hampered by the
Benthamian altruists. Benthamian altruism can thus be related
to the sense of justice see (Khalil, 2003)10.
At the light of these considerations we can also explain the
genesis and the role of sentiments and behaviors, such as envy
and gossip, seemingly the opposite to altruism, but which have
a great effect on human behavior11. Envy is a perception that
someone else unjustly or without merit obtains resources or
status and is essentially an outcome of the interaction between
Benthamian altruism and selfishness.
Gossip, on the other hand, is the morbid aspect of the infor-
mative activity (but it is precisely its morbidity that makes this
activity attractive), in turn a consequence, and necessary for the
aims of Benthamian altruism itself. If regarded from this view-
point, behaviors and sentiments usually detested, but very
widespread, such as gossip and envy (the former partially de-
pendent on the latter; another cause of gossip could be admira-
tion and the spirit of emulation, attitudes we can define as
adaptive, because they incentive the productivity), are justified
by the social control that they permit. They are essentially atti-
tudes which, as long as they do not destroy the cooperative
spirit, can be productive for the group, in a context like the
human one in which, due to the fact that all individuals are
vehicles of selection, selfishness cannot disappear.
Conclusions
We can conclude that evolution may have favored the emer-
gence in humans of altruistic attitudes for the reasons evidenced
by (Paolilli, 2009), i.e. the existence of growing marginal out-
puts dependent on the effort of the agents. However, the prob-
able existence, for some activities, of average outputs that grow
depending on the increase in cooperative subjects, may have
favored the appearance of teams composed of more than two
individuals. Moreover, the need to reduce the interaction costs
stimulated more stable relations, leading to a concentration of
populations in the space. This concentration favored the emer-
gence of group selection. In competition for common resources,
the more efficient groups prevailed, and precisely those which
had, along with Benthamian altruism (typically devoted to the
group), developed a nodal organization in teams composed of
more than two individuals and between teams as well. They had
also developed more efficient control mechanisms (sense of
justice and, in some cases, also envy and gossip). These control
mechanisms are a direct consequence of Benthamian altruism
and its interaction with selfishness, which maintains its utility
at least in binary relations and at any rate cannot disappear from
the human genre since all humans are vehicles of reproduction
and selection. From this viewpoint, therefore, altruism gener-
ates groups (there are no groups without altruism or, at least,
cooperative attitudes), and it is only after this that group selec-
tion stimulates, rather than the prevalence of altruism within the
groups, its evolution into Benthamian altruism and the appear-
ance of control mechanisms.
References
Alexander, R. D. (1987). The biology of moral systems. New York:
Aldine de Gruyter.
Altman, M. (1996). Human agency and material welfare: Revisions in
microeconomics and their implications for public policy. Boston,
Dordrecht, London: Kluwer Academic Publishers.
Altman, M. (2001). Worker satisfaction and economic performance.
Armonk, New York: M. E. Sharpe Publishers.
Altman, M. (2005). Reconciling altruistic, moralistic, and ethical be-
havior with the rational economic agent and competitive markets.
Journal of Economic Psychology, 26, 732-757.
doi:10.1016/j.joep.2005.06.004
Altman, M. (2006). Opening-up the objective function: choice behavior
and economic and non-economic variables-core and marginal altru-
ism. Economics Bulletin, 4, 1-11.
Axelrod, R., & Hamilton, W. D. (1981). The evolution of cooperation.
Science, 211, 1390-1396. doi:10.1126/science.7466396
Bergstrom, T. C., & Stark, O. (1993). How altruism can prevail in an
evolutionary environment. The American Economic Review, 83, 149-
155.
Biavati, M., et al. (2002). Preferenze endogene e dinamiche relazionali:
Un modello coevolutivo. In P. L. Saccoand, & S. Zamagni (Eds.),
Complessità relazionale e comportamento economico (pp. 431-485).
Bologna: Il Mulino.
Bowles, S., & Gintis, H. (2003). The origins of human cooperation. In
P. Hammerstein (Ed.), Genetic and cultural evolution of cooperation.
Cambridgwe, MA: MIT Press, 430-443.
Bowles, S., & Choi, J.-K. (2003). The co-evolution of love and hate.
Quaderni, 401, Università degli Studi di Siena.
Cosmides, L., & Toby, J. (1996). Are humans good intuitive statisti-
cians after all? Rethinking some conclusions from the literature on
judgment under uncertainty. Cognition, 58, 1-73.
doi:10.1016/0010-0277(95)00664-8
Dawkins, R. (1976), The selfish gene, Oxford: Oxford University Press.
10Though (Alexander, 1987) does not believe in group selection, he
compares human moral systems to the genetic rules of meiosis. As the
latter eliminate fitness differences among genes, human moral systems
have the same role for the members of a group.
11Envy, in particular, and its role, have been studied by several scholars
(see, among others, Vignolo, 2005), about the role of envy in coordina-
tion).
Eberhard, M. J. W. (1975). The evolution of social behavior by kin
selection. The Quarterly Review of Biology, 50, 1-33.
doi:10.1086/408298
Fehr, E., Kirchsteiger, & G., Riedl, A. (1993). Does fairness prevent
market clearing? An experimental investigation. The Quarterly Journal
of Economics, 108, 437-459. doi:10.2307/2118338
Field, A. J. (2001). Altruistically inclined: The behavioral sciences,

A. L. PAOLILLI
150
evolutionary theory and the origins of reciprocity. Ann Arbor, MI:
University of Michigan Press.
Forges Davanzati, G., & Paolilli, A. L. (2004). Altruismo, scambi e
sviluppo economico. In L. Tundo Ferente (Eds.), La responsabilità
del pensare (pp. 289-307). Napoli: Liguori Editore.
Forsythe, R., et al. (1994). Fairness in simple bargaining experiments.
Games and Economic Behavior, 6, 347-369.
doi:10.1006/game.1994.1021
Frank, S.A. (1998). Foundation of social evolution. Princeton: Prince-
ton University Press.
Frank, S.A. (2006). Social selection. In C. W. Fox, & J. B. Wolf (Eds.),
Evolutionary genetics: Concepts and case studies (pp. 350-363).
New York: Oxford University Press.
Gigerenzer, G., & Hoffrage, U. (1995). How to improve Bayesian
reasoning without instruction: Frequency format. Psychological Re-
view, 102, 684-704. doi:10.1037/0033-295X.102.4.684
Gintis, H. (2000). Strong reciprocity and human sociality. Journal of
Theoretical Biology, 206, 169-179. doi:10.1006/jtbi.2000.2111
Gintis, H. (2003). The Hitchhiker’s guide to altruism: Genes, culture,
and the internalization of norms. Journal of Theoretical Biology, 220,
407-418. doi:10.1006/jtbi.2003.3104
Güth, W., et al. (1982). An experimental analysis of ultimatum bar-
gaining. Journal of Economic Behavior and Organization, 3, 367-
388. doi:10.1016/0167-2681(82)90011-7
Güth, W. (1995). On ultimatum bargaining experiments—A personal
review. Journal of Economic Behavior and Organization, 27, 329-
344. doi:10.1016/0167-2681(94)00071-L
Khalil, E. L. (2003). What is altruism? Journal of Economic Psychol-
ogy, 1, 97-123.
Leibenstein, H. (1966). Allocative efficiency vs. “x-efficiency”. Ameri-
can Economic Review, 56, 392-415.
Leibenstein, H. (1973). Notes on x-efficiency and technical change. In
E. B. Ayal (Eds.), Micro-aspects of development (pp. 18-38). New
York: Praeger.
Lorenz, K. (1994). L’aggressività. Milano: Il Saggiatore. Italian trans-
lation of Das sogenannte böse: Zur naturgeschichte der aggression
(1963). Wien: Verlag.
North, D. C. (1990). Institutions, institutional change and economic
performance. Cambridge: Cambridge University Press.
Paolilli, A. L. (2009). About the “economic” origin of altruism. The
Journal of Socio-Economics, 38, 60-71.
doi:10.1016/j.socec.2008.08.006
Paolilli, A. L., & Pollice, F. (2011). Trajectories of State Formation in
Eurasia: a Discussion. Historical Social Research, 136, 343-376.
Price, G. R. (1970). Selection and covariance. Nature, 227, 520-521.
doi:10.1038/227520a0
Reilly, W. J. (1929). Methods for the study of retail relationships. Uni-
versity of Texas Bulletin, Monograph 4, n. 2944.
Simon, H. A. (1957). Models of man: Social and rational. New York:
Wiley.
Simon, H. A. (1983). Reason in human affairs. Stanford: Stanford
University Press.
Simon, H. A. (1990). A mechanism for social selection and successful
altruism. Science, 250, 1665-1668. doi:10.1126/science.2270480
Simon, H. A. (1992). Bounded rationality and the cognitive revolution.
Elgar: Aldershot.
Simon, H. A. (1993). Altruism and economics. The American Eco-
nomic Review, 83, 156-161.
Sober, E. (1991). Organisms, individuals, and units of selection. In A. I.
Tauber (Eds.), Organism and the origins of self (pp. 275-296).
Dordrecht, Netherlands: Kluwer. doi:10.1007/978-94-011-3406-4_13
Sober, E., & Wilson, D. S. (1998). Unto others: Evolution and psy-
chology of unselfish behavior. Cambridge, MA: Harvard University
Press.
Tversky, A., & Kahnemann, D. (1974). Judgment under uncertainty:
heuristics and biases. Science, 185, 1124-1131.
doi:10.1126/science.185.4157.1124
Vignolo, T. (2005). When envy helps explain coordination. Economics
Bulletin, 3, 1-7.
Williams, G. C. (1966). Adaptation and natural selection: A critique of
some current evolutionary thought. Princeton: Princeton University
Press.
Wilson, D. S., & Sober, E. (1994). Reintroducing group selection to the
human behavioral sciences. Behavioral and Brain Sciences, 17, 585-
654. doi:10.1017/S0140525X00036104
Winne-Edwards, V. C. (1962). Animal dispersion in relation to social
behaviour. New York: Hafner.
Witt, U. (1985). Imagination and leadership-the neglected dimension of
an evolutionary theory of the firm. Journal of Economic Behavior &
Organization, 35, 161-177. doi:10.1016/S0167-2681(98)00058-4
Witt, U., & Yaary, M. (1992). An evolutionary approach to explain
reciprocal behavior in a simple strategic game. In: U. Witt (Eds.),
Explaining process and change—Approaches to evolutionary eco-
nomics (pp. 23-34). Ann Arbor: University of Michigan Press.