Applied Mathematics, 2011, 2, 1196-1203
doi:10.4236/am.2011.210166 Published Online October 2011 (http://www.SciRP.org/journal/am)
Self-Similar Solution of Heat and Mass Transfer
of Unsteady Mixed Convection Flow on a Rotating
Cone Embedded in a Porous Medium Saturated
with a Rotating Fluid
Saleh M. Al-Harbi
Department of Mathematics, Makkah Teacher College, Makkah, Saudi Arabia
Received June 6, 2011; revised August 22, 2011; accepted August 29, 2011
Abstract
A self-similar solution of unsteady mixed convection flow on a rotating cone embedded in a porous medium
saturated with a rotating fluid in the presence of the first and second orders resistances has been obtained. It
has been shown that a self-similar solution is possible when the free stream angular velocity and the angular
velocity of the cone vary inversely as a linear function of time. The system of ordinary differential equations
governing the flow has been solved numerically using an implicit finite difference scheme in combination
with the quasi-linearization technique. Both prescribe wall temperature and prescribed heat flux conditions
are considered. Numerical results are reported for the skin friction coefficients, Nusselt number and Sher-
wood number. The effect of various parameters on the velocity, temperature and concentration profiles are
also presented here.
Keywords: Unsteady Mixed Convection, Heat and Mass Transfer, Rotating Cone, Rotating Fluid, Porous
Media, Self-Similar Solution
1. Introduction
The study and analysis of heat and mass transfer in po-
rous media has been the subject of many investigations
due to their frequent occurrence in industrial and tech-
nological applications. Examples of some applications
include geothermal reservoirs, drying of porous solids,
thermal insulation, enhanced oil recovery and many oth-
ers. Cone–shaped bodies are often encountered in many
engineering applications and the heat transfer problem of
mixed convection boundary layer flow over a rotating
cone, which occurs in rotating heat exchangers, are ex-
tensively used by the chemical and automobile industries.
Moreover, convective heat on a rotating cone has several
important applications such as design of canisters for
nuclear waste disposal, nuclear reactor cooling system,
geothermal reservoirs. Earlier investigations of flow and
heat transfer in rotating systems are given by Hartnett
and Deland [1], Hering and Grosh [2] and Tien and Tsuji
[3]. Hering and Grosh [4] have obtained a number of
similarity solutions for cones with prescribed wall tem-
perature being a power function of the distance from the
apex along the generator. Himasekhar et al. [5] found the
similarity solution of the mixed convection flow over a
vertical rotating cone in an ambient fluid for a wide
range of Prandtl numbers. Wang [6] has also obtained a
similarity solution of boundary layer flows on rotating
cones, discs and axisymmetric bodies with concentrated
heat sources. Further, Yih [7] has presented non-similar
solutions to study the heat transfer characteristics in
mixed convection about a cone in saturated porous media.
The effect of thermal radiation on the non-Darcy natural
convection flow over a vertical cone and wedge embed-
ded in a porous medium with variable viscosity and wall
mass flux was investigated numerically by EL-Harby [8].
All these studies pertain to steady flows.
There is a large body of literature on unsteady, bound-
ary-layer flows past bodies of different geometries em-
bedded in porous media. Takhar et al. [9] have presented
a study on unsteady mixed convection flow over a verti-
cal cone rotating in an ambient fluid with a time-de-
pendent angular velocity in the presence of a magnetic

S. M. AL-HARBI
1197
field. Therefore, as a step towards the eventual develop-
ment of studies on unsteady mixed convection flows, it is
interesting as well as useful to investigate the combined
effects of thermal and mass diffusion on a rotating cone
in a rotating viscous fluid where the angular velocity of
the cone and the free stream angular velocity vary arbi-
trarily with time. The problem of unsteady mixed con-
vection flow on a rotating cone in a rotating fluid has
been considered by Anilkumar and Roy [10]. The inter-
ested reader can find an excellent collection of papers on
unsteady convective flow problems over heated bodies
embedded in a fluid-saturated porous medium in the
book papers by Pop and Ingham [11] and in the book by
Nield and Bejan [12]. Hassanien et al. [13] have studied
the unsteady free convection flow in the stagnation-point
region of a rotating sphere embedded in a porous me-
dium. Also, the problem of unsteady free convection flow
in the stagnation-point region of a three-dimensional
body embedded in a porous media has been studied by
Hassanien et al. [14]. Recently, the problem of unsteady
MHD free convection flow past a semi infinite vertical
permeable moving plate with heat source and suction has
been studied by Ibrahim et al. [15]. Roy et al. [16] have
obtained Non-similar solution of an unsteady mixed
convection flow over a vertical cone in the presence of
surface mass transfer. The effect of combined viscous
dissipation and Joule heating on unsteady mixed conven-
tion magnetohydrodynamics (MHD) flow on a rotating
cone in an electrically conducting rotating fluid in the
presence of Hall and ion-slip currents was investigated
by Osalusi et al. [17].
The aim of the present paper is to develop a new
self–similarity solutions for the heat and mass transfer of
unsteady mixed convection flow on a rotating cone em-
bedded in a porous medium saturated with a rotating
fluid in the presence of the first and second orders resis-
tances which to the best of our knowledge have not been
investigated yet. The system of ordinary differential
equations governing the flow has been solved numeri-
cally using the method of an implicit finite difference
scheme.
2. Mathematical Analysis
We consider the unsteady laminar viscous incompressi-
ble fluid flowing over an infinite rotating cone in a rotat-
ing fluid–saturated porous medium. Both the cone and
the fluid are rotating about the axis of the cone with
time-dependent angular velocities either in the same di-
rection or in the opposite direction. This introduces un-
steadiness in the flow field. We have taken the rectangu-
lar co-ordinate system (x, y, z) where x is measured along
a meridian section, the y-axis along a circular section and
z-axis normal to the cone surface as shown in Figure 1.
Let u, v and w be the velocity components along x (tan-
gential), y (circumferential) and z (normal) directions,
respectively.
The buoyancy forces arise due to the temperature and
concentration variations in the fluid and the flow is taken
to be axi-symmetric. The wall and the free stream are
maintained at a constant temperature and concentration.
Under the above assumptions and using the Boussinesq
approximation, the governing boundary layer momentum,
energy and diffusion equation can be expressed as:




0,
xu xw
xz



 (1)


2 22
2
2
2
1/2 cos
cos ,
e
v uu uvu
uw
txzxxz
uug TT
kK
gCC

 





 
 
 

 


(2)
2
2,
e
v vv vuv
uw
txzxt z

v

 
 
  (3)
2
2,
TT TT
uw
txz z

 
 
  (4)
2
2.
CC CC
uwD
txz z
 

  (5)
The initial conditions are






0,,( ,),
0, ,,,
0, ,,,
0, ,,,
0, ,,,
i
i
i
i
i
uxzuxz
vxzvxz
wxzuxz
TxzTxz
CxzCxz








(6)

1

,yv
,
x
u
,
z
w
or
ww
Tq
2
T
0


Figure 1. Physical model and coordinate system.

1198 S. M. AL-HARBI
and the boundary conditions are given by



 



 
1
1
1
2
,,0,,0 0,
,,0sin 1,
,,0and ,,0,
,, 0,
,,sin 1,
,,and ,,,
ww
utx wtx
vtx xst
Ttx TCtx C
utx
vtx xst
TtxT CtxC








 



 
(7)
here

 is the semi-vertical angle of the cone;

is
the kinematics viscosity;

is the density; t and
t
are the dimensional and dimensionless
times, respectively; 1
 and 2 are the angular ve-
locities of the cone and the fluid far away from the sur-
face, respectively; 12
is the composite an-
gular velocity; K and are the respective permeability
and the inertia coefficient of the porous medium;

tx





sin 







is
the porosity; g is the acceleration due to gravity; T is the
temperature; C is the species concentration;

is the
volumetric co-efficient of thermal expansion;


is the
volumetric co-efficient of expansion for concentration;

and D are thermal and mass diffusivity, respectively;
Subscripts t, x and z denote partial derivatives with re-
spect to the corresponding variables and the subscripts e,
i, w and denote the conditions at the edge of the
boundary layer, initial conditions, conditions at the wall
and free stream conditions, respectively; , ,

0
T0 C T

and are constants.
C
Equations (1)-(5) are a system of partial differential
equations with three independent variables x, z and t. It
has been found that these partial differential equations
can be reduced to a system of ordinary differential equa-
tions, if we take the velocity at the edge of the boundary
layer e and the angular velocity of the cone to vary
inversely as a linear function of time. Consequently, ap-
plying the following transformations:
v












1
2
1/2 1/2
1
1
1/ 21/ 2
sin 1,
sin 1,
sin ,
,,12 sin1,
,,sin 1,
,,sin1,
e
vx st
st z
tt
utxzst f
vtxzxst g
wtxzst f



















 
 


 
 
 
(8)
to Equations (1)-(5), we find that the continuity Equation
(1) is identically satisfied, and Equations (2)-(5) reduce
to,


2
22
1
1
2
121
2
1
22
0,
fff fg
Nsff
ff

 


 
 











(9)

1
2
1
12
0,
g
fg gfsgg
gg











(10)
111
Pr2 0,
22
ffs  

 






(11)
111
20
22
Scff s  

 ,







(12)
where


Re
L
L
Da   is the first resistance parame-
ter, is the second resistance parameter,
21
2
x
Da 

 /2
x
2
Re sinL

 
L
 is the Reynolds number,
2
L
DaKL is the Darcy number, 2
11
Re ,
L
Gr  
2
22
Re
L
Gr   are the buoyancy parameters


32
10 cos ,GrTTLg







32
20 cosGrTTLg





are the Grashof numbers, 11

  is the angular ve-
locity of the cone to the composite angular velocity,
21
N



is the ratio of Grashof numbers, Pr



is the Prandtl number and Sc D

 is the Schmidt
number. s is the parameter characterizing the unsteady-
ness in the free stream velocity

1
21sin e
vxst




 
0 1st. The flow is accelerating if provided
s

and
the flow decelerating if 0.s

Further, 10

 implies
that the cone is stationary and the fluid is rotating,
11


represents the case where the cone is rotating in
an ambient fluid, and for 10.5

, the cone and the
fluid are rotating with equal angular velocity in the same
direction. The ratio of Grashof numbers denoted by the
parameter N measures the relative importance of thermal
diffusion in inducing the buoyancy forces which drive
the flow. N = 0 for no species diffusion, infinite for the
thermal diffusion, positive for the case when the buoy-
ancy forces due to temperature difference act in the same
direction and negative when they act in the opposite di-
rection.
The boundary conditions Equation (7) can be expressed
as






 
1
1
000,0 ,001
0,1 ,0.
ffg
fg
 
 
,



 

(13)
Here

is the similarity variable; f is the dimen-
sionless stream function;
f
 and g are, the respectively
dimensionless velocity along x- and y- directions;

and

S. M. AL-HARBI
1199

are the dimensionless temperature and concentra-
tion.
The set of partial differential Equations (1)-(5) gov-
erning the flow has to be solved subjected to initial con-
ditions (6) and boundary conditions (7). The ordinary
differential Equations (9)-(12) under the boundary condi-
tions (13) are solved using the self-similar solution
which implies that the solution at different times may be
reduced to a single solutions i.e., the solution at the one
value of time t is similar to the solution at any other
value of time t. This similarity property permits a de-
crease in the number of independent variables from three
to one (in the present case) and yields treatment using
ordinary differential equations instead of partial differen-
tial equations.
The quantities of physical interest are as follows:
The surface skin friction co-efficient in x- and y- di-
rections are, respectively, given by



2
1
0
1/2
2sin1
Re0 ,
fx z
x
Cuzxst
f









 









2
1
0
1/2
2sin1
Re0 .
fy z
x
Cvzx st
g








 












Thus


1/2
1/2
Re0 ,
Re0 ,
fx x
fy x
Cf
Cg



 (14)
where

1
2
Resin 1.
xxst





The Nusselt number and Sherwood number can be ex-
pressed as:


1/ 2
1/2
Re 0
Re0 ,
x
x
Nu
Sh






  (15)
where
 
0w z
NukT zTT

  
and
 
0w z
ShDCzCC 


 
3. Results and Discussion
The similarity Equations (9)-(12) are coupled nonlinear
and exhibit no closed-form solution. Therefore, they
must be solved numerically subject to the boundary con-
ditions. The implicit finite-difference method with itera-
tion similar to that discussed by Inouye and Tate [18]
have proven to be successful for the solution of such
equations and for this reason, it will be employed herein.
The computations have been carried out with 0.01



(0 5), for various values of , Pr(7Pr 10)




11
( 0.251.0),



 (0.22 2.57)
,
Sc Sc (1 1),ss


( 0.51.0),NN

 0.0,0.5,1.0,


. The
edge of the boundary layer
0.0,0.5,1.0

 is taken between 4 and 6
depending on the values of parameters. In order to verify
the correctness of our method, we have compared our
results with those of Himasekher et al. [5] and Anilku-
mar and Roy [10]. The results are found to be in excel-
lent agreement and some of the comparisons are shown
in Tables 1 and 2. Also the effects of

and

on the
skin friction coefficients, Nusselt number and Sherwood
number (1/ 2
Re
f
xx
C, 1/ 2 Re
f
yx
1/2
Rex Nu  C, , 1/2 Re
x
Sh

) are
presented in Tabl e 3.
Figures 2-5 show the effect of the first resistance pa-
rameter γ, the second resistance parameter Δ and the
buoyancy parameter

on the velocity profiles in the
tangential direction


f


, on the temperature profiles



 and on the concentration profiles


 for accel-
erating flows s0.5

, 1.0N

, and P0.Sc 94 r 0.7

.
The presence of a porous medium in the flow presents
resistance to flow, thus, slowing the flow and increasing
the pressure drop across it.
Therefore, as the first and second resistances increases,
Table 1. Comparison of the results (–f''(0), –g'(0), –θ'(0))
with those of Himasekher et al. [5] and Anilkumar and Roy
[10].
Present results
Pr 
(0)f



(0)g  (0)



1.0189 0.6155 0.4347
0.0
1.0256* 0.6158* 0.4299*
2.07736 0.84997 0.61173
1.0
2.2021* 0.8496* 0.6120*
8.52447 1.40365 1.01729
0.7
10
8.5041* 1.3990* 1.0097*
0.0 1.0189 0.6155 0.51858
1
1.0 2.00333 0.82459 0.7003
Anilkumar and Roy [10]
0.0 1.0199 0.6160 0.4305
1.0 2.1757 0.8499 0.6127
0.7
10 8.5029 1.4061 1.0175
0.0 1.0199 0.6160 0.51808
1
1.0 2.0627 0.8250 0.7005
*Values taken from Himasekher et al. [5].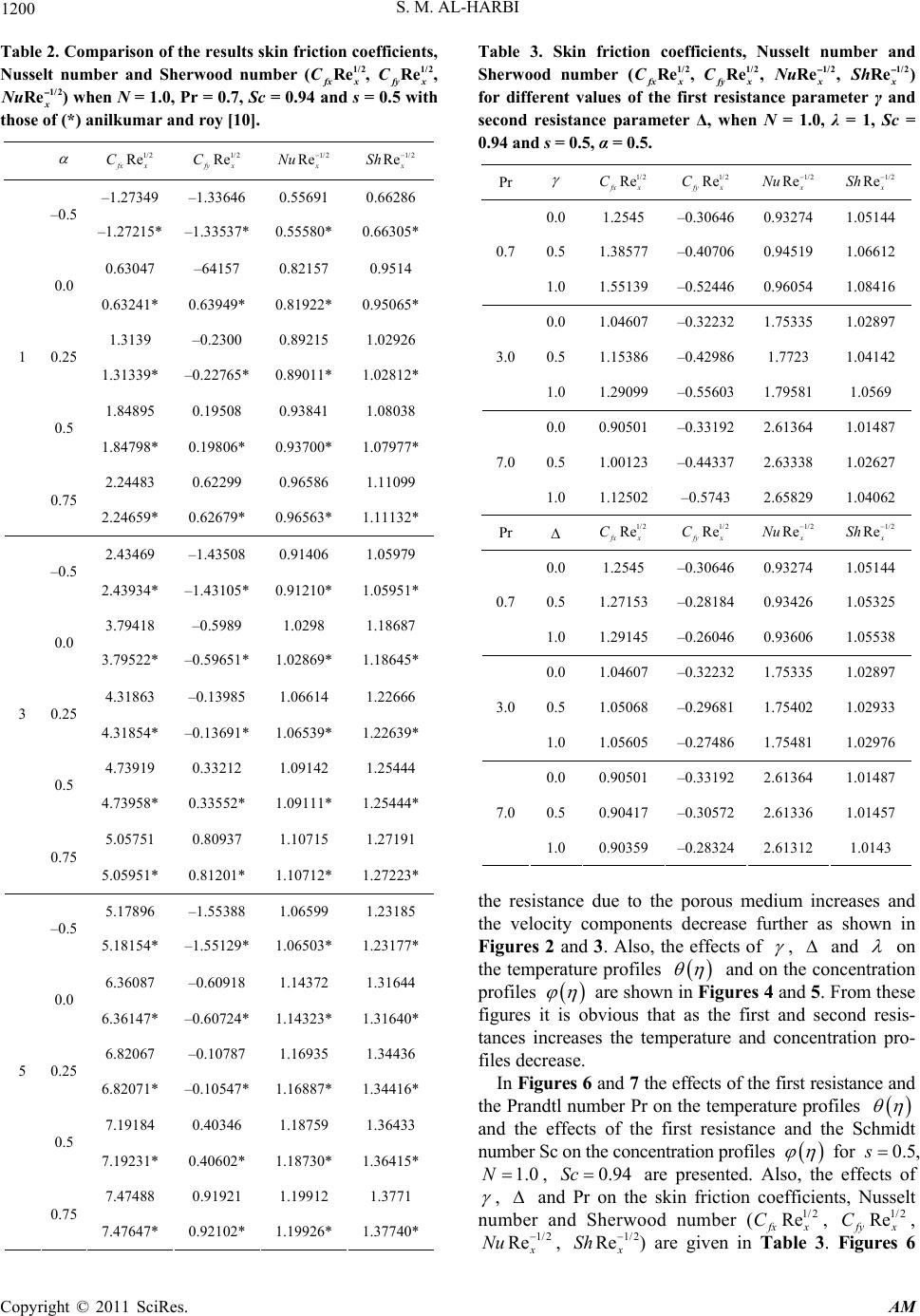

1200 S. M. AL-HARBI
Table 2. Comparison of the results skin friction coefficients,
Nusselt number and Sherwood number (1/2
Re
f
xx
C, ,
) when N = 1.0, Pr = 0.7, Sc = 0.94 and s = 0.5 with
those of (*) anilkumar and roy [10].
1/2
Re
fy x
C
1/2
Rex Nu 

1/ 2 Re
fx x
C 1/ 2 Re
fy x
C 1/2 Rex Nu  1/ 2 Rex Sh 
–1.27349 –1.33646 0.55691 0.66286
–0.5
–1.27215* –1.33537* 0.55580* 0.66305*
0.63047 –64157 0.82157 0.9514
0.0
0.63241* 0.63949* 0.81922* 0.95065*
1.3139 –0.2300 0.89215 1.02926
0.25
1.31339* –0.22765* 0.89011* 1.02812*
1.84895 0.19508 0.93841 1.08038
0.5
1.84798* 0.19806* 0.93700* 1.07977*
2.24483 0.62299 0.96586 1.11099
1
0.75
2.24659* 0.62679* 0.96563* 1.11132*
2.43469 –1.43508 0.91406 1.05979
–0.5
2.43934* –1.43105* 0.91210* 1.05951*
3.79418 –0.5989 1.0298 1.18687
0.0
3.79522* –0.59651* 1.02869* 1.18645*
4.31863 –0.13985 1.06614 1.22666
0.25
4.31854* –0.13691* 1.06539* 1.22639*
4.73919 0.33212 1.09142 1.25444
0.5
4.73958* 0.33552* 1.09111* 1.25444*
5.05751 0.80937 1.10715 1.27191
3
0.75
5.05951* 0.81201* 1.10712* 1.27223*
5.17896 –1.55388 1.06599 1.23185
–0.5
5.18154* –1.55129* 1.06503* 1.23177*
6.36087 –0.60918 1.14372 1.31644
0.0
6.36147* –0.60724* 1.14323* 1.31640*
6.82067 –0.10787 1.16935 1.34436
0.25
6.82071* –0.10547* 1.16887* 1.34416*
7.19184 0.40346 1.18759 1.36433
0.5
7.19231* 0.40602* 1.18730* 1.36415*
7.47488 0.91921 1.19912 1.3771
5
0.75
7.47647* 0.92102* 1.19926* 1.37740*
Table 3. Skin friction coefficients, Nusselt number and
Sherwood number (, , ,
1/2
Re
fx x
C1/2
Re
fy x
C1/2
Rex Nu 1/2 Rex Sh

)
for different values of the first resistance parameter γ and
second resistance parameter Δ, when N = 1.0, λ = 1, Sc =
0.94 and s = 0.5, α = 0.5.
Pr

1/ 2
Re
fx x
C1/ 2 Re
fy x
C 1/2 Rex Nu  1/2 Rex Sh 
0.0 1.2545 –0.30646 0.93274 1.05144
0.5 1.38577 –0.40706 0.94519 1.06612 0.7
1.0 1.55139 –0.52446 0.96054 1.08416
0.0 1.04607 –0.32232 1.75335 1.02897
0.5 1.15386 –0.42986 1.7723 1.04142 3.0
1.0 1.29099 –0.55603 1.79581 1.0569
0.0 0.90501 –0.33192 2.61364 1.01487
0.5 1.00123 –0.44337 2.63338 1.02627 7.0
1.0 1.12502 –0.5743 2.65829 1.04062
Pr

1/ 2
Re
fx x
C1/ 2 Re
fy x
C 1/2 Rex Nu  1/2 Rex Sh 
0.0 1.2545 –0.30646 0.93274 1.05144
0.5 1.27153 –0.28184 0.93426 1.05325 0.7
1.0 1.29145 –0.26046 0.93606 1.05538
0.0 1.04607 –0.32232 1.75335 1.02897
0.5 1.05068 –0.29681 1.75402 1.02933 3.0
1.0 1.05605 –0.27486 1.75481 1.02976
0.0 0.90501 –0.33192 2.61364 1.01487
0.5 0.90417 –0.30572 2.61336 1.01457 7.0
1.0 0.90359 –0.28324 2.61312 1.0143
the resistance due to the porous medium increases and
the velocity components decrease further as shown in
Figures 2 and 3. Also, the effects of

, and 

on
the temperature profiles



 and on the concentration
profiles



 are shown in Figures 4 and 5. From these
figures it is obvious that as the first and second resis-
tances increases the temperature and concentration pro-
files decrease.
In Figures 6 and 7 the effects of the first resistance and
the Prandtl number Pr on the temperature profiles




and the effects of the first resistance and the Schmidt
number Sc on the concentration profiles



 for 0.5s

,
1.0N

, 0.94Sc

are presented. Also, the effects of

,

and Pr on the skin friction coefficients, Nusselt
number and Sherwood number (1/2 Re
f
xx
C, 1/ 2 Re
f
yx
,
,
C
1/
Rex Nu 2 1/2 Re
x
Sh

) are given in Table 3. Figures 6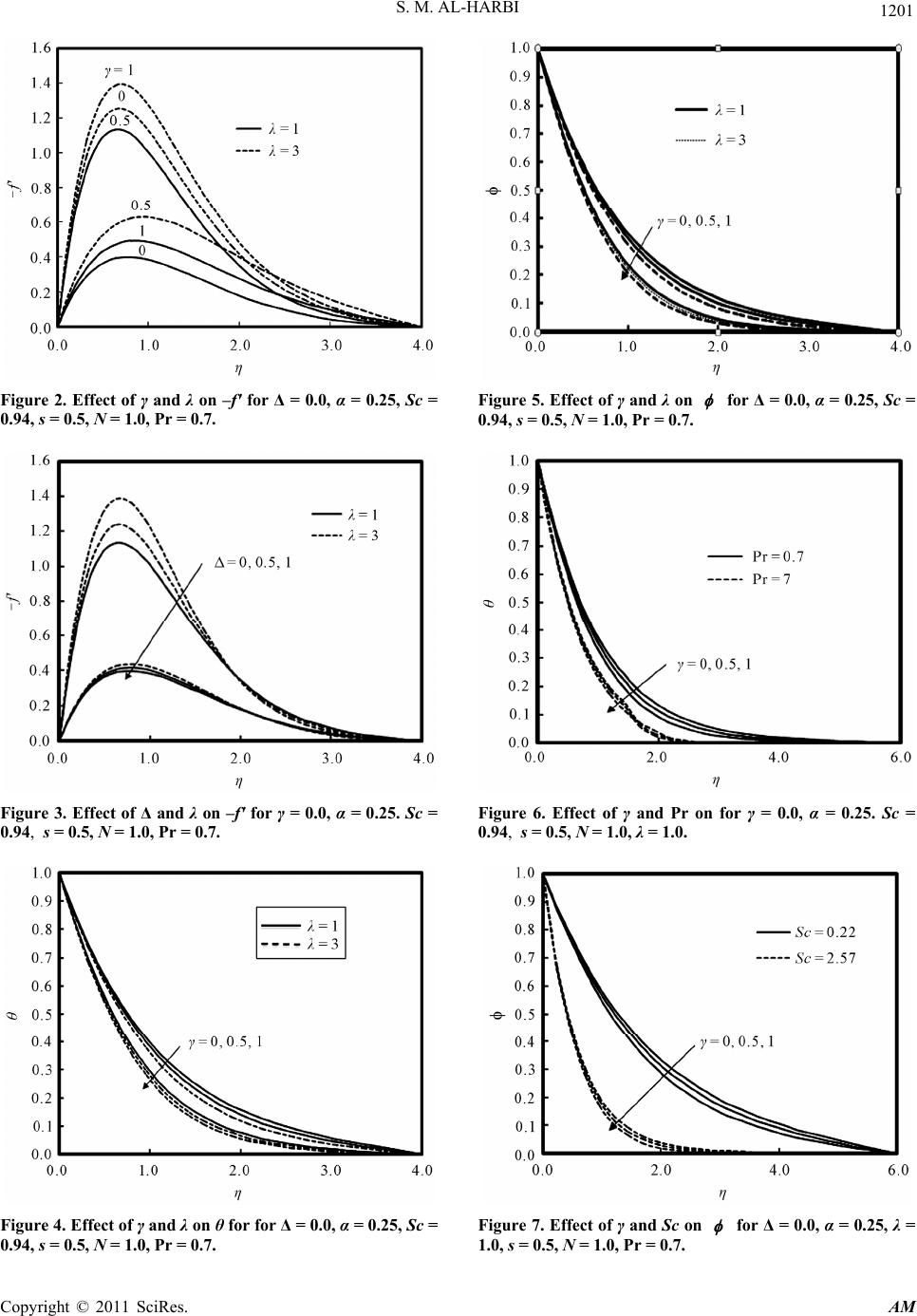

S. M. AL-HARBI
1201
Figure 2. Effect of γ and λ on –f' for Δ = 0.0, α = 0.25, Sc =
0.94, s = 0.5, N = 1.0, Pr = 0.7.
Figure 3. Effect of Δ and λ on –f' for γ = 0.0, α = 0.25. Sc =
0.94, s = 0.5, N = 1.0, Pr = 0.7.
Figure 4. Effect of γ and λ on θ for for Δ = 0.0, α = 0.25, Sc =
0.94, s = 0.5, N = 1.0, Pr = 0.7.
Figure 5. Effect of γ and λ on

for Δ = 0.0, α = 0.25, Sc =
0.94, s = 0.5, N = 1.0, Pr = 0.7.
Figure 6. Effect of γ and Pr on for γ = 0.0, α = 0.25. Sc =
0.94, s = 0.5, N = 1.0, λ = 1.0.
Figure 7. Effect of γ and Sc on

for Δ = 0.0, α = 0.25, λ =
1.0, s = 0.5, N = 1.0, Pr = 0.7.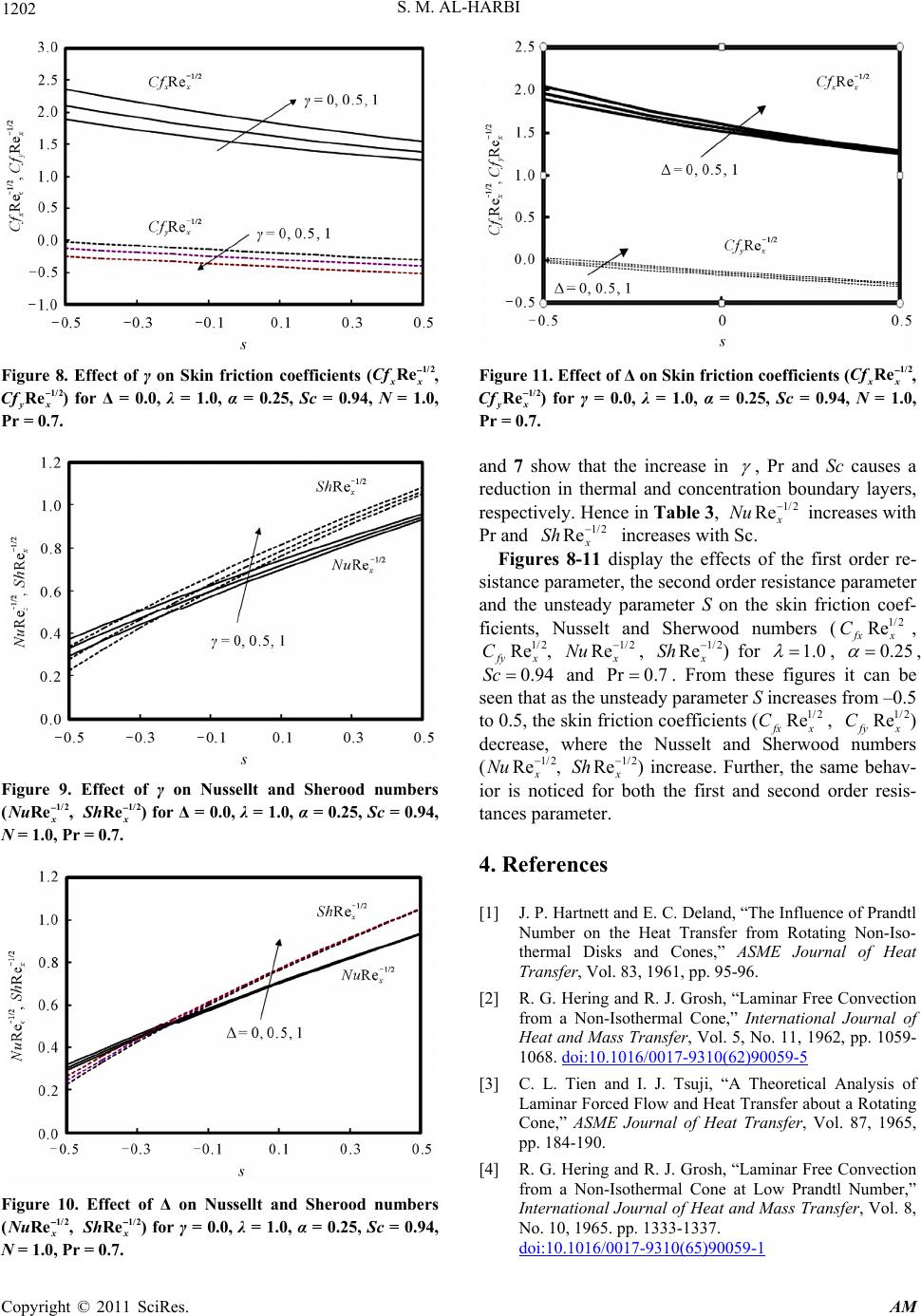

1202 S. M. AL-HARBI
Figure 8. Effect of γ on Skin friction coefficients (1/
2
Re
xx
Cf

,
1/
2
Re
yx
C
f
) for Δ = 0.0, λ = 1.0, α = 0.25, Sc = 0.94, N = 1.0,
Pr = 0.7.
Figure 9. Effect of γ on Nussellt and Sherood numbers
(, ) for Δ = 0.0, λ = 1.0, α = 0.25, Sc = 0.94,
N = 1.0, Pr = 0.7.
1/2
Rex Nu 1/2 Rex Sh 
Figure 10. Effect of Δ on Nussellt and Sherood numbers
(, ) for γ = 0.0, λ = 1.0, α = 0.25, Sc = 0.94,
N = 1.0, Pr = 0.7.
1/2
Rex Nu 1/2 Rex Sh 
Figure 11. Effect of Δ on Skin friction coefficients (1/
2
Re
xx
Cf

,
1/
2
Re
yx
C
f

) for γ = 0.0, λ = 1.0, α = 0.25, Sc = 0.94, N = 1.0,
Pr = 0.7.
and 7 show that the increase in

, Pr and Sc causes a
reduction in thermal and concentration boundary layers,
respectively. Hence in Table 3, 1/2 Re
x
Nu  increases with
Pr and increases with Sc.
1/2
Rex Sh 
Figures 8-11 display the effects of the first order re-
sistance parameter, the second order resistance parameter
and the unsteady parameter S on the skin friction coef-
ficients, Nusselt and Sherwood numbers (1/2
Re
f
xx
C,
1/ 2
Re
f
yx
C, ,
1/2
Rex Nu 1/2 Re
x
Sh ) for 1.0

, 0.25


,
0.94Sc

and Pr0.7

. From these figures it can be
seen that as the unsteady parameter S increases from –0.5
to 0.5, the skin friction coefficients (1/2 Re
f
xx
C, 1/2 Re
f
yx
C)
decrease, where the Nusselt and Sherwood numbers
(21/ Re
x
Nu

, 1/2 Re
x
Sh

) increase. Further, the same behav-
ior is noticed for both the first and second order resis-
tances parameter.
4. References
[1] J. P. Hartnett and E. C. Deland, “The Influence of Prandtl
Number on the Heat Transfer from Rotating Non-Iso-
thermal Disks and Cones,” ASME Journal of Heat
Transfer, Vol. 83, 1961, pp. 95-96.
[2] R. G. Hering and R. J. Grosh, “Laminar Free Convection
from a Non-Isothermal Cone,” International Journal of
Heat and Mass Transfer, Vol. 5, No. 11, 1962, pp. 1059-
1068. doi:10.1016/0017-9310(62)90059-5
[3] C. L. Tien and I. J. Tsuji, “A Theoretical Analysis of
Laminar Forced Flow and Heat Transfer about a Rotating
Cone,” ASME Journal of Heat Transfer, Vol. 87, 1965,
pp. 184-190.
[4] R. G. Hering and R. J. Grosh, “Laminar Free Convection
from a Non-Isothermal Cone at Low Prandtl Number,”
International Journal of Heat and Mass Transfer, Vol. 8,
No. 10, 1965. pp. 1333-1337.
doi:10.1016/0017-9310(65)90059-1

S. M. AL-HARBI
1203
[5] K. Himasekhar, P. K. Sarma and K. Janardhan, “Laminar
Mixed Convection from Vertical Rotating Cone,” Inter-
national Communications in Heat and Mass Transfer,
Vol. 16, No. 1, 1989, pp. 99-106.
doi:10.1016/0735-1933(89)90045-6
[6] C. Y. Wang, “Boundary Layers on Rotating Cones, Discs
and Axisymmetric Surfaces with a Concentrated Heat
Source,” Acta Mechanica, Vol. 81, No. 3-4, 1990, pp.
245-251. doi:10.1007/BF01176992
[7] K. A. Yih, “Mixed Convection about a Cone in a Porous
Medium: The Entire Regime,” International Communica-
tions in Heat and Mass Transfer, Vol. 26, No. 7, 1999, pp.
1041-1050. doi:10.1016/S0735-1933(99)00093-7
[8] S. M. Al-Harbi, “Numerical Study of Natural Convection
Heat Transfer with Variable Viscosity and Thermal Ra-
diation from a Cone and Wedge in Porous Media,” Ap-
plied Mathematics and Computation, Vol. 170, 2005, pp.
64-75. doi:10.1016/j.amc.2004.10.093
[9] H. S. Takhar, A. Chamkha and G. Nath, “Unsteady
Mixed Convention Flow from a Rotating Vertical Cone
with a Magnetic Field,” Heat and Mass Transfer, Vol. 39,
No. 4, 2003, pp. 297-304.
[10] D. Anilkumar and S. Roy, “Unsteady Mixed Convection
Flow on a Rotating Cone in a Rotating Fluid,” Applied
Mathematics and Computation, Vol. 155, No. 2, 2004, pp.
545-561. doi:10.1016/S0096-3003(03)00799-9
[11] I. Pop and D. B. Ingham, “Convective Heat Transfer:
Mathematical and Computational Modeling of Viscous
Fluids and Porous Media,” Pergamon, Oxford, 2001.
[12] D. A. Nield and A. Bejan, “Convection in Porous Me-
dia,” Springer-Verlag, New York, 2005.
[13] I. A. Hassanien, F. S. Ibrahim and Gh. M. Omer,
“Unsteady Free Convection Flow in the Stagnation-Point
Region of a Rotating Sphere Embedded in a Porous Me-
dium,” Mechanical Engineering, Vol. 7, 2004, pp. 89-98.
[14] I. A. Hassanien, F. S. Ibrahim and Gh. M. Omer,
“Unsteady Flow and Heat Transfer of a Viscous Fluid in
the Stagnation Region of a Three-Dimensional Body
Embedded in a Porous Medium,” Journal of Porous Me-
dia, Vol. 9. No. 4, 2006, pp. 357-372.
doi:10.1615/JPorMedia.v9.i4.60
[15] F. S. Ibrahim, A. M. Elaiw and A. A. Bakr, “Effect of
Chemical Reaction and Radiation Absorption on the Un-
steady MHD Free Convection Flow Past a Semi Infinite
Vertical Permeable Moving Plate with Heat Source and
Suction,” Communications in Nonlinear Science and
Numerical Simulation, Vol. 13, No. 6, 2008, pp. 1056-
1066. doi:10.1016/j.cnsns.2006.09.007
[16] S. Roy, P. Datta and N. C. Mahanti, “Non-Similar Solu-
tion of an Unsteady Mixed Convection Flow over a Ver-
tical Cone with Suction or Injection,” International Jour-
nal of Heat and Mass Transfer, Vol. 50, 2007, pp. 181-
187. doi:10.1016/j.ijheatmasstransfer.2006.06.024
[17] E. Osalusi, J. Side, R. Harris and P. Clark, “The Effect of
Combined Viscous Dissipation and Joule Heating on Un-
steady Mixed Convection MHD Flow on a Rotating Cone
in a Rotating Fluid with Variable Properties in the Pres-
ence of Hall and Ion-Slip Currents,” International Com-
munications in Heat and Mass Transfer, Vol. 35, No. 4,
2008, pp. 413-429.
doi:10.1016/j.icheatmasstransfer.2007.09.002
[18] K. Inouye and A. Tate, “Finite Difference Version Qua-
silinearization Applied to Boundary Layer Equations,”
AIAA Journal, Vol. 12, No. 4, 1974, pp. 558-560.
doi:10.2514/3.49286
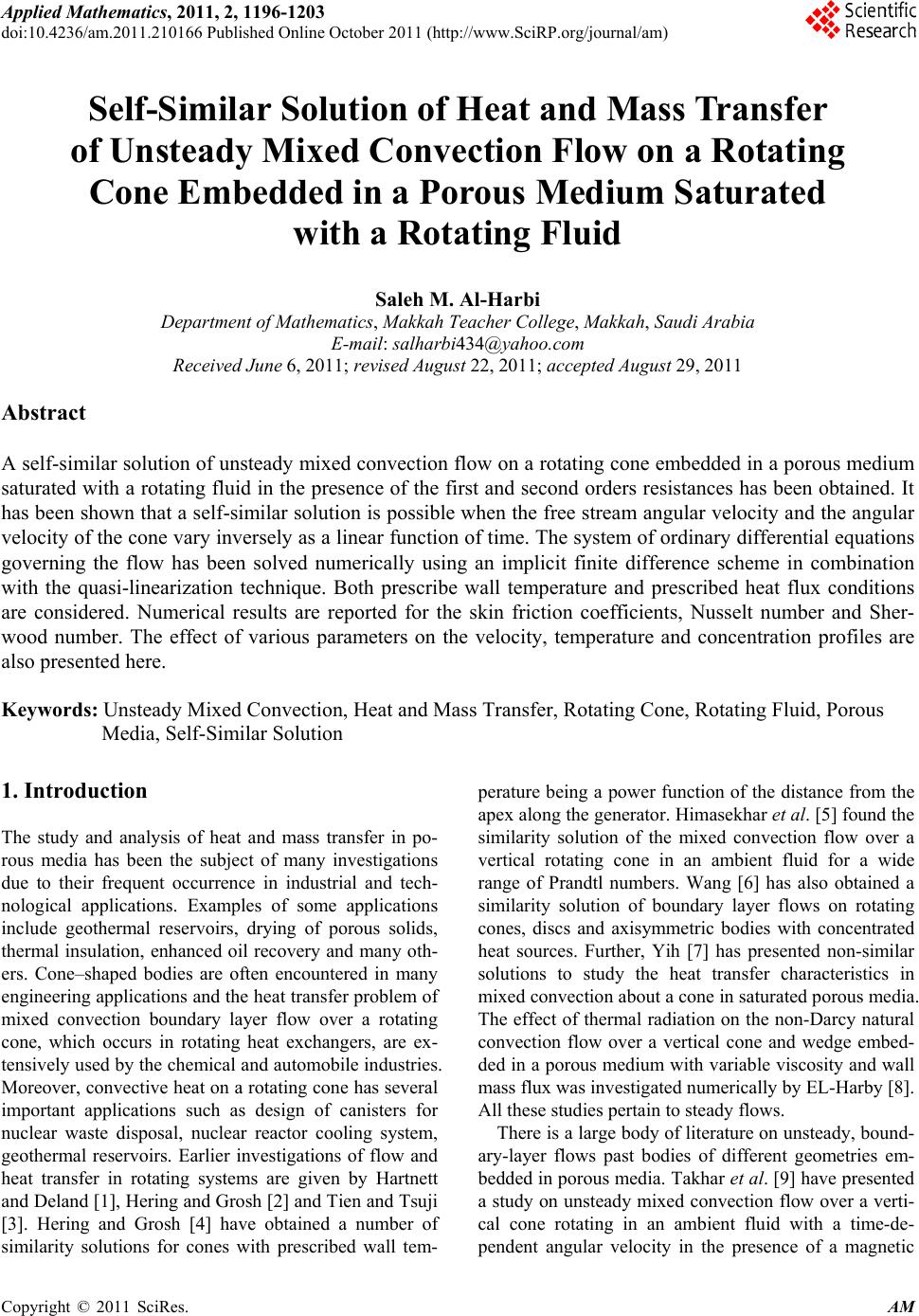