ABSTRACT

In this paper, multidimensional weakly singular integrals are solved by using rectangular quadrature rules which base on quadrature rules of one dimensional weakly singular and multidimensional regular integrals with their Euler-Maclaurin asymptotic expansions of the errors. The presented method is suit for solving multidimensional and singular integrals by comparing with Gauss quadrature rule. The error asymptotic expansions show that the convergence order of the initial quadrature rules is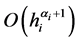, where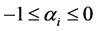. The order of accuracy can reach to by using extrapolation and splitting extrapolation, where h₀ is the maximum mesh width. Some numerical examples are constructed to show the efficiency of the method.

Keywords:

Multidimensional Weakly Singular Integrals, Euler-Maclaurin Errors Asymptotic Expansions, Splitting Extrapolation

1. Introduction

It is well known that multidimensional singular integrals are models arising in diverse engineering problems and mathematical applications. For example, in the boundary element fracture analysis problem, elasticity problem [1], bimaterial interfacial cracks [2] and wedge-sharped bimaterial interface [3], etc. Few of these integrals and equations can be solved explicitly, it is necessary to find a good numerical method. At present, there are many numerical techniques to calculate one-dimensional singular integrals or integral equations, such as collocation method [4], Gaussian quadrature method [5] [6], mechanical quadrature method [7] [8]. The Gauss-quadrature rules are considered to be a good choice for solving high dimensional integrals because they were accurate for polynomial approximation and the cost is low. However, Gaussian formula is not suitable for dealing with more than five-dimensional problems. So, we give a new algorithm for solving the following integral

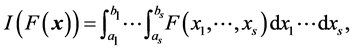(1)

where.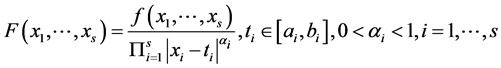

The structure of this paper is as follows: In Section 2, we give quadrature rules for weakly singular integral with multivariate errors asymptotic expansions. In Section 3, we construct the splitting extrapolation algorithm. In Section 4, some examples are given to illustrate the validity of the proposed method. Section 5 concludes the paper with a brief summary.

2. Multi-Parameters Asymptotic Expansions of the Errors for Weakly Singular Integrals

In this part, we mainly consider multidimensional weakly singular integrals. We give the corresponding results of multidimensional weakly singular integrals according to the quadrature formula and asymptotic expansions of the errors of one-dimensional integrals.

Theorem 1. If 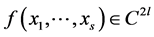on the interval, and

we assume that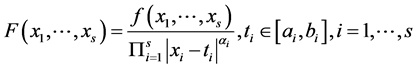, , 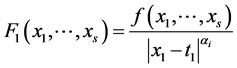, ,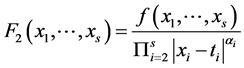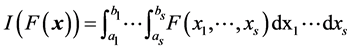. Then we have the following asymptotic expansions of the errors

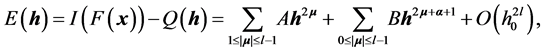(2)

where, , , , .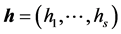

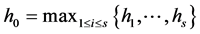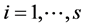Proof: We prove the theorem by the mathematic induction method. First, the conclusion is obvious right for. Now, we assume that the result also holds when. Next, we just need to prove the case of

(3)

where, , are functions which are independent of. The integral can be written as

(4)

we consider

(5)

then can be represented as

(6)

We need to consider the following formula

(7)

we know the above equation is obviously right by induction. Next, we calculate

(8)

The same as we can easy obtain

(9)

(10)

Now, we consider

(11)

(12)

(13)

Now, we obtain the following equation by taking the and into Equation (4)

(14)

where are constant which are independent of. The proof has been completed.

3. Splitting Extrapolation Algorithm

Now, we introduce the splitting extrapolation algorithm

(15)

where, .

First, we have to eliminate the minimum term of the errors expansions. According to (2), we can easily find that, are low order terms when. Assuming that, and we use splitting extra- polation in the direction of

(16)

Then, we use and obtain the following equation

17)

where, and are

constants which are unrelated to. We can obtain higher accuracy and convergence order by repeating the above process.

4. Examples

In this section, we give some examples to illustrate the efficiency of the proposed method.

Example 1. We consider the following -dimensional integral [9]

(18)

We give the numerical results of the splitting extrapolation of types 1 and 2 and Gauss quadrature methods. Table 1 gives the relative error (RE) and CPU time for different dimension () and splitting times (). From the Table 1, we can find that the splitting extrapolation method is suit for solving high dimensional integrals, and Gauss quadrature rule is difficult for solving more than five dimensional problems.

Example 2. we consider the following integral

(19)

This is a high dimensional weakly singular integral which can be solved by splitting extrapolation algorithm. In Table 2, we give the absolute errors and convergence orders for splitting extrapolation of each step. From the table, we can find that the convergence order can reach to by using splitting extrapolation twice, and the orders are coincide with the theoretical analysis. In Figure 1, we give the curves of absolute errors for each splitting extrapolation. From the Vertical direction, the images sink and the slopes of the curves increase with the increasing of the splitting times, which indicates that the errors decrease and the convergence orders increase. From the horizontal coordinate, the errors are reduced with the increasing of the node numbers. This shows that the splitting extrapolation not only enhance the numerical precision but also the order of accuracy.

5. Conclusion

In this paper, we give the quadrature formula with the asymptotic expansions of errors for solving multidimensional integrals with arbitrary points weakly singular. According to the asymptotic expansions of errors, we construct splitting extrapolation algorithm to improve the accuracy and the convergence order of the numerical results. By comparing the numerical results of our method with Gauss quadrature method, we can conclude that the splitting extrapolation method is efficient for solving high dimensional integral and weakly singular integrals. Next, we consider how to use the method to deal with boundary integral and differential equations.

Acknowledgements

The authors are very grateful to the referees and editors. This work was partially supported by the financial support from National Natural Science Foundation of China (Grant no. 11371079).

Cite this paper

Pan, Y.B., Huang, J. and Liu, H.Y. (2017) Euler-Maclaurin Expansions of Errors for Multidimensional Weakly Singular Integrals and Their Splitting Extrapolation Algorithm. Journal of Applied Mathematics and Physics, 5, 252- 258. https://doi.org/10.4236/jamp.2017.52022

References

NOTES

*This work was supported by the National Natural Science Foundation of China (11371079).