Open Access Library Journal
Vol.04 No.01(2017), Article ID:73624,21 pages
10.4236/oalib.1103342
On the Conformal Unity between Quantum Particles and General Relativity
Risto Raitio
02230 Espoo, Finland

Copyright © 2017 by author and Open Access Library Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: December 29, 2016; Accepted: January 16, 2017; Published: January 19, 2017
ABSTRACT
I consider the standard model, together with a preon version of it, to search for unifying principles between quantum particles and general relativity. Argument is given for unified field theory being based on gravitational and electromagnetic interactions alone. Conformal symmetry is introduced in the action of gravity with the Weyl tensor. Electromagnetism is geometrized to conform with gravity. Conformal symmetry is seen to improve quantization in loop quantum gravity. The Einstein-Cartan theory with torsion is analyzed suggesting structure in spacetime below the Cartan scale. A toy model for black hole constituents is proposed. Higgs metastability hints at cyclic conformal cosmology.
Subject Areas:
Particle Physics
Keywords:
Preons, Standard Model, Conformal Symmetry, Torsion, Loop Quantum Gravity, Cosmology, Dark Energy, Dark Matter

1. Introduction
The purpose of this article is to search for unifying principles for quantum models of matter and spacetime on all possible length scales: from the tiniest distances of high energy accelerators and colliders up to galaxies and towards the radius of the universe. Even the Planck scale Gedanken experiments are con- sidered. Cosmological developments are included with a lesser emphasis― though not less important. This task is motivated by a large number of theore- tical results on the various sectors of the subject, not necessarily on the most fashionable areas of main stream research, but they are unfortunately scattered around widely in the literature. The author feels therefore that trying to collect some of the pieces of the puzzle together is well justified. Evidence for mathe- matical unity of matter and spacetime structure is indeed found, even though this work is bound to require much more effort in the future. Some of material of this note is of this author but mostly what follows is a mini review based on a personal, and partly random, selection of papers.
In the last fifty years, or more, symmetry has been the leading principle in classifying particles and their interactions. Specifically, the gauge symmetry is the basis of particle phenomenology and theory. All known particles belong to a presentation of some group, be it e.g. the Lorentz, Poincaré or an SU(N) group. The standard model (SM) of particles with its some twenty parameters describes all measured accelerator data available today. There are known limitations and problems with the standard model. Bigger problems occur when one considers astrophysical and cosmological measurements, like dark energy and dark matter. Gravity has its own known problems with quantum theory in general. String theory was a promising candidate for unifying the standard model with gravity. Unfortunately, not much progress has taken place in about fortyfive years, apart from experimentally discovering the top quark, the Higgs boson and the acce- lerating expansion of the universe. Have we considered in depth all possible symmetries now? Perhaps not.
One specific symmetry has long been known in special circumstances: scale, or conformal, invariance in deep inelastic scattering, magnetism and the primor- dial cosmic microwave background (CMB) fluctuations.1 Here I use the term conformal symmetry. The standard model is conformal symmetric if one leaves out the Higgs sector. The standard model is stable towards Planck scale but the Higgs sector is metastable. This metastability is a problem to inflationary cosmo- logy but it fits well to conformal cyclic cosmology where the decay of the current vacuum is a prediction ending the current cycle and beginning the next one [1] [2] . The SM, including the Higgs mechanism, has been formulated as a con- formal theory together with gravity [3] . Conformal symmetry changes cosmo- logy allowing to solve classical Friedmann-Robertson-Walker (FRW) equations through big crunch-big bang transitions. Consequently, a satisfactory standard model has emerged for both small scale and large scale phenomena covering distances between  cm and
cm and 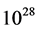 cm. Even dark matter and dark energy seem to find their place in this scenario.
cm. Even dark matter and dark energy seem to find their place in this scenario.
Together with several other people, I have gone quite some time ago one step further down to preon level, for a review of early work see e.g. [4] . The term preon means here any new kind of hypothetical point particle or geometrical structure in spacetime near the Planck length scale. There is no direct experi- mental evidence for preons, they are rather objects of Gedanken experiments. Recently, I have reanalyzed a phenomenological preon model for quarks and leptons [5] trying to find an theoretical reference frame for it [6] [7] [8] . Unifica- tion was discussed on two levels: unification of gravitational and electromagnetic interactions only, instead of the traditional grand unification (GUT), and secondly, unification of both these interactions and spacetime, i.e. unification of everything.
Unification of physics based on particle internal symmetry has been successful until recently when geometry has taken a significant role in the form of the local conformal symmetry. Gravity itself is not limited to Einstein gravity (EG). Several extra terms in the gravitational action have been studied with more and less success. In fact, a good old starting point is the gravity based on the Weyl tensor [9] , which provides a unique form of the gravitational action as the square of the Weyl tensor. An almost equally old idea is, due to Einstein and Rosen, that particles themselves would be structures in pure vacuum geometry [10] . Intri- guingly, Cartan introduced spinors long before spin was discovered for particles [11] .
Quantization of geometry has been pursued for a few decades within a theory called loop quantum gravity (LQG) [12] . There again introducing conformal symmetry certain details of quantization have become clearer [3] . Most consi- derations in this note apply to the SM as well to the preon model. In the latter I wish to keep the Higgs scalar sector clean as long as possible to endorse the massless particles for conformal cosmology. On the other hand, fermion mass is considered in a generalized gravity theory, the Einstein-Cartan theory, which includes torsion [13] and brings interesting new light to fermion behavior in curved spacetime at high energy density. I give arguments for the existence of structure of spacetime at Cartan length scale, where quantum gravity is expected to begin to appear. A toy model for black hole constituents is proposed in Section 8.
This note is organized as follows. In Section 2 I briefly recall the preon model, which is discussed partly for historical reasons. In Subsection 3.1 conformal gravity and in Subsection 3.2 loop quantum gravity are summarized. In Section 4 electromagnetism is geometrized. The conformal standard model is discussed in Section 5. Section 6 is on the outer edge of this study. The Dirac field in the presence of torsion is introduced in Subsection 6.1. The massive Dirac field is discussed in Subsection 6.2. Cosmology is reviewed in Section 7. Conformal symmetry and black holes are treated in Section 8. Finally, conclusions are given in Section 9.
The presentation of the material is concise and goal oriented rather than comprehensive but an attempt is made for it to be reasonably self contained. The author feels he had to go through all the material presented in this note but the reader may find it better to start with the first and last section on first reading and save Sections 2 and 6 for later.
2. The Preon Model
2.1. Conformal Preons
The virtue of conformal symmetry is that the action for conformal gravity is defined uniquely by the Weyl tensor, described in Subsection 3.1. All particles in conformal theory are massless. Other properties of conformal theory include renormalizability, unitarity, and the theory is ghost-free. It has been shown to explain dark matter and energy [14] [15] [16] [17] [18] . Therefore in this note I assume zero mass preons on action level.
Requiring charge quantization 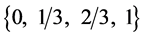 and preon permutation antisymmetry for identical preons, one can define three preon bound states which form the first generation quarks and leptons [6] [7]
and preon permutation antisymmetry for identical preons, one can define three preon bound states which form the first generation quarks and leptons [6] [7]
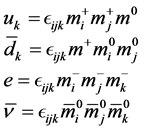 (2.1)
(2.1)
A binding interaction between preons is needed to make the quark and lepton bound states possible. I have at the moment no detailed form for this interaction. Its details are not expected to be of primary importance at this preliminary stage. I suppose this attractive, non-confining interaction is strong enough to keep together the charged preons but weak enough to liberate the preons at high temperature. Some more thoughts are indicated in Subsection 2.2.
A useful feature in (2.1) with two identical preons2 is that the construction provides a three-valued subindex for quark SU(3) color, as it was originally discovered [19] . In addition, the weak SU(2) left handed doublets can be read from the first two and last two lines in (2.1). The SM gauge structure can be deduced in this sense from the present preon model. One could also have preon charges 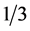 and
and 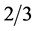 but then the index k distinction between quarks and leptons in (2.1) would be lost.
but then the index k distinction between quarks and leptons in (2.1) would be lost.
One may now propose that, as far as there is an ultimate unified theory, it is a preon theory with only gravitational and electromagnetic interactions operating between preons. The strong and weak forces are generated in the early universe later when massless preons combine into quarks and leptons at lower tempera- ture and they operate only with short range interaction within nuclei making atoms, molecules and chemistry possible. In a contracting phase of the universe processes take place in the opposite order.
The unification picture is supposed to hold in the present scheme up to the energy of about 1016 GeV. The electroweak interaction has the spontaneously broken symmetry phase below an energy of the order of 100 GeV and symmetric phase above it. The electromagnetic and weak forces take separate ways at higher energies ( ), the latter melts away due to ionization of quarks and leptons into preons, but the former stays strong towards Planck scale,
), the latter melts away due to ionization of quarks and leptons into preons, but the former stays strong towards Planck scale,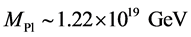 . Likewise the quark color interaction suffers the same destiny as the weak force. One is left with the electromagnetic and gravitational forces only at Planck scale.
. Likewise the quark color interaction suffers the same destiny as the weak force. One is left with the electromagnetic and gravitational forces only at Planck scale.
The proton, neutron, electron and 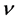 can be constructed of 12 preons and 12 anti-preons. The construction (2.1) is matter-antimatter symmetric on preon level, which is desirable for early cosmology. The model makes it possible to create from vacuum a universe with only matter: combine e.g. six
can be constructed of 12 preons and 12 anti-preons. The construction (2.1) is matter-antimatter symmetric on preon level, which is desirable for early cosmology. The model makes it possible to create from vacuum a universe with only matter: combine e.g. six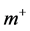 , six
, six 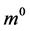 and their antiparticles to make the basic
and their antiparticles to make the basic 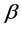 -decay particles. Corresponding antiparticles may occur equally well.
-decay particles. Corresponding antiparticles may occur equally well.
The baryon number (B) is not conserved [20] [21] in this model: a proton may decay at Planck scale temperature by a preon rearrangement process into a positron and a pion. This is expected to be independent of the details of the preon interaction. Baryon number minus lepton number (B-L) is conserved.
Unification of gravity and electromagnetism is discussed in Section 4.
2.2. Geometrical Preons
The preon model described in [6] is based on a statistical black hole model in loop quantum gravity (LQG). In LQG the geometry is quantized such that the lowest area eigenvalue is zero, which allows zero mass particles in the model construction as the Brown-York energy is proportional to area. The idea that a particle can be defined in pure gravity theory was first put forward in [10] . There it was also found that a charged black hole with Reissner-Nordström metric can have zero mass.
The preons have to be kept inside the quarks and leptons using some elegant, preferably non-confining mechanism. I also want to exclude scalar, vector and spinorial self-interactions. Same sign charges should be kept inside the bound states. Therefore a possibility could be that the zero mass black hole preons would form together one single non-spherical horizon around the quark or lepton.
Unification of black hole particles (i.e. preons) and spacetime is discussed in Subsection 3.2.
3. Gravity
3.1. Conformal Gravity
Weyl introduced, while working on the geometrization of electromagnetism, a new tensor called the Weyl or conformal tensor [9] (for an introduction to conformal theories, see e.g. [22] )
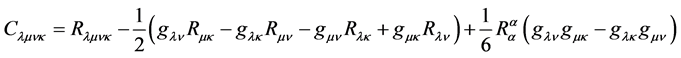
(3.1)
where 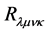 is the Riemann tensor. Under local conformal transformation
is the Riemann tensor. Under local conformal transformation  of the metric tensor
of the metric tensor 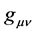 of the form
of the form
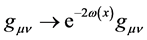 (3.2)
(3.2)
the Weyl tensor transforms simply as
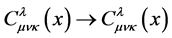 (3.3)
(3.3)
The pure local conformal gravity is based on the following action

where 

Conformal gravity is power-counting renormalizable and unitary [16] . In 

invariant. The cosmological constant will appear later when conformal symme- try is dynamically broken and gives the dimensionful 
The reason for local conformal invariance is that massless particles move on the light cone which is invariant under the 15 parameter conformal group SO(4,2) [16] . The covering group of SO(4,2) is SU(2,2). This is generated by the 15 Dirac matrices (
Functional variation of 






where

By defining the left hand side of (3.6) as the energy-momentum tensor 

We see that gravity and matter sectors are on equal footing and the total energy-momentum tensor of the universe is zero.
The connection between Einstein gravity and conformal gravity is that the solutions of the former are solutions of the latter [24] [25] . However, conformal gravity has more general solutions to be explored. From these the Einstein solutions can be chosen by imposing a certain Neumann boundary condition on the metric at the future boundary. The boundary condition eliminates ghosts and the theory becomes an Einstein theory with a cosmological constant.
As a final piece of support to conformal invariance it can be mentioned that the high energy limit of all non-trivial renormalizable field theories is comfor- mally invariant [26] .
3.2. Loop Quantum Gravity
A statistical physics model for quantum black holes has been presented in [7] . It is based on Brown-York energy E for an area 



where the sum is over punctures 




Among the problems in LQG is finding quantization without quantum anomalies. A second difficulty is making contact with the semiclassical physical picture of gravity. The existence of Planck scale sets restrictions in going to the continuum limit. If one adds points to the spin network to refine it, the conti- nuum approximation of volumes and areas does not get better, one just adds volume to the spacetime as the area eigenvalue has a minimum value. In a con- formal theory there is no length scale available and it is possible to improve the situation. Thirdly, though the theory is discrete and therefore finite, a finite renormalization is needed to separate the lower energy physics from the Planck scale features [3] .
Conformal invariance helps in all the above difficulties. In a conformal theory spin networks can be defined which can be indefinitely refined to arbitrary precision. In the renormalization problem no counter terms are needed in spacing dependent renormalization.
The geometric operators of area of a surface and the volume of a region can be generalized to their conformal invariant counterparts which are the same as before but now without factors of Planck length to the relevant power. Thus a conformal geometry of a spin network can be defined [3] .
4. Geometrization of Electromagnetism
Conformal invariance is also important for understanding the geometrization of other interactions. Metrication of electromagnetism coupled to a Dirac field 
The generalized Dirac action is

where the 




which is obtained from the generalized connection

where 



where 

Secondly, the Weyl connection drops out from the generalized Dirac action and therefore does not provide geometrization of electromagnetism. Replacing 

and 


where 

The action 

One sees that 
5. Conformal Standard Model
It is possible to couple massless particles to conformal gravity. One can also couple the massless standard model, or even the complete standard model in a way in which the Higgs boson acquires mass in the gauge fixed conformal theory [3] [31] . When the gauge is fixed the Planck scale is determined and so further the Higgs mass and the masses of all other SM particles, determined by the dimensionless constants of the theory.
The SM total Lagrangian can be written as a sum of the gravitational and matter terms

where





(5.2)
where the 


Choosing the gauge 

where 

This is a brief summary of a conformal invariant theory of gravity coupled to the SM that can be quantized by the LQG techniques [3] .
6. Torsion
6.1. Dirac Field in the Presence of Torsion
In this section I want to emphasize another kind of treatment of conformal gravity and the Dirac field, which can be a quark, lepton, or preon. In GR, one has to discuss torsion arising from rotations and translations of the Poincaré group, just as energy gives rise to curvature [33] [34] . Experimentally there is no evidence for torsion. It will be shown below that constraints coming from the antisymmetry of the spin do not yield complete antisymmetry of torsion but cause constraints to the metric. In particular, the spinorial self-interactions are absent. If, in addition, no scalar interactions are allowed for spinors their zero masses are well protected. In the case of preons, this sector of the model joins smoothly to the conformal SM when the energy or temperature becomes so low as to allow quark and lepton bound states.
The Riemann-Cartan geometry with metric and torsion is defined in terms of the metric tensor 


The torsion tensor is antisymmetric in its lower indices. A symmetric connec- tion is known as torsion-free.
The most general conformal transformation for the metric and torsion are with

where 


The Dirac field conformal transformation is

Let us introduce the modified metric-torsional curvature tensor with the Riemann curvature tensor

whose irreducible part is

and it is conformally covariant. The commutator of covariant derivatives obeys the equation

The conformal transformation for torsion is not uniquely defined [35] . The most general invariant obtainable from 

with the parameters 



(6.9) is antisymmetric in the first and second pair of indices, irreducible and conformally covariant. This reduces to the form 

with k the gravitational constant. By variation one gets


where 

The Dirac action is

where


and the massless matter field equations are

To see the effects of the complete antisymmetry of the spin on the structure of the field equations one should rewrite the field equations as follows


with the massless matter field equations (6.16).
In Weyl gravity there is no more a completely antisymmetric torsion and there are additional constrictions on the curvature tensor. This happens because both field equations for the spin and energy couple to both torsion and curva- ture so that the complete antisymmetry of the spin is partly imposed on torsion and partly on the curvature. We may decompose torsional terms away from the torsionless ones in all curvatures and derivatives. Thereafter all curvatures and derivatives are written in terms of purely metric curvature and derivatives given by the Weyl conformal tensor 



where 


In Weyl-type of gravity there is no possibility to substitute torsion with the spin of the spinors and there are no longer non-linear self-interactions in the spinor field equations. The Dirac equation is linear even in the presence of torsion.
The conclusion from all the above analysis of torsion in GR is that the non- linear self-interactions of Dirac matter fields are absent.
6.2. Massive Dirac Field in the Presence of Torsion
A Dirac field with mass is a non-conformal theory, but it is a very interesting case and is discussed briefly. The Einstein-Cartan (EC) [11] [36] , or Einstein- Cartan-Kibble-Sciama (ECKS) [37] [38] [39] theory of gravity is a natural extension of GR to include matter with spin as is necessary to take into account the local gauge invariance with respect to the full Poincaré group. This produces torsion. The effect of torsion occur only at very high density of matter, much larger than the density of nuclear matter. Torsion modifies Dirac-Kerr-Newman ring singularity by a non-singular toroidal structure with the outer radius of the Compton wave length size and inner radius of the Cartan size (see (6.31) below). The Cartan size may introduce an effective UV cutoff for fermionic quantum field theory.
The dynamical variables in Einstein-Cartan theory are the vierbein 

where 



The variation with respect to spin connection 

The ECKS Lagrangian density is

where




where 

where 


where () denotes symmetrization, is quadratic in
The Cartan equation (6.25) is a linear relation and torsion is proportional to spin density. Therefore the torsion is zero outside material bodies. This makes detection of torsion difficult. The torsion field of ECKS theory does not propagate, unlike curvature.
The relativistic Dirac Lagrangian density in curved spacetime is

where the 






This spin density (6.29) does not depend on 


If one assumes the simplest possible fermion system, namely a point particle or a system of point particles, it turns out that there exist no solutions for the spinor field, i.e.






For an electron, 





These results imply that the Dirac wave function of an electron forms a non- singular form of spacetime structure of a toroid which has the outer radius of the electron Compton wave length and the inner radius of its Cartan radius. This is valid both for charged and uncharged leptons. The weak interactions do not change the situation in any significant amount. The toroid structure works also for quarks for which asymptotic freedom holds at distances



Finally, for the cosmological constant it is derived in [40] , using the type of four-fermion interaction (6.30)

This



7. Cyclic Conformal Cosmology
The running standard model quartic Higgs coupling 


But for the metastable Higgs there is a better solution, cyclic cosmology [2] . According to the cyclic picture, the vacuum is required to be metastable in order for the current phase of accelerated expansion to end and for a big crunch/big bang transition to occur enabling a new cycle to begin. It is important that scalar fields exist that tunnel from the current vacuum of positive potential energy density to a phase with negative potential energy density which decreases deeper with increasing field magnitude. For the cyclic model, this behavior not only is part of the future but also part of our distant past leading to the most recent bounce, the big bang.
In [2] Bars, Steinhardt and Turok construct a theoretical model that supports all known accelerator and satellite physics and describes the evolution of the Higgs through the big bounce. A major question is whether there exist solutions that will return the Higgs to the metastable vacuum after each big crunch/big bang transition.
The guiding principle of the model is conformal symmetry. The Weyl invariant action 

The term 













The action (7.1) is invariant under Weyl transformations by a local function 

where 


In the gauge 


The action (7.1) defines a conformally invariant homogenous and isotropic Friedman-Robertson-Walker (FRW) universe [42]

where 








Cosmological variables and gauges are briefly discussed, in 








where 



The authors are now able to conclude having found a band of continuous solutions that undergo acceptable repeated cycles of expansion and contraction as illustrated in detail in [2] .
8. Conformal Symmetry and Black Holes
The conformal action is (3.4). The general static, spherically symmetric solutions of (3.8) is [43]

where the function 

where 


which gives

where 

For certain values of the parameters (8.4) is a black hole line element. The metric on the spacelike surfaces of constant 


This metric describes a non-compact hyperbolic two-space 








There are several possibilities to find black holes with non-trivial topologies. With 







which is singular at






This spacetime is similar to the Schwarzschild-de Sitter solution but with non- trivial topology. As 





In the limit 

with

Depending on the parameter values (8.10) may represent a black hole. The angular sector has a flat metric 

on gets a compact orientable surface, a torus, with a topology




This is for 



For different genus values one has:

I propose a toy model for generic black hole structure, or constituents, in which the hole consist of tori of decreasing sizes starting from the radius of the hole. The next torus radius is the previous torus tube radius increasing the complexity of the hole topology. The scale dependence of this spacetime structure should be studied by this scale method.
9. Conclusions
The elegance and power of general relativity are realized when the basic Einstein equations are generalized to the largest local symmetry groups including the Weyl conformal symmetry and the full Poincaré symmetry with torsion of spacetime. The statement “to modify it [EG] without destroying the whole structure seems to be impossible” did not turn out to be true, if the modification is done properly. The main conclusion of this study is that local conformal symmetry allows us to obtain a unified description of gravity and the standard model. All interactions are described in geometrical or geometrized formalism which contains the familiar SM quantum particles. A possible model for matter- spacetime unification was reviewed in Subsection 3.2. I proposed in Section 8 a toy model for black hole structure, or constituents, in which the holes consist of tori of decreasing sizes starting from the radius of the hole.
With conformal symmetry the applicability of GR is greatly expanded. The cosmological picture of the universe is changed substantially as became clear several decades later [2] [44] [45] . The “one start” big bang is replaced by the cyclic picture of the universe. Conformal symmetry makes it possible to geome- trize all other interactions, of which electromagnetism received in Section 4 special attention. Further it has been shown that dark matter of galaxies can be explained with the different velocity profile of conformal equations [17] . A theory for dark energy has also been proposed [18] . At high energy/matter den- sity, like inside black holes, the pure massless conformal symmetry must be mo- dified to include mass of Dirac fields. Torus solutions in theories with massive Dirac field in the presence of torsion are interesting. Most likely, torsion is a neglected important sector of gravity. Interesting structures in spacetime with certain area and volume quantization methods should be restudied. The area and volume of a torus are 




Acknowledgements
I thank Dr. William Straub for correspondence and comments on the manu- script.
Cite this paper
Raitio, R. (2017) On the Conformal Unity between Quan- tum Particles and General Relativity. Open Access Library Journal, 4: e3342. http://dx.doi.org/10.4236/oalib.1103342
References
- 1. Bars, I., Steinhardt, P. and Turok, N. (2013) Local Conformal Symmetry in Physics and Cosmology. Physical Review D, 89, Article ID: 043515. arXiv:1307.1848.
- 2. Bars, I., Steinhardt, P. and Turok, N. (2013) Cyclic Cosmology, Conformal Symmetry and the Metastability of the Higgs. Physics Letters B, 726, 50-55. arXiv: 1307.8106. https://doi.org/10.1016/j.physletb.2013.08.071
- 3. Campiglia, M., Gambini, R. and Pullin, J. (2016) Conformal Loop Quantum Gravity Coupled to the Standard Model. arXiv:1609.04028.
- 4. Harari, H. (1984) Composite Models for Quarks and Leptons. Physics Reports, 104, 159-179. https://doi.org/10.1016/0370-1573(84)90207-2
- 5. Raitio, R. (1980) A Model of Lepton and Quark Structure. Physica Scripta, 22, 197-198. https://doi.org/10.1088/0031-8949/22/3/002
- 6. Raitio, R. (2016) Combinatorial Preon Model for Matter and Unification. Open Access Library Journal, 3, e3032. https://doi.org/10.4236/oalib.1103032
- 7. Raitio, R. (2016) Standard Model Matter Emerging from Spacetime Preons. Open Access Library Journal, 3, e2788. https://doi.org/10.4236/oalib.1102788
- 8. Raitio, R. (2016) A Conformal Preon Model. Open Access Library Journal, 3, e3262. https://doi.org/10.4236/oalib.1103262
- 9. Weyl, H. (1918) Sitzsungber. Preussischen Akademie der Wissenschaften, 465-480.
- 10. Einstein, A. and Rosen, N. (1935) The Particle Problem in the General Theory of Relativity. Physical Review, 48, 73-77. https://doi.org/10.1103/PhysRev.48.73
- 11. Cartan, é. (1923) (1924) et (1925) Sur les variétés á connexion affine et la théorie de la relativité généralisée. Annales scientifiques de l’école Normale Supérieure, 42, 17-88.
- 12. Rovelli, C. (2011) Zakopane Lectures on Loop Gravity. arXiv:1102.3660.
- 13. Carroll, S. (2004) Spacetime and Geometry: An Introduction to General Relativity. Addison-Wesley, San Francisco.
- 14. Mannheim, P. (2007) Solution to the Ghost Problem in Fourth Order Derivative Theories. Foundations of Physics, 37, 532-571. arXiv:hep-th/0608154. https://doi.org/10.1007/s10701-007-9119-7
- 15. Bender, C. and Mannheim, P. (2008) Giving up the Ghost. Journal of Physics A, 41, Article ID: 304018. arXiv:0807.2607. https://doi.org/10.1088/1751-8113/41/30/304018
- 16. Mannheim, P. (2012) Making the Case for Conformal Gravity. Foundations of Physics, 42, 388-420. arXiv:1101.2186. https://doi.org/10.1007/s10701-011-9608-6
- 17. Mannheim, P. (2006) Alternatives to Dark Matter and Dark Energy. Progress in Particle and Nuclear Physics, 56, 340-445. astroph/0505266. https://doi.org/10.1016/j.ppnp.2005.08.001
- 18. Mannheim, P. (2016) Mass Generation, the Cosmological Constant Problem, Conformal Symmetry, and the Higgs Boson. arXiv:1610.08907.
- 19. Greenberg, O. (1964) The Color Charge Degree of Freedom in Particle Physics. arXiv:0805.0289.
- 20. Bekenstein, J. (1972) Non Existence of Baryon Number for Static Black Holes I and II. Physical Review D, 5, 1239-1246. https://doi.org/10.1103/PhysRevD.5.1239
- 21. Wheeler, J. (1971) Cortona Symposium on Weak Interactions. Accademia Nazionale dei Lincei, Rome.
- 22. Fabbri, L. (2008) Higher Order Theories of Gravitation, PhD Thesis. arXiv: 0806.2610.
- 23. Lanczos, C. (1938) A Remarkable Property of the Riemann-Christoffel Tensor in four Dimensions. Annals of Mathematics, 39, 842-850. https://doi.org/10.2307/1968467
- 24. Maldacena, J. (2011) Einstein Gravity from Conformal Gravity. arXiv:1105.5632.
- 25. Anastasiou, G. and Olea, R. (2016) From Conformal to Einstein Gravity. Physical Review D, 94, Article ID: 086008. arXiv:1608.07826. https://doi.org/10.1103/physrevd.94.086008
- 26. Coleman, S. (1988) Aspects of Symmetry: Selected Erice Lectures. Cambridge University Press, Cambridge.
- 27. Ariwahjoedi, S., Astuti, V., Kosasih, J., Rovelli, C. and Zen, F. (2016) Statistical Discrete Geometry. arXiv:1607.08629.
- 28. Barbero G., J.F. and Perez, A. (2015) Quantum Geometry and Black Holes. arXiv:1501.02963.
- 29. Mannheim, P. (2014) PT Symmetry, Conformal Symmetry, and the Metrication of Electromagnetism. arXiv:1407.1820.
- 30. Mannheim, P. (2016) Conformal Invariance and the Metrication of the Fundamental Forces. arXiv:1603.08405.
- 31. Fabbri, L. (2014) Conformal Standard Model. General Relativity and Gravitation, 44, 3127-3138. arXiv:1107.0466. https://doi.org/10.1007/s10714-012-1440-6
- 32. Brans, C. and Dicke, R. (1961) Mach’s Principle and a Relativistic Theory of Gravitation. Physical Review, 124, 925-935. https://doi.org/10.1103/PhysRev.124.925
- 33. Fabbri, L. (2014) Metric-Torsional Conformal Gravity. Physics Letters B, 707, 415-417. arXiv:1101.1761. https://doi.org/10.1016/j.physletb.2012.01.008
- 34. Fabbri, L. (2013) Conformal Gravity with Dirac Matter. Annales de la Fondation Louis de Broglie, 38, 155-165. arXiv:1101.2334.
- 35. Shapiro, I (2002) Physical Aspects of the Space-Time Torsion. Physics Reports, 357, 113-213. hep-th/0103093. https://doi.org/10.1016/s0370-1573(01)00030-8
- 36. Trautman, A. (2006) The Einstein-Cartan Theory. In: Francoise, J.-P., Naber, G.L. and Tsou, S.T., Eds., Encyclopedia of Mathematical Physics, Elsevier, Amsterdam, Vol. 2, 189-195. https://doi.org/10.1016/B0-12-512666-2/00014-6
- 37. Kibble, T. (1961) Lorentz Invariance and the Gravitational Field. Journal of Mathematical Physics, 2, 212-221. https://doi.org/10.1063/1.1703702
- 38. Sciama, D. (1962) On the Analogy between Charge and Spin in General Relativity. In: Casciaro, B., Ed., Recent Developments in General Relativity, Pergamon Press, Oxford, 415-439.
- 39. Sciama, D. (1964) The Physical Structure of General Relativit. Reviews of Modern Physics, 36, 463-469. https://doi.org/10.1103/RevModPhys.36.463
- 40. Poplawski, N. (2011) Cosmological Constant from Quarks and Torsion. Annalen der Physik, 523, 291-295. https://doi.org/10.1002/andp.201000162
- 41. Degrassi, G., Di Vita, S., Elias-Miro, J., Espinosa, J., Giudice, G., Isidori, G. and Strumia, A. (2012) Higgs Mass and Vacuum Stability in the Standard Model at NNLO. JHEP, 1208, 098. arXiv:1205.6497.
- 42. Bars, I., Chen, S.-H., Steinhardt, P. and Turok, N. (2012) Complete Set of Homogeneous Isotropic Analytic Solutions in Scalar-Tensor Cosmology with Radiation and Curvature. Physical Review D, 86, Article ID: 083542. arXiv:1207.1940. https://doi.org/10.1103/PhysRevD.86.083542
- 43. Klemm, D. (1998) Topological Black Holes in Weyl Conformal Gravity. Classical and Quantum Gravity, 15, 3195-3201. gr-qc/9808051. https://doi.org/10.1088/0264-9381/15/10/020
- 44. Penrose, R. (2016) Fashion, Faith, and Fantasy in the New Physics of the Universe. Princeton University Press, Princeton. https://doi.org/10.1515/9781400880287
- 45. Gielen, S. and Turok, N. (2016) Quantum Propagation across Cosmological Singularities. arXiv:1612.02792.
NOTES
1To airfoil designers the Joukowsky transformation has been the first example.
2An assumption appears here that the same charge preons inside quarks have the same spin z-com- ponents.
3This result is intuitively strange.












