ABSTRACT

The Cobb-Douglas function not only leads to a long-term relationship between the rate of output change and the interest rate, but also analyzes why they fluctuate in the short-term. This paper first divides the fluctuation cycle of the interest rate in the statistical data of the past 45 years by using the mathematical phase diagram method, and draws the phase diagram of the rate of output change on the interest rate according to the cycle equation of output. From this phase diagram, we explain the reason that the phase difference between the interest rate and the rate of output change in the fluctuation. Then, according to the optimal relation between L and K in the Cobb-Douglas function, we further derive the employment equation and its relation to the real interest rate and the rate of real output change, and verify the theoretical speculation with statistical data. Finally, it is concluded that the business cycle is a kind of endogenous production phenomenon.

Subject Areas:

Economics

Keywords:

Cobb-Douglas Function, Business Cycles, Phase Diagram, Unemployment Rate

1. Introduction

Neoclassic theories believe that the surplus of output and unemployment would be cleaned out during competition and equilibrium is the normality of the economic system, therefore the fluctuation of macroeconomic variables is generated by external factors. The “Real Business Cycle Model” (RBC) arisen during 1980s considers the total factor productivity or the random perturbations of technology determine fluctuation of other variables [1] [2] [3] . However, other economists [4] [5] gave this theory some questions: according to RBC, if the economic boom was generated by the technological progress, then the economic recession should blame on technological setbacks. Nevertheless, what are reasons of technological setbacks? Moreover, if we use other methods to measure the technological impact, it can be a factor that eliminates business cycles [6] [7] [8] [9] [10] .

Hayek said: “the incorporation of cyclical phenomena into the system of economic equilibrium theory, which they are on apparent contradiction.” [11] . Since the interaction of the total supply and total demand leads to convergence rather than divergence, the convergence would flatten the fluctuation ever if there were external impacts assumed by the RBC theory. Perhaps the biggest problem with macroeconomics is explaining business cycles.

2. The Business Cycle Equation and Divisions

From the Cobb-Douglas function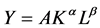, we deduce the marginal revenue of the capital Kin the paper “A kind of neither Keynesian nor neoclassical model (1): fundamental equation” [12] :

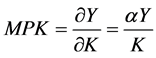(1)

In a competitive market, assuming that the marginal cost of using K is determined by the market interest rate r, the optimal allocation condition for K in production is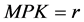, then

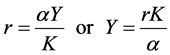, (2)

differential on both sides of the equation, so:

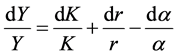, (3)

according to the basic equation,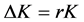

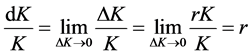, (4)

so Equation (3) can be rewritten as:

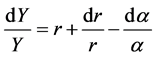(5)

When r and 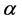are constant, 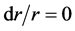, ,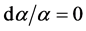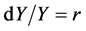. This is the macroeconomic basic equation. Equation (5) contains more information than the fundamental equation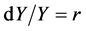. It can be used to analyze the relationship of short-term fluctuations between 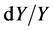and r.

In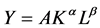, the income distribution parameter 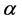mainly affects the long-term growth (We will analyze this problem in another paper “The economic growth”), assuming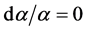, then Equation (5) can be simplified as:

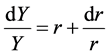(6)

This is the business cycle equation. The change of in short term depends on changes of r and. The interaction between r and might leads to endless fluctuation of.

By we can see that r reflects the production efficiency in the use of K. The periodic fluctuation in the statistical data can be described by the phase diagram in the mathematical model. Using r as the horizontal axis and as the vertical axis, we can plot the phase diagram of.

As shown in Figure 1(a), since, at points A and C, so r should not continue to change. However, since，, the point A or C is not a stable point on the Phase diagram.

At point B or D, , so r will continue to change. Therefore, once r moves up, the phase diagram will endogenously change and make in equation (7) periodically fluctuate. Moreover, we will see that the fluctuations of other variables are directly or indirectly related to the fluctuation of r.

According to the phase diagram, we can define the characteristic of periodic change of r, or be called the three elements of the phase diagram: firstly, point E in Figure 1(a) is the core of one cycle, and the interest rate that corresponds to point E is an average value of interest rate of a business cycle (, namely the core interest rate). Secondly, EA or EC is the radius of fluctuation of r. Thirdly, the rotation of the phase diagram is clockwise. Theoretically, we can call four phases of A→B, B→C, C→D and D→A in Figure 1 as period of recovery, prosperity, recession and depression.

Based on the phase diagram, we can easily draw out the time path of r as Figure 1(b) shows. In reality, since the value of core interest rate might be affected by external factors, such as the technological impact, therefore the location and radius of can be affected also. Therefore the time path of r cannot be a regular path as trigonometric functions.

Figure 2 is a phase diagram based on statistical data of the United States during 1970-2015. Although we can distinguish different cycles by these phase diagrams (as indicated by the dashed line in Figure 2 for two periods of 1972-1976 and 1976-1983, the cycle starting point is always on r-axis or the nearest it below), most of them have only two phases of rise and fall, without theoretical four phases of recovery, prosperity, recession and depression. Some of them even rotate counterclockwise, for example, during 1983-1985.

This is caused by the collection of data has longer interval time than the real fluctuation. Take semi-annual data to redraw this diagram, it is the dotted curve rather than the solid curve during 1983-1985. Therefore, 1976-1983 and 1983-1986 are two different cycles. In the same way, 2003-2009 and 2009-2012 are also two cycles in 2003-2012.

According to annual statistical data and the dividing rule showed in Figure 1(a), there are 9 cycles of fluctuation of interest rate during 1970-2015 of the United States: 1972-1976, 1976-1983, 1983-1986, 1986-1993, 1993-1998, 1998-2003, 2003-2009, 2009- 2012, 2012-. According to the present statistical data (10/2016), r in 2016 may not be

higher than 2015, so we guess 2016 is also in the cycle since 2012. Figure 3 shows the corresponding time path.

3. The Relationship between the Periodicity of dY/Y and r

According to Equation (6), the change rate of output is not only related to r but also to the change rate of interest rate. Based on the phase diagram, we can draw a phase diagram as Figure 4 shows. In order to deduce the phase diagram from, we first draw an auxiliary line which is, then fix points a, b, c, d and e that correspond to points A, B, C, D and E on based on.

Point a and c on are easy to be fixed, since on these two points, (on the phase diagram, r is the minimum value or the maximum value), therefore point a and c are located on the auxiliary line. Points b, d and e correspond to the maximum, minimum and average values of. In that case, ,. Similarly, we can determine the other points, connect these points to get the phase diagram.

Figure 5 shows the time path of and r according to. Figure 5(b) and Figure 5(c) are time paths of and r, respectively. In order to compare, we exchange the horizontal and vertical coordinates of Figure 5(b) to get Figure 5(d). By comparing Figure 5(c) and Figure 5(d), there is different of fluctuation of and r. We call it “phase difference”.

As shown in Figure 5(c) and Figure 5(d), the time path of and r are related as: when r increases,. From point b to c, when decreases, r keeps increase. Similarly, when r decreases,. And from point d to a, when starts to increase, r keeps decrease. This shows the peak and trough of exist earlier than that of r. Although the change of r might exist later that of, it does not mean is the cause of the change of r. Contrarily, the change of is the result of interaction of r and.

In Figure 6, statistical data during 1970-2015 verified the phase difference between time paths of and r. Figure 4 is generated under the assumption of. If, then the auxiliary line should be. Therefore when, the phase diagram would move downwards or be deflected, vice versa. So the flunctuation of in real world is much more complex than that of r, the peak or trough of would move forward or backward in the business cycle under the effect of.

The foundation equation derived assuming that r and are constant. According to Figure 4, it should be rewritten as. Although the core rate of output growth is equal to the core interest rate, the value of and rare not always equal, since them have different phases. Compared to the analysis of total demand and supply, the analysis of phase diagram could give better explanation of relations between short-terms values of macroeconomic variables.

4. Periodicity of the Unemployment Rate

Based on algebraic rules, no matter what are the original state of and, there is a dynamic upper limit of Cobb-Douglas function:

(7)

Apparently, the condition of to reach its upper limit is:

(8)

Equation (8) not only expresses the endogenous optimization of L and K, but also defines the relation between L and K. According to economic principles, the amount of production factors used in the system is determined by its marginal revenue. Therefore the amount of L that be used should be closely related to the marginal output of L (). Based on the marginal equation of:, assume, then

(9)

since, ,

among them, , (“A kind of neither Keynesian nor neoclassical model (1): The fundamental equation” [12] ), Assuming is a constant, so and, then

(10)

In, L is the monetized human resources, we cannot directly use amount of the employment population E as amount of L. There might be relation between (the change rate of employment) and (the change rate of marginal output of the monetized human resources), since we notice that the larger of the marginal contribution of L the more willingness of hiring employees of companies. Figure 7 shows, the fluctuation of and are move to the same direction in short- term, even though the range of fluctuation are different especially when the inflation is more serious.

The statistical data Y and r are the nominal values with money when calculate. E is the employed population. The nature of E is the non-monetary real variable.

When, which is calculated based on nominal values is greater than which is calculated based on real values. If Equation (10) is calculated based on real values and, then

(11)

Since, and，in which is the rate of inflation, then

Since, the real variable and the nominal variable is expressed as

(12)

As Figure 8 shows, the fluctuation of and are approximately similar. The reason for they are not completely consistent is because we assume keep unchanged and therefore the change of is not included in the impact on.

Above statistical data show that the change rate of employment is determined by the change rate of marginal real output of human resources. Therefore we assume and replaced in Equation (11) with, then

According to Equation (6) assume, then

(13)

The structure of Equation (13) is similar to that of, therefore we can draw the phase diagram according to the phase diagram as Figure 4 shows. According to the rate of change of employed population and (labor population as N, employed population as E), we can convert

into:, according to Equation (13)

(14)

As Figure 9 shows, the direction of rotation and shape of the phase diagram is the same as that of. Core variables and locate differently on the vertical coordinate, and the difference is determined by the change rate of labor population. In other words, we get the phase diagram of by moving the phase diagram of downward a distance of. Therefore, we can predict that the fluctuation of and are consistent.

According to Equation (13), the reason of short-term fluctuation of is the periodicity factor, which is similar to the reason of the fluctuation of. If there is no phase or only tiny difference between the fluctuation of and, and the phase difference between r and r_r is small, then the fluctuating paths of, and would be consistent. The statistical data of Figure 10 can verify this conjecture.

The phase diagram in Figure 9 shows that the change rate of employment rate maybe 0 under the effect of sometimes. Mathematically, first order differential indicates there is extreme value or inflection point on. Therefore, there is a phase diagram between and probably. The phase diagram in Figure 11 is from the statistical data.

In order to discuss the relationship between the unemployment rate and other macroeconomic variables based on the expression of Phillips curve, we often need to convert the employment rate to the unemployment rate. Since the labor population N is the sum of employed population E and unemployed population U, namely, then the relation between and, and the relation between and are:

(15)

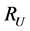
Based on Equation (15) we can convert the phase diagram to. The phase diagram of and is almost mirror images.

The is not the. As there is a phase difference between and, there is also a phase difference between and, although their phase differences may not be significant in yearly statistical data. Figure 12 shows the fluctuation of and in the statistical data. Before 1986, the peaks of and appeared at the same time (which is the reason for inflation, which we will describe in later papers). After 1986, the peak of appeared later than. The peaks of and are the same in Figure 10, because and are the opposite of the peak, therefore the peak of will lag behind the peak of after 1986. This helps us understand the relationship and causes of fluctuations of unemployment and output.

While the above analysis explains the reasons for the cycle in the unemployment rate, there is one fundamental problem that remains unsolved: We cannot determine the value of or according to Equations (17) and (14) in a business cycle. Because in the differential equation, we need to know some initial conditions to determine or. As and, we cannot determine from without knowing the initial condition of. This means that even if the of the two economic entities are exactly the same, their are not necessarily equal, because the marketization degree of employment and the culture of employment is different in different countries or regions.

Friedman considered although the unemployment state would be affected by “market imperfections, stochastic variability in demands and supplies, the cost of gathering information about job vacancies and labor availabilities, the costs of mobility, and so

on” [13] , the unemployment rate fluctuated around the natural rate of unemployment in short term. Factors that Friedman took as examples are also initial conditions that affect the differential equation. However, the natural rate of unemployment is not the core unemployment rate, since move back and forwards in different cycles as Figure 11 shows.

5. Conclusions

5.1. Hypothesis

² Production function in the market system:.

² A marginal condition:.

² , ,.

5.2. Results

² The cycle equation about the output:.

² The cycle equation about the employment:, or.

5.3. Discussion

² Traditional macroeconomics cannot logically explain contradictions of economic problems in long-term and short-term. Classical theory seems to be handy in explaining relationships between variables in long-term, but it is difficult to understand the phenomenon in short-term. Keynesian theory, while able to explain some phenomenon in short-term, but there will be ridiculous inference in long-term. From the model in this paper and “A kind of neither Keynesian nor neoclassical model (1): the fundamental equation” [12] , we can logically and consistently see relationships between the macroeconomic variables in the long-term and the short- term, and use statistical data to verify these relationships.

² The cycle is affected by many factors, but as long as the marginal product of the economic system is not zero, there is the business cycle even without these external stochastic factors. Since r and are the first and second order differentials of Y, the fluctuation of output is due the effect of the second order differential on the first order differential. Therefore the business cycle is fundamentally an endogenous producing action. Because both endogenous and exogenous forces have impacts on the cycle, so it is difficult to see two cycles are the same in terms of fluctuation and time consumption. On the interchangeability between inflation and unemployment in the Phillips Curve, we need to further our study on the relationship between money and Cobb-Douglas function.

² Due to the limited data sources and the heavy workload of processing data, this paper is limited to the verification of annual data. It is not known whether these periodic equations also apply to quarterly or monthly data.

Cite this paper

Zhan, M.A. and Zhan, Z. (2016) A Kind of Neither Keyne- sian Nor Neoclassical Model (2): The Busi- ness Cycle. Open Access Library Journal, 3: e3215. http://dx.doi.org/10.4236/oalib.1103215

References