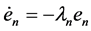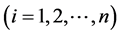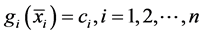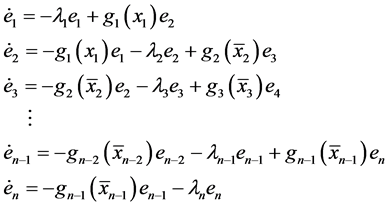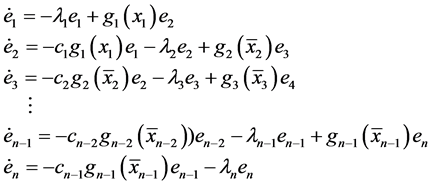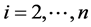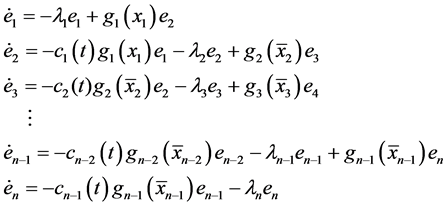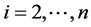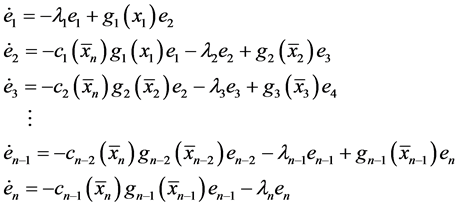Open Access Library Journal
Vol.03 No.11(2016), Article ID:71849,12 pages
10.4236/oalib.1103061
Backstepping Technique Based on Error
Li Wang
School of Hydraulic, Energy and Power Engineering, Yangzhou University, Yangzhou, China

Copyright © 2016 by author and Open Access Library Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: September 14, 2016; Accepted: November 5, 2016; Published: November 9, 2016
ABSTRACT
Backstepping technique usually adopts back step design to construct the Lyapunov function gradually, and then to design the corresponding virtue controller. The backstepping technique based on error also adopts back step design process, but the design of virtue controllers depends on the corresponding errors which are designed to satisfy some expected behaviors. Six different error equations are deduced by changing the results of the virtue controls arbitrarily while guaranteeing the system behaviors such as stability, and an example shows the effectiveness of these six versions. Simulated results illustrate that these six versions of backstepping technique based on error are effective.
Subject Areas:
Dynamical System
Keywords:
Backstepping Technique, Lyapunov Function, Error, Strictly Feedback Nonlinear Control System

1. Introduction
Backstepping design methodology is among the most important nonlinear control design techniques with numerous applications. It was first presented by P. V. Kokotovic and his coauthors, see [1] [2] [3] and references therein, and was formulated by Krstic et al. in [4] , and was advanced in many papers and not limited in the following. Murat Arcak et al. [5] presented robustification of backstepping design methodology by two versions: cancellation backstepping and LGV-backstepping. In reference [6] , about ten-year development of this recursive design was introduced. Dragan B. Dacic et al. [7] studied the use of backstepping design methodology in power integrator triangular systems. Dimitrios Karagiannis et al. [8] considered an alternative to adaptive backstepping. Keng Peng Tee et al. [9] employed a barrier Lyapunov function for the control of output-constrained nonlinear systems.
In all the references mentioned above, the purpose of backstepping design methodology is the construction of various types of control Lyapunov functions: stable, adaptive, robust, etc. D. Swaroop et al. [10] introduced Dynamic Surface Control (DSC), which was similar to backstepping and multiple surface control methods, but with an important addition, one low pass filter was included in the design which ended the complexity arising due to the “explosion of terms” that had made backstepping methods difficult to implement in practice. In recent years, DSC has received a great deal attention, see [11] [12] and references therein.
A new redesign backstepping technique, backstepping technique based on error is presented in this paper, which also adopts backstepping design process, but doesn’t construct the system, control Lyapunov function; the design of the virtue controller depends on the corresponding errors which are designed to satisfy some expected behaviors. The method is also more flexible than DSC. Based on some results of [13] , we deduce six different error equations by changing the result of the virtue control law arbitrarily while guaranteeing the system behaviors such as stability.
In this paper, we call backstepping technique based on control Lyapunov functions as conventional backstepping, and call backstepping technique based on error as error backstepping. Section 2 presents the design and six results of error backstepping, in Section 3 an example shows the effectiveness of these six versions, and Section 4 concludes this paper.
2. Backstepping Technique Based on Error
Consider a usual strictly feedback nonlinear system as follows:
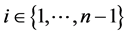
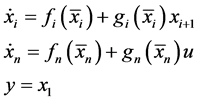 (1)
(1)
where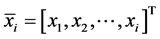 ,
, 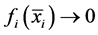 , when
, when 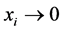
 ,
, 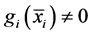 ,
,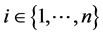 . The design objective is to make
. The design objective is to make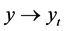 .
.
The backstepping design process is as follows.
Step 1: Consider the control goal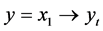 , take
, take  as the first virtual control and pretend that
as the first virtual control and pretend that 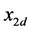 satisfies the following equation.
satisfies the following equation.
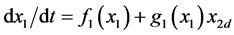 (2)
(2)
Define the first tracking error
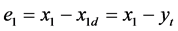 (3)
(3)
Error 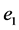 is wanted to converge to zero exponentially, therefore select the desired behaviour to be
is wanted to converge to zero exponentially, therefore select the desired behaviour to be 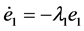
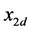 is chosen to satisfy the required dynamic characteristic.
is chosen to satisfy the required dynamic characteristic.
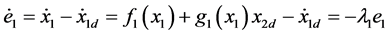 (4)
(4)
Then the following equality corresponds to desired behaviour
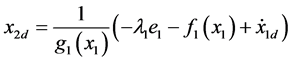
Step 2: but we cannot just choose 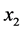
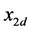
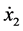
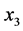
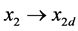
Introduce
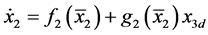
Define the second tracking error:
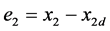
The error 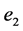
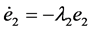
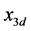
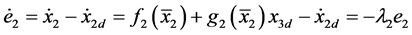
Then the following equality corresponds to desired behavior.

Step i: Choose 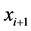
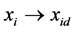
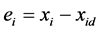
Select its time derivative to satisfy the following behaviou

Introduce
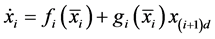
Choose 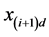
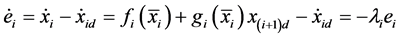
Then has
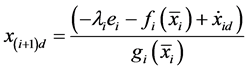
Step n: Choose u to solve the 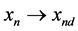
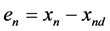
Its derivative satisfies.
In fact:
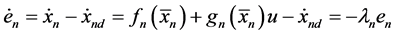
The last equality corresponds to what we are forcing.
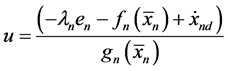
The deduced error equation is:

Defining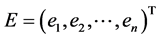
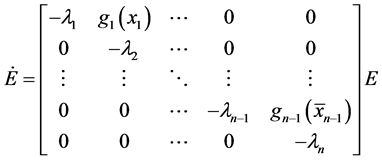
Proposition 2.1: When choose parameters

where 
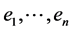
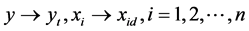
Proposition 2.2: When 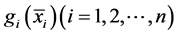
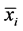
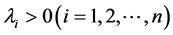
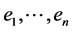
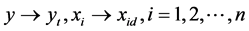
Proof: error Equation (19) is in short as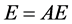
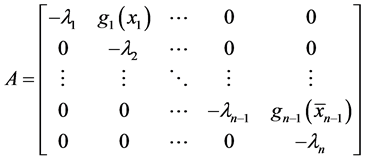
Introduce
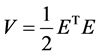
Its time derivative is
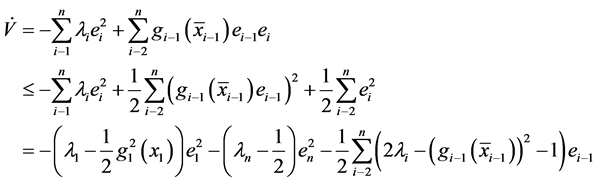
If parameters 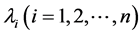
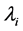
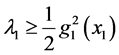
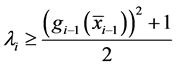

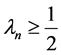
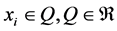
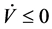
End of proof.
Proposition 2.3: Assume the expression of virtual control (14) is changed into:
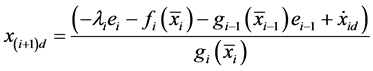
where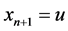
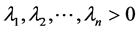
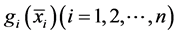

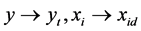
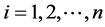
Proof: when virtual control (24) takes place (14) in recursive procedure, the new error equation is changed into
or
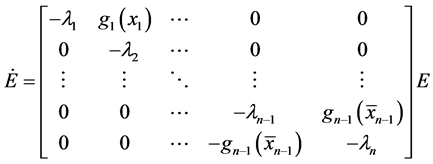
It is the same as (2.27). Then all the errors converge to origin globally.
End of proof.
Proposition 3.4: Similarly assume the expression of virtual control (8) is changed into:
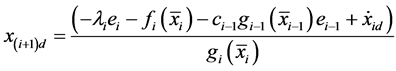
where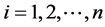
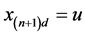
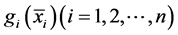
or

When choose the parameters 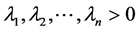
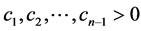
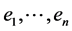
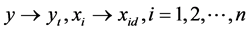
Proof:
Defining
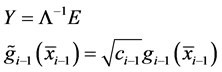
where
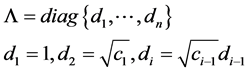
Then
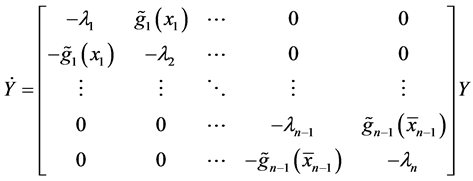
Introduce a positive definite quadratic function
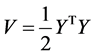
It can be obtained

Then 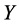
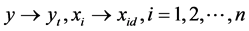
End of proof.
Proposition 2.5: The virtual control can also be choose as
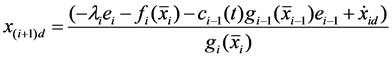
where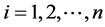
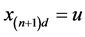
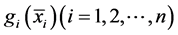
or
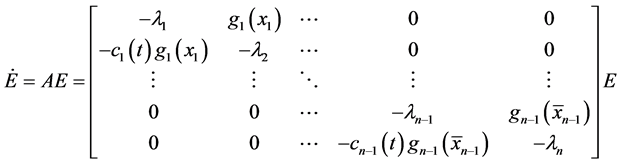
When choose parameters 
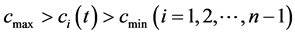

Errors 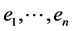
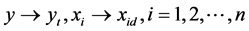
Proof:
Define 
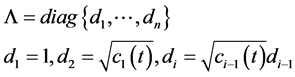
Then it can be obtained

where
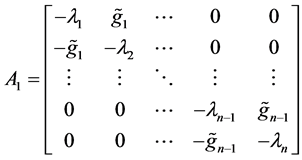
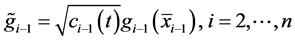
Introduce a positive definite function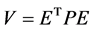
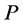

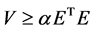
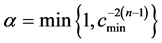
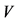
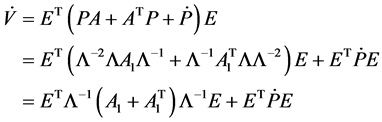
Because
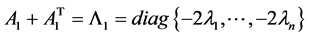
So
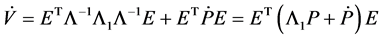
It is obvious that 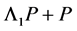
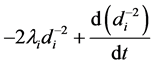
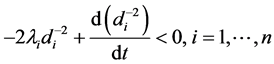
Substituting (42) into (43), it can be deduced that 
End of proof.
Proposition 2.6: The virtual control can also be choose as
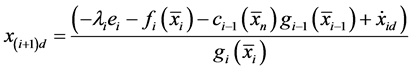
where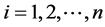


or
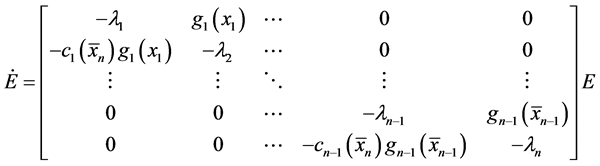
When choose parameters 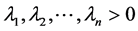
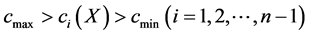
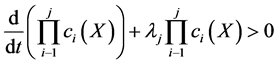
Errors 

Proof: it is similar to the proof of Proposition 2.5.
3. Numerical Simulation
Consider the following two-order system:
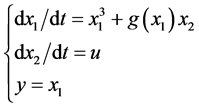
The control objective is to design a state feedback control to asymptotically stabilize the origin.
We adopt backstepping technique based on error to design control law. The calculated results are presented in Table 1 by choosing parameters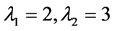
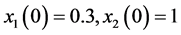
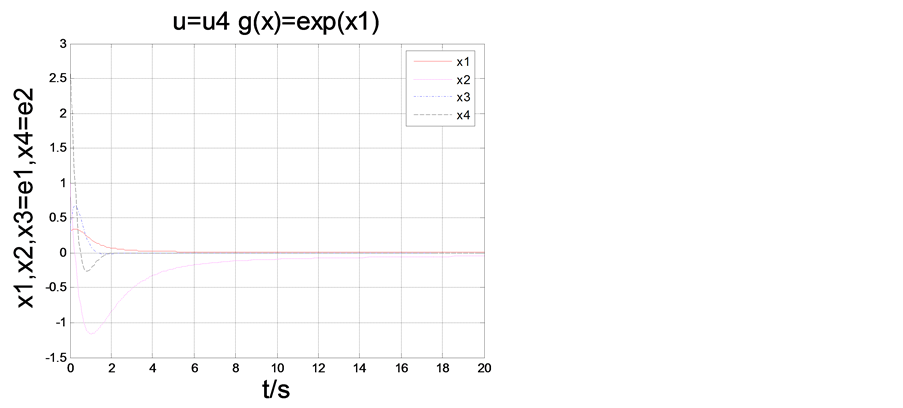
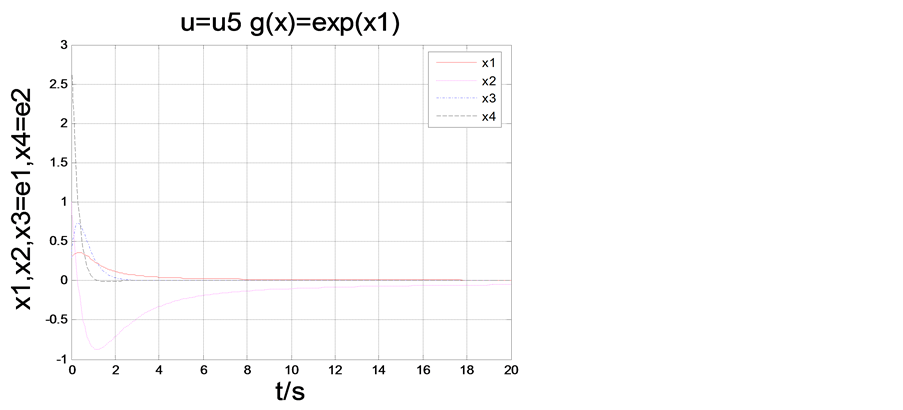
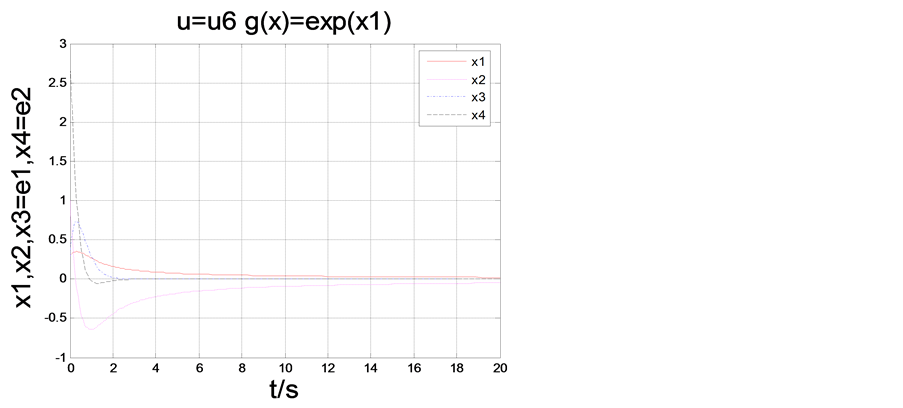
As it is shown in Figure 1 the transients of state variables 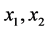
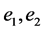
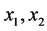
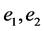
Table 1. Calculated results.
Figure 1. Simulated results.
4. Conclusion
The backstepping technique based on error is the expansion of the backstepping technique, it adopts backstepping design process, but the design of the virtue controller depends on the corresponding errors which are designed to satisfy some expected behaviors. This method makes the design systematical and structural, and it can change the result of the virtue control law arbitrarily in six forms while guaranteeing the system stability. The method can be used for both stabilization control problems and tracking control problems. Subjects of future research include the discussions of systems that contain uncertain terms, unknown parameters or unmeasured signals.
Cite this paper
Wang, L. (2016) Backstepping Technique Based on Error. Open Access Library Journal, 3: e3061. http://dx.doi.org/10.4236/oalib.1103061
References
- 1. Kanellakopoulos, I., Kokotovic, P.V. and Morse, A.S. (1991) Systematic Design of Adaptive Controllers for Feedback Linearizable Systems. IEEE Transactions on Automatic Control, 36, 1241-1253.
- 2. Kovotovic, P.V. (1992) The Joy of the Feedback: Nonlinear and Adaptive. IEEE Control System, 12, 7-17.
- 3. Kokotovic, P.V., Krstic, M. and Kmellakopoulos, I. (1992) Backstepping to Passivity: Recursive Design of Adaptive Systems. Proceedings of the 31st Conference on Decision & Control, Tucson, 16-18 December 1992, 3276-3280.
- 4. Krstic, M., Kanellakopoulos, I. and Kokotovic, P.V. (1995) Nonlinear and Adaptive Control Design. John Wiley & Sons, New York.
- 5. Arcak, M., Seron, M., Braslavsky, J. and Kokotovic, P.V. (2000) Robustification of Backstepping against Input Unmodeled Dynamics. IEEE Transactions on Automatic Control, 45, 1358-1363.
http://dx.doi.org/10.1109/9.867048 - 6. Kokotovic, P. and Arcak, M. (2001) Constructive nonlinear control: A Historical Perspective. Automatica, 37, 637-662.
http://dx.doi.org/10.1016/S0005-1098(01)00002-4 - 7. Dacic, D.B. and Kokotovic, P.V. (2005) A Scaled Feedback Stabilization of Power Integrator Triangular Systems. Systems & Control Letters, 54, 645-653.
http://dx.doi.org/10.1016/j.sysconle.2004.11.004 - 8. Karagiannis, D. and Astolfi, A. (2008) Nonlinear Adaptive Control of Systems in Feedback Form: An Alternative to Adaptive Backstepping. Systems & Control Letters, 57, 733-739.
http://dx.doi.org/10.1016/j.sysconle.2008.02.006 - 9. Tee, K.P., Ge, S.S. and Tay, E.H. (2009) Barrier Lyapunov Functions for the Control of Output-Constrained Nonlinear Systems. Automatica, 45, 918-927.
http://dx.doi.org/10.1016/j.automatica.2008.11.017 - 10. Swaroop, D., Hedrick, J.K., Yip, P.P. and Gerdes, J.C. (2000) Dynamic Surface Control for a Class of Nonlinear Systems. IEEE Transactions on Automatic Control, 45, 1893-1899.
http://dx.doi.org/10.1109/TAC.2000.880994 - 11. Chen, L. and Pan, C.P. (2009) Dynamic Surface Backstepping Control Design for One Hypersonic Vehicle. Proceedings of the 2009 IEEE International Conference on Mechatronics and Automation, Changchun, 9-12 August 2009, 4770-4774.
http://dx.doi.org/10.1109/ICMA.2009.5246455 - 12. Song, B. (2006) Decentralized Dynamic Surface Control for a Class of Interconnected Nonlinear Systems. Proceedings of the 2006 American Control Conference, Minneapolis, 14-16 June 2006, 130-135.
http://dx.doi.org/10.1109/ACC.2006.1655342 - 13. Liu, B. and Zhang, Z.K. (2007) Stability of Nonlinear Systems with Tridiagonal Structure and Its Application. Acta Automatica Sinica, 33, 442-445.