F. BUKHARI
1174
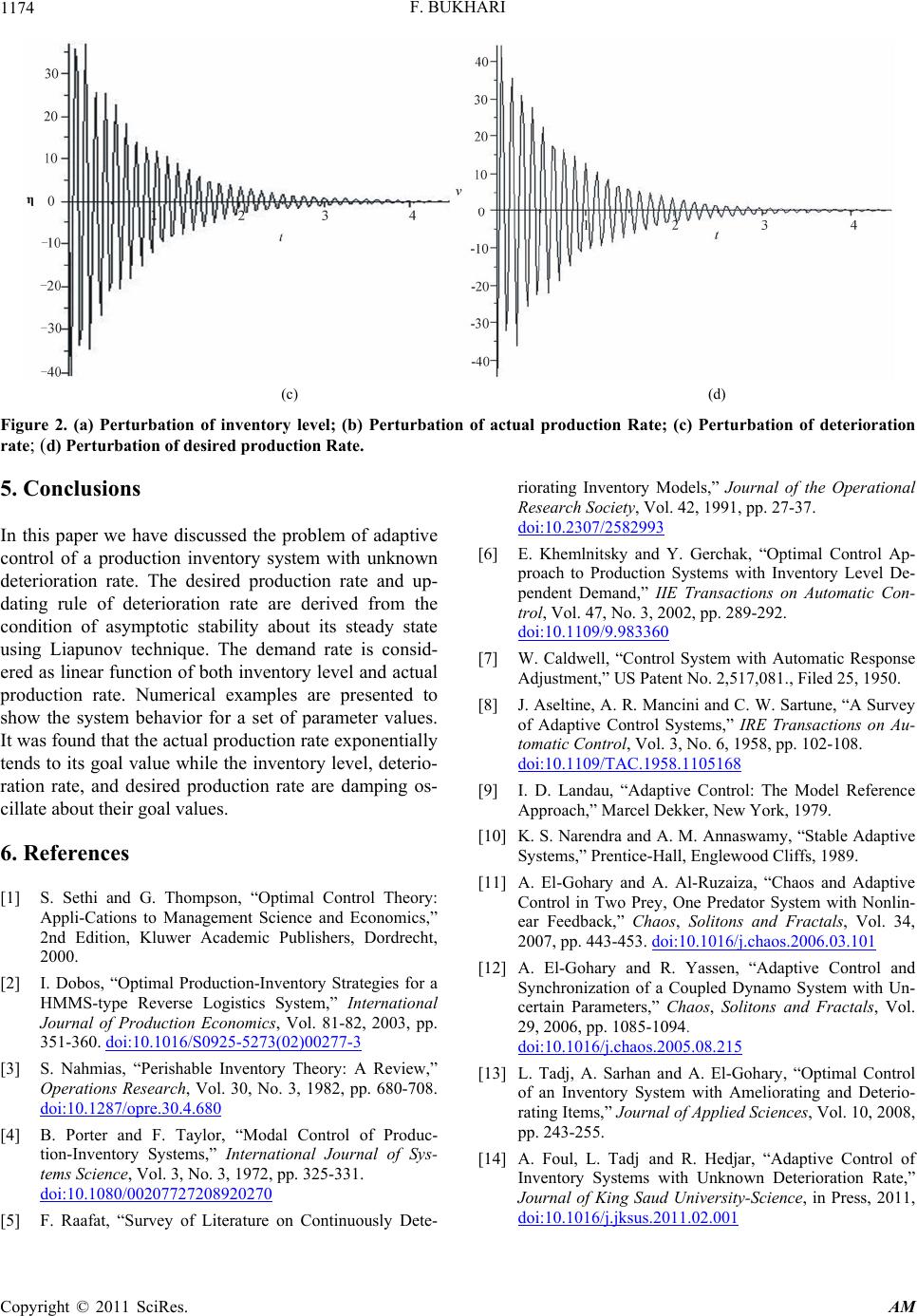
(c) (d)
Figure 2. (a) Perturbation of inventory level; (b) Perturbation of actual production Rate; (c) Perturbation of deterioration
rate; (d) Perturbation of desired production Rate.
5. Conclusions
In this paper we have discussed the problem of adaptive
control of a production inventory system with unknown
deterioration rate. The desired production rate and up-
dating rule of deterioration rate are derived from the
condition of asymptotic stability about its steady state
using Liapunov technique. The demand rate is consid-
ered as linear function of both inventory level and actual
production rate. Numerical examples are presented to
show the system behavior for a set of parameter values.
It was found that the actual production rate exponentially
tends to its goal value while the inventory level, deterio-
ration rate, and desired production rate are damping os-
cillate about their goal values.
6. References
[1] S. Sethi and G. Thompson, “Optimal Control Theory:
Appli-Cations to Management Science and Economics,”
2nd Edition, Kluwer Academic Publishers, Dordrecht,
2000.
[2] I. Dobos, “Optimal Production-Inventory Strategies for a
HMMS-type Reverse Logistics System,” International
Journal of Production Economics, Vol. 81-82, 2003, pp.
351-360. doi:10.1016/S0925-5273(02)00277-3
[3] S. Nahmias, “Perishable Inventory Theory: A Review,”
Operations Research, Vol. 30, No. 3, 1982, pp. 680-708.
doi:10.1287/opre.30.4.680
[4] B. Porter and F. Taylor, “Modal Control of Produc-
tion-Inventory Systems,” International Journal of Sys-
tems Science, Vol. 3, No. 3, 1972, pp. 325-331.
doi:10.1080/00207727208920270
[5] F. Raafat, “Survey of Literature on Continuously Dete-
riorating Inventory Models,” Journal of the Operational
Research Society, Vol. 42, 1991, pp. 27-37.
doi:10.2307/2582993
[6] E. Khemlnitsky and Y. Gerchak, “Optimal Control Ap-
proach to Production Systems with Inventory Level De-
pendent Demand,” IIE Transactions on Automatic Con-
trol, Vol. 47, No. 3, 2002, pp. 289-292.
doi:10.1109/9.983360
[7] W. Caldwell, “Control System with Automatic Response
Adjustment,” US Patent No. 2,517,081., Filed 25, 1950.
[8] J. Aseltine, A. R. Mancini and C. W. Sartune, “A Survey
of Adaptive Control Systems,” IRE Transactions on Au-
tomatic Control, Vol. 3, No. 6, 1958, pp. 102-108.
doi:10.1109/TAC.1958.1105168
[9] I. D. Landau, “Adaptive Control: The Model Reference
Approach,” Marcel Dekker, New York, 1979.
[10] K. S. Narendra and A. M. Annaswamy, “Stable Adaptive
Systems,” Prentice-Hall, Englewood Cliffs, 1989.
[11] A. El-Gohary and A. Al-Ruzaiza, “Chaos and Adaptive
Control in Two Prey, One Predator System with Nonlin-
ear Feedback,” Chaos, Solitons and Fractals, Vol. 34,
2007, pp. 443-453. doi:10.1016/j.chaos.2006.03.101
[12] A. El-Gohary and R. Yassen, “Adaptive Control and
Synchronization of a Coupled Dynamo System with Un-
certain Parameters,” Chaos, Solitons and Fractals, Vol.
29, 2006, pp. 1085-1094.
doi:10.1016/j.chaos.2005.08.215
[13] L. Tadj, A. Sarhan and A. El-Gohary, “Optimal Control
of an Inventory System with Ameliorating and Deterio-
rating Items,” Journal of Applied Sciences, Vol. 10, 2008,
pp. 243-255.
[14] A. Foul, L. Tadj and R. Hedjar, “Adaptive Control of
Inventory Systems with Unknown Deterioration Rate,”
Journal of King Saud University-Science, in Press, 2011,
doi:10.1016/j.jksus.2011.02.001
Copyright © 2011 SciRes. AM