Applied Mathematics, 2011, 2, 1124-1128
doi:10.4236/am.2011.29155 Published Online September 2011 (http://www.SciRP.org/journal/am)
On the Growth and Polynomial Coefficients of
Entire Series
Huzoor H. Khan1, Rifaqat Ali2
1Department of Mathematics, Aligarh Muslim University, Aligarh, India
2Department of Applied Mathematics, Aligarh Muslim University, Aligarh, India
Received July 9, 2011; revised August 6, 2011; accepted August 13, 2011
Abstract
In this paper we have generalized some results of Rahman [1] by considering the maximum of ()
f
z over a
certain lemniscate instead of considering the maximum of ()
f
z, for zr

and obtain the analogous re-
sults for the entire function
 
1
1
() k
k
k
fzp zqz






 where


qz is a polynomial of degree m and

k
p
z is of degree m  1. Moreover, we have obtained some inequalities on the lover order, type and lower
type in terms of polynomial coefficients.
Keywords: Lemniscate, Lower Order, Lower Type, Slowly Changing Function, Polynomial Coefficients and
Entire Functions.
1. Introduction
Let
0
() n
n
n
f
za


z
be a nonconstant entire function and assume that an ≠ 0
for n = 1, 2, 3, For classifying entire functions by
their growth, the concept of order was introduced. If the
order is a (finite) positive number, then the concept of
type permits a subclassification. For the class of order

= 0 and
 =  no subclassification is possible. For exam-
ple all entire functions that grow at least as fast as exp
(exp (z)) have to be kept in one class. For this reason,
numerous attempts have been made to refine the concept
of order and type. Boas [2] define the order
 (0 ≤  ≤  )
and the type T (0 ≤ T ≤) as follows:

1
log log,log
lim suplim sup
log log| | rn
n
Mrfnn
ra


 
 (1.1)

/
log ,1
lim suplim sup||n
n
rn
MrfTna
e r


  

 




|
(1.2)
where
 
||
,max|
zr
M
rff z

,
Rahman [1] studied the type by taking the function
, in place of

1
1
log a rr


log k
a
krr

and shown
that


 
 
1
1
1
1
/ 1
1
log ,
lim sup()
log,..... log
||
lim sup
log,..... log
k
k
a a
rk
n
a
n
a a
nk
MrfT
rr r
na
enn












(1.3)
In this paper, we show that instead of considering the
maximum of |f(z)|, for |z| = r, we can consider the maxi-
mum of f(z) over a certain lemniscate and obtained
analogous results for entire function
f(z) = ,

1
1
() k
k
k
pzqz





Walsh [3], Borwein [4], where q(z) is a polynomial of
degree m and pk(z) is of degree m
 1 and the equipoten-
tial curve |q(z)| = R defines the lemniscate mentioned
above, various authors such as Rice ([5,6]), Juneja [7],
Juneja and Kapoor [8], Kumar [9], Kumar and Kaur [10]
studied the growth of above entire function but non of
them studied the analogous results of (1.3). Therefore we
have obtained lower order, type and lower type in terms
of polynomial coefficients.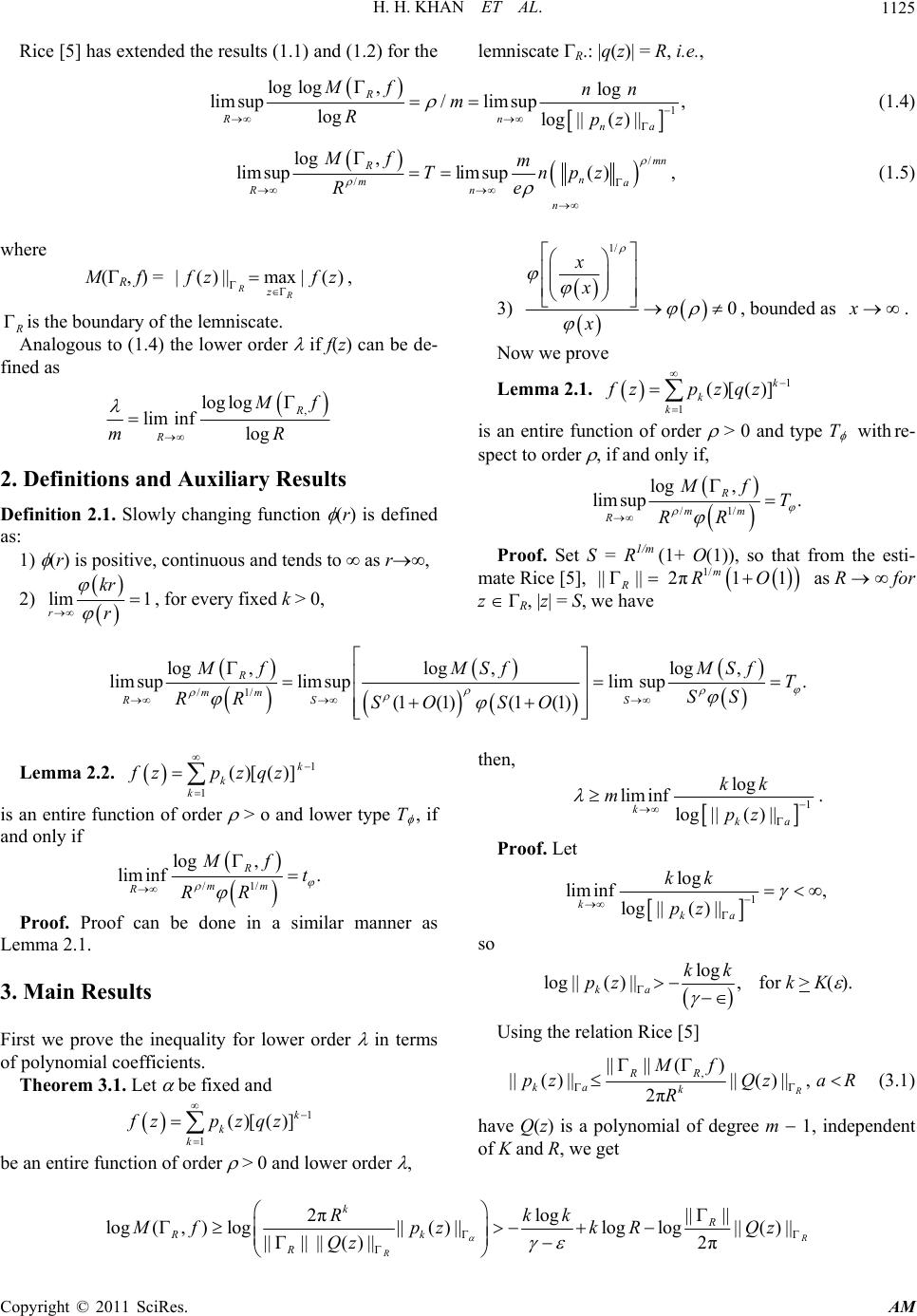

H. H. KHAN ET AL.1125
Rice [5] has extended the results (1.1) and (1.2) for the lemniscate R.: |q(z)| = R, i.e.,


1
log log,log
lim sup/lim sup,
log log||() ||
R
Rn
na
Mf nn
m
Rpz


 
 (1.4)


/
/
log ,
lim suplim sup(),
mn
R
n
ma
Rn
n
Mf m
Tnpz
e R




 

 (1.5)
where
M(R, f) = |()|| max|()
RR
z
f
zf

z
,
R
is the boundary of the lemniscate.
Analogous to (1.4) the lower order
 if f(z) can be de-
fined as

,
log log
lim inflog
R
R
M
f
mR




2. Definitions and Auxiliary Results
Definition 2.1. Slowly changing function  (r) is defined
as:
1)  (r) is positive, continuous and tends to  as r,
2)


lim 1
r
kr
r


 , for every fixed k > 0,
3)

 
1/
0
x
x
x











 , bounded as
x
.
Now we prove
Lemma 2.1.

1
1
()[()]
k
k
k
fz pzqz



is an entire function of order  > 0 and type T
 with re-
spect to order
 , if and only if,


/1/
log ,
lim sup.
R
mm
R
Mf
T
RR




Proof. Set S = R1/m (1+ O(1)), so that from the esti-
mate Rice [5], as R   for
z  R, |z| = S, we have
 
1/
|||| 2π11
m
RRO 








/1/
log,log ,log ,
lim suplim suplimsup.
(1 (1)(1 (1)
R
mm
RSS
Mf MSfMSf
T
SS
RR SO SO





  








Lemma 2.2.

1
1
()[()]
k
k
k
fz pzqz



is an entire function of order  > o and lower type T
 , if
and only if



/1/
log ,
lim inf.
R
mm
R
Mf
t
RR





Proof. Proof can be done in a similar manner as
Lemma 2.1.
3. Main Results
First we prove the inequality for lower order  in terms
of polynomial coefficients.
Theorem 3.1. Let  be fixed and

1
1
()[()]
k
k
k
fz pzqz



be an entire function of order
 > 0 and lower order  ,
then,

1
log
lim inf
log||() ||
k
ka
kk
m
pz


 
.
Proof. Let

1
log
lim inf,
log||() ||
k
ka
kk
pz


 

so

log
log||() ||,
ka
kk
pz

  for k > K(
 ).
Using the relation Rice [5]
,
|||| ()
||()||||() ||,
2πR
RR
ka k
Mf
pzQza R
R



 (3.1)
have Q(z) is a polynomial of degree m  1, independent
of K and R, we get
|| || 2πlog
log(,)log||() ||loglog||()||
||||||() ||2π
R
R
k
R
Rk
R
Rkk
MfpzkR Qz
Qz







 





H. H. KHAN ET AL. 1126
Choose R =

1/ ,ek

  since
 < R, for sufficiently large k, we obtain


1/
1
log(,)loglog( )log( )
2π2π
R
R
RR
R
MfkRkQz kQz
 





or

log,log( )
2π
R
R
R
MfRe Qz
 


 
or
 

log log,loglog1log()
2π
R
R
R
MfRe eRQz





 


In view of a result of Rice [5]

1/
|| ||1(1)
2π
m RRO

,
we get


log log,(1)
log
R
Mf O
R

 .
Proceeding to limit as R  , we get
m


.
Hence the proof is complete.
Theorem 3.2. Let  be fixed. The necessary and suf-
ficient condition that

1
1
()[()]
k
k
k
fz pzqz



is an entire function of type T
 (0 ≤ T 
<  ) with respect
to order  (0 ≤  < ), is


/ log ,
lim suplim sup||()||
() () ()
mk
k
rk
Mrfmk
Tp
ek rr




  

 


z (3.2)
Proof. Let

/
lim sup()
()
mk
k
k
kpz
k





.
Then for a given  > 0, we get
/
()
||() ||()
mk
kzk
k









.
For an infinite sequence of values of k, so that from
(3.1), we have
/
/()
2π()
(,) () R
mk
m
R
R
Rk
k
Mf Qz









 

( 3.3)
Choosing a sequence of values of R such that


mke
Rk



.
For these R the right hand side of (3.3) attains its
maximum value, that is,
/
2π()
(,) ()
R
mk
R
R
e
Mf Qz



,
or
log(,)log 2πlog( )R
RR
mk
Mf Qz



 

or


/1/ /1/1/
log(, )()
(1) (1)
() ()
()(
R
mm mm
Mf mk mk
OO
e
RR RRke
k
 
 

 











Applying the limits as R  , we get
()1
()
m
Te





 (3.4)
In order to prove reverse inequality, from (3.2) we
have

/
() ,
()
mk
k
kpz
k





 for sufficiently large k.
(3.5)
Now following the same manner as in the proof of
(3.4), we get
C
opyright © 2011 SciRes. AM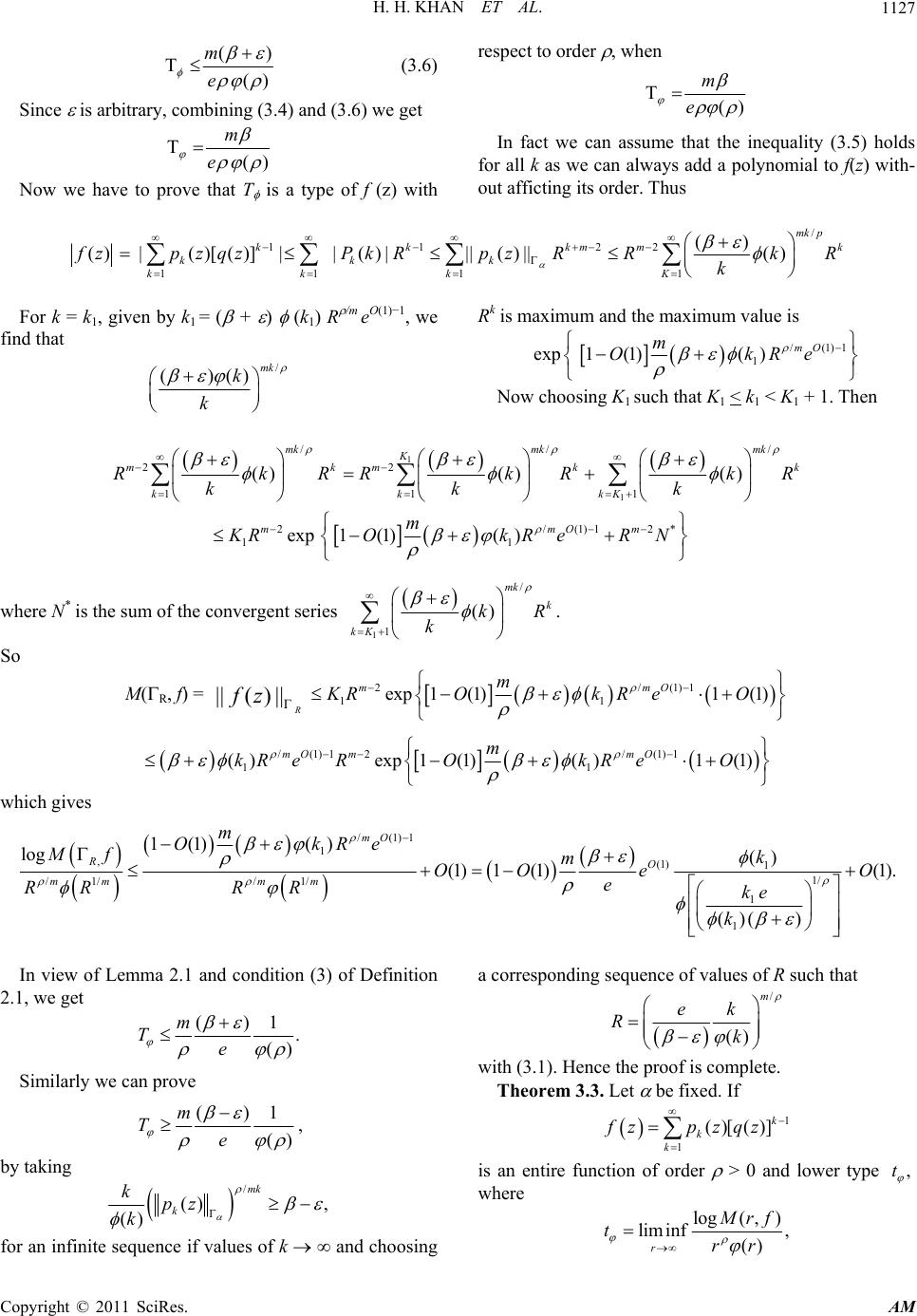

H. H. KHAN ET AL.1127
(
()
m
e

)





 (3.6)
Since  is arbitrary, combining (3.4) and (3.6) we get
()
m
e





Now we have to prove that T

is a type of f (z) with
respect to order
 , when
()
m
e





In fact we can assume that the inequality (3.5) holds
for all k as we can always add a polynomial to f(z) with-
out afficting its order. Thus
/
11 22
1111
()
()|()[()]|| ()|||()||()
mk p
kk kmm
kkk
kkkK
k
f
zpzqzPkR pzRRk
k




 

 


 


 R
For k = k1, given by k1 = (  +  )  (k1) R
 /m eO(1)−1, we
find that
/
()()
mk
k
k

 



Rk is maximum and the maximum value is
 
/(1)1
1
exp1(1)()mO
m
OkR




e






Now choosing K1
such that K1 < k1 < K1 + 1. Then
 


1
1
//
22
111
2/(1)12*
11
()() ()
exp1(1)()
mkmk mk
K
mkmk
kkkK
mmOm
R kRR kRkR
kkk
m
KROk ReRN


 



 



 

 
 





/
k


where N* is the sum of the convergent series

1
/
1
()
mk
k
kK
kR
k







.
So
M(R, f) =
R
zf ||)(||

 
2/
11
exp 1(1)1(1)
mm
m
KROk ReO



(1)1O


 



   
/(1) 12/(1) 1
11
()exp1 (1)()1 (1)
mO mmO
m
kR eROkR eO



 


 



which gives






/(1)1
1
,(1) 1
1/ /1//1/
1
1
1(1) ()
log ()
(1) 1(1)(1).
()( )
mO
RO
mmm m
m
OkRe
Mf k
m
OO eO
e
RR RRke
k

 
 


 













In view of Lemma 2.1 and condition (3) of Definition
2.1, we get
()1
.
()
m
Te





Similarly we can prove
()1
()
m
Te




,
by taking

/
() ,
()
mk
k
kpz
k






for an infinite sequence if values of k   and choosing
a corresponding sequence of values of R such that

/
()
m
ek
Rk







with (3.1). Hence the proof is complete.
Theorem 3.3. Let  be fixed. If

1
1
()[()]
k
k
k
fz pzqz



is an entire function of order  > 0 and lower type ,t

where
log( ,)
lim inf,
() r
M
rf
trr

 


H. H. KHAN ET AL. 1128
Then

/
lim inf()
() ()
mk
k
k
mk
pz t
ek



 


 (3.7)
Proof. The proof of this theorem follows on the lines
of the necessary part of Theorem 3.2, by writing


/
'liminf( )
()
mk
k
k
kpz
k






and for a given  > 0,


/
()( ')
mk
k
kpz
k






for k > K(  ),
so that we may choose a sequence of values of R satisfy-
ing
/.
()( ')
mke
Rk




Now using the relation (3.1) and Lemma 2.2, we get
the required result. Hence the proof is complete.
Remark 3.1. Theorem 3.2 is the generalization of the
result (1.3) by Rahman [1].
Remark 3.2. Rahman’s theorem [1], if

1
1
log ,
() lim inf(log),....(log) k
rk
Mrf
T
krr r

 



then
1
1
1
/
1
() liminf||
(log),....(log)k
n
n
nk
n
Ta
enn

 







is a special case of Theorem 3.3, if we take
and consider the circle |z| =
r, instead if the lemniscate |q(z)| = R.
  
1
1
log,... logk
a a
k
rr r  
4. References
[1] Q. I. Rahman, “On the Coefficients of an Entire Series of
Finite Order,” Math Student, Vol. 25, 1957, pp. 113-121.
[2] R. P. Boas, “Entire Functions,” Academic Press, New
York, 1954, pp. 9-11.
[3] J. L. Walsh, “Interpolation and Approximation,” Ameri-
can Mathematical Society, Colloquim Publications, Provi-
dence, Vol. 20, 1960, p. 56.
[4] P. Borwein, “The Arc Length of the Lemniscate {|p(z)| =
1},” Proceedings of the AMS—American Mathematical
Society, Vol. 123, 1995, pp. 797-799.
[5] J. R. Rice, “A Characterization of Entire Functions in
Terms of Degree of Convergence,” Bulletin of the Ameri-
can Mathematical Society, Vol. 76, 1970, p. 129.
doi:10.1090/S0002-9904-1970-12396-5
[6] J. R. Rice, “The Degree of Convergence for Entire Func-
tions,” Duke Mathematical Journal, Vol. 38, No. 3, 1971,
pp. 429-440. doi:10.1215/S0012-7094-71-03852-X
[7] O. P. Juneja, “On the Coefficients of Entire Series,”
Journal of Mathematical Analysis, Vol. 24, 1971, pp.
395-401.
[8] O. P. Juneja and G. P. Kapoor, “Polynomial Coefficients
of Entire Series,” Yokohama Mathematical Journal, Vol.
22, 1974, pp. 125-133.
[9] D. Kumar, “Approximation Error and Generalized Orders
of an Entire Function,” Tamsui Oxford Journal of
Mathematical Sciences, Vol. 25, No. 2, 2009, pp. 225-
235.
[10] D. Kumar and H. Kaur, “Lp—Approximation Error and
Generalized Growth Parameters of Analytic Functions in
Coratheodory Domains,” International Journal of Mathe-
matical Analysis, Vol. 3, No. 30, 2009, pp. 1461-1472.