M. N. BAGDE ET AL.245
the stresses, which they experienced earlier. The best
studied memory effects are the memory effect in AE,
known as Kaiser Effect, and the deformation memory
effects, or memory effects in strain, which make a phy-
sical basis of the Deformation Rate Analysis (DRA)
stress measurement method. Both kinds of effects take
place while the rock is cyclically loaded to stress levels,
increasing from cycle to cycle [15]. Both deformation
and acoustic emission memory effects are due to the de-
velopment of irreversible micro-fractures in rock sub-
jected to cyclic loading [9,18]. According to Filimonov
et al., [15] this leads to the absence of crack growth and
sliding processes (dislocation movements) at stress val-
ues smaller than the maximum previously applied stress.
As soon as this “memorized” stress value is attained,
crack propagation is again initiated, which is accompa-
nied with AE pulses and non-linear inelastic strain de-
velopment. Memory effects are related to irreversible,
stress-induced changes in the rock’s structure occurring
in the first cycle loading. Therefore, the elastic limit pre-
sents a natural threshold for memory formation. This is
confirmed by the tests in which the first stage cycle axial
stress was smaller than the elastic limit for the given
loading condition. In second stage cycle stress and defor-
mation of such specimen were similar to those of “fresh”
specimen, which did not undergo any first cycle loading.
This was due to the absence of micro-fracture damage
below the elastic limit, which could form a stress mem-
ory. Distinct memory effects in the third stage cycle took
place where the maximum axial stress exceeded the elas-
tic limit took place in the second stage cycle. From the
Figure 7, it can be seen that in final stage of cycling and
loading, rock has followed the same path experienced in
stage II and III loading path. A more detailed study of
the stress memory effect under dynamic cyclic loading
could be carried out in the future.
According to Smith et al., [19] often cyclic loads are
not repetitive, i.e. cyclic stress or deformation does not
have constant amplitude or frequency. According to
them it is necessary therefore to know how irregularity in
loading sequences affects accumulation of damage. Here
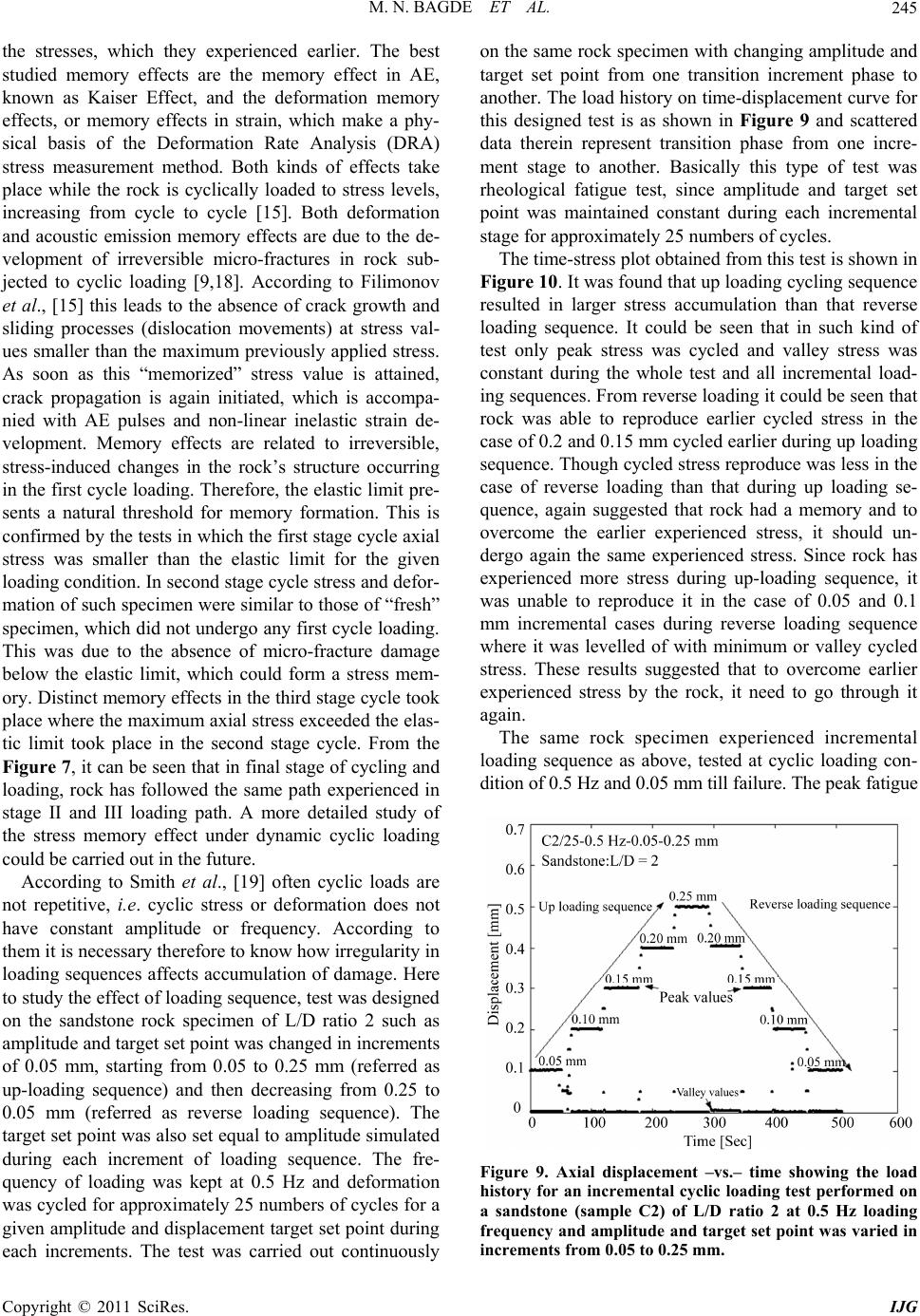
to study the effect of loading sequence, test was designed
on the sandstone rock specimen of L/D ratio 2 such as
amplitude and target set point was changed in increments
of 0.05 mm, starting from 0.05 to 0.25 mm (referred as
up-loading sequence) and then decreasing from 0.25 to
0.05 mm (referred as reverse loading sequence). The
target set point was also set equal to amplitude simulated
during each increment of loading sequence. The fre-
quency of loading was kept at 0.5 Hz and deformation
was cycled for approximately 25 numbers of cycles for a
given amplitude and displacement target set point during
each increments. The test was carried out continuously
on the same rock specimen with changing amplitude and
target set point from one transition increment phase to
another. The load history on time-displacement curve for
this designed test is as shown in Figure 9 and scattered
data therein represent transition phase from one incre-
ment stage to another. Basically this type of test was
rheological fatigue test, since amplitude and target set
point was maintained constant during each incremental
stage for approximately 25 numbers of cycles.
The time-stress plot obtained from this test is shown in
Figure 10. It was found that up loading cycling sequence
resulted in larger stress accumulation than that reverse
loading sequence. It could be seen that in such kind of
test only peak stress was cycled and valley stress was
constant during the whole test and all incremental load-
ing sequences. From reverse loading it could be seen that
rock was able to reproduce earlier cycled stress in the
case of 0.2 and 0.15 mm cycled earlier during up loading
sequence. Though cycled stress reproduce was less in the
case of reverse loading than that during up loading se-
quence, again suggested that rock had a memory and to
overcome the earlier experienced stress, it should un-
dergo again the same experienced stress. Since rock has
experienced more stress during up-loading sequence, it
was unable to reproduce it in the case of 0.05 and 0.1
mm incremental cases during reverse loading sequence
where it was levelled of with minimum or valley cycled
stress. These results suggested that to overcome earlier
experienced stress by the rock, it need to go through it
again.
The same rock specimen experienced incremental
loading sequence as above, tested at cyclic loading con-
dition of 0.5 Hz and 0.05 mm till failure. The peak fatigue
Figure 9. Axial displacement –vs.– time showing the load
history for an incremental cyclic loading test performed on
a sandstone (sample C2) of L/D ratio 2 at 0.5 Hz loading
frequency and amplitude and target set point was varied in
increments from 0.05 to 0.25 mm.
Copyright © 2011 SciRes. IJG