Journal of Service Science and Management
Vol.6 No.1(2013), Article ID:28952,13 pages DOI:10.4236/jssm.2013.61007
Measuring Service Value Based on Service Semantics
![]()
School of Computer Science and Technology, Harbin Institute of Technology, Harbin, China.
Email: *machao8396@163.com, rainy@hit.edu.cn, xiaofei@hit.edu.cn, wqian0725@163.com
Received November 5th, 2012; revised December 8th, 2012; accepted December 22nd, 2012
Keywords: Service Value; Value Measurement; Value Dependency; Uncertainty; Service Element
ABSTRACT
Service participants can obtain different service values through participating in multiple service solutions with the same function but different performances and these solutions are usually represented as the pre-designed service models. Whether or to what degree the service values can be implemented under the support of the pre-designed service models is as a critical criterion for evaluating and selecting the most appropriate one from these service solutions. Therefore an approach of service value measurement based on service semantics (i.e. meaning of service models) is presented in this paper. Starting from a definition of service value, we present a series of concepts (e.g. value indicators, value profit constraints, etc.) and measure them based on the pre-designed service models. This paper also defines the value dependency relationships among the corresponding service values due to the uncertainty of relationships between multiple quality parameters of service elements, and then analyzes the impact of the value dependency on service value measurement. In order to complement the discussions above, a real-world case study from ocean transportation service is conducted for demonstration.
1. Introduction
With the rapid development of modern service industry, the market competition has become increasingly fierce. In order to obtain larger value persistently, service providers need to constantly provide better service for customers through service innovation. So service providers need to evaluate the profitability of the innovative service. On the other hand, with more and more services available, customers can obtain various services with different values of the same function. So customers need to select the most appropriate service from multiple existing services measured by service value.
For instance, ocean transportation service is a typical IT-enabled modern service, and it includes some business scenarios such as cabin booking, land transportation, customs inspection, etc. The business scenario of cabin booking is taken as an example to explore the above issues. In this business scenario, the ship company can directly provide cabin booking service for the consigners. But this business is not belonging to the core business scope of the ship company. In order to decrease in its cost and pay more attention to its core business, it can outsource this business to the forwarders, and ask the forwarders to assist it to provide cabin booking service for the consigners. So the ship company needs to evaluate whether the latter service solution can really bring him larger value than the former one. On the other hand, one consigner wants to book a cabin, he can directly send a request to the ship company, and can also send a request to the forwarders, asking the forwarders to help him to book the cabin. So the consigner needs to evaluate which service solution is better by comparing the values that the two solutions bring him. In summary, the consigners and the ship company all face the problems of which service solution is best.
Customers and service providers all expect to obtain largest value during the participation in service. In order to obtain largest value, customers need to find out the most appropriate service solution among multiple existing services solutions with the same function but different performances by comparing the values that these service solutions bring them. In order to obtain largest value, service providers need to constantly provide innovative and more attractive service solution for customers. And then service providers need to evaluate whether the innovative service solution can really bring them larger value than the service solutions that already exist. Therefore, customers and service providers all need a method to measure the values brought through the execution of service solution.
So what is value actually? Some researchers think: for customers, value is whether or to what degree their requirements are met by services or goods, and for service providers, value is whether or to what degree their utility (or profit) is brought by delivering services or goods to customers [1]. Some other researchers mainly focus on customer value and believe that customer value is the price that customers are willing to pay for delivered services or goods [2].
The above concepts of value are all used to explore the relationship between value and service business activity at the macro level. But in this paper, the concept of value is supposed to explore the relationship between value and service activity at the operational level, because that customers need to decide which service solution (represented as the service process model that consists of service activities and their corresponding physical resources and information at the operational level) should be chose by comparing the values obtained from the service solutions, and service providers need to decide which service solution (represented as the service process model at the operational level) should be used to provide service for customers by comparing the values brought through service delivery.
Therefore, a service value’s intuitive definition is given here. The state or degree of specific aspects of a participant (customer or service provider) will be improved after the execution of service, and the profit or utility that such improvement brings to him is defined as the service value. More specifically, there are two types of service values: the first refers to the values generated by transferring specific “things” (e.g., information, a product, money, or the right to use resources) from value producers to value receivers; the second refers to the values generated by improving certain states (e.g., physical states, spiritual states, physical states of possessions) of value receivers.
Moreover, any state improvement or any thing transfer is implemented under the support of various service elements (e.g., service activities, physical resources, or information) in service models [3] . So whether or to what degree service values can be implemented depends on the pre-designed service model related to the existing service solution. If one pre-designed service model enables and supports service values implementation most sufficiently, the corresponding service solution should be chose by customers (or service providers). Therefore, a method of service value measurement based on service semantics (i.e. meaning of service model) is presented in this paper.
Many researchers have begun to pay attention to service value measurement. According to different usages of measurement results, the several common kinds of measurement methods are as follow:
• For economic investment Value only refers to benefits. Value is defined as a value structure that consists of several value factors in the first layer and there are several relevant measures in the second layers for each value factors. An automated Analytical Hierarchy Process (AHP)-based tool can enable the entire process to be achieved [4].
• For service business analysis Some researchers measure the values that service system generates, taking into account the economic values [5]. The values are calculated according to the exchange of offerings (goods, services) and the participants’ satisfaction.
Some other researchers think that the values are generated not only by exchange of goods, services, or revenue, but also by the exchange of knowledge and intangible benefits [6-8]. They qualitatively measured the values of various participants according to the exchange of goods, services, revenue, knowledge or intangible benefits.
In general, the above two kinds of researchers use measurement results of values for analyzing, evaluating and optimizing service business at the macro level.
• For innovative service idea evaluation In this kind of method, some researchers present an e3-value model (i.e. a business model) to describe how economic values are created and exchanged in an innovative service idea [9-12]. According to the number of exchanged value objects (e.g., products, services, revenue and experience, etc.) between the various participants in e3-value model and the economic values generated by each value object exchange, the values of each participant are calculated, and then a profit table is built to reflect the potential profit of participants.
Our approach to measure service values is based on the meaning of service models (refer to process model at the operational level, not business model at the macro level), because this paper mainly focuses on which predesigned service model enables and supports service values implementation most sufficiently. The approach combines the meaning of service models with service values to calculate service values and to enable the measurement result of service value to reflect whether or to what degree service values is implemented under the support of service models.
In addition, the relationships between quality parameters of multiple service elements may be uncertain, which may result in the value dependency relationships between the corresponding service values. Therefore, the method of service value measurement not only needs to explore how to measure an independent value, but also take into consideration the impact that the value dependency has on service value measurement.
For an independent value, firstly, the quality parameters belonging to its Value Profit Constraint are calculated based on the meaning of the pre-designed service model, secondly, the influences (produced by the gaps between the realized quality parameters and those constraints) are calculated by utilizing some typical functions, and then the influences are mapped to the initial values of Value Indicators. Finally, the realized service value can be obtained according to the realized Value Indicators. For a non-independent value, the calculation of the quality parameters belonging to its Value Profit Constraint is related to the dependency function (that is introduced to measure the influences of other values on the non-independent value). The influences should be taken into consideration in the whole measurement process of the non-independent service value.
This paper is organized as follows. Section 2 introduces some basic concepts of service semantics and service value. Section 3 presents an approach of the independent value measurement. And the approach of the non-independent value measurement is explored in Section 4. Section 5 shows a simple case study. Section 6 closes with a conclusion.
2. Service Models and Service Value
2.1. Service Models
Semantics is the meaning of models (i.e. meaning of the systems represented by a set of logical components), such as activity, state, attribute, etc. [13] . So the concept “service semantics” refers to the meaning of service models. The pre-designed service model is the basis of service value measurement. Therefore, it is necessary to explore which kind of service model specification should be selected to represent service solution and what service semantics should include.
Business Process Modeling Notation (BPMN) is the service model specification that is generally adopted to represent service solution. Comparing with other service models (e.g. Unified Modeling Language, Service Model Driven Architecture, etc.), BPMN provides the service elements that are more complete and more suitable to describe service business process of service solution, and can be directly supported by executable Business Process Execution Language for Web Services. Therefore, BPMN is selected BPMN model, like other service models, consists of service elements and their relationships. In BPMN model, the service elements that directly affect service value measurement include service activities, their information and physical resources. The corresponding graphic modeling construct of them is Activity, Data Object and a new artifact (defined by service model designers for modeling physical resources) respectively.
In BPMN, Activity can represent task and sub-process. Sub-process is a set of tasks which are inter-connected. In order to support service value measurement, the attributes of task should include:
• the set of participants who are concerned with task;
• the set of action objects which are manipulated by task and their states are changed by the effect of task;
• the set of action objects’ state transitions;
• the set of resources that support task’s execution to realize state transitions of action objects;
• the set of quality parameters that are attached to task to measure its execution performance.
For the other graphic modeling constructs, their attributes should uniformly include: the resource name, the resource classification (including physical resources and information resources), and the set of quality parameters attached to the resources. The quality parameter set of task and resource are all used to measure some characteristics of service elements, so they are uniformly called quality of service (QoS).
For the above attributes, only QoS is used to directly calculate service values, the other attributes enables and supports value annotation approach [14,15] for identifying the corresponding relationships between service elements and service values. These corresponding relationships are the basis of service value measurement. Therefore, all the above attributes should be expressed by service model designers in BPMN model.
In addition, the quality parameters of service elements and the relationships between quality parameters of multiple service elements may be uncertain. The uncertainty of quality parameters can be described by probability distribution of discrete random variable, and then the uncertainty of relationships can be described by a set of conditional probability.
It is assumed that there are two service elements sei and sej, whose quality parameters are uncertain. The uncertainty of a sei’s quality parameter qx can be described by the probability distribution for discrete random variable A, and A represent the value of quality parameter sei·qx. All the possible values of A is , ak is a range of value of quality parameter
, ak is a range of value of quality parameter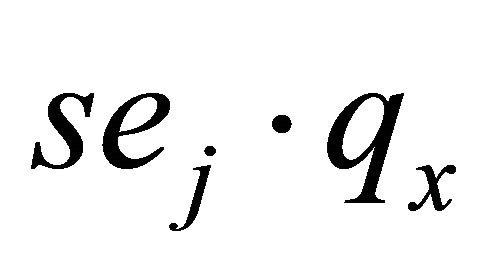 . The uncertainty of sei·qx can be denoted by
. The uncertainty of sei·qx can be denoted by ,
,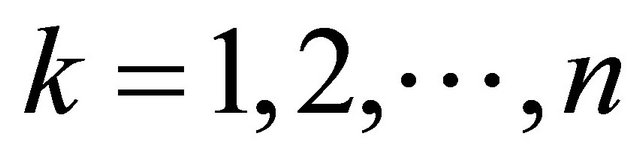 . The expression of uncertainty of
. The expression of uncertainty of 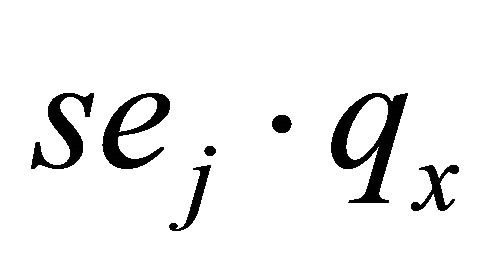 is similar to one of
is similar to one of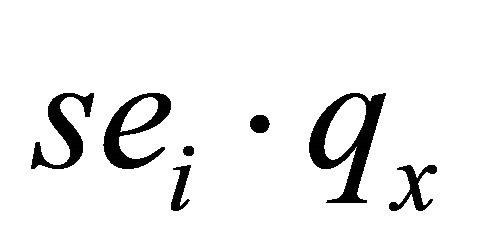 , and it can be denoted by P
, and it can be denoted by P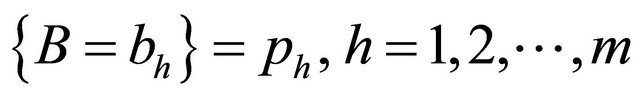 . Therefore, the uncertainty of the relationship between
. Therefore, the uncertainty of the relationship between 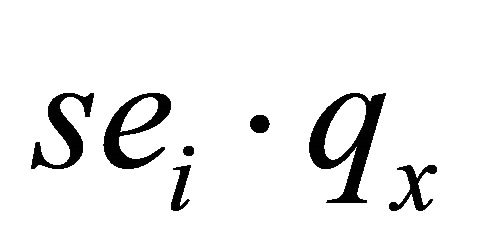 and
and  can be denoted by P(Bh|Ak)
can be denoted by P(Bh|Ak)  where Ak is the event “A = ak”, and Bh is the event “B = bh”.
where Ak is the event “A = ak”, and Bh is the event “B = bh”.
The uncertainty of relationships between quality parameters of multiple service elements can result in value dependency relationships between the corresponding service values. And value dependency relationships can affect service value measurement. Therefore, the uncertainty of relationships is useful for service value measurement and also should be expressed in BPMN model.
Service semantics is introduced to explain the known condition of service value measurement. The above service elements and their attributes, especially QoS and uncertainty, are taken as the known condition of service value measurement.
2.2. Service Value
In our definition, service value (i.e. economic profit) is defined as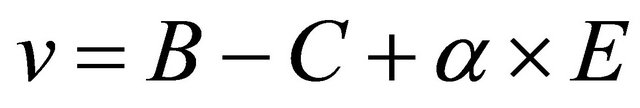 , where:
, where:
• B – C is the direct economic profit that value’s receiver obtains, B is the direct benefit, and C is the direct cost.
• a ´ E is the contribution made by an indirect profit to the direct profit, E is the indirect economic profits that value’s receiver obtain, a is the influence coefficient that is used to measure the influence of E on B – C.
Service value v is mainly affected by B, C and E. They are uniformly called Value Indicator. Moreover, according to the definition of service value, the Value Indicator is affected by the state improvement or degree improvement of the specific aspects of value’s receiver. Therefore, Value Profit Constraint (CON) is introduced to measure the state improvement and the degree improvement. CON is a set of quality parameters.
The specific aspect of value’s receiver is called Value Realization Carrier (rc). The state (or degree) improvement is represented as the rc’s state transition which is the transformation from the rc’s initial state to its expected final state. This state transition brings economic profit “B – C + a ´ E” to service value receiver.
A quality parameter of CON is used to measure a specific characteristic of the rc’s state transition. In the above concepts, CON is used to directly calculate service values, other concepts (rc, initial state, expected final state and the state transition) are used to support value annotation approach.
Referring to the quality parameters that are used to measure services in the reference [16], CON also include five dimensions: Time/Efficiency, Price/Cost, Service Content, Resource/Condition and Risk/Credit. There are several relevant quality parameters for each dimension. The quality parameters are chose according to the actual application domain.
Any state transition of rc is implemented under the support of various service elements. So the quality parameters of CON rely on the quality parameters of corresponding service elements in BPMN model.
As shown in Figure 1, service value v is mainly dependent on B, C and E. The Value Indicators are affected by CON, which can be calculated by utilizing the function fC, fB, and fE. The quality parameters of CON should be calculated according to the corresponding service elements’ QoS by utilizing the function G. At last, service value v affects the satisfaction degree of v’s receiver, which can be calculated by utilizing the function H. By this way, the service value can be very well combined together with the QoS of service elements, to support service value measurement based on service semantics.
Moreover, according to different roles of v’s receiver and different kinds of service interaction, the meaning of Value Indicators is different. There are two types of role in service interaction: customers and service providers. There are two kinds of service interaction, as shown by Figure 2. In the first hind, service providers provide service for customers and charge customers for certain reasonable fees. In the second kind, service providers provide free service for customers C1 and obtain some “utility”, and service providers provide service for customers C2 by utilizing the “utility” and charge customers C2 for certain reasonable fees. In the service interaction between service providers and customers C1, service provider only can obtain the indirect profit which is the economic profit may be transformed from the “utility” in the future during the service interaction between service providers and customers C2 occurring.
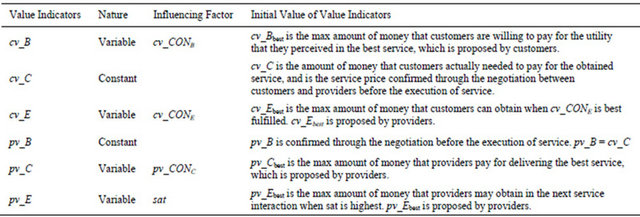
For the first kind of service interaction, the meaning of Value Indicators is given as follows. For customers, the value that he receives is customer value (represented as cv). The meaning of cv’ Value Indicators is given in Table 1. For service providers, the value that he receives is provider value (represented as pv). The meaning of pv’ Value Indicators is also given in Table 1.
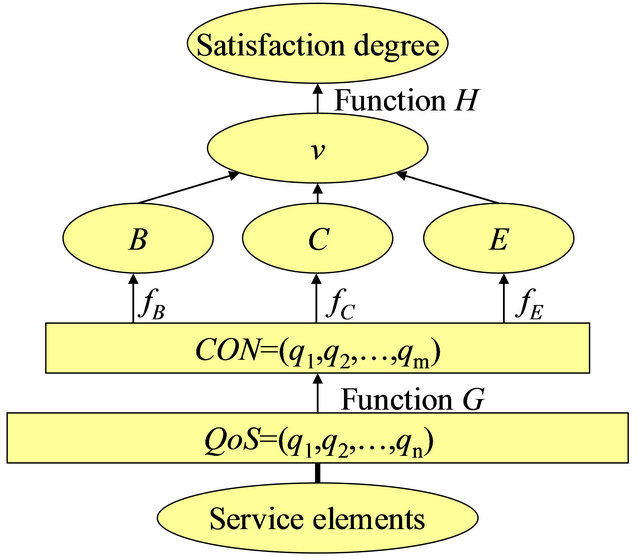
Figure 1. The computation structure of service value.
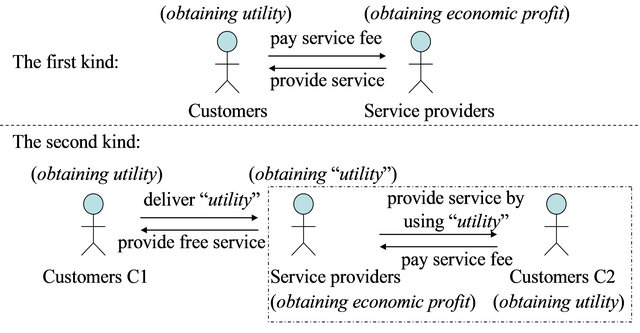
Figure 2. Two kind of service interaction.
Table 1. The detailed information of six kinds of Value Indicators in the first kind of service interaction.
For the second kind of service interaction, the meaning of Value Indicators is given as follows. For customers, the meaning of cv_B and cv_C is the same as their meanings in the first kind of service interaction. But because the service obtained by customers C1 is free, cv_C equals 0 and cv_E no longer exists.
For providers, the meaning of pv_B and pv_C is also the same as their meanings in the first kind of service interaction, especially pv_B equals 0. But the meaning of pv_E is different from its meaning in the first kind of service interaction. pv_E is the amount of money that service providers may obtain in the future by providing service for customer C2 by using the “utility” that is obtained from customer C1 in the current service interaction. It is affected by the value profit constraint pv_CONE proposed by providers. The quality parameters of pv_CONE are used to measure some specific characteristic of customers’ behavior. The initial value of pv_E is represented as pv_Ebest. pv_Ebest is the max amount of money that providers may obtain by delivering the “utility” in the future when pv_CONE is best fulfilled. pv_Ebest is proposed by providers.
2.3. Value Dependency Relationship
Service value does not exist independently. There could be value dependency relationship among multiple service values. Value dependency relationship is defined as the implementation degree of a service value is completely or partially dependent on one of the others, which is denoted as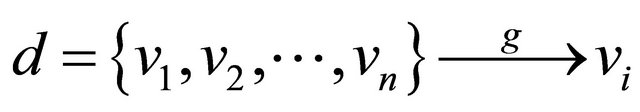 . Obviously, value dependency relationship can affect the service value measurement. Dependency function g is used to measure the impact of
. Obviously, value dependency relationship can affect the service value measurement. Dependency function g is used to measure the impact of  on
on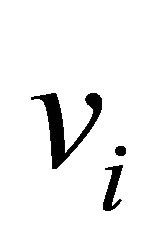 . As mentioned above, the value dependency relationship among multiple service values are caused by the uncertainty of relationships between various quality parameters of the corresponding service elements.
. As mentioned above, the value dependency relationship among multiple service values are caused by the uncertainty of relationships between various quality parameters of the corresponding service elements.
As shown in Figure 3, there are two service values vi and vj, and vj is affected by vi. The value dependency relationship is denoted as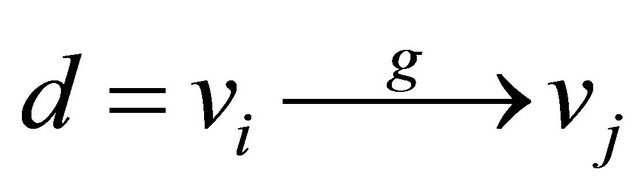 , where g is used to measure the impact of vi on vj. The impact is actually caused by the uncertainty of relationships between the quality parameters of sej related to vj and the quality parameters of sei related to vi. A quality parameter of sei directly affects the corresponding one of sej, and can indirectly affect the corresponding quality parameters of vj’CON. And then it continues to indirectly affect vj’ value indicators, at last indirectly affect vj.
, where g is used to measure the impact of vi on vj. The impact is actually caused by the uncertainty of relationships between the quality parameters of sej related to vj and the quality parameters of sei related to vi. A quality parameter of sei directly affects the corresponding one of sej, and can indirectly affect the corresponding quality parameters of vj’CON. And then it continues to indirectly affect vj’ value indicators, at last indirectly affect vj.
Value dependency relationship may be caused by one or more quality parameters of service elements. For one, its dependency function is denoted as g = P(Bh|Ak) (for ) which represent the uncertainty of relationships between sej·qx and sei·qx (as mentioned in Section 2.1). For more quality parameters, its dependency function is also represented as the set of conditional probability.
) which represent the uncertainty of relationships between sej·qx and sei·qx (as mentioned in Section 2.1). For more quality parameters, its dependency function is also represented as the set of conditional probability.
3. Measurement of Independent Service Value
Independent service value refers to the service value that is not dependent on other service values. Its implementation is not affected by the implementation of the others. It

Figure 3. The impact of value dependency relationship.
is measured only based on Qos of its corresponding service elements.
3.1. Independent Customer Value
In the first kind of service interaction, for cv, its cv_B is affected by cv_Bbest and cv_CONB, and its cv_E is affected by cv_Ebest and cv_CONE. Therefore, cv can be denoted as
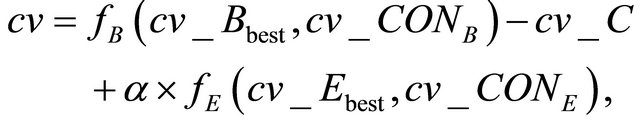
where the function fB is:
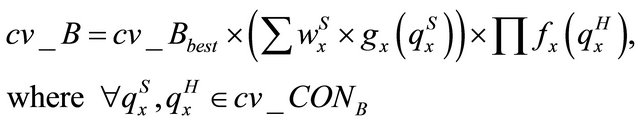 (1)
(1)
In the formula (1), 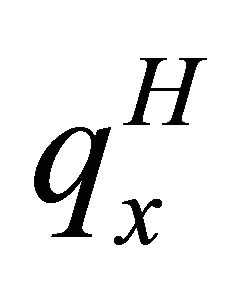 is the hard quality parameter, which means its expected constraint must be fulfilled. The function fx is used to measure whether
is the hard quality parameter, which means its expected constraint must be fulfilled. The function fx is used to measure whether ’s expected constraint can be fulfilled by the value of
’s expected constraint can be fulfilled by the value of . The proposition of
. The proposition of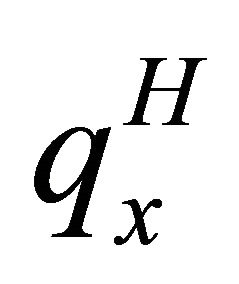 ’s expected constraint and the
’s expected constraint and the ’s calculation is as follow.
’s calculation is as follow.
The quality parameter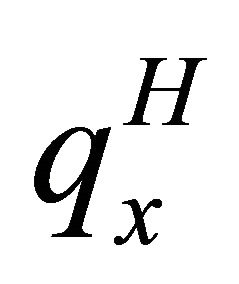 ’s constraint is proposed by customers, and its constraint range is divided into the expected range and the unacceptable range. There are two kinds of quality parameters: the first one is that the bigger the quality parameter is, the higher the quality of the corresponding service is; and the second one is that the smaller the quality parameter is, the higher the quality of the corresponding service is.
’s constraint is proposed by customers, and its constraint range is divided into the expected range and the unacceptable range. There are two kinds of quality parameters: the first one is that the bigger the quality parameter is, the higher the quality of the corresponding service is; and the second one is that the smaller the quality parameter is, the higher the quality of the corresponding service is.
Taking  that belongs to the first kind as an example, its expected range can be denoted as
that belongs to the first kind as an example, its expected range can be denoted as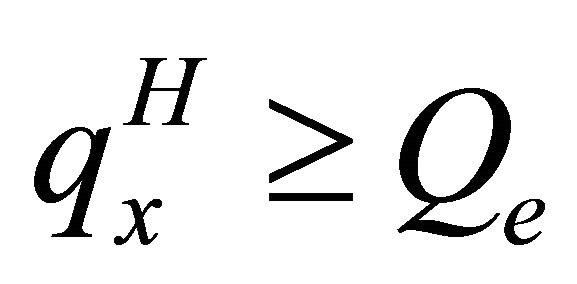 , and
, and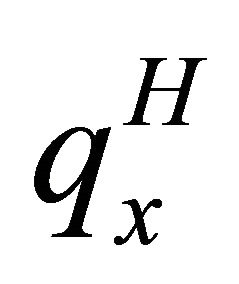 ’s unacceptable range is denoted as
’s unacceptable range is denoted as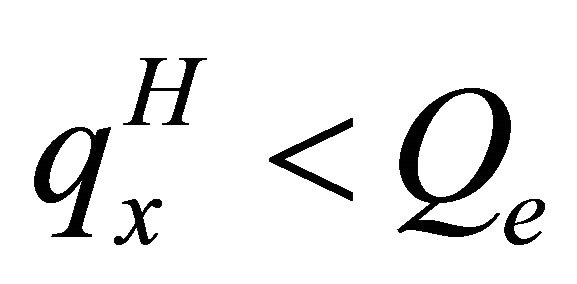 , where Qe is the smallest one in all the acceptable values of
, where Qe is the smallest one in all the acceptable values of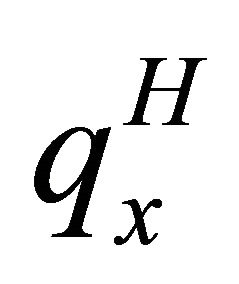 . For
. For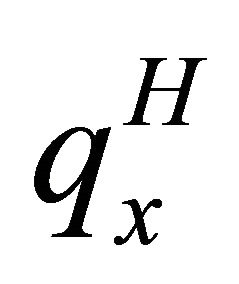 , any values that do not belong to the expected range can not be acceptable.
, any values that do not belong to the expected range can not be acceptable.
The quality parameter 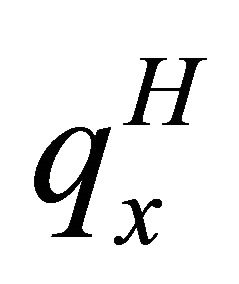 is calculated based on the QoS of a service element (or a set of service elements). If
is calculated based on the QoS of a service element (or a set of service elements). If 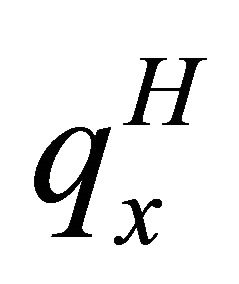 is related to a service element se, then
is related to a service element se, then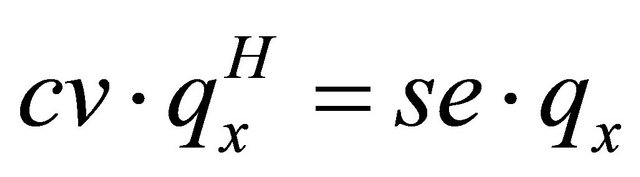 . If
. If 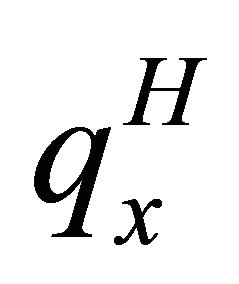 is related to a set of service elements
is related to a set of service elements , then
, then
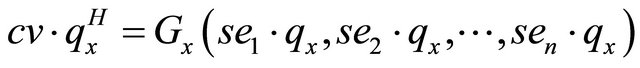 where according to different
where according to different 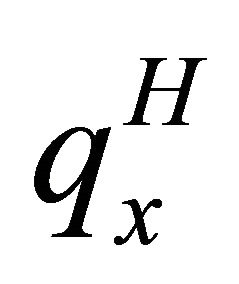 and the different sequence relationships between various service elements, the function Gx may be one of the simple operations (e.g. P, å, min, max etc.).
and the different sequence relationships between various service elements, the function Gx may be one of the simple operations (e.g. P, å, min, max etc.).
In order to express the meanings of  in the formula (1), the range of
in the formula (1), the range of 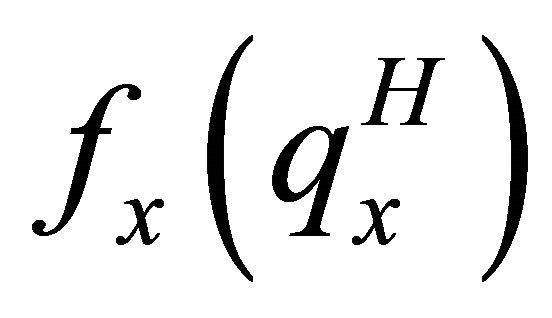 is defined as {0, 1}, and the corresponding weight of
is defined as {0, 1}, and the corresponding weight of  is defined as 1. It is assumed that the range of
is defined as 1. It is assumed that the range of  can be denoted as [min, max], then the function fx is:
can be denoted as [min, max], then the function fx is:
• For  belonging to the first kind, the function fx is
belonging to the first kind, the function fx is .
.
• For 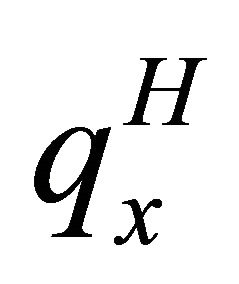 belonging to the second kind, the function fx is
belonging to the second kind, the function fx is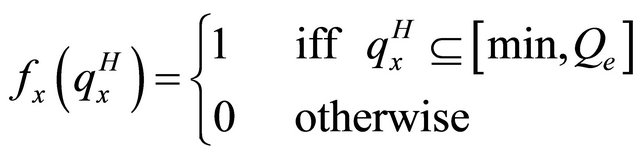 .
.
If the value of 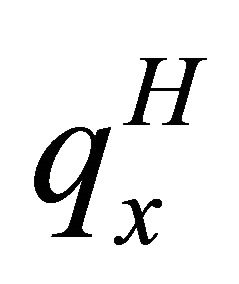 belongs to the expected range,
belongs to the expected range, 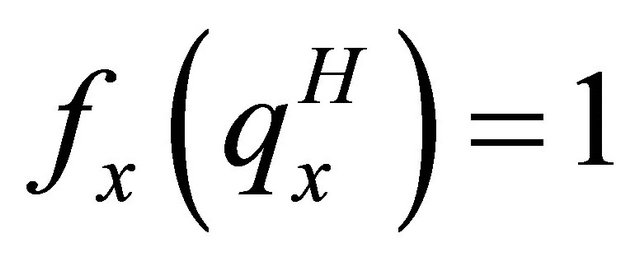 , or else
, or else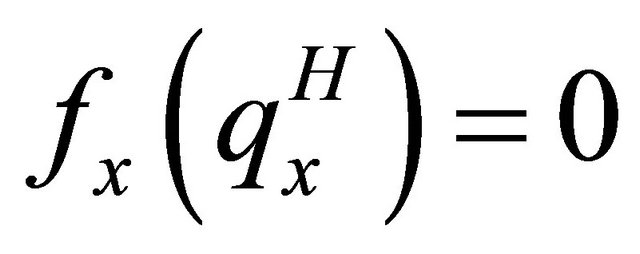 . When
. When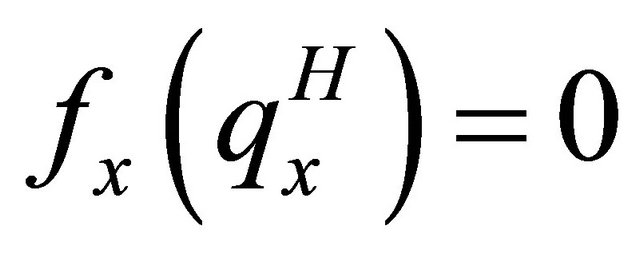 , the weight of
, the weight of  is 1, then cv_B = 0, which means that when the value of
is 1, then cv_B = 0, which means that when the value of  is not belonging to the expected range the value’s receiver will not be willing to pay any money.
is not belonging to the expected range the value’s receiver will not be willing to pay any money.
In the formula (1), the part “ ” is similar to the formula to calculate the overall quality proposed by the reference [17]. The formula to calculate the overall quality is firstly to calculate the gaps between expectations of various quality parameters and their perceptions, and then, each gap multiplies with its weighting, and finally the result of the overall quality is obtained. The function
” is similar to the formula to calculate the overall quality proposed by the reference [17]. The formula to calculate the overall quality is firstly to calculate the gaps between expectations of various quality parameters and their perceptions, and then, each gap multiplies with its weighting, and finally the result of the overall quality is obtained. The function  is also related to the gaps between expectations of various quality parameters
is also related to the gaps between expectations of various quality parameters 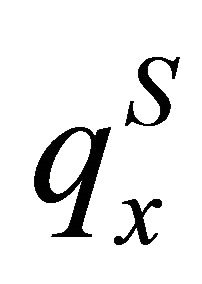 and their perceptions. But the objective of the function
and their perceptions. But the objective of the function 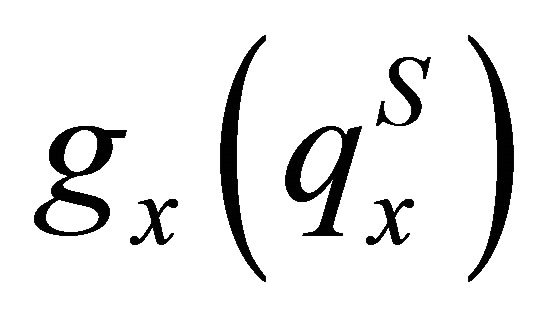 is not to simply calculate the gaps but to measure the impact of the gaps on cv_Bbest. And then, each impact degree multiplies with its weighting
is not to simply calculate the gaps but to measure the impact of the gaps on cv_Bbest. And then, each impact degree multiplies with its weighting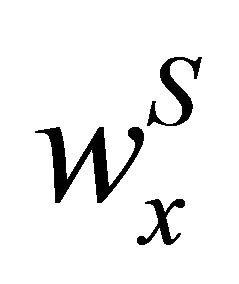 , and finally the result of cv_B is obtained. In this paper, the expectations of quality parameters are represented by the expected constraints that are proposed by value receivers, and the perceptions of quality parameters are represented by the values of quality parameters that are designed by service model designers.
, and finally the result of cv_B is obtained. In this paper, the expectations of quality parameters are represented by the expected constraints that are proposed by value receivers, and the perceptions of quality parameters are represented by the values of quality parameters that are designed by service model designers.
In formulas (1), 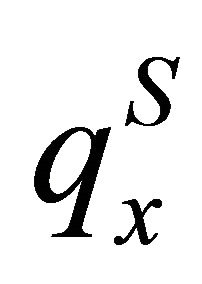 is the soft quality parameter that means whether or to what degree its constraint is fulfilled is not strictly required. The function gx is used to measure whether or to what degree
is the soft quality parameter that means whether or to what degree its constraint is fulfilled is not strictly required. The function gx is used to measure whether or to what degree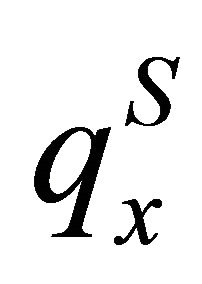 ’s expected constraint is fulfilled by the value of
’s expected constraint is fulfilled by the value of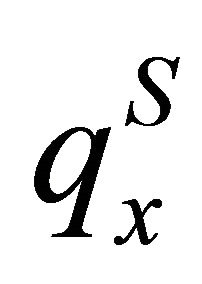 .
. 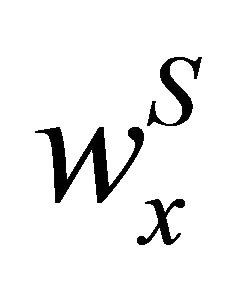 is the weight of
is the weight of
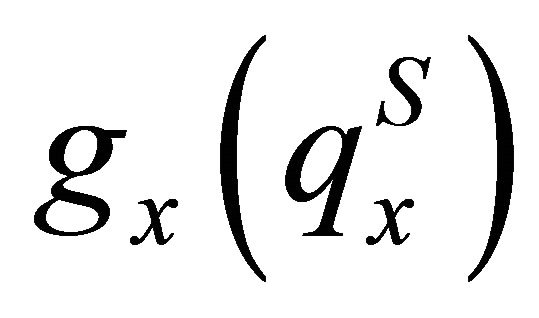 , and
, and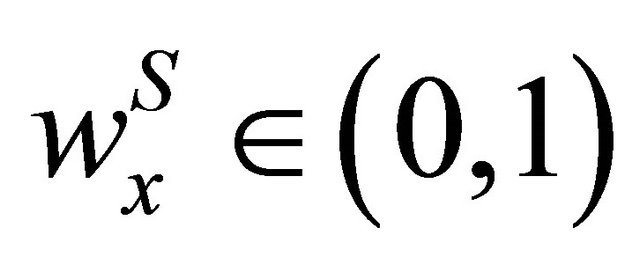 . According to the importance of
. According to the importance of  to cv_B,
to cv_B, 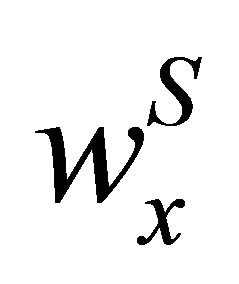 can be assigned by the experts in correlative domains.
can be assigned by the experts in correlative domains.
Similar to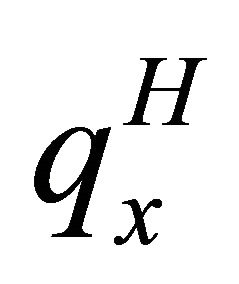 ,
, 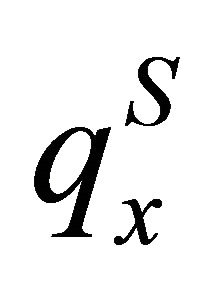 is calculated based on the QoS of a service element (or a set of service elements), which is denoted as
is calculated based on the QoS of a service element (or a set of service elements), which is denoted as  or
or
 .
.
The quality parameter ’s constraint is proposed by customers, and its constraint range is divided into the expected range, the acceptable range and the unacceptable range. As the space is limited, taking
’s constraint is proposed by customers, and its constraint range is divided into the expected range, the acceptable range and the unacceptable range. As the space is limited, taking 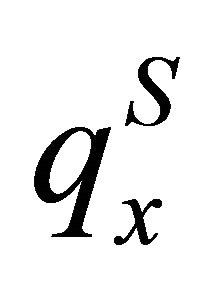 belonging to the first kind as an example, the proposition of
belonging to the first kind as an example, the proposition of ’s constraint and some typical formulas of gx are given as follow.
’s constraint and some typical formulas of gx are given as follow.
For  belonging to the first kind, the expected range of
belonging to the first kind, the expected range of 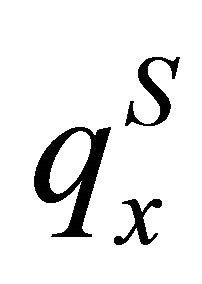 is denoted as
is denoted as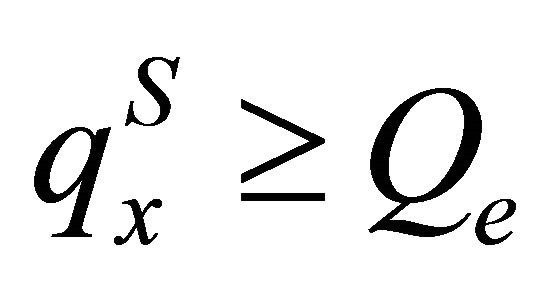 , the unacceptable range of
, the unacceptable range of 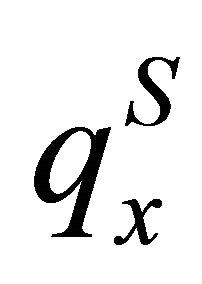 is denoted as
is denoted as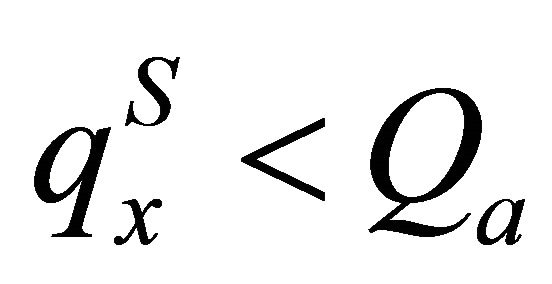 , and the acceptable range of qxS is denoted as
, and the acceptable range of qxS is denoted as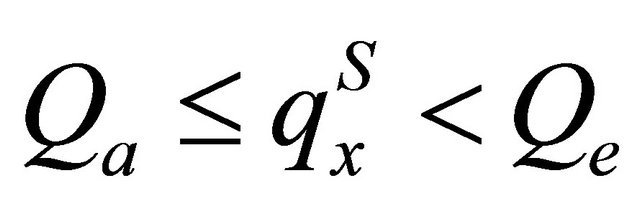 , where Qe is the smallest one in all the expected values, and Qa the smallest one in all the acceptable values.
, where Qe is the smallest one in all the expected values, and Qa the smallest one in all the acceptable values.
The range of  is [0,1]. For
is [0,1]. For 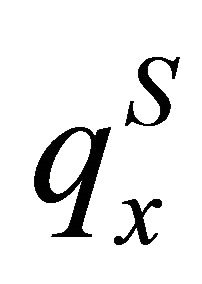 belonging to the first kind, some typical formulas and their images of the function gx is given as follow.
belonging to the first kind, some typical formulas and their images of the function gx is given as follow.
In Figure 4, the formula (a) is applicable to the case, where the impact of ’s change on cv_B is linear. The formula (b) is applicable to the case, where the impact of
’s change on cv_B is linear. The formula (b) is applicable to the case, where the impact of ’s change on cv_B is nonlinear and segmented. In the formula (c), 0 < a < 1, b > 0, it is applicable to the case, where the impact of
’s change on cv_B is nonlinear and segmented. In the formula (c), 0 < a < 1, b > 0, it is applicable to the case, where the impact of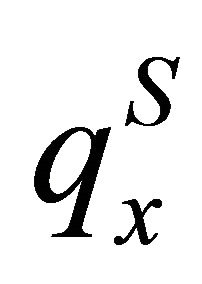 ’s change on cv_B is nonlinear and coincides with the law of marginal effect.
’s change on cv_B is nonlinear and coincides with the law of marginal effect.
The approach for measuring cv_E is similar to cv_B measurement, and the only difference is that the constraints of the quality parameters of cv_CONE and cv_Ebest are proposed by providers.
For the second kind of service interaction, as mentioned above, for value cv, its value indicator cv_B is affected by cv_Bbest and cv_CONB, its value indicator cv_C is 0, and value indicator cv_E does not exist. Therefore, cv can is denoted as cv = fB(cv_Bbest, cv_CONB), where the function fB is the same as the function fB which is used in the first kind of service interaction.
3.2. Independent Provider Value
In the first kind of service interaction, for pv, its pv_C is affected by pv_Cbest and pv_CONC, and its pv_E is affected by pv_Ebest and Sat. Therefore, pv can be denoted as
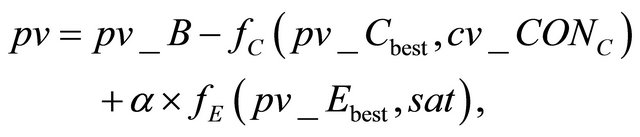
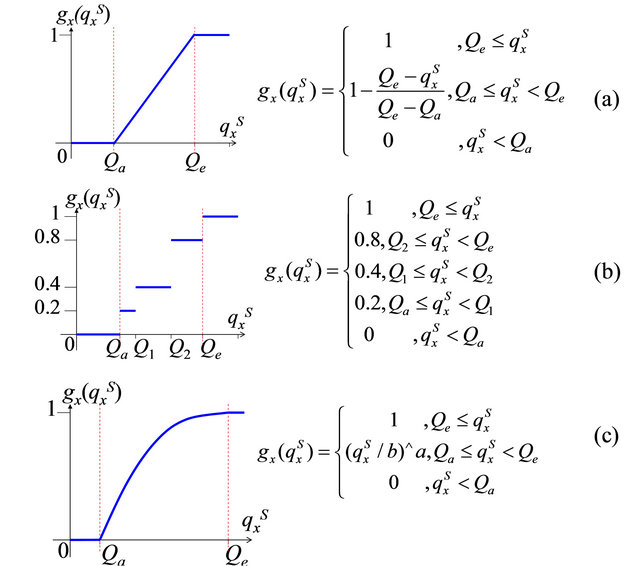
Figure 4. Some typical formulas of the function gx.
where the function fC is:
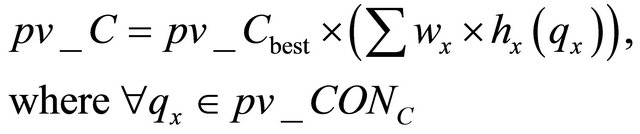 (2)
(2)
In formula (2), the function hx is used to measure the degree that the constraint of the quality parameter qx is fulfilled by the value of qx·wx is the weight of hx(qx), and wx Î (0,1). According to the importance of hx(qx) to pv_C, wx can be assigned by the correlative domain experts. The quality parameter qx is calculated based on the QoS of a service element (or a set of service elements), which is denoted as 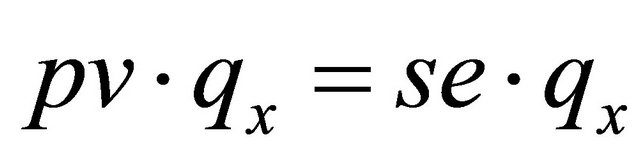 or
or
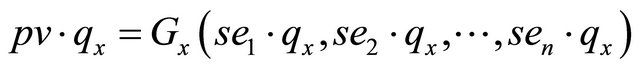 .
.
The quality parameter qx’s constraint is proposed by providers, and its constraint range is divided into the biggest cost range, the variable cost range and the smallest cost range. As the space is limited, taking qx belonging to the first kind as an example, the proposition of qx’s constraint and some typical formulas of the function hx are given as follow.
For qx belonging to the first kind, the biggest cost range of qx is denoted as qx ³ Qend, the smallest cost range of qx is denoted as qx < Qstart, and the variable cost range of qx is denoted as Qstart £ qx < Qend, where Qstart is the smallest value of qx which can cause the change of hx(qx) that represents the impact of qx’s change on pv_C, and Qend is the biggest value of qx which can cause the change of hx(qx). Qend is actually the smallest value of qx which service providers can provide by paying the biggest cost.
In actual services, the cost that providers pay is impossible to become infinitely smaller along with that the quality of the service that providers deliver to customers becomes infinitely lower. Therefore, the range of hx(qx) is defined as [b,1]. If all the quality parameters of pv_CONC belong to the smallest cost range, pv_Cmin = pv_Cbest ´ b. The cost pv_Cmin is the smallest value of pv_C. Some typical formulas and their images of the function hx are given as follow.
In Figure 5, the formula (a) is applicable to the case, where the impact of qx’s change on pv_C is linear. In the variable cost range, the change of qx makes the hx(qx) change, and the rate of change of hx(qx) is constant. The formula (b) is applicable to the case, where the impact of qx’s change on pv_C is nonlinear and segmented. In a subrange of the variable cost range, sometimes the change of qx can not make the hx(qx) change, and sometimes the tiny change of qx can cause the step change of hx(qx).
In the formula (c), 0 < a < 1, b > 0, it is applicable to the case, where the impact of qx’s change on pv_C is nonlinear and coincides with the law of marginal effect. In the variable cost range, the rate of change of hx(qx) is not constant. At the beginning of the change process of qx
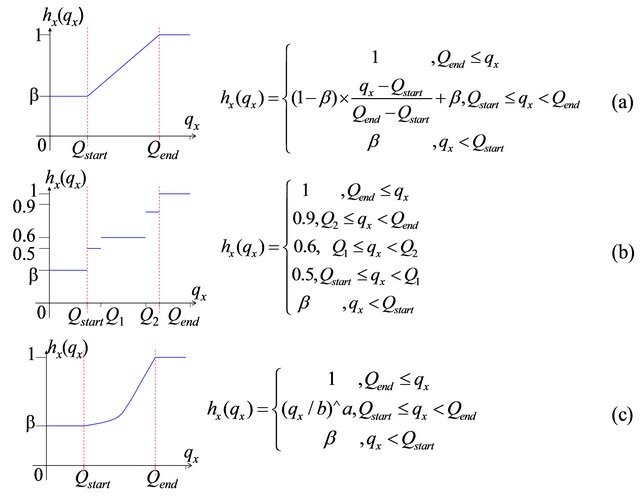
Figure 5. Some typical formulas of the function hx.
(from Qstart to Qend), the rate of change of hx(qx) is lower. And the rate of change becomes higher gradually with the increase of qx. Sometimes a combination of many kinds of formulas may also be selected for applying to some complicated service situation.
The function fE is used to measure the impact of sat on pv_E, which is denoted as fE(pv_Ebest, sat) = pv_Ebest ´ sat, where pv_Ebest is the max amount of money that providers may obtain in the next service interaction, and pv_Ebest may be assigned to a value by some forecasting method [18]; sat is enumerated type, and its range is the fuzzy set {very satisfied, satisfied, poorly satisfied, unsatisfied}. In order to support the measurement of pv_E, the fuzzy set of sat may be quantified using the set {1, 0.8, 0.4, 0}, where very satisfied corresponds to 1, satisfied corresponds to 0.8, and so on. As mentioned in the above Figure 2, sat is dependent on cv, which is denoted as sat = H(cv). The function H is used to measure the impact of the implementation degree of cv on sat. The formulas mentioned in Figure 6 may be adapted to instantiate the function H when Qe is defined as the smallest cv in all the cv that customers expect to obtain and Qa is defined as the smallest cv in all the cv that customers can accept.
For the second kind of service interaction, as mentioned above, for the value pv, its value indicator pv_B is 0, its value indicator pv_C is affected by pv_Cbest and pv_CONC, and its value indicator pv_E is affected by pv_Ebest and pv_CONE. Therefore, pv can be denoted as
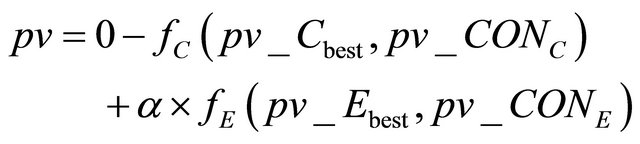
where the function fC is the same as the function fC which is used in the first kind of service interaction.
The function fE is different from the function fE which is used in the first kind of service interaction. The function fE is similar to the formula (1) in the Section 3.1
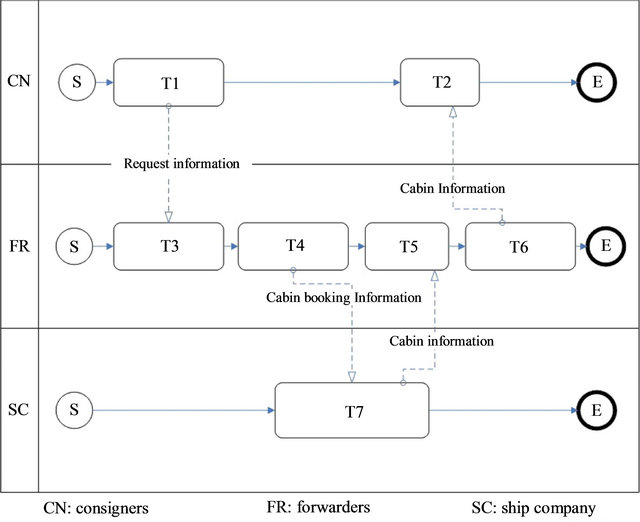
Figure 6. Service process model for the first solution.
which is used to calculate the function fB of cv in the first kind of service interaction. The difference is that the constraints of the quality parameters of pv_CONE and pv_Ebest are proposed by providers. The quality parameters of pv_CONE are used to measure some specific characteristic of customers’ behavior. In order to support the measurement of pv_E, some new quality parameters are need to be added into the Table 2 in the Section 2.2, for example, a quality parameter that is used to measure whether customers register to be free member, a quality parameter that is used to measure whether customers responded to the questionnaires after obtaining service, and so on.
4. Measurement of Non-Independent Service Value
Non-independent service value refers to the service value that is dependent on other service values. Its implementation of non-independent service value is completely or partially affected by one of the others. The dependency function g should be used to support the non-independent value measurement. For the first kind of service interaction or the second one, whether a non-independent value is cv or pv, its measurement process is similar to the one of an independent one. There is only one difference: the quality parameters of CON are calculated based not only on the QoS of the corresponding service elements but also on the corresponding dependency function g.
The dependency relationship
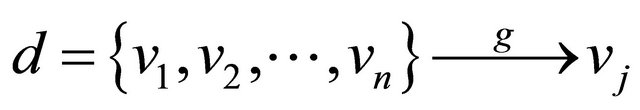
is caused by the uncertainty of relationship between the quality parameters of their corresponding service elements (sej is related to vj, and 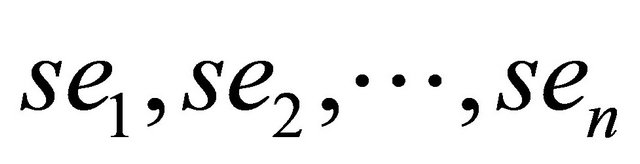 is related to
is related to 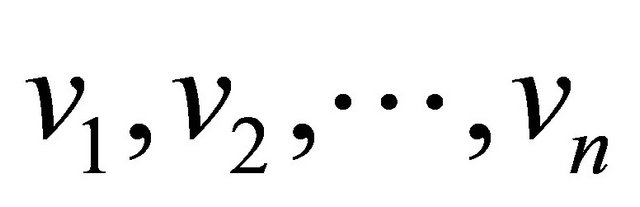 respectively). Therefore, if a quality parameter qx of vj. CON is affected by the uncertainty, the
respectively). Therefore, if a quality parameter qx of vj. CON is affected by the uncertainty, the
Table 2. The detailed information of service tasks.
RRT: Request response time; ET: Execution time; US: Usability; RE: Reliability; CA: Consumption amount.
measurement of qx refers to not only the quality parameter 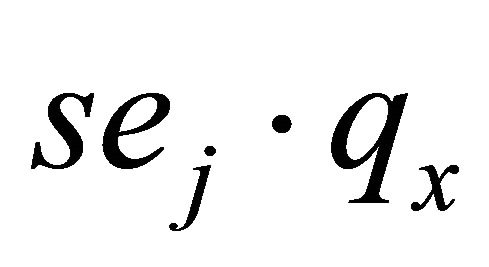 but also the quality parameter set
but also the quality parameter set
 .
.
And because the dependency function g is used to measure the uncer tainty of relationship between 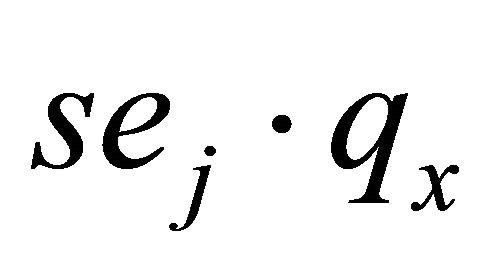 and
and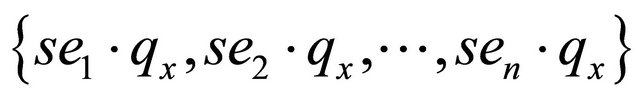 , the dependency function g should be taken into consideration while calculating the quality parameter qx of vj·CON. Because of the effect of the dependency function g, the value of the quality parameter qx may not be a single range, but a set of range in which each range is with a probability.
, the dependency function g should be taken into consideration while calculating the quality parameter qx of vj·CON. Because of the effect of the dependency function g, the value of the quality parameter qx may not be a single range, but a set of range in which each range is with a probability.
Taking the service value pv as an example, how to calculate the quality parameters of CON of a non-independent value is given as follow.
It is assumed that there is a value dependency relationship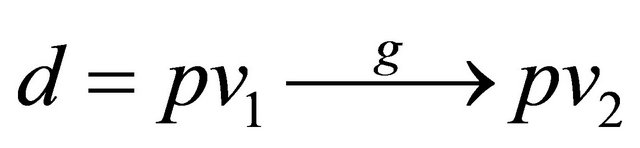 , which is caused by the uncertainty of relationship between the quality parameters of se2 and se1 (se1, se2 is related to pv1 and pv2 respectively).
, which is caused by the uncertainty of relationship between the quality parameters of se2 and se1 (se1, se2 is related to pv1 and pv2 respectively).
If only one quality parameter qx of pv2·CON is related to the uncertainty of relationship, the dependency function can be denoted as g = P(Bh|Ak) (for ;
;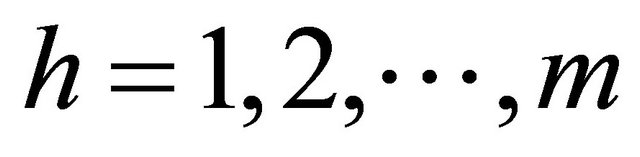 ).
).
As mentioned above,  , and
, and  depends on
depends on  and g. The probability set “
and g. The probability set “ ,
,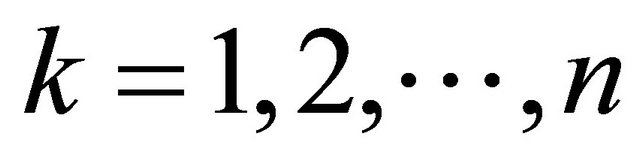 ” may be transformed into the matrix
” may be transformed into the matrix . The set of conditional probability P(Bh|Ak) (for
. The set of conditional probability P(Bh|Ak) (for 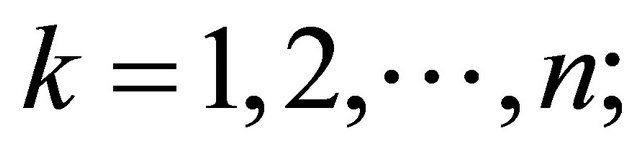
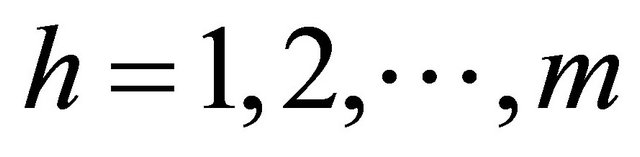 ) may also be transformed into the matrix
) may also be transformed into the matrix .
.
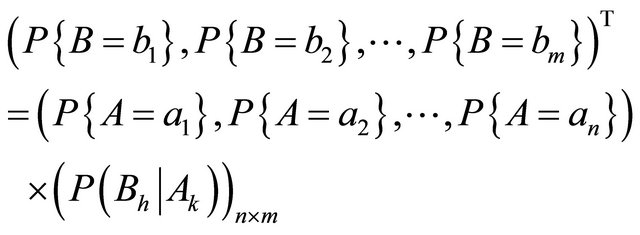
and 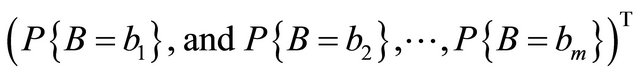 can be transformed from the probability set “
can be transformed from the probability set “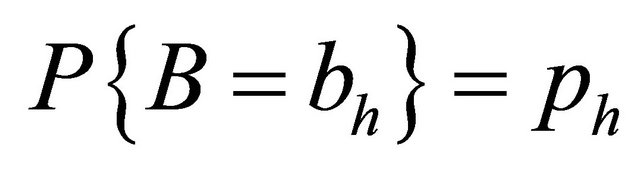 ,
, ”, therefore,
”, therefore, 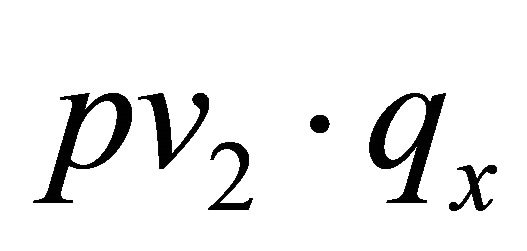 can be calculated by using the multiply operation of matrix. The data of the matrix (P(Bh|Ak))n´m should be provided by service model designers, and the data of the matrix M(A) may be obtained by analyzing all relevant historical data.
can be calculated by using the multiply operation of matrix. The data of the matrix (P(Bh|Ak))n´m should be provided by service model designers, and the data of the matrix M(A) may be obtained by analyzing all relevant historical data.
To bring 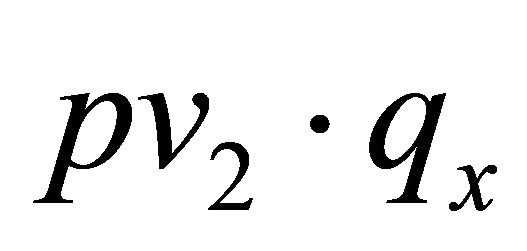 to the formula (2), and
to the formula (2), and  may be a set of values in which each value is with a probability, at last it may result in that pv2 may also be a probability distribution.
may be a set of values in which each value is with a probability, at last it may result in that pv2 may also be a probability distribution.
If there are two quality parameters qx and qy which are related to the uncertainty of relationship, then the dependency function can be denoted as g = {P(Bh|Ak) (for ), P(Dh|Ck) (for
), P(Dh|Ck) (for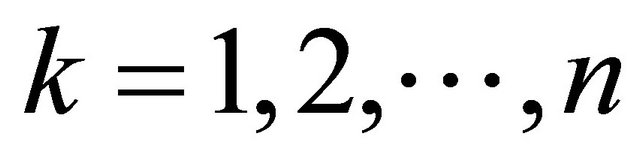 ;
;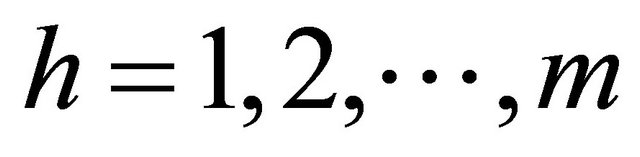 )}. The discrete random variable C represents the value of quality parameter se1·qy. The discrete random variable D is the value of quality parameter
)}. The discrete random variable C represents the value of quality parameter se1·qy. The discrete random variable D is the value of quality parameter .
.
Therefore, pv2·qx is the probability set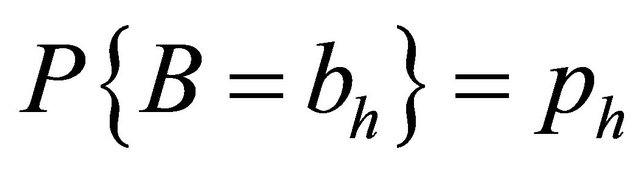 ,
,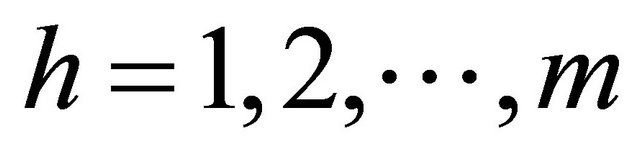 . and
. and  is the probability set
is the probability set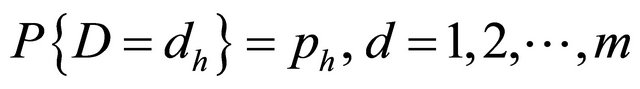 . To bring
. To bring  and
and 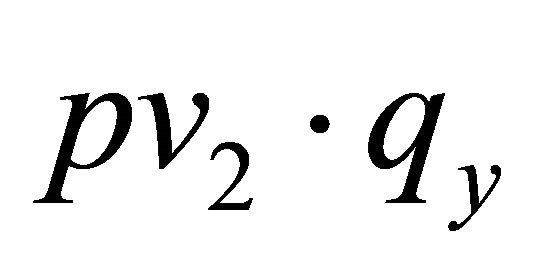 to the formula (2),
to the formula (2),  and hy(pv2·qy) can be obtained respectively. If the amount of members of the probability set related to
and hy(pv2·qy) can be obtained respectively. If the amount of members of the probability set related to 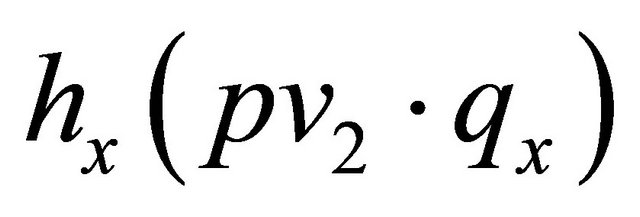 is K and the one related to
is K and the one related to 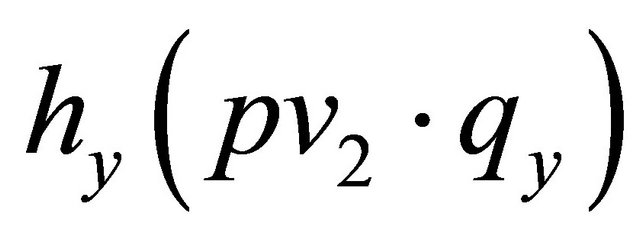 is L, then at last, by carrying on synthetical calculation, the amount of members of the probability set related to pv2 may be K ´ L.
is L, then at last, by carrying on synthetical calculation, the amount of members of the probability set related to pv2 may be K ´ L.
In the above measurement process, the result of the function h and the result of the value indicators pv_C are always a set of probability. For these probability sets, their corresponding event may be the same, which results in that the amount of members of the probability set related to output is less than the one related to input. In the most extreme case imaginable, pv2 is a probability of an event, and the probability is 100%.
For the situation of that there are more quality parameters affected the uncertainty of relationship, the measurement process is similar to the above one, and it is unnecessary gives more details.
5. Case Study
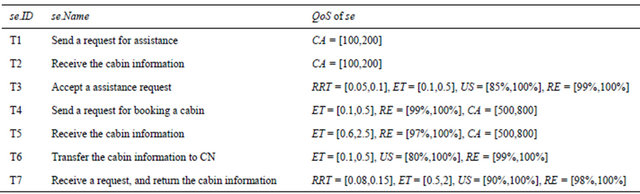
As mentioned in Section 1, there are two service solutions for cabin booking service. In the first service solution, the consigners send a request to the forwarders, asking the forwarders to help him to book a cabin. The corresponding pre-designed service process model is shown in Figure 6. The detailed information of service tasks in this model is listed in Table 2.
In this service process model, the quality parameter T7.ET, T5.ET and their relationship are uncertain. The uncertainty of T7.ET is denoted as P{A = ak} = pk, k = 1, 2. A1 is “A = [0.5,1]”, and A2 is “A = [1,2]”. The uncertainty of T5.ET is denoted as P{B = bh} = ph, h = 1, 2, 3. B1 is “B = [0.6,1]”, B2 is “B = [1,2]”, and B3 is “B = [2,2.5]”. And the set of conditional probability P(Bh|Ak) (for k = 1, 2; h = 1, 2, 3) = {{P(B1|A1) = 5%, P(B2|A1) = 80%, P(B3|A1) = 15%}, {P(B1|A2) = 0%, P(B2|A2) = 70%, P(B3|A2) = 30%}} can be used to describe the uncertainty of the relationship between T7.ET and T5.ET. The service process model and its relevant data are supposed to be collected and provided by model designers.
In the first solution, there are two service values cv1 and cv2. Their detailed information is given in Table 3. In this example, the unit of all the value indicators is Yuan.
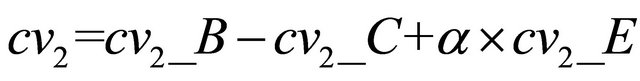 , where cv2_B and cv2_E is calculated as follow:
, where cv2_B and cv2_E is calculated as follow:
 ,
,
where "qiS, q4H Î cv2_CONB, and they are calculated as follow:
• 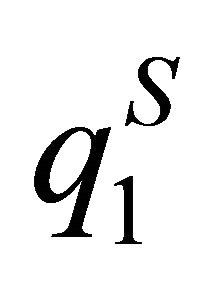 = T7.RRT =[0.08,0.15];
= T7.RRT =[0.08,0.15];
• 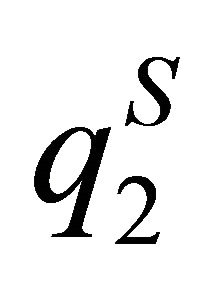 = T7.ET =[0.5,2];
= T7.ET =[0.5,2];
•  = T7.US= [90%,100%];
= T7.US= [90%,100%];
• 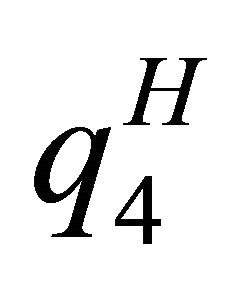 = T7.RE = [98%,100%].
= T7.RE = [98%,100%].
 , where
, where , and q5S = T4.CA+T5.CA = [1000, 1600].
, and q5S = T4.CA+T5.CA = [1000, 1600].
The constraints for ,
, 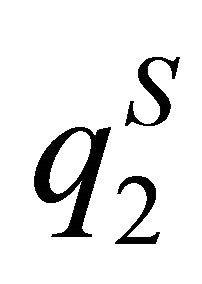 ,
,  ,
, 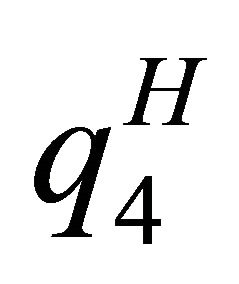 have been proposed by the forwarders. The constraints for q5S have been proposed by the ship company. And then the formulas of the functions
have been proposed by the forwarders. The constraints for q5S have been proposed by the ship company. And then the formulas of the functions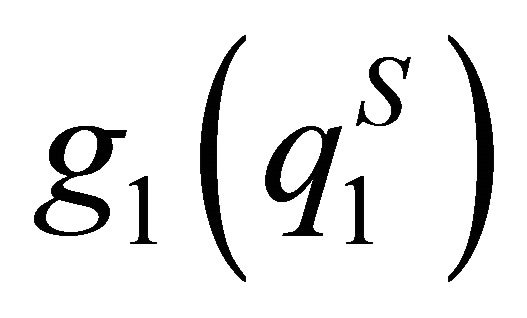 ,
, 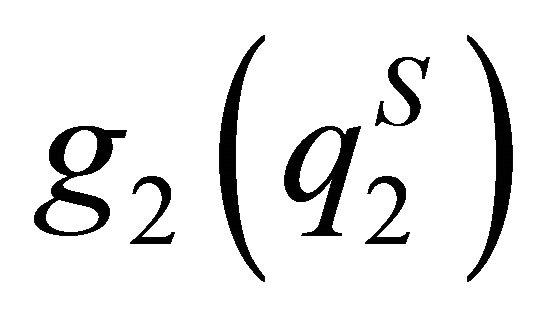 ,
,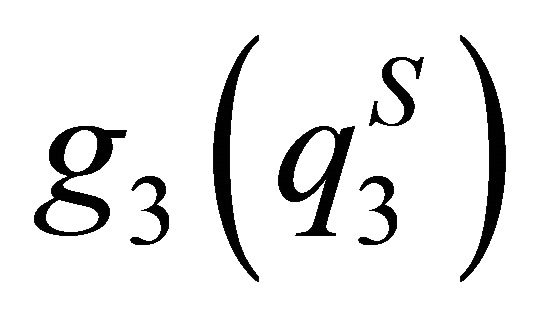 ,
,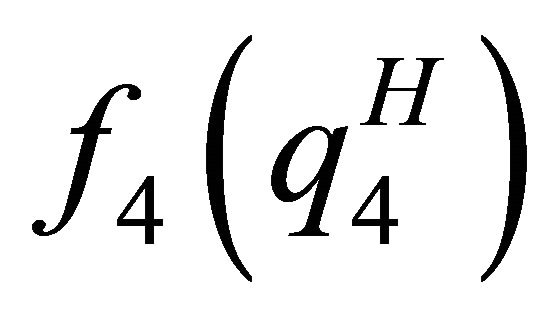 and
and 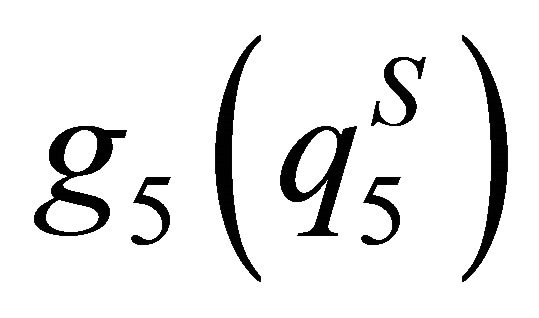 are shown in Figure 7.
are shown in Figure 7.
In the formula to caulcualte cv2_B, the weights of the functions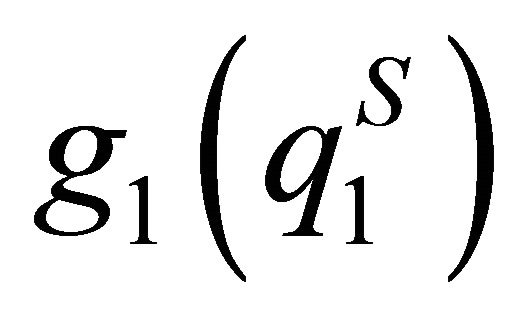 ,
, 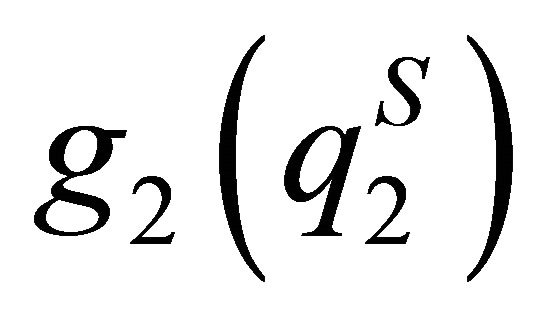 ,
, 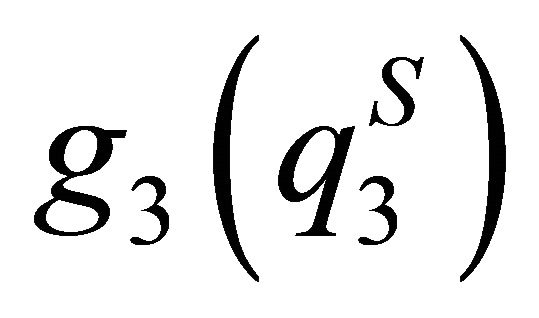 are assigned to 0.4, 0.4, 0.2 respectively. Therefore, the results can be obtained:
are assigned to 0.4, 0.4, 0.2 respectively. Therefore, the results can be obtained: ,
, 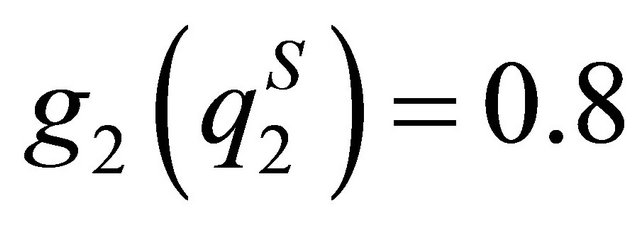 ,
,  ,
, 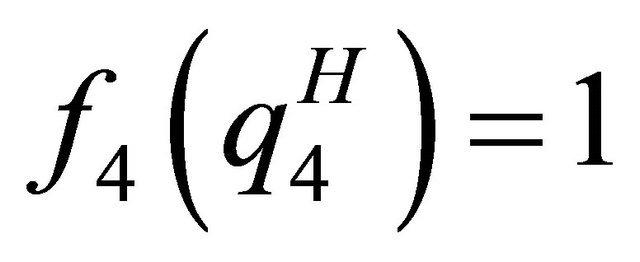 ,
, 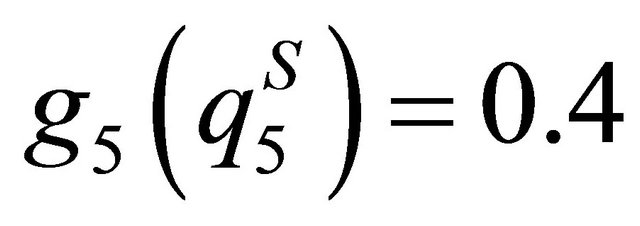 , and then cv2 = 84 – 40 + 8 = 52 (Yuan).
, and then cv2 = 84 – 40 + 8 = 52 (Yuan).
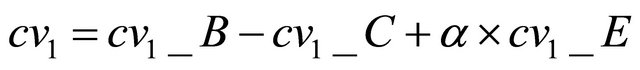 . cv1 is dependent on cv2, and the dependency function is P(Bh|Ak) (for k = 1, 2; h = 1, 2, 3). The calculation of cv1 is similar to the one of cv2, only one difference is that the quality parameter
. cv1 is dependent on cv2, and the dependency function is P(Bh|Ak) (for k = 1, 2; h = 1, 2, 3). The calculation of cv1 is similar to the one of cv2, only one difference is that the quality parameter 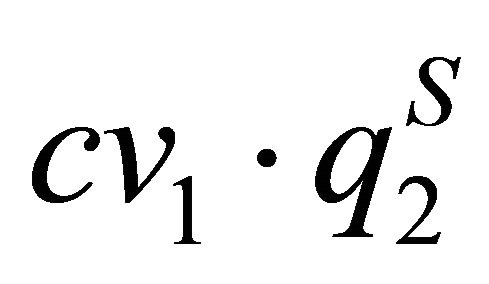 is calculated based on not only the quality parameters T3.ET, T4.ET, T5.ET, T6.ET (related to cv1) but also the quality parameter T7.ET (related to cv2).
is calculated based on not only the quality parameters T3.ET, T4.ET, T5.ET, T6.ET (related to cv1) but also the quality parameter T7.ET (related to cv2).
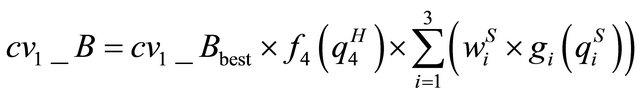 where
where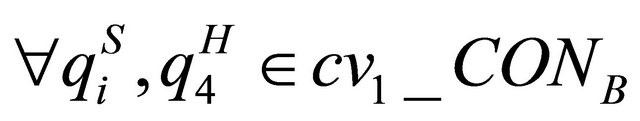 , and they are calculated as follow:
, and they are calculated as follow:
• 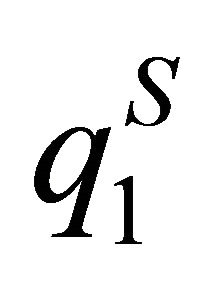 = T3.RRT = [0.05,0.1];
= T3.RRT = [0.05,0.1];
• 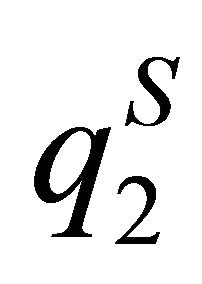 = T3.ET + T4.ET + T5.ET + T6.ET = [0.3,1.5] + T5.ET;
= T3.ET + T4.ET + T5.ET + T6.ET = [0.3,1.5] + T5.ET;
• 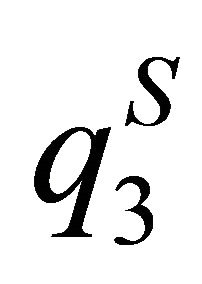 = min(T3.US, T6.US) = [80%,100%];
= min(T3.US, T6.US) = [80%,100%];
• 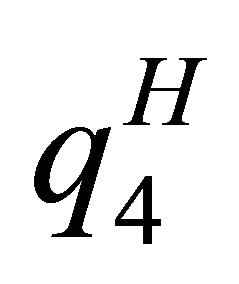 = T3.RE ´ T4.RE ´ T5.RE ´ T6.RE = [94%, 100%].
= T3.RE ´ T4.RE ´ T5.RE ´ T6.RE = [94%, 100%].
The constraints for 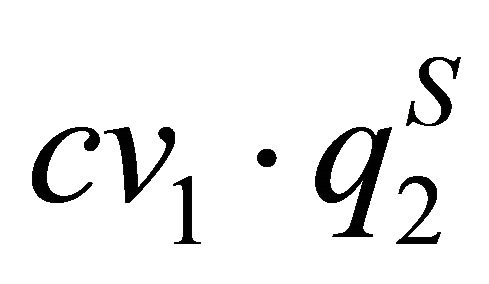 have been proposed by the consigners. And then in the formula to calculate cv1_B, the formulas of the function
have been proposed by the consigners. And then in the formula to calculate cv1_B, the formulas of the function 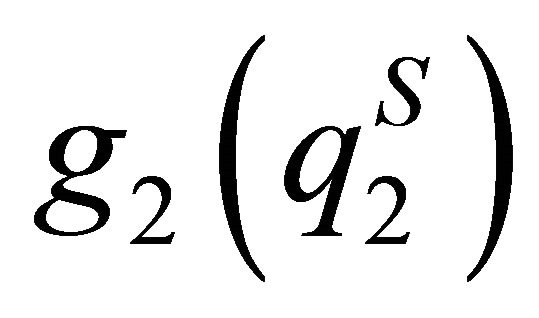 is
is
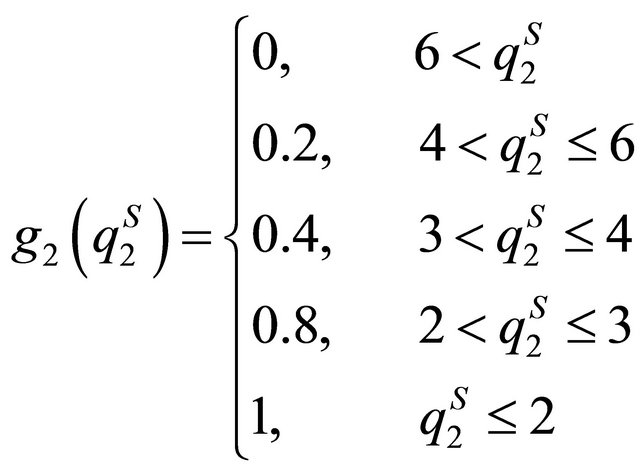 .
.
The formulas of the other functions in the formula to caulcualte cv1_B are the same as the corresponding ones in the formula to caulcualte cv2_B.
By analyzing all relevant historical data, P{A = [0.5,1]} = 80% and P{A = [1,2]} = 20% can be obtained. And then T5.ET can be calcualted by utilizing the multiply operation of matrix “(P{A = [0.5,1]}, P{A = [1,2]}) ´ (P(Bh|Ak))2´3”. The results of T5.ET can be obtained: P(B = [0.6,1]) = 4%, P(B = [1,2]) = 78% and P(B = [2,2.5]) = 18%. Therefore, the results of  and
and 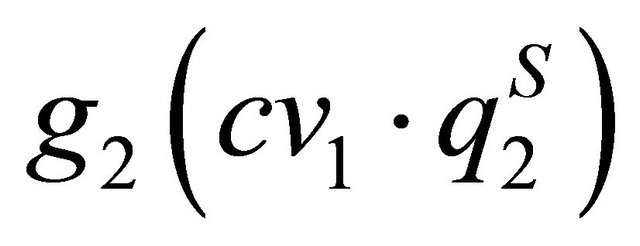 can be given as follow:
can be given as follow:
Table 3. The detailed information of two values belonging to cv.

 : Request response time;
: Request response time; : Execution time;
: Execution time;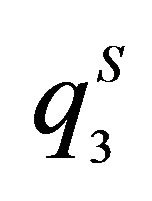 : Usability;
: Usability;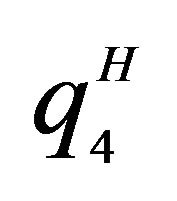 : Reliability;
: Reliability;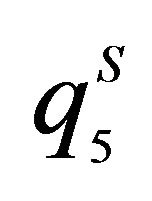 : Consumption amount.
: Consumption amount.
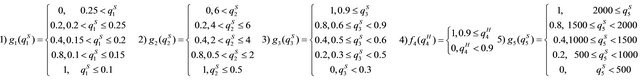
Figure 7. The formulas of the functions ,
, 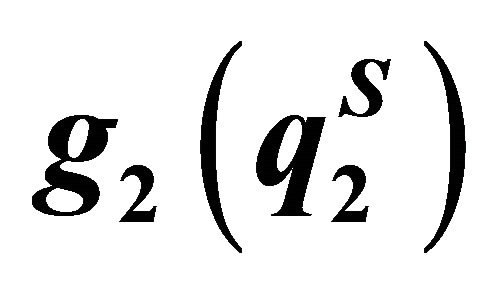 ,
,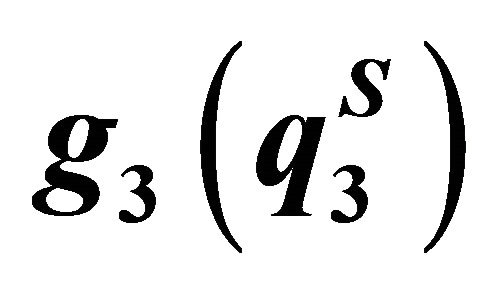 ,
,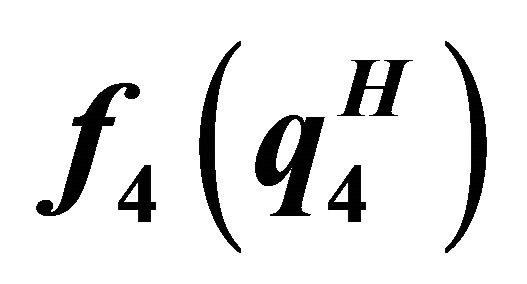 and
and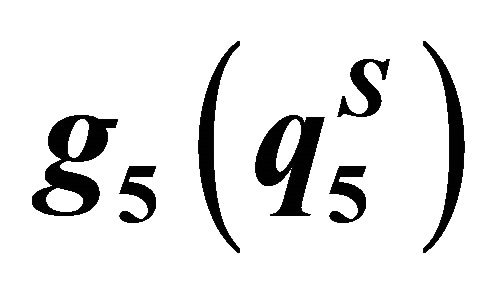 .
.
• B1 is the event “B = [0.6,1]”, if B1 occurred,  ,
,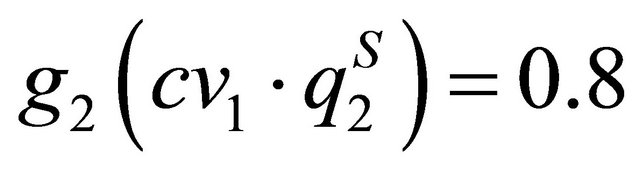 ;
;
• B2 is the event “B = [1,2]”, if B2 occurred,  ,
, ;
;
• B3 is the event “B = [2,2.5]”, if B3 occurred,  ,
,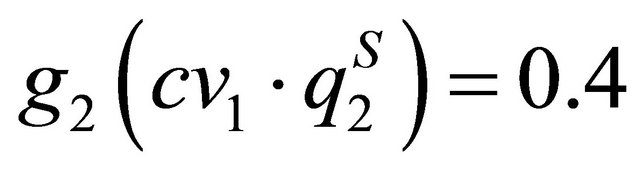 .
.
And then by utilizing the other corresponding formulas of function, 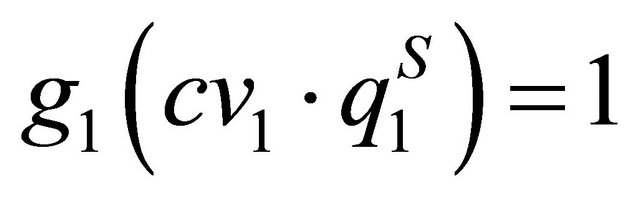 ,
, 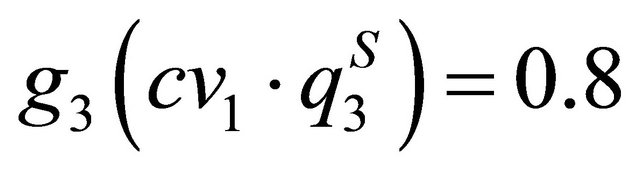 and
and 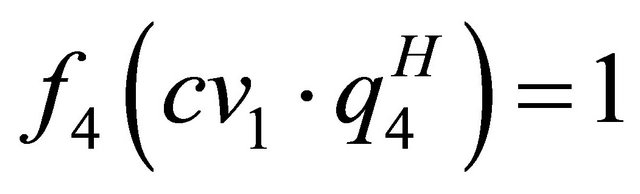 can be obtained.
can be obtained.
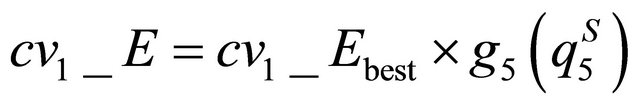 where
where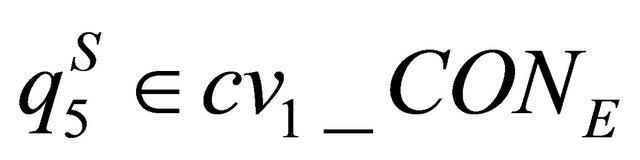 , and
, and 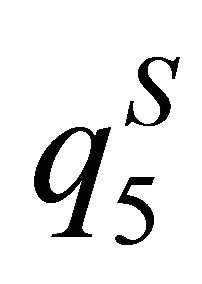 = T1.CA + T2.CA = [200,400].
= T1.CA + T2.CA = [200,400].
The constraints for 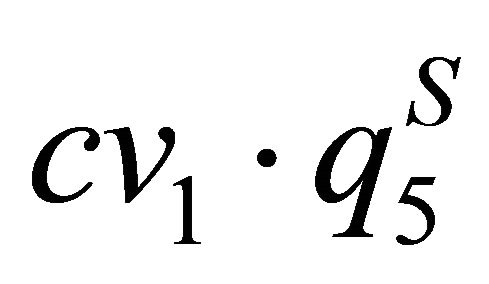 have been proposed by the forwarders. And then in the formula to calculate cv1_E, the formulas of the function
have been proposed by the forwarders. And then in the formula to calculate cv1_E, the formulas of the function 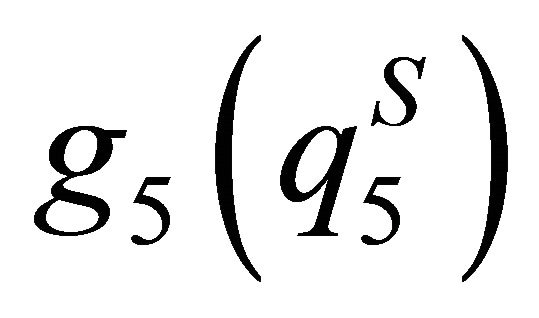 is
is
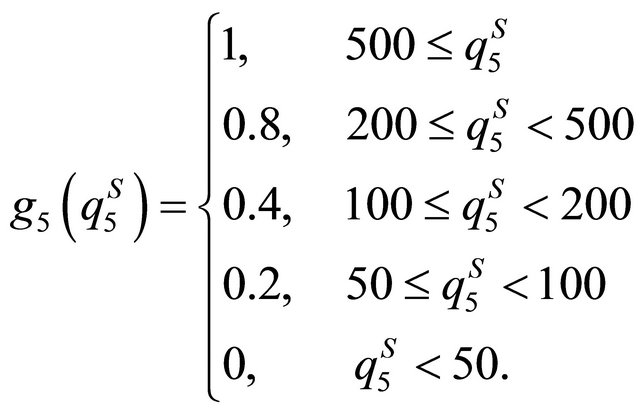
So,  can be obtained. At last, the measurement result of cv1 is: P{cv1 = 84} = 4%, P{cv1 = 52} = 96%.
can be obtained. At last, the measurement result of cv1 is: P{cv1 = 84} = 4%, P{cv1 = 52} = 96%.
In the first solution, there are two service values pv1 and pv2. The detailed information is given in Table 4.
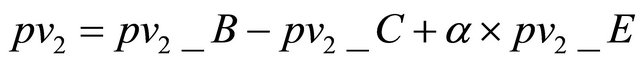 , where pv2_C and pv2_E is calculated as follow:
, where pv2_C and pv2_E is calculated as follow:
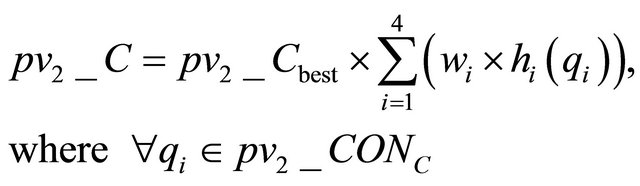
and they are calculated as follow:
• q1 = T7.ET = [0.08,0.15];
• q2 = T7.ET = [0.5,2];
• q3 = T7.US = [90%,100%];
• q4 = T7.RE = [98%,100%].
pv2_E = pv2_Ebest ´ sat.
In the above two formulas, the formulas of the functions h1(q1), h2(q2), h3(q3), h4(q4) are:
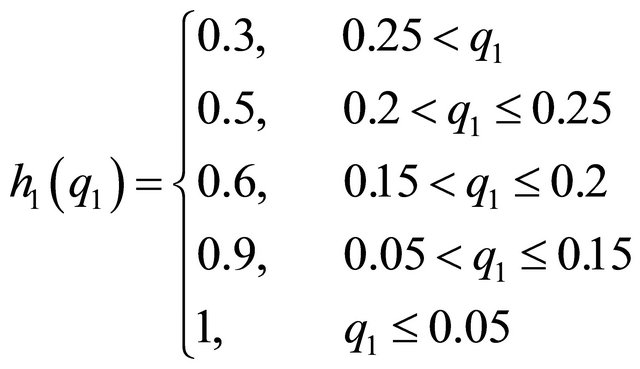
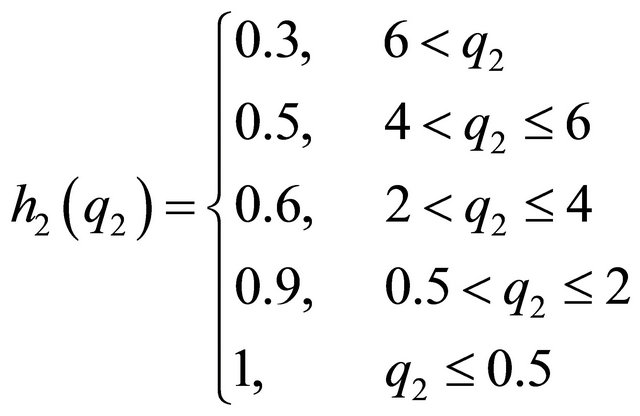
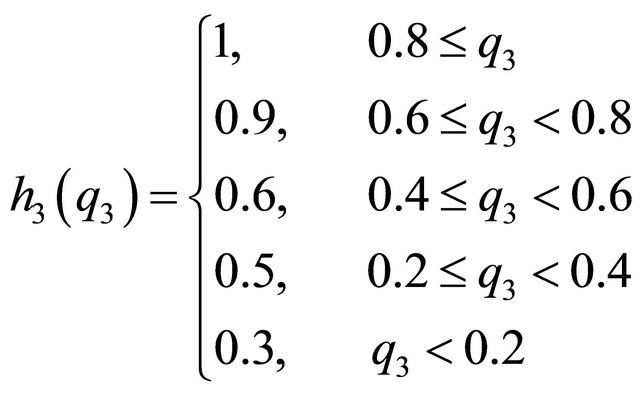
 .
.
And then the weights of the functions h1(q1), h2(q2), h3(q3) and h4(q4) are assigned to 0.3, 0.3, 0.2, 0.2 respectively. Therefore, pv2 = 40 – 9.7 + 16 = 46.3 (Yuan). Referring to the measurement process of pv2 and cv1, the measurement result of pv1 can be obtained: P{pv1 = 65.7} = 82%, P{pv1 = 71.2} = 18%.
In the second solution, the consigners directly send a request to the ship company for booking a cabin. The detailed information of the corresponding pre-designed service tasks is listed in Table 5. Simultaneously, there are two service values cv1 and pv1 in this solution, as shown in Tables 6 and 7. The measurement process of cv1 and pv1 is similar in the first solution, and result is given in Table 8.
The comparison results between the first and the second service solution is shown in Table 8. As the table implies, for the consigners, the first service solution is better and should be chose because that: 1) the realized CONB (1, <0.8, 0.4>, 0.8, 1) in the first one is be superior
Table 4. The detailed information of two values belonging to pv.
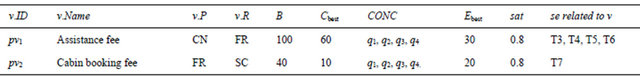
q1: Request response time; q2: Execution time; q3: Usability; q4: Reliability.
Table 5. The detailed information of service tasks.

Table 6. The detailed information of cv1 that the consigners receive.

Table 7. The detailed information of pv1 that the ship company receives.

Table 8. The comparison results between the first and the second service solution.
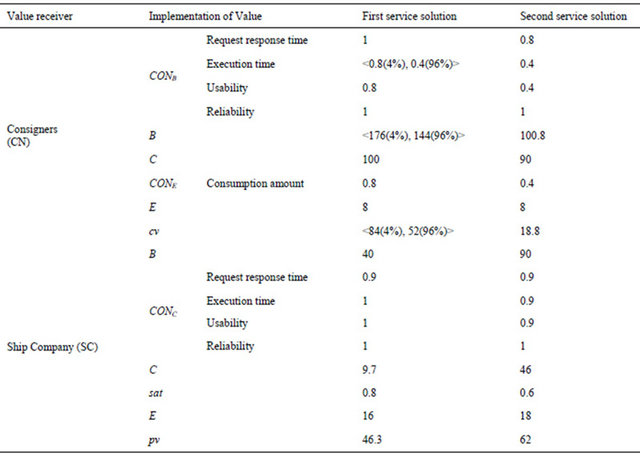
to the realized CONB (0.8, 0.4, 0.4, 1)in the second one overall. And the realized cv <84, 52> in the first one is larger than the realized cv 18.8 in the second one. So the service delivered to the consigners in the first one are better.
For the ship company, although in the first solution the value that it receives is smaller (i.e. 46.3 < 62), on the one hand, the cost that ship company need to pay for providing cabin booking service is smaller (i.e. 9.7 < 46), which can lead to an increase of cash flow, on the other hand, the total value that it receives may is larger in the future period of time because that the forwarders can help the ship company to obtain more customers. So the first service solution should also be chose by the ship company.
6. Conclusions
In order to help service participants to evaluate the existing service solutions, and select the best one among them measured by value, this paper presents an approach of service value measurement based on service semantics. In this paper, an intuitive definition of service value is given firstly, and secondly several concepts (e.g. customer value, provider value, value indicators, value profit constraints, etc.) related to service value measurement are discussed, then a series of calculation formulas are introduced to measure the above concepts based on the pre-designed service process model (that is used to represent the service solution being evaluated), and last the effect of value dependency relationships on service value measurement is taken into consideration.
By utilizing the proposed approach, service participants can select the best service solutions among multiple existing service solutions measured by value. Simultaneously, the corresponding service process model has been also chose. And then by utilizing model-driven idea and component-based software development technology, the appropriate service systems can be developed rapidly based on the service process model. The proposed approach is a beneficial supplement to Service Engineering.
6. Acknowledgements
Research works in this paper are supported by the National Natural Science Foundation (NSF) of China (Nos. 61033005, 61272187 and 70971029).
REFERENCES
- Z. J. Yan, “New Reading of Labour Value Theory,” Journal of Peking University (Humanities and Social Sciences), Vol. 6, 2001, pp. 10-17. (in Chinese)
- M. E. Porter, “Competitive Advantage,” Huaxia Press, Beijing, 1997.
- X. F. Xu and Z. J. Wang, “Value-Aware Service Model Driven Architecture and Methodology,” Service Science Cross Session of the 20th World Computer Congress. EGovernment: ICT Professionalism and Competences— Service Science, IFIP International Federation for Information Processing, Springer, Boston, 2008, pp. 277-286.
- CIO Council, “Value Measuring Methodology: How to Guide,” 2002. http://www.cio.gov/documents/ValueMeasuring_Methodology_HowToGuide_Oct_2002.pdf
- N. Caswell, S. Feldman, C. Nikolaou, J. Sairamesh and M. Bitsaki, “Estimating Value in Value Networks,” 2010. http://www.tsl.csd.uoc.gr/media/workingpaper_value_nets.pdf.
- V. Allee, “Reconfiguring the Value Network,” Journal of Business Strategy, Vol. 21, No. 4, pp. 2000, pp. 36-39. doi:10.1108/eb040103
- N. Caswell, C. Nikolaou, J. Sairamesh, M. Bitsaki, G. D. Koutras and G. Iacovidis, “Estimating Value in Service Systems: A Case Study of a Repair Service System,” IBM Systems Journal, Vol. 47, No. 1, 2008, pp. 87-100. doi:10.1147/sj.471.0087
- V. Allee, “A Value Network Approach for Modeling and Measuring Intangibles,” Transparent Enterprise, Madrid, 2002.
- J. Gordijn and J. M. Akkermans, “e3-Value: Design and Evaluation of e-Business Models,” IEEE Intelligent Systems, Vol. 16, No. 14, 2001, pp. 11-17.
- J. Gordijn, E. Yu and B. van de Raadt, “e-Service Design Using i* and e3-Value Modeling,” IEEE Software, Vol. 23, No. 3, 2006, pp. 26-33. doi:10.1109/MS.2006.71
- J. Gordijn, P. van Eck and R. Wieringa, “Requirements Engineering Techniques for e-Services. Service-Oriented Computing,” The MIT Press, Cambridge, 2009.
- J. Gordijn and J. M. Akkermans, “Value-based Requirements Engineering: Exploring Innovative e-Commerce Ideas,” Requirements Engineering, Vol. 8, No. 2, 2003, pp. 114-134. doi:10.1007/s00766-003-0169-x
- Q. Li and Y. L. Chen, “Modeling and Analysis of Enterprise and Information System,” Higher Education Press, Beijing, 2007. (in Chinese)
- C. Ma, Z. J. Wang and X. F. Xu, “A Semi-Automatic Service Value Annotation Method Supporting Service Model Analysis,” Computer Integrated Manufacturing Systems, Vol. 17, No. 10, 2011, pp. 2286-2297. (in Chinese)
- Z. J. Wang, X. F. Xu, D. H. Chu, et al., “Value Annotation for Service Model Analysis,” 2010 International Conference on Service Science (ICSS 2010), Hangzhou, 14-15 May 2010, pp. 219-225.
- S. Liu, X. F. Xu and Z. J. Wang, “A SQFD Approach for Service System Design Evaluation & Optimization,” Proceedings of 5th International Conference on Interoperability for Enterprise Software and Applications, Beijing, 21-21 April 2009, pp. 23-27.
- J. M. Carman, “Consumer Perceptions of Service Quality: An Assessment of the SERVQUAL Dimensions,” Journal of Retailing, Vol. 66, No. 1, 1990, pp. 33-55.
- G. Box, G. M. Jenkins and G. Reinsel, “Time Series Analysis: Forecasting and Control,” Prentice Hall, Upper Saddle River, 1994.
NOTES
*Corresponding author.

