Abstract

In this paper, we are concerned with Reflected Geometric Brownian Motion (RGBM) with two barriers. And the stationary distribution of RGBM is derived by Markovian infinitesimal generator method. Consequently the first passage time of RGBM is also discussed.

1. Introduction

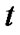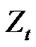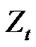We consider a finite-capacity fluid queue, the level of which at time is denoted by. And satisfies the following differential equation:

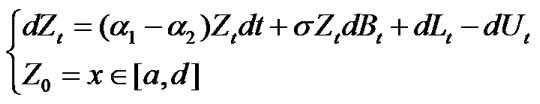(1)

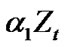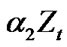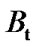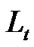This model shows fluid arrives into this queue at rate and leaves the queue at rate. This fluid level can be also varied by a local variance function and a standard Brownian motion. and 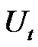are nondecreasing processes, interfering only when hits a or d and make vary between a and d.

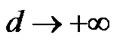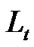In particular, when and, and 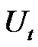disappear. Then the process 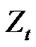becomes Geometric Brownian Motion. So we call determined by (1) Reflected Geometric Brownian Motion(RGBM).

Speak precisely, we are concerned with RGBM 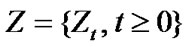with two barriers a and d (d > a > 0), which is defined by

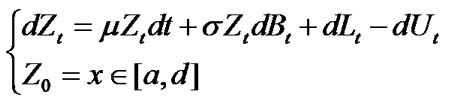(2)

where 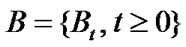is a standard Brownian Motion, and 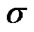are constants and satisfy.

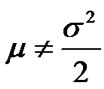Moreover, the processes L and U are uniquely determined by the following property [1,2]:

1) Both L and U are continuous nondecreasing processes with;

2) L and U increase only when and; respectively, i.e.,

According to the theory of stochastic differential equation, (2) is equivalent to

(3)

Such a process is a regenerative Markov process with state space [a,d] compact. Then it has a unique stationary distribution [1,3,4]. In the coming section, our objective is to derive the stationary distribution and give an expression for the Laplace Transform of the first passage time of RGBM by the method in [5-7].

2. Main Results on RGBM

2.1. On the Stationary Distribution of RGBM

In this section, we firstly give a Lemma on the stationary distribution of the reflected process with two-sided barriers and omit its proof.

Lemma 2.1 Let Z be the RGBM defined by (2) (or(3)). Then, as a Markov process, the stationary distribution 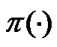of the process must satisfy the following equation

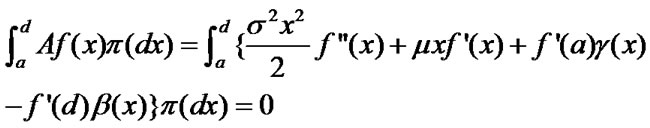(4)

where and which denotes the space of all bounded continuous functions having twicely continuous derivatives on [a,d].

Proof. See similar argument in [1].

Suppose be a probability distribution on [a,d] and satisfies that

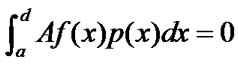(5)

for.

Define 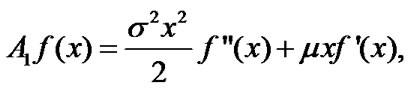then by (4) and (5) it is equivalent to the following equation (Note that)

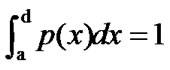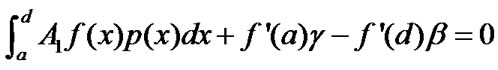(6)

where 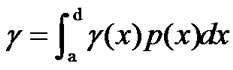and

On one h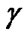and, and can be computed by the same method in [5].

Proposition 2.1 Choose and, then they respectively satisfy the following equations,

Then we have

Proof. A straightforward calculation.

On the other hand, since satisfies that for all,

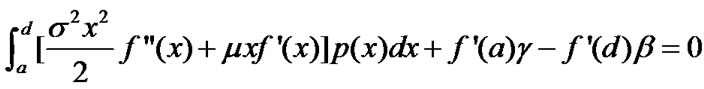
By twice integral changes, the above equation becomes that

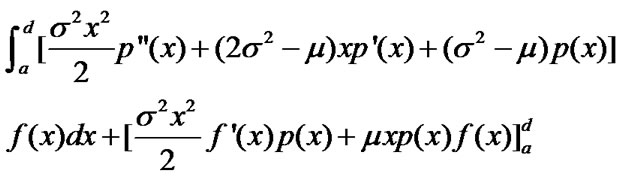
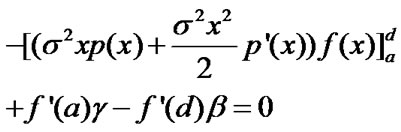
i.e.,

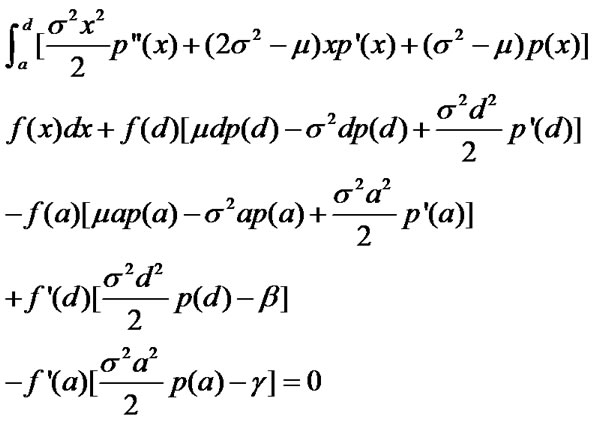(7)

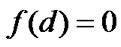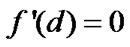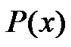Assume that, satisfying that, , and satisfies 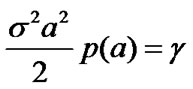and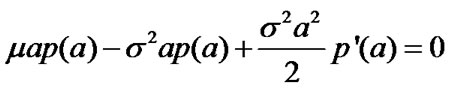, then it follows from (7) that

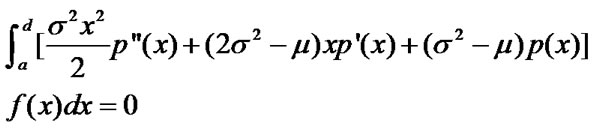(8)

Summarizing the discussion, we get the following theorem.

Theorem 2.1 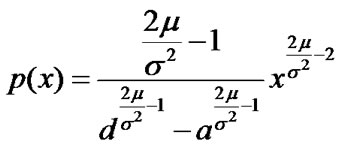is the solution of

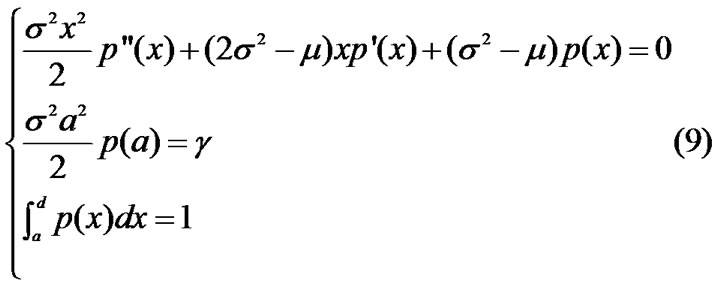
Then for all satisfying, , 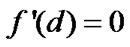(5) holds, i.e.,.

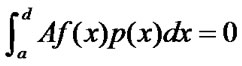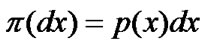Furthermore (5) holds for all. This implies that is a stationary distribution of the corresponding Markov process.

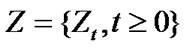Remark 2.1 This theorem is a standard application of renewal theorems, so we sketch its proof.

Thus 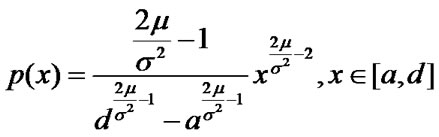is the density of the stationary distribution of RGBM. Finally we will give an expression for the Laplace transform of the first passage time of RGBM.

2.2. On the First Passage Time of RGBM

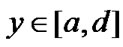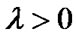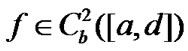In this section, we consider equation (2). Let, define the first passage time by with the usual convention. On the other hand, suppose, for, define a operator

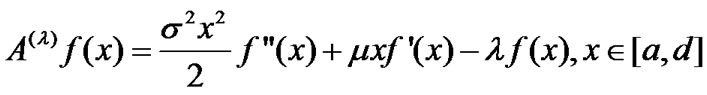
Finally we are going to give the expression of the Laplace transform of.

Theorem 2.2. For 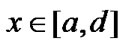and, then

(10)

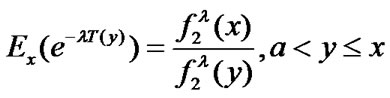(11)

where

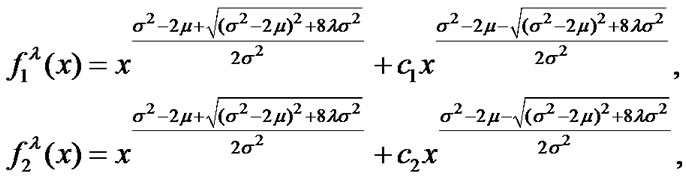
and

Proof. Letfor. Then applying formula for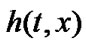, we have

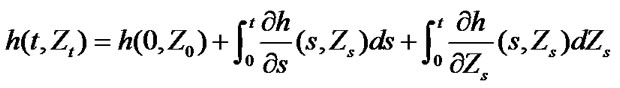
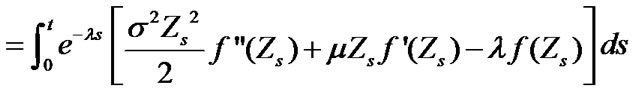
The last equation holds, for 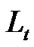and 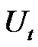increase only when and. Let be a stopping time and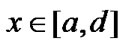. It follows from martingale optional theorem, that

(13)

In particular, take 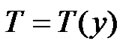for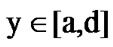, and note that

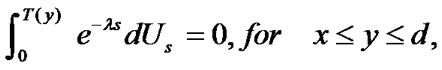
and

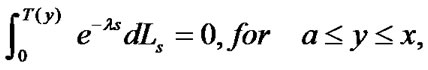
Then

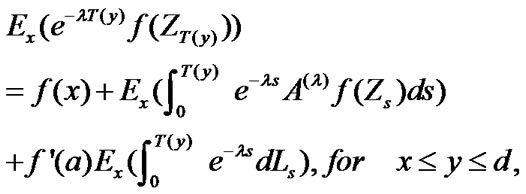(14)

and

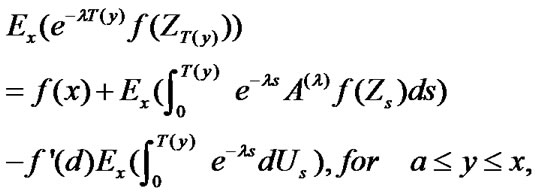(15)

Replace 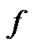by 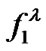in (14) and by 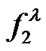in (15), we immediately get (10) and (11) by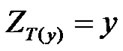, and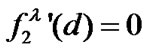. Thus the Proof of the theorem is completed.

3. Conclusions

This paper studies Reflected Geometric Brownian Motion (RGBM) with two barriers. Both the stationary distribution and Laplace transform of the first passage time of RGBM are derived. The studies for RGBM not only have practical significance, but also give an important result in theory of stochastic process.

4. Acknowledgements

This research is supported by the National Natural Science foundation of China (Grant No.70671074) and the Research Foundation of Tianjin university of Science and technology (Grant No.20080207). The authors would like to thank an anonymous referee for his constructive comments and suggestions on the first version of the manuscript.

5. References

[1] J. M. Harrison, “Brownian motion and stochastic flow systems,” John Wiley & Sons Ltd., New York, 1986.

[2] X. Y. Xing, W. Zhang and Y. J. Wang, “The Stationary Distributions of Two Classes of Reflected OrnsteinUhlenbeck Processes,” Journal of Applied Probability, Vol. 46, No. 3, 2009, pp. 709-720.

[3] S. Asmussen and O. Kella, “A Multi-Dimensional Martingale for Markov Additive Process and its Applications,” Advances in Applied Probability, Vol. 32, No. 2, 2000, pp. 376-393.

[4] S. Either and T. Kurtz, “Markov Processes: Characterization and Convergences,” John Wiley and Sons, New York, 1986.

[5] L. J. Bo, L. D. Zhang and Y. J. Wang, “On the First Passage Times of Reflected O-U Processes with Two-Sided Barriers,” Queueing Systems, Vol. 54, No. 4, December 2006, pp. 313-316.

[6] V. Linetsky, “On the Transition Densities for Reflected Diffusions,” Advances in Applied Probability, Vol. 37, No. 2, 2005, pp. 435-460.

[7] L. Rabehasaina and B. Sericola, “A Second-Order Markov-Modulated Fluid Queue with Linear Service Rate,” Journal of Applied Probability, Vol. 41, No. 3, 2004, pp. 758-777.