Natural Science
Vol.4 No.4(2012), Article ID:18629,6 pages DOI:10.4236/ns.2012.44036
Motion machines of second kind
![]()
Plovdiv University “P. Hilendarski”, Plovdiv, Bulgaria; *Corresponding Author: epitropov@uni-plovdiv.bg
Received 12 January 2012; revised 19 February 2012; accepted 28 February 2012
Keywords: Tardyons; Luxons; Physical Field; Second Law of Thermodynamics; Perpetual Motion Machine of Second Kind
ABSTRACT
The second law of thermodynamics, i.e. the law stating that the entropy in isolated macroscopic system can never decrease, is tightly connected to the work of the device called perpetual motion machine of second kind. Often this law is also defined as the inability to construct such a device. In the current paper we give complete, independent and consistent definitions of static, stationary and changing physical field. Based on that for the first time we give summarising, correct and complete definitions of natural resource machine and perpetual motion machine of second kind as well as motion machine of second kind in the set of tardyons and luxons. We present a principal structure of a motion machine of second kind using which we show that the Clausius statement and its equivalent statements in the thermodynamics can be violated for a practically big interval-time even under equilibrium fluctuations.
1. INTRODUCTION
Let 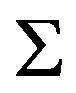 be the set of tardyons and luxons in a given macroscopic system. Tardyons and luxons are abovevacuum particles with respectively positive and zero standard rest masses which exist even freely in a physical three dimensional space. Tardyons are electrons, protons, neutrons, etc. Luxons are photons, the neutrino as well as the hypothetical particles gravitons the definite existence of which has not been experimentally proven yet. Exactly in the set
be the set of tardyons and luxons in a given macroscopic system. Tardyons and luxons are abovevacuum particles with respectively positive and zero standard rest masses which exist even freely in a physical three dimensional space. Tardyons are electrons, protons, neutrons, etc. Luxons are photons, the neutrino as well as the hypothetical particles gravitons the definite existence of which has not been experimentally proven yet. Exactly in the set 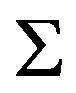 all the unsuccessful attempts in the history of humanity to create a perpetual motion machine of second kind have taken place [1].
all the unsuccessful attempts in the history of humanity to create a perpetual motion machine of second kind have taken place [1].
In this paper we give for the first time summarising, correct and complete definitions in 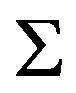 of natural resource machine and of perpetual motion machine of second kind. (Strict definitions of these terms are not given anywhere in the literature.) We also define in
of natural resource machine and of perpetual motion machine of second kind. (Strict definitions of these terms are not given anywhere in the literature.) We also define in 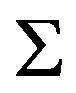 a new term—motion machine of second kind. We present a principal structure of such a machine and exactly using it we show that the Clausius statement in thermodynamics (and its equivalent statements) can be violated for a practically big interval-time even under equilibrium fluctuations.
a new term—motion machine of second kind. We present a principal structure of such a machine and exactly using it we show that the Clausius statement in thermodynamics (and its equivalent statements) can be violated for a practically big interval-time even under equilibrium fluctuations.
2. ABOUT THE SECOND LAW OF THERMODYNAMICS
The second law of thermodynamics is defined based on summarising of experimental facts but cannot be proven in the borders of thermodynamics. From statistical physics’ point of view it has a statistical character, i.e. it holds for the most probable behaviour of a system.
There are a few equivalent statements connected to the second law of thermodynamics, namely Thomson (Kelvin), Caratheodory and others. The oldest of them, formulated in 1850 is the Clausius statement according to which in an isolated macroscopic system consisting of two materials with different temperature under constant external parameters energy can flow only from the material at higher temperature to the material at lower temperature.
But this statement as we show in our paper can be violated for a practically big interval-time.
In 1854 Clausius gives another form of the second law of thermodynamics: “heat cannot of itself, without the intervention of any external agency, pass from a colder to a hotter body” [2]. He also formulates the second law the following way: “it is impossible to construct a device that, operating in a cycle, will produce no effect other than the extraction of heat from a cooler to a warmer body” [3].
According to the second law of thermodynamics the existence of a motion machine which continuously and without external agency converts heat into work is impossible. But there is no such restriction for a motion machine which continuously converts work into heat— indeed, work is converted into heat whenever there is friction. Therefore the second law states that some processes are time-asymmetric, i.e. irreversible. Thus this time-asymmetric law cannot be proven using only timesymmetric considerations. This problem has provoked a large number of researches (for example, see [3-6]).
In 1865 Clausius defines the concept of entropy the following way: for each quasi-equilibrium thermodynamic system there is such unambiguous function of the thermodynamic state , called entropy, such that its full differential is
, called entropy, such that its full differential is 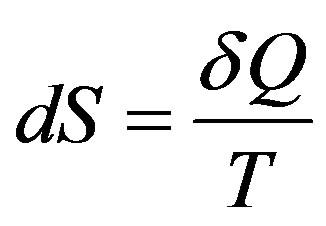 where
where 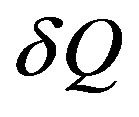 is the elementary amount of heat given to the system and
is the elementary amount of heat given to the system and 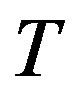 is its absolute temperature [7].
is its absolute temperature [7].
The second law of thermodynamics is based precisely upon the term entropy as a function of the state of a system and it states: the most probable consequence of a macro state of an isolated macroscopic system is the non-decrement of its entropy [8]. Thus in states with maximal entropy in an isolated system macroscopic irreversible processes (and the process of a heat flow is always irreversible according to Clausius statement) are impossible.
Clausius, looking into the second law of thermodynamics comes to the conclusion that the entropy of the Universe as an isolated system is heading to maximum and at the end in it all the macroscopic processes will end. This state of the Universe is named “heat death”. On the other hand Boltzmann states that the current state of the Universe is a giant fluctuation which means that in most of the time the Universe is in a state of thermodynamic equilibrium (“heat death”) [9]. According to Landau, the key to the solution of that contradiction is in the field of the general theory of relativity because “the Universe as a whole must be regarded not as a closed system in a variable gravitational field. Consequently the application of the law of increase of entropy does not prove that statistical equilibrium must necessarily exist” [10]. Therefore the second law of thermodynamics is inapplicable to infinite parts of the Universe and to the Universe as a whole [8].
Not only the classic but also a wide spectre of quantum-mechanic systems also increase their entropy with time [11].
It is also known that the second law of thermodynamics can also be violated under fluctuations. A fluctuation of a physical variable in a macroscopic system is a random diversion of the values of this variable from its mean value under an equilibrium state of the system. If that diversion does not change the equilibrium state of the system, i.e. its absolute value is relatively small, we will be talking about equilibrium fluctuation. However, if the diversion gets the system out of its equilibrium state, i.e. its absolute value is relatively big, we will be talking about non-equilibrium fluctuation. According to the statistical physics non-equilibrium fluctuations which get a closed macroscopic system in a state of less entropy are possible.
As the possibility of non-equilibrium fluctuations of a macroscopic system in a sensible interval of time is neglectfully small, this does not give a possibility for a working perpetual motion machine of second kind to exist in  based upon such fluctuations. However, the possibility is significantly greater for each sensible interval-time for heat fluctuations.
based upon such fluctuations. However, the possibility is significantly greater for each sensible interval-time for heat fluctuations.
In the current paper we would restrict the examination of the second law only in the set 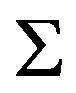 of the tardyons and luxons and in macroscopic systems. It is certain that a perpetual motion machine of second kind in
of the tardyons and luxons and in macroscopic systems. It is certain that a perpetual motion machine of second kind in 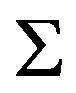 under usual thermodynamic systems cannot be made. For unusual thermodynamic systems, i.e. systems with negative absolute temperatures, in this set it is possible to construct the so-called Thomson-Planck perpetual motion machine of second kind. This is a device which carries out periodically positive work only at the cost of the cooling of a heat reservoir [8].
under usual thermodynamic systems cannot be made. For unusual thermodynamic systems, i.e. systems with negative absolute temperatures, in this set it is possible to construct the so-called Thomson-Planck perpetual motion machine of second kind. This is a device which carries out periodically positive work only at the cost of the cooling of a heat reservoir [8].
Outside the set 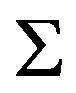 are the tachyons with purely imaginary standard rest masses but their existence is not proven yet. Even if it was proven that such particles exist then perpetual motion machine of second kind for them is indefinable term because with tachyons the cause is later in time in respect to the consequence.
are the tachyons with purely imaginary standard rest masses but their existence is not proven yet. Even if it was proven that such particles exist then perpetual motion machine of second kind for them is indefinable term because with tachyons the cause is later in time in respect to the consequence.
If there are any existing above-vacuum particles found freely in the physical three dimensional space which have negative standard rest masses (in the observed by us part of the Universe the existence of such particles is not stated) then perpetual motion machine of second kind would be possible because for a macroscopic system of particles with negative rest mass the phase hyper-surfaces are not closed; for such system there are no equilibrium micro-canonical and canonical distribution and besides it does not have minimal energy [12].
3. PHYSICAL FIELDS
In order to be able to strictly define a natural resource machine, perpetual motion machine of second kind and perpetual motion machine of second kind we will give a few definitions in respect to the term physical field.
Definition 1. A physical field U is called an objective reality which is generated in the physical three dimensional space by a respective to the reality charge.
Thus the physical field is one of the forms of matter characterising all points of the space and having infinite number of degrees of freedom. To each points of the space this way are assigned certain physical variables.
Let us remind that a physical variable in a given physical field U directly depends on the time t if it is not a complicated function of t and indirectly depends on the time t if it is a complicated function of t.
Let  is the full set of physical variables which characterise a physical field U. Then for U we introduce the following axiomatic terms which satisfy the conditions for completeness, consistency and independency:
is the full set of physical variables which characterise a physical field U. Then for U we introduce the following axiomatic terms which satisfy the conditions for completeness, consistency and independency:
1) U is a static field if none of the variables in  depends neither directly nor indirectly on the time;
depends neither directly nor indirectly on the time;
2) U is a stationary field if none of the variables in  depends directly in the time but at least one of them depends indirectly on the time;
depends directly in the time but at least one of them depends indirectly on the time;
3) U is a changing field if at least one variable in  depends directly on the time.
depends directly on the time.
Let’s point out that the static fields (gravitational, electrostatic, magnetostatic and other) and the stationary fields (constant water, air fields and other) in physics are called fields which are constant in the time.
4. NATURAL RESOURCE MACHINE, PERPETUAL MOTION MACHINE OF SECOND KIND AND MOTION MACHINE OF SECOND KIND
There are many known and constructed natural resource machines, i.e. machines using the nature’s resources.
Definition 2. Natural resource machine (NRM) is a real periodical device in the set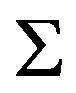 , which works for a certain interval-time at the obligatory cost of changing or stationary physical fields and possibly at the cost of static physical fields.
, which works for a certain interval-time at the obligatory cost of changing or stationary physical fields and possibly at the cost of static physical fields.
Such devices are possible not only theoretically, but are really working based on the natural laws, examined by mechanics, electrodynamics, thermodynamics and the theory of gravity. They use, for example, the energy of wind (windmills and wind generators); the energy of water (hydro-electric power stations); the sun energy (sun batteries) and other. Moreover, these machines are ecological.
The creation of NRM is a highly intensive science field as many scientists consider that they are the future source of power.
It is accepted that the supposed natural motion machines are classified according to which law of thermodynamics they would violate [13].
Perpetual motion machine of first kind (PMMFK) is an imaginary device which can convert work into nothing (without flow of energy out) or creates work from nothing (without using energy flow) [8]. Such device is impossible to construct in 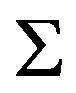 as the law for preservation of the energy cannot be violated (even when fluctuations occur—equilibrium or non-equilibrium of the physical variables in a closed macroscopic system of particles).
as the law for preservation of the energy cannot be violated (even when fluctuations occur—equilibrium or non-equilibrium of the physical variables in a closed macroscopic system of particles).
According to the settled understanding the perpetual motion machine of second kind is an imaginary device which for unlimited amount of time spontaneously converts energy into mechanical work.
Based on the defined in Section 3 terms we for the first time give strict definition of perpetual motion machine of second kind, namely:
Definition 3. Perpetual motion machine of second kind (PMMSK) is an imaginary periodically working device in the set 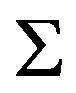 which without compensations works in eternal interval-time. Moreover, the energy it uses is at the cost of constant with the time fields and possibly at the cost of spontaneously generated in the device changing fields.
which without compensations works in eternal interval-time. Moreover, the energy it uses is at the cost of constant with the time fields and possibly at the cost of spontaneously generated in the device changing fields.
The requirement for spontaneously generated changing fields, i.e. fields depending directly on time and generated by the action of the system on itself, is natural— this specification about the PMMSK we give for the first time.
As we already pointed out it is not possible to create a PMMSK in 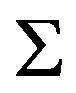 i.e. a device which without compensations can do positive work in a practically eternal interval-time. This is why all attempts to create a PMMSK have ended in total failure as the history of physics shows.
i.e. a device which without compensations can do positive work in a practically eternal interval-time. This is why all attempts to create a PMMSK have ended in total failure as the history of physics shows.
Definition 4. Motion machine of second kind (MMSK) is a real periodically working device in the set 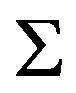 which without compensations works practically long finite interval-time. Moreover, the energy it uses is at the cost of constant with the time physical fields and possibly at the cost of spontaneously generated in the device changing fields.
which without compensations works practically long finite interval-time. Moreover, the energy it uses is at the cost of constant with the time physical fields and possibly at the cost of spontaneously generated in the device changing fields.
There is no natural prohibition in the set 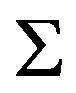 preventing the creation of MMSK—its construction does not violate the first law of thermodynamics for conservation of energy. And based on the given by us definition a number of different methods can be used in order to construct a MMSK.
preventing the creation of MMSK—its construction does not violate the first law of thermodynamics for conservation of energy. And based on the given by us definition a number of different methods can be used in order to construct a MMSK.
5. PRINCIPLE MODEL OF MOTION MACHINE OF SECOND KIND
The device on Figure 1 is a principle scheme of the suggested by us MMSK given in vertical section. It consists of two thermoses 1 and 2 with spherical shape in which have been put in liquid colloid disperse systems, respectively 3 and 4, differentiating from each other with respect to a few characteristics. The disperse environment 3 is chosen to be electrically non-conductive and its disperse phase consists of Brownian non-conducting (i.e. with non-conductive surfaces) particles 5, which are electrically charged. (The technique of making such type of disperse systems is well known and is not a significant practical difficulty.) The disperse environment 4 is chosen to be absorbing long-wave electromagnetic radiation.
In the initial stage of work of the device the disperse system 3 inside the thermos 1 is at lower temperature than the one in thermos 2. The walls of the thermoses 1 and 2 are required to be made of a material (such as
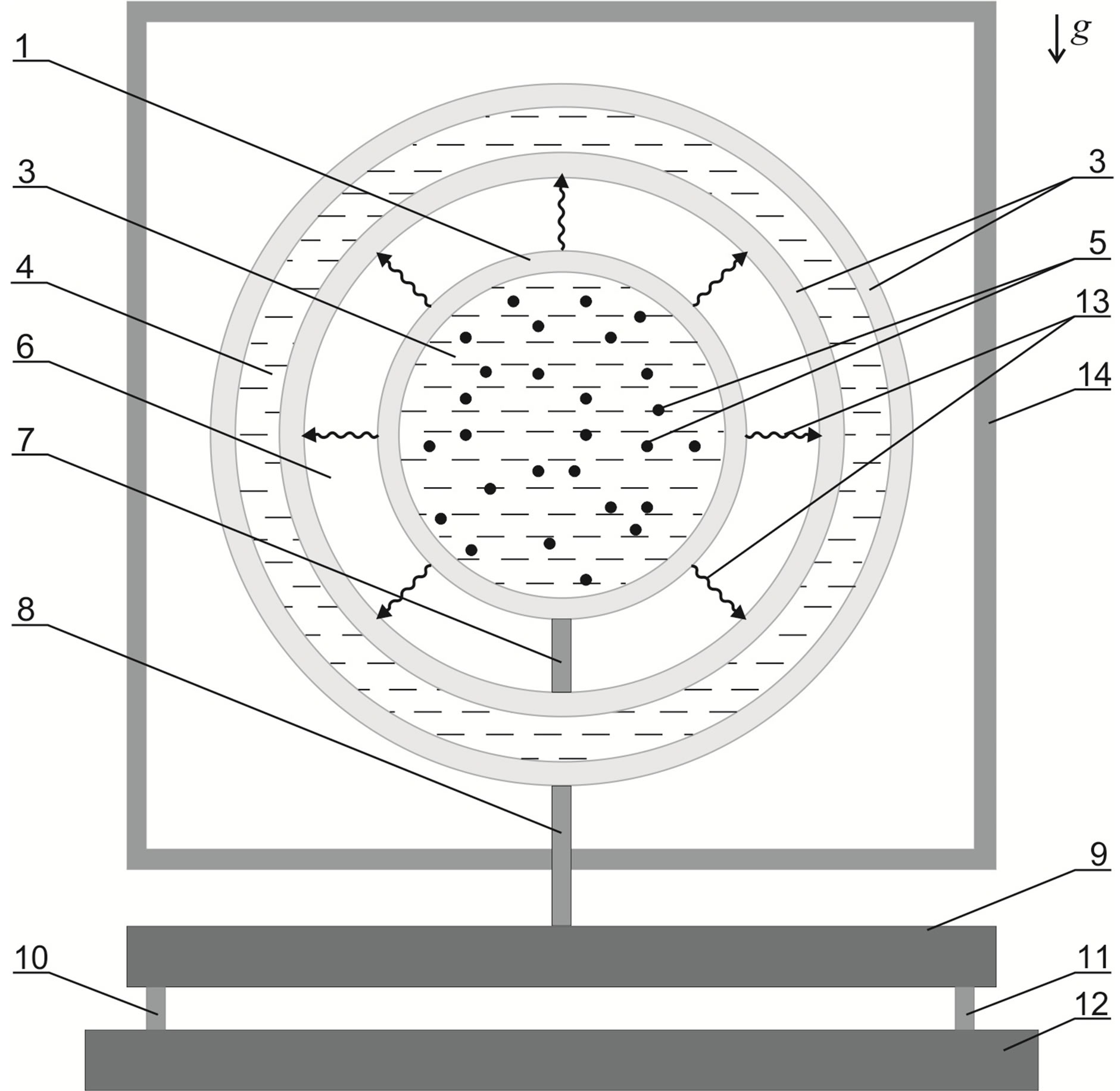
Figure 1. A principle model of MMSK.
suitable kind of glass) transparent enough for electromagnetic radiation in the long-wave part of the spectrum. The space 6 between the outer wall of thermos 1 and the inner wall of thermos 2 is vacuumed. The two thermoses 1 and 2 are fixed immovably in respect to each other using the support 7 and the thermos 2 is fixed immovably using support 8 to a massive panel 9. The panel 9 itself using supports 10 and 11 is fixed to the Earth 12.
The physical operation of the device on Figure 1 is as following: the electrically charged Brownian disperse particles 5 experiencing hits from the particles of the disperse environment 3 are moving chaotically—accelerating or decelerating. As it is known from the classic electrodynamics in a random inertial frame of reference a changing electromagnetic field can be radiated only by electrical charges with non-zero acceleration. (Upon this idea the whole operation of the device is based.) The electrically charged Brownian disperse particles are moving exactly with non-zero acceleration in the disperse environment of the thermos 1 and thus will generate changing electromagnetic field (mainly in the longwave spectrum) some quanta 13 of which are formally represented on Figure 1. This is possible, especially when the radius of thermos 1 is relatively small and the radiation is not absorbed by the disperse environment 3.
The disperse environment 3 in the thermos 1 will very weakly absorb the long-wave electromagnetic radiation which is generated by the electrically charged chaotically moving disperse particles. However, the disperse environment 4 in the thermos 2 will strongly absorb the same radiation. (Of course some part of the radiation will be reflected by the disperse particles themselves).
If we lock down adiabatically the whole device using a suitable isolating heat material sheath 14 then only after long enough interval-time 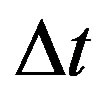 a thermodynamic equilibrium between the material and the radiation will be settled, i.e. the whole device will be in a state of thermodynamic equilibrium. Moreover, part of the heat energy of the disperse system 3 found inside the thermos 1 will be transferring to disperse environment 4 in the thermos 2 randomly. This way the material environment in the thermos 1 will be cooling down (randomly) and the one in the thermos 2 will be heating up (randomly). This means that in each interval-time
a thermodynamic equilibrium between the material and the radiation will be settled, i.e. the whole device will be in a state of thermodynamic equilibrium. Moreover, part of the heat energy of the disperse system 3 found inside the thermos 1 will be transferring to disperse environment 4 in the thermos 2 randomly. This way the material environment in the thermos 1 will be cooling down (randomly) and the one in the thermos 2 will be heating up (randomly). This means that in each interval-time 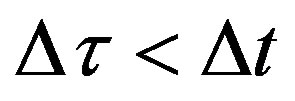 the device will work (in full agreement with the fundamental basis of the statistical physics) as a real, working MMSK.
the device will work (in full agreement with the fundamental basis of the statistical physics) as a real, working MMSK.
Naturally, in order to ensure the normal and continuous work the whole device should be well enough protected from the influence of outer disturbing factors – vibrations, noise, etc.
6. DISCUSSION
As early as XIX century Loschmidt as on opponent of Maxwell and Boltzmann reaches the conclusion that a PMMSK is possbile. He was convinced that he found an inexhaustible source of energy for the humanity but his ideas were not accepted. According to some contemporary researchers though, for example Trupp [14,15] Loschmidt was right.
In 1867 Maxwell gives the hypothesis of the so called Maxwell’s demon in order to use the methods of the statistical physics to shed some light on the possible violation of the second law of thermodynamics [16]. The impressive number of publications in recent years concerning this topic (for example the thorough chronological bibliography in [17]) shows that the confusion and the controversy in its interpretation does not decay. Earman and Norton [18,19] suggest that usually the proofs are successful because they assume whatever is actually to be proven but that does not mean that they are incorrect. Regrettably, the idea of Maxwell is often introduced without proper discussion of its context and this gives the possibility of wrong interpretation. For example, Leff and Rex [17,20] state: “Maxwell’s thought experiment dramatizes the fact that the second law is a statistical principle that holds almost all the time for a system composed of many molecules”. However, we think that Maxwell does not dramatise this fact, but shows the conditions under which it does not hold.
Many theoretical and experimental researches already show that the second law of thermodynamics is not always valid in the set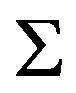 . Aristov and Nikulov believe [21] that considering a non-chaotic Brownian motion of particles with non-zero average velocity the second law of thermodynamics can be violated. (An example for such a movement of particles is the constant current with non-zero resistance.) They interpret an experimental observation of alternating current of quantum source of energy as an evidence for violation of the second law of thermodynamics.
. Aristov and Nikulov believe [21] that considering a non-chaotic Brownian motion of particles with non-zero average velocity the second law of thermodynamics can be violated. (An example for such a movement of particles is the constant current with non-zero resistance.) They interpret an experimental observation of alternating current of quantum source of energy as an evidence for violation of the second law of thermodynamics.
Having looked into the trajectories of colloid particles caught in an optic trap and having calculated their entropy in [22] it is shown that it is possible to violate the second law in microscopic system for a short period of time. This means that in such conditions it is possible to be realised a statistically lowly possible event in which enough number of warm particles take away energy from colder particles and thus transfer heat from colder to warmer source.
In [23] the possibility that an open quantum system can periodically produce mechanical work at the cost only of heat is demonstrated. Even though the effect is whole quantum and is not realised in classical systems it is an example for PMMSK which violates Thomson (Kelvin) statement in thermodynamics.
7. CONCLUSION
Through the formulated by us complete, independent and consistent definitions of static, stationary and changing fields we for the first time define the concept of motion machine of second kind. Moreover, our principal model of such motion machine shows that Clausius statement in thermodynamics can be violated for practically big interval of time.
The efficiency of the suggested in this paper device is not big enough. However, there are other possible technical solutions for MMSK with much higher efficiency than the suggested here device which can possibly find application for supplying the humanity with energy. Moreover, NRM and MMSK can be combined.
Actually every possible machine constructed by humans is in broader sense based on natural resources as it always uses given natural resources (petrol, gas, coals, uranium and other ores, etc.) This is also applied to the real MMSK and to the imaginary PMMSK if they could be realised. At the end the “given” natural resources are complexly determined by economical and financial causes. In that sense if a PMMSK could exist then it could be called “maximal natural resource motion machine” because it would ensure maximum efficiency.
In the set 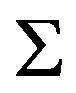 except for NRM and MMSK the socalled triggering mechanisms are also possible. Using them with neglectfully small amount of energy a large amount of energy can be unlocked. One of the most obvious examples for such a mechanism is the effect of the avalanche. It consists in the fact that even a really small quake or loud shout could unlock the colossal energy of the falling from the ridge of the mountain snow. These mechanisms are used, for example, when a super precise apparatus for proving the existence of gravitational waves is constructed.
except for NRM and MMSK the socalled triggering mechanisms are also possible. Using them with neglectfully small amount of energy a large amount of energy can be unlocked. One of the most obvious examples for such a mechanism is the effect of the avalanche. It consists in the fact that even a really small quake or loud shout could unlock the colossal energy of the falling from the ridge of the mountain snow. These mechanisms are used, for example, when a super precise apparatus for proving the existence of gravitational waves is constructed.
8. ACKNOWLEDGEMENTS
The current research is done with the financial support of the Fund “Scientific Studies” of the Bulgarian Ministry of Education, Youth and Science as part of the contract DTK 02/35.
REFERENCES
- Kamenov, P. (1972) Perpetual motion machines. Narodna Prosveta, Sofia.
- Glasstone, S. (1946) Textbook of physical chemistry. D. Van Nostrand Co., New York.
- Sklar, L. (1993) Physics and chance. Philosophical issues in the foundations of statistical mechanics. Cambridge University Press, Cambridge. doi:10.1017/CBO9780511624933
- Reichenbach, H. (1956) The direction of time. University of Los Angeles Press, Los Angeles.
- Horwich, P. (1987) Asymmetries in time: Problems in the philosophy of science. MIT Press, Cambridge.
- Albert, D. (2000) Time and chance. Harvard University Press, Cambridge.
- Kvasnikov, I. (2002) Thermodynamics and statistical physics. Vol І: Theory of Equilibrium systems: Thermodynamics. Editorial URSS, Moscow.
- Bazarov, I. (2010) Thermodynamics. Lan, Saint Petersburg.
- Sivukhin, D. (2005) General physics course. Volume 2. Fizmatlit, Moscow.
- Landau, L. and Lifshitz, Е. (1997) Statistical physics part 1. (Course of theoretical physics Volume 5). Butterworth Heinemann.
- Gemmer, J., Otte, A. and Mahler, G. (2001) Quantum approach to a derivation of the second law of thermodynamics. Physical Review Letters, 86, 1927-1930. doi:10.1103/PhysRevLett.86.1927
- Terletskiy, Y. (1994) Statistical physics. Vishaya shkola, Moscow.
- Rao, Y. (2004) An introduction to thermodynamics. Universities Press, Hyderabad.
- Trupp, A. (1999) Energy, entropy: On the occasion of the 100th anniversary of Josef Loschmidt’s death in 1895: Is Loschmidt’s greatest discovery still waiting for its discovery? Physics Essays, 12, 614-628. doi:10.4006/1.3028792
- Trupp, A. (2002) Second law violations by means of a stratification of temperature due to force fields. 1st International Conference on Quantum Limits to the Second Law, 28-31 July 2002, San Diego.
- Garber, E., Brush, S. and Everitt, C. (1995) Maxwell on heat and statistical mechanics. On “avoiding all personal enquiries” of molecules. Associated University Presses, Cranbury.
- Leff, H. and Rex, A. (2003) Maxwell’s demon 2: Entropy, classical and quantum information, computing. Institute of Physics, London.
- Earman, J. and Norton, J. (1998) Exorcist XIV: The wrath of Maxwell’s demon, Part I. From Maxwell to Szilard. Studies in the History and Philosophy of Modern Physics, 29, 435-471. doi:10.1016/S1355-2198(98)00023-9
- Earman, J. and Norton, J. (1999) Exorcist XIV: The wrath of Maxwell’s demon. Part II. From Szilard to Landauer and beyond. Studies in the History and Philosophy of Modern Physics, 30, 1-40. doi:10.1016/S1355-2198(98)00026-4
- Leff, H. and Rex, A. (1990) Maxwell’s demon: Entropy, information, computing. Institute of Physics, London.
- Aristov, V. and Nikulov, A. (2002) Quantum power source putting in order of a Brownian motion without Maxwell’s demon. 1st International Symposium on Quantum Informatics, Lipki, 1-3 October 2002.
- Wang, G., Sevick, E., Mittag, E., Searles, D. and Evans, D. (2002) Experimental demonstration of violations of the second law of thermodynamics for small systems and short time scales. Physical Review Letters, 89, 050601/1- 050601/4.
- Capek, V. and Bok, J. (2001) Violation of the second law of thermodynamics in the quantum microworld. Physica A, 290, 379-401. doi:10.1016/S0378-4371(00)00345-9

