Received 28 March 2015; accepted 19 July 2015; published 22 July 2015

ABSTRACT

The constraints on the number of neutrinos generations, 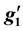coupling and 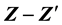mixing angle through the invisible width method, and in the framework of a 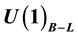model are obtained. Based on the experimental value reported by the LEP for the rate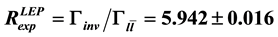, we obtained a bound on the coupling,. In addition, we derive 90% C.L. bounds on the 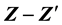mixing angle, improving the existing bounds by one order of magnitude.

Keywords:

Ordinary Neutrinos, Neutral Currents, Models beyond the Standard Model

1. Introduction

The number of fermion generations, which is associated to the number of light neutrinos, is one of the most important predictions of the Standard Model of the electroweak interactions (SM) [1] -[3] . In the SM, the decay width of the Z boson into each neutrino family is calculated to be 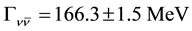[4] . Additional generations, or other new weakly interacting particles with masses below, would lead to a decay width of the Z into invisible channels larger than the SM prediction for three families while a smaller value could be produced, for example, by the presence of one or more right-handed neutrinos mixed with the left-handed ones [5] . Thus the number of light neutrinos generations, defined as the ratio between the measured invisible decay width of the Z, 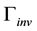, and the SM expectation for each neutrino family, needs not be an integer number and has to be measured with the highest possible accuracy.

The most precise measurement of the number of light 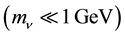active neutrino types, and therefore the number of associated fermion families, comes from the invisible Z width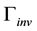, obtained by subtracting the observed width into quarks and charged leptons from the total width obtained from the lineshape. The number of effective neutrinos is given by [4]

(1)

where, the SM expression for the ratio of widths into a charged lepton and a single active neutrino, is introduced to reduce the model dependence and the experimental value for the ratio [4]

(2)

In the SM the experimental value from the four LEP experiments for the number of light neutrinos species is [4] [6] - [8] , excluding the possibility of a fourth family unless the neutrino is very heavy. This result is in agreement with cosmological constraints on the number of relativistic species around the time of Big Bang nucleosynthesis, which seems to indicate the existence of three very light neutrinos species [9] - [12] .

The existence of a heavy neutral vector boson is a feature of many extensions of the standard model. In particular, one (or more) additional gauge factor provides one of the simplest extensions of the SM. Additional gauge bosons appear in Grand Unified Theories (GUTs) [13] , Superstring Theories [14] , Left- Right Symmetric Models (LRSM) [15] - [17] and in other models such as models of composite gauge bosons [18] . In particular, it is possible to study some phenomenological features associates with this extra neutral gauge boson through of the minimal (baryon minus lepton number) extension of the SM [19] [20] , where the gauge group is given by. This model is considered as one of the candidates to study physics beyond the standard model, contain a significant set of particles and interactions whose existence could be proven both at the LHC [21] and future Linear Colliders ILC/CLIC [22] . Detailed discussions on the minimal model can be found in the literature [23] .

Our aim in this paper is to estimate constraints on the number of neutrinos generations, coupling and mixing angle through the invisible width method, and in the framework of a model.

This paper is organized as follows. In Section 2, we present the theoretical framework. In Section 3 we present the numerical computation. Finally, we summarize our conclusions in Section 4.

2. Theoretical Framework

We consider a model consisting of one doublet and one singlet and briefly describe the lagrangian including the scalar, fermion and gauge sector. The Lagrange for the gauge sector is given by [24] [25]

(3)

where, and are the field strength tensors for, and, respectively.

The Lagrangian for the scalar sector of the model is

(4)

where the potential term is [23] ,

(5)

with and as the complex scalar Higgs doublet and singlet fields, respectively. The covariant derivatives for the doublet and singlet are given by [23]

(6)

where the doublet and singlet scalars are

(7)

with, and the Goldstone bosons of, and, respectively.

From the Lagrangian of the model, the terms for the interactions between neutral gauge bosons to a pair of fermions of the SM can be written in the form

(8)

where the couplings of the bosons with the SM fermions are given by

(9)

(10)

where and is the mixing angle [23] . The current bound on this parameter is [4] . In the decoupling limit, that is to say, when and the couplings of the SM are recovered.

3. Results

In order to compare the respective expressions with the experimental result for the number of light neutrinos species, we will use the definition for in a SM analysis [26] given in Equation (1) and the LEP result for the Z invisible width [4] [6] -[8] given in Equation (2).

Since Equation (2) reduces the influence of the top quark mass, the expression is replaced. In

order to get information on the meaning of in the model we should define the corresponding expression [4] [27] - [29] .

The definition given in Equation (2) replaces the expression since (2) reduces the influence of the

top quarks mass. To get information on the meaning of in the model we should define the corresponding expression [4] [27] - [29]

(11)

where

(12)

(13)

with and, while the and couplings constant are given in Equation (9).

This new expression is a function of the mixing angle and the coupling, and in this case the quan- tity defined as the number of light neutrinos species is not a constant and not necessarily an integer. Also, in formula (11) is independent from the mass and therefore depends only of the mixing angle and the coupling of the model. Experimental values for and for are reported in the literature which, in our case, can give a bound for the mixing angle. However, we can look to those experimental numbers in another way. The partial widths and were reported recently [4] , but we use the value given by (2) for the ratio [4] . All these measurements are independent of any model and can be fitted with the parameter in terms of and.

In order to estimate a limit for the number of light neutrinos species in the framework of a model, we plot the expression (11) to see the general behavior of as a function of the mixing angle and the coupling in Figure 1.

In Figure 2, we plot the function (11) in the plane with. In this figure we show the allowed region for as a function of with 90% C.L. The allowed region is the inclined band that

is a result of both factors in Equation (11). In this figure gives the inclination while gives the

broading. This analysis was done using the experimental value given in Equation (2) for with a 90% C.L. In the same figure we show the SM result at 90% C.L. with the dashed horizontal lines. The allowed region in the model for is wider that the one for the SM, and is given by:

(14)

whose central value is quite close to the standard model of three active neutrinos species.

The correlation between the mixing angle and the coupling of the model for is presented in Figure 3. From the plot we see that there is a strong corre- lation between and.

By reversing the process we determined a limit on the coupling from the expression for the number of light neutrinos species given in Equation (11). The bound on the coupling have been obtained by using the upper bound on the mixing angle reported in the literature [4] . In Figure 4, we show the dependence of the number of light neutrinos species with respect to the coupling. Using for the mixing angle, the following limit for is obtained:

(15)

which is consistent with that obtained through from a Renormalisation Group Equation (RGE) analysis [30] - [32] .

Finally, is clear that the effects induced by the tree level and couplings in the model increase the decay widths and, and the predictions on the number of light neutrinos species are better estimated. Therefore of the coupling constant given in Equation (9) we estimated bounds on the mixing angle to different values of. The plot of this quantity as a function of is show in Figure 5. The horizontal lines give the experimental region at 90% C.L. From this figure the bounds obtained for are show in Table 1. The bounds obtained in Table 1 for the mixing angle are one order of magnitude stronger than the one obtained in the literature [4] .

4. Conclusion

From the invisible width method and and in the framework of a model, we obtained bounds on the number of light neutrinos species and on the coupling using the data published by the LEP for the rate. In addition, we get 90% C.L. bounds on the mixing angle of the model. Our results in Table 1 compare favorably and improve the existing bounds reported in the literature [4] by one order of mag- nitude. Our work complements other studies on the number of light neutrinos species, coupling and mixing angle. In the decoupling limit, that is to say, when and, we recover the bounds on N_v for the SM previously reported in the literature [4] [6] -[8] , as well as the and couplings of the SM.

Acknowledgments

We acknowledge support from CONACyT, SNI, PROMEP and PIFI (México).

Cite this paper

A.González-Sánchez,A.Gutiérrez-Rodríguez,M. A.Hernández-Ruíz, (2015) Bounds on the Number of Light Neutrinos Species, g'₁ Coupling and Z - Z′ Mixing Angle in a U(1)_B-L Model. Journal of Modern Physics,06,1077-1084. doi: 10.4236/jmp.2015.68112

References

NOTES

^*Corresponding author.