Journal of Modern Physics
Vol.2 No.1(2011), Article ID:3767,4 pages DOI:10.4236/jmp.2011.21007
Stationary Super-Gravitational States
Department of Physics the Antelope Valley College Lancaster, Lancaster, USA
E-mail: jtowe@avc.edu
Received November 20, 2010; revised December 21, 2010; accepted December 25, 2010
Keywords: Super-Gravity, Higgs events, gauge invariance, spectrum of fermions
ABSTRACT
The string background AdS7XS4 is adopted and the early universe is modeled in the eleven dimensional SUGRA theory that is dual to this background. Specifically the ground state of the vacuum is associated with an arbitrary distribution of closed, spin-2 strings, and excited states are modeled as geometric combinations of individual strings. Combinations or combining iterations are, by hypothesis, admissible or geometric if each iteration intrinsically incorporates the metrical scale that is assigned to the individual spin-2 string. It is demonstrated that a generalization of this process, if appropriately calibrated, establishes theoretical fermionic masses that correspond approximately to observed values. The proposed model also predicts a new quark of mass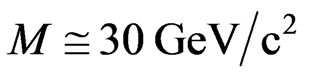 .
.
1. Introduction
A model was proposed in 2008 [1] that is based upon AdS/CFT correspondence [2] and upon the string background AdS7XS4 [3]. The 2008 model is specificallyfounded upon Osp(1/4) pure super-gravity, which is regarded as dual to AdS7XS4. The Lagrangian density
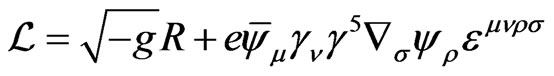 (1)
(1)
which is basic to pure super-gravity involves the superPoincare algebra
 (2)
(2)
where the generators MA are
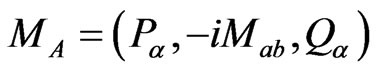 (3)
(3)
The 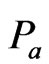 generate the translation group, the –iMab constitute the adjoint representation of the Lorentz group and the
generate the translation group, the –iMab constitute the adjoint representation of the Lorentz group and the  are components of the SUSY generator. The
are components of the SUSY generator. The 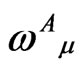 describe all connection fields:
describe all connection fields:
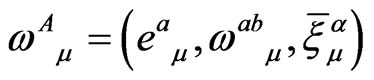 (4)
(4)
which transform under Osp (1/4) as [4]
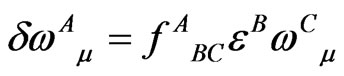 (5)
(5)
The model that was proposed in 2008 postulates that SUGRA interactions involve net absorptions of spin-2 action by the super-gravitationally interacting vacuum and that each absorption of action beyond a critical threshold results in an increment of 4-curvature that seeks the gravitational equilibrium or Friedman flatness that results from inflation. By hypothesis, each inflation event is associated with a class of gauge transformations that is intrinsic to local super-symmetry:
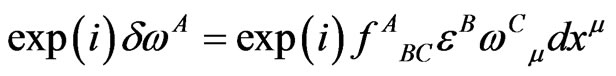 . (6)
. (6)
Moreover it is required that gauge transformations be restricted to those that preserve gauge (as in London’s extension of the Weyl theory [5,6]):
 (7)
(7)
where N = 1,2,3,… It is argued that gauge transformations that are so restricted preserve maximal Riemannian symmetry. Thus it is concluded that the galactic hierarchy as modeled is restricted to stationary super-gravitational states that are states of maximal Riemannian symmetry. The 2008 model is calibrated in terms of a large scale boundary condition that is established by observation and in this context indicates a theoretical number of galaxies (about 3.6 × 1011) which is roughly equal to that established by observation.
The model that is now proposed attempts to complement the 2008 model with a more detailed hypothesis. The absorptions of spin-2 action that are discussed in the earlier model are now identified with classes of geometric combinations which, by hypothesis, occur to arbitrary distributions of spin-2 elements.
2. Geometric Combinations as Stationary SUGRA States
The proposed hypothesis identifies spin-2 elements as closed, spin-2 strings and adopts the metrical scale, S, on each individual string:
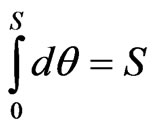 . (8)
. (8)
The ground state of the vacuum is identified with arbitrary distributions of the postulated strings and excited states are associated with geometric combinations of closed, spin-2 strings. A combination or combining iteration is, by hypothesis, admissible or geometric if that iteration intrinsically incorporates the metrical scale that is assigned to the individual spin-2 string:
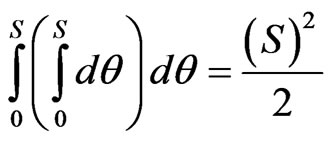 . (9)
. (9)
It is argued that a generalization of the combination (9) produces N admissible combining iterations:
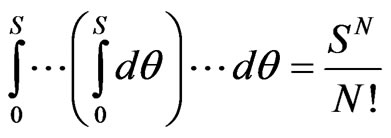 (10)
(10)
N = 1,2,3,… It will now be argued that the iterations described by (10), if appropriately calibrated, approximate the spectrum of fermionic masses.
3. Calibration of the Proposed Model
The states that are modeled by (10) are interpreted as excited vacuum states in a SUGRA GUT theory; i.e. as excited spin-2 states that participate in SUGRA GUT interactions. It is assumed that SUGRA GUT interactions involve only quark-lepton (and lepton-quark) transitions; e.g. it is assumed that SUGRA GUT interactions preserve I3 and generation. Accordingly the expression (10) is calibrated in terms of the most massive composite state that reflects these specifications:
 , (11)
, (11)
where  represents an LH field of spin-2, where
represents an LH field of spin-2, where  is an LH top quark and where
is an LH top quark and where 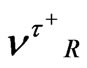 is an RH anti-tauon’s neutrino. Specifically the calibration of (10) will adopt the metric S = [180(GeV/c2)]1/6 on the individual closed, spin-2 string, so that (10) becomes
is an RH anti-tauon’s neutrino. Specifically the calibration of (10) will adopt the metric S = [180(GeV/c2)]1/6 on the individual closed, spin-2 string, so that (10) becomes
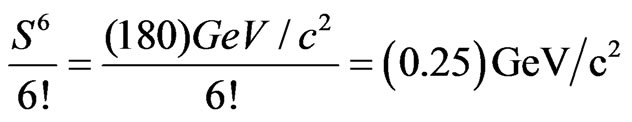 (12)
(12)
or
 . (13)
. (13)
It is argued that simultaneous divisions of the sides of (13) by the indicated integers: 6, 5, 4, 3 and 2 represent additional geometrically admissible increments of vacuum excitation. Specifically the division of (13) by six produces
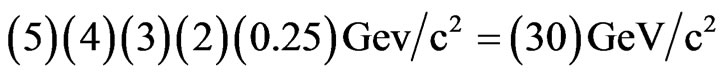 , (14)
, (14)
which is identified as the approximate mass of a spin-2 state that has not been observed. Expression (14) is interpreted as the composite spin-2 state: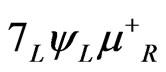 , where “7” represents a new quark and
, where “7” represents a new quark and 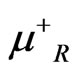 is an RH antimuon. Thus the proposed model predicts a new quark of mass
is an RH antimuon. Thus the proposed model predicts a new quark of mass 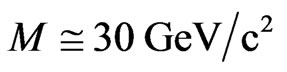 (thereby exposing the proposed model to experimental falsification).
(thereby exposing the proposed model to experimental falsification).
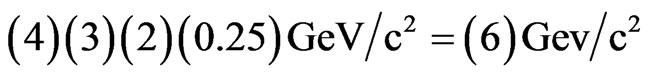 , (15)
, (15)
Both sides of (14) are now divided by five to produce
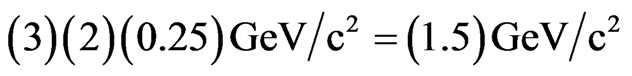 , (16)
, (16)
which is interpreted as the approximate mass of a composite spin-2 state  or
or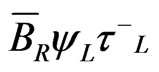 , where BL is an LH bottom quark (a mass of about 4.3 GeV/c2), where
, where BL is an LH bottom quark (a mass of about 4.3 GeV/c2), where  is an RH anti-bottom, where
is an RH anti-bottom, where 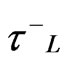 is an LH tauon and where
is an LH tauon and where 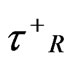 is an RH anti-tauon (a mass of about 1.7 GeV/c2). Continuing, both sides of (15) are divided by four to produce
is an RH anti-tauon (a mass of about 1.7 GeV/c2). Continuing, both sides of (15) are divided by four to produce
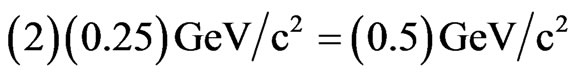 , (17)
, (17)
which is interpreted as the approximate mass of a composite spin-2 field  or
or 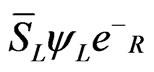 where
where 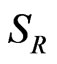 and
and 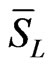 respectively represent the RH strange quark and the LH anti-strange and where
respectively represent the RH strange quark and the LH anti-strange and where 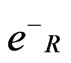 and
and 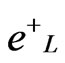 respectively represent the RH electron and the LH anti-electron. Finally, both sides of (17) are divided by two to produce
respectively represent the RH electron and the LH anti-electron. Finally, both sides of (17) are divided by two to produce
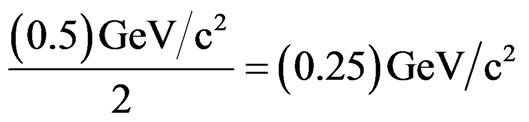 , (18)
, (18)
which is interpreted as the approximate mass of the quark-lepton pair that is obtained from the averaging of those I3 states that contain the (almost equally massive) up quark and down quark. The masses that are indicated by expressions (14) through (18) are approximately equal to those determined by observation [7,8].
The averaging of the two lepto-quark states that include the up quark and down quark (producing the mass (18)) clearly results in a state for which I3 = 0. Thus the existence (and averaging) of an equal number of leptoquark states for which I3 = +1/2 and I3 = –1/2 admits a context in which expressions (13) through (18) can be regarded as a partial or partially broken symmetry of quantized increments of mass scale that preserve metrical scale. The former can be depicted as increments of rotation and the latter as a constant radius of the partially broken symmetry (see Figure 1).
By the preceding discussion each vertex of Figure 1 associates with a spin-2 composite. By super-symmetry, there is a second symmetry, also consisting of six vertices, which is isomorphic to the Figure 1 symmetry. The vertices of this second symmetry are regarded as representing spin-(3/2) super-partners of the spin-2 composites that are associated with the Figure 1 vertices. Super-partners that are characterized by a common value of I3 and by a common generation are interpreted as constituting a stationary super-gravitational state. Thus I3 and generation (as well as super-symmetry) are preserved by super-gravitational interactions which occur among super-partners that constitute a stationary super-gravitational state. Such interactions are exemplified by the following (see Figure 2).
The notation 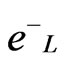 represents a left-handed electron,
represents a left-handed electron, 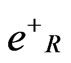 represents an RH anti-electron and
represents an RH anti-electron and 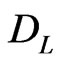 is an LH down quark.
is an LH down quark.
4. Conclusions
Traditionally it has been difficult to compare models based upon string backgrounds and dual field theories with physical reality. However the model that was proposed by this author in 2008, in which multiple inflation events are identified with gauge preserving phase transformations on SUGRA connections appears to provide a

Figure 1. Broken lepto-quark symmetry.

Figure 2. A first order model of SUGRA GUT interactions.
credible model of large scale structure. Specifically this model is calibrated in terms of a large scale boundary condition that is established by observation and in this context indicates a theoretical number of galaxies (about 3.6 × 1011) which is roughly equal to that established by observation.
The model that is now proposed complements the above described large scale model by appropriating scale preservation in the micro-domain. This model introduces scale preserving combinations that occur to arbitrary distributions of spin-2 elements, which are identified as closed spin-2 strings. Combinations are, by hypothesis, admissible or geometric if each combining iteration intrinsically incorporates the metrical scale of the previous iteration (this is the metrical scale that is initially assigned to the individual spin-2 string). It is shown that generalizations of this process, if appropriately calibrated, establish theoretical mass scales that approximate the masses of known lepto-quark states and predict a new quark of mass  30 GeV/c2. Again therefore, physical structure is derived from a model that is based upon a string background and super-gravity.
30 GeV/c2. Again therefore, physical structure is derived from a model that is based upon a string background and super-gravity.
It appears that the events described by expression (10) cumulatively absorb one dimension for each value of the quantum number N. Thus additional research is needed to determine the topological and geometric properties of the internal manifold K6 which is indicated by this compactification (which may indicate possibilities for generalization). Some insights may emerge from the following observations: Since the initially adopted SUGRA model is characterized by N = 1 super-symmetry and since the manifold M4 is (by the author’s 2008 model) maximally symmetric, it appears that the internal manifold K6 is Ricci flat. Secondly, a holonomy group may be constituted by a more general category of SUGRA interactions that do not necessarily conserve I3 and generation but do conserve super-symmetry. If the proposed, super-symmetric versions of the Figure 1 symmetry are regarded as a single symmetry, then the appropriated, general category of interactions provides mechanisms of transition from one vertex to another. In this general context, SUGRA interactions that are restricted to stationary SUGRA states cumulatively constitute an identity operation. Moreover, it can be established that preservation of super-symmetry by interactions that transcend vertices requires a triplet holonomy. These topological and geometric specifications approximate the topology and geometry of a popular compactification scheme in terms of the proposed model. In this more general context higher order diagrams (integrals) may provide a more precise description of fermionic masses.
REFERENCES
- J. Towe, “The Gauge Invariant Spectrum of Local Super-Symmetry as the Universe that is Observed,” International Journal of Theoretical Physics, Vol. 47, No. 11, 2008, pp. 2898-2903. doi:10.1007/s10773-008-9722-1
- E. D’Hoker and D. Freedman, “Super-Symmetric Gauge Theories and the AdS/CFT Correspon-Dance,” arXiv: hep-th/020123, 2002.
- O. Aharony, S. Gubser, J. Maldacena, H. Ooguri and Y. Oz, “Large N Theories, String Theory and Gravity,” arXiv: hep-th/9905111, 1999.
- M. Kaku, “Quantum Field Theory,” Oxford University Press, New York, 1993, pp. 692-696.
- H. Weyl, “Space, Time and Matter,” Metheun and Co. Ltd., London, 1922.
- F. London, “Quantenmechanische Deutung die Theorie von Weyl,” Zeitschrift für Physik, Vol. 42, No. 5-6, 1927, pp. 375-389. doi:10.1007/BF01397316
- SLAC Virtual Visitor Center, SLAC National Accelerator Laboratory, Menlo Park, CA, 2009.
- R. Bock, at CERN, Geneva and A. Vasilesca, at IFA, Bucuresti, Particle Detecto Brief Book, 1999.

