Applied Mathematics
Vol.06 No.04(2015), Article ID:55895,5 pages
10.4236/am.2015.64064
On the Derivation and Implementation of a Four Stage Harmonic Explicit Runge-Kutta Method*
Ashiribo Senapon Wusu1, Moses Adebowale Akanbi1, Bakre Omolara Fatimah2
1Department of Mathematics, Lagos State University, Lagos, Nigeria
2Department of Mathematics, Federal College of Education (Technical), Lagos, Nigeria
Email: wussy_ash@yahoo.com, akanbima@gmail.com, larabakre@gmail.com
Copyright © 2015 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 19 January 2015; accepted 22 April 2015; published 23 April 2015
ABSTRACT
In recent times, the derivation of Runge-Kutta methods based on averages other than the arithmetic mean is on the rise. In this paper, the authors propose a new version of explicit Runge-Kutta method, by introducing the harmonic mean as against the usual arithmetic averages in standard Runge-Kutta schemes.
Keywords:
Explicit, Harmonic, Runge-Kutta, Autonomous

1. Introduction
During the last few decades, there has been a growing interest in problem solving systems based on the Runge- Kutta methods. Several methods have been developed using the idea different means such as the geometric mean, centroidal mean, harmonic mean, contra-harmonic mean and the heronian mean.
In previous papers [1] and [2] , the authors presented a three stage method based on the harmonic mean and a multi-derivative method using the usual arithmetic mean respectively. Akanbi [3] developed a third-order method based on the geometric mean. In [4] and [5] , the concept of the heronian mean was introduced. Evans and Yaacob [6] introduced a fourth-order method based on the harmonic mean while Yaacob and Sanugi [7] also developed a fourth-order method which is an embedded method based on the arithmetic and harmonic mean. Wazwaz [8] presented a comparison of modified Runge-Kutta methods based on varieties of means. Using the definition of the harmonic mean, a fourth-order Runge-Kutta method is developed and implemented.
2. Derivation of the 4sHERK Method
The schemes introduced by [7] and [9] respectively are
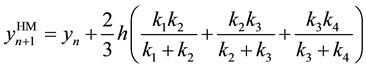 (1)
(1)
where
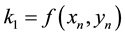

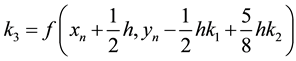
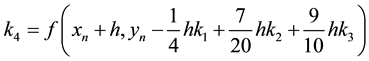
and
 (2)
(2)
where
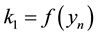
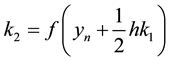
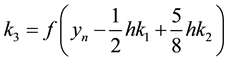
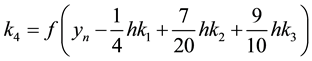
Scheme (2) was referred to as RK-HM-AM. Using the definition of harmonic mean, the following scheme is proposed in this paper:
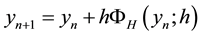 (3)
(3)
where,
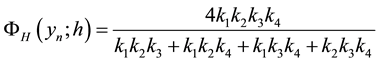 (4)
(4)

 (5)
(5)
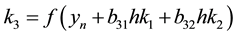 (6)
(6)
 (7)
(7)
where 

The expansion of 




Substituting (8), (9) and (10) into (4) and simplifying the resulting expression using MATHEMATICA (version 8.0.1) package, the coefficients of the powers of h in (4) are compared with that of the Taylors’ expansion of 

Thus, the incremental function (4) of the proposed scheme is

and the proposed scheme (3) is

where



3. Stability of the 4sHERK Method
For the analysis of the absolute stability of the proposed 4sHERK scheme, the scalar test problem 






Substituting (17)-(20) in (3) and simplifying the resulting expression results in,

Letting 


The absolute stability region of the 4sHERK scheme is given in Figure 1.
4. Error Estimation
Definition: The local truncation error at 

And 
Using the above definition together with (12), the local truncation error (LTE) of the proposed scheme is given as

Figure 1. Absolute stability region of the 4sHERK method.
where 
5. Numerical Experiments
Consider the IVP

with the theoretical solution

Table 1. h =0.125, 


Table 2. h = 0.1, 


Table 3. h = 0.01, 


We apply the new 4sHERK method (13) to the above IVP and the results obtained are compared with the classical 4-stage fourth-order Runge-Kutta method and the methods of [6] and [4] .
The results generated by the newly derived scheme in this paper evidently proved the extent of accuracy of the scheme in comparison with the other methods.
6. Conclusion
Evidently, the newly derived scheme is more accurate as seen from the computational results presented in Table 1, Table 2 and Table 3, since its absolute error is the least of all the methods presented in this paper. It therefore follows that the scheme is quite efficient. We therefore conclude that the 4sHERK method proposed is reliable, stable and with high accuracy in computation.
References
- Wusu, A.S., Okunuga, S.A. and Sofoluwe, A.B. (2012) A Third-Order Harmonic Explicit Runge-Kutta Method for Autonomous Initial Value Problems. Global Journal of Pure and Applied Mathematics, 8, 441-451.
- Wusu, A.S. and Akanbi, M.A. (2013) A Three-Stage Multiderivative Explicit Runge-Kutta Method. American Journal of Computational Mathematics, 3, 121-126.
- Akanbi, M.A. (2011) On 3-Stage Geometric Explicit Runge-Kutta Method for Singular Autonomous Initial Value Problems in Ordinary Differential Equations. Computing, 92, 243-263.
- Evans, D.J. and Yaacob, N.B. (1995) A Fourth Order Runge-Kutta Method Based on the Heronian Mean Formula. International Journal of Computer Mathematics, 58, 103-115.
- Evans, D.J. and Yaacob, N.B. (1995) A Fourth Order Runge-Kurla Method Based on the Heronian Mean. International Journal of Computer Mathematics, 59, 1-2.
- Evans, D.J. and Yaacob, N.B. (1993) A New Fourth Order Runge-Kutta Formula Based on Harmonic Mean. Department of Computer Studies, Loughborough University of Technology, Loughborough.
- Yaacob, N. and Sanugi, B. (1998) A New Fourth-Order Embedded Method Based on the Harmonic Mean. Matematica, Jilid, 1998, 1-6.
- Wazwaz, A.M. (1994) A Comparison of Modified Runge-Kutta Formulas Based on Variety of Means. International Journal of Computer Mathematics, 50, 105-112.
- Sanugi, B.B. and Evans, D.J. (1993) A New Fourth Order Runge-Kutta Method Based on Harmonic Mean. Computer Studies Report, Louborough University of Technology, UK.
- Lambert, J.D. (1973) Computational Methods in ODEs. Wiley, New York.
NOTES
*Four Stage Harmonic Runge-Kutta Method.






