Applied Mathematics
Vol.4 No.7A(2013), Article ID:34101,6 pages DOI:10.4236/am.2013.47A007
Mild Solutions of Fractional Semilinear Integro-Differential Equations on an Unbounded Interval
Department of Mathematics, University of Carthage, Bizerte Preparatory Engineering Institute, Jarzouna, Tunisia
Email: jawahdou@gmail.com
Copyright © 2013 Adel Jawahdou. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Received May 12, 2013; revised June 14, 2013; accepted June 24, 2013
Keywords: Semilinear Integrodifferential Equations; Mild Solutions; Schauder Fixed Point Theorem
ABSTRACT
In this paper, we study the existence of mild solutions for fractional semilinear integro-differential equations in an arbitrary Banach space associated with operators generating compact semigroup on the Banach space. The arguments are based on the Schauder fixed point theorem.
1. Introduction
The purpose of the present paper is to present an alternative approach to the existence of solution of fractional semilinear integro-differential equations in an arbitrary Banach space 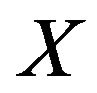 of the form
of the form
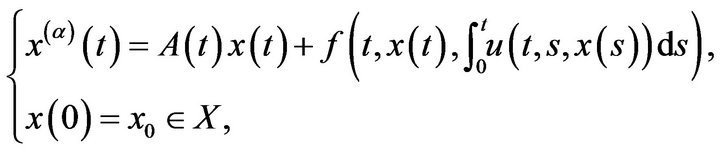 (1)
(1)
where  and
and 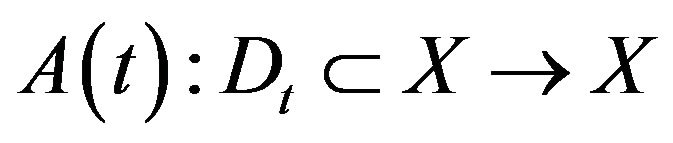 generates an evolution system
generates an evolution system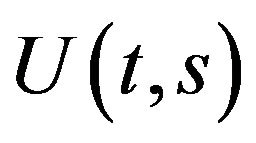 , satisfying:
, satisfying:
• 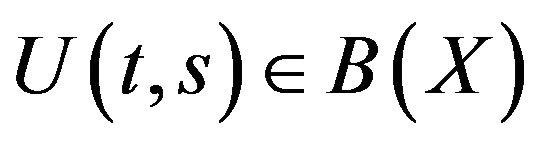 , where
, where 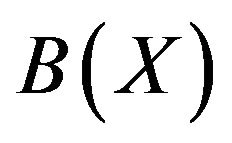 denotes the Banach space of bounded linear operators from
denotes the Banach space of bounded linear operators from 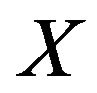 into
into 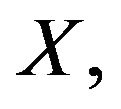
• 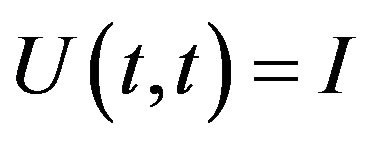 (
(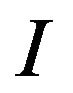 is the identity operator in
is the identity operator in )•
)• 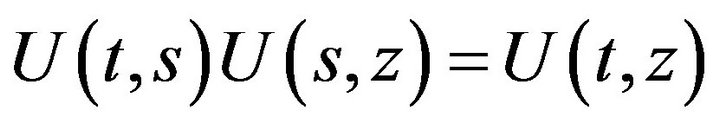 for
for 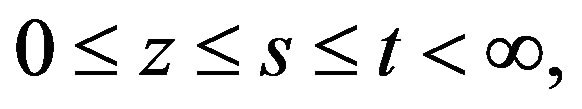
• the mapping 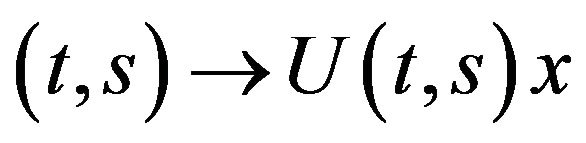 is strongly continuous in
is strongly continuous in 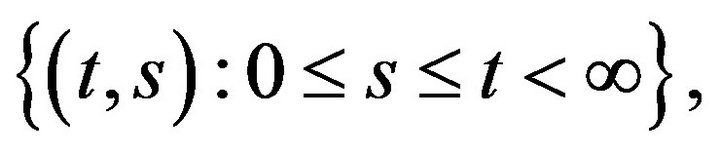 and
and 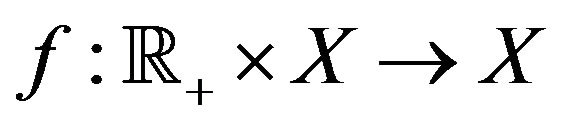 is a given function.
is a given function.
Differential equations of fractional order have recently proved to be valuable tools in the modeling of many phenomena in various fields of science and engineering. This equations also serve as an tool for the description of hereditary properties of various materials and processes. For details, see [1-5]. The most important problem examined up to now is that concerning the existence of solutions of considered equations. In order to solve (1), many different methods have been applied in the literature. Most of these methods use the notion of a measure of noncompactness in Banach spaces, see [6-10]. Such a method can be to apply in this work. The method we are going to use is to reduce the existence of mild solutions of fractional semilinear integro-differential equations of type (0.1) to searching a fixed points of a suitable map on the space 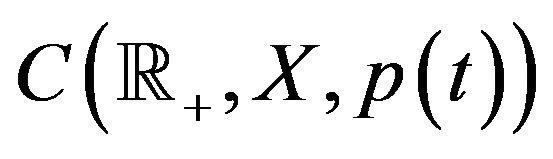 tempered by an arbitrary positive real continuous function
tempered by an arbitrary positive real continuous function 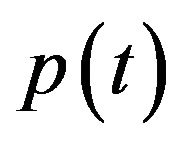 defined on
defined on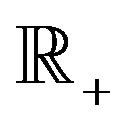 . In order to prove the existence of fixed points, we shall rely on the Schauder theorem. Moreover, an application to fractional differential equations is provided to illustrate the results of this work.
. In order to prove the existence of fixed points, we shall rely on the Schauder theorem. Moreover, an application to fractional differential equations is provided to illustrate the results of this work.
2. Preliminary Tools
In what follows, 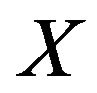 will represent a Banach space with norm
will represent a Banach space with norm . Denote by
. Denote by 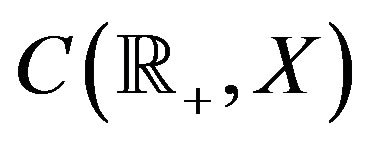 the space of continuous functions
the space of continuous functions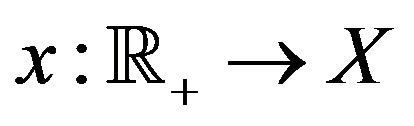 . Now, let us assume thet
. Now, let us assume thet 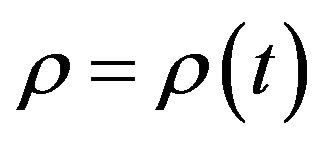 is a given function defined and continuous on the interval
is a given function defined and continuous on the interval 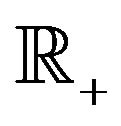 with real positive values. Denote by
with real positive values. Denote by
 the Banach space consisting of all functions
the Banach space consisting of all functions 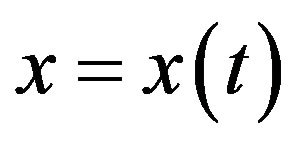 defined and continuous on
defined and continuous on 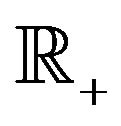 with values in the Banach space
with values in the Banach space 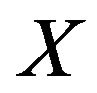 such that
such that
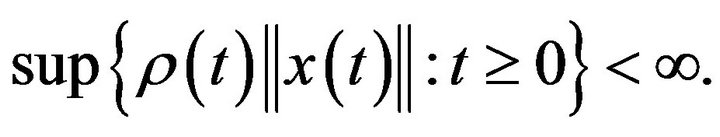
The space 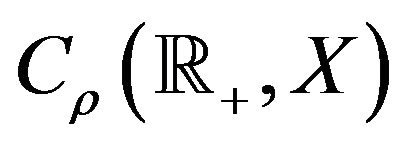 is furnished with the following standard norm
is furnished with the following standard norm
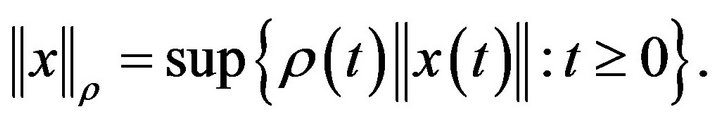
Let us recall two facts:
• The convergence in 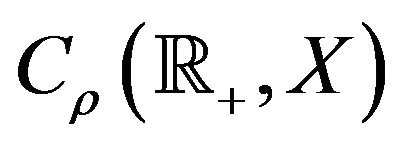 is the uniform convergence in the compact intervals, i.e.
is the uniform convergence in the compact intervals, i.e. 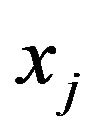 converge to
converge to 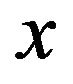 in
in 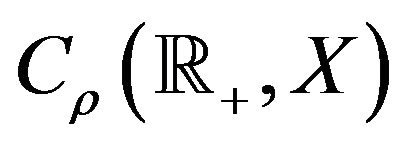 if and only if
if and only if 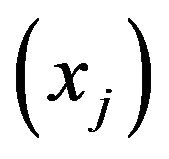 is uniformly convergent to
is uniformly convergent to 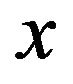 on compact subsets of
on compact subsets of 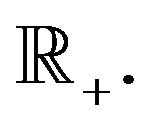
• A subset 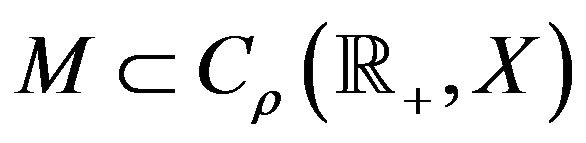 is relatively compact if and only if the restrictions to
is relatively compact if and only if the restrictions to 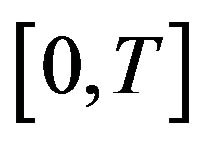 of all functions from X form an equicontinuous set for each
of all functions from X form an equicontinuous set for each 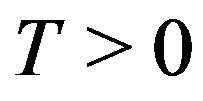 and
and 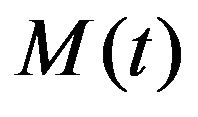 is relatively compact in
is relatively compact in 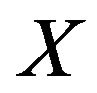 for each
for each 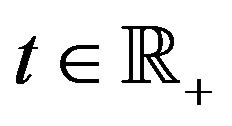 ,where
,where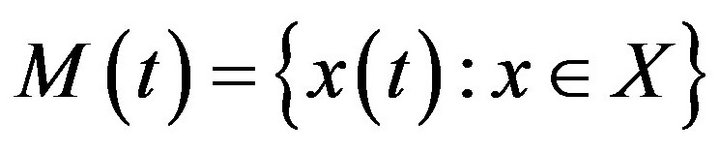 , See [11].
, See [11].
Definition 1 A nonempty subset 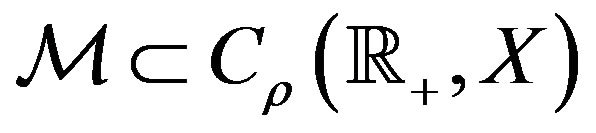 is said to be bounded if the there is a function
is said to be bounded if the there is a function 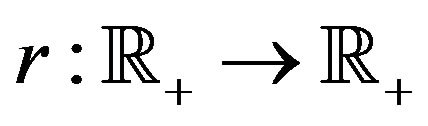 such that
such that 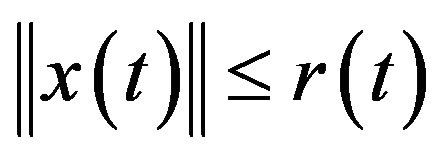 for each
for each 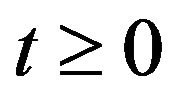 and
and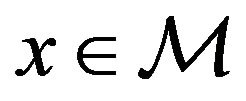 .
.
Namely, denote by 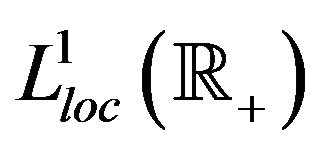 the space of real functions defined and Lebesgue integrable on
the space of real functions defined and Lebesgue integrable on 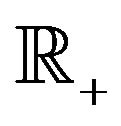 and equiped with the standard norm. For
and equiped with the standard norm. For 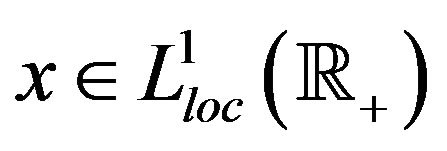 and for a fixed number
and for a fixed number 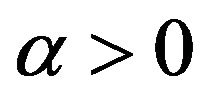 we define the Riemann-Liouville fractional integral of order
we define the Riemann-Liouville fractional integral of order 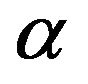 of the function
of the function 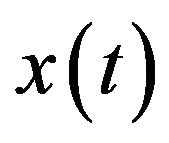 by putting
by putting
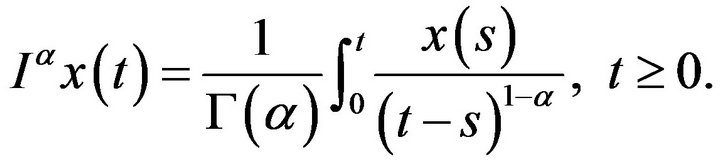
It may be shown that the fractional integral operator  transforms the space
transforms the space 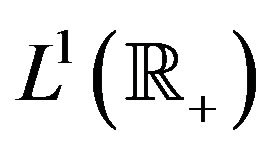 into itself and has some other properties (see [6-8], for example). More generally, we can consider the operator
into itself and has some other properties (see [6-8], for example). More generally, we can consider the operator 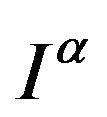 on the function space
on the function space 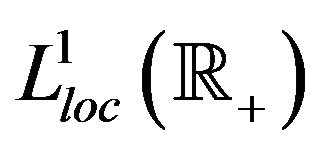 consisting of real functions being locally integrable over
consisting of real functions being locally integrable over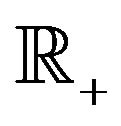 .
.
The following result is well known, one can see Michalski [12]
Lemma 1 For all 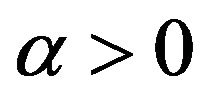 and
and .
.
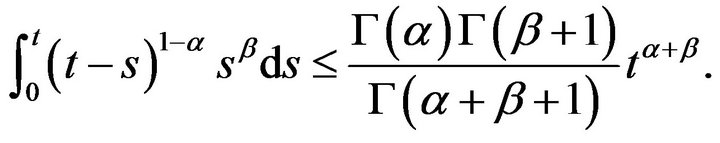 (2)
(2)
Our consideration is based on following Schauder fixed point theorem.
Theorem 1 [13] Let 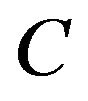 be a closed convex subset of the Banach space
be a closed convex subset of the Banach space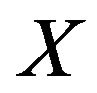 . Suppose
. Suppose 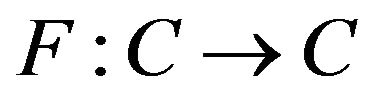 and
and 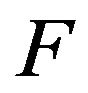 is compact (i.e., bounded sets in
is compact (i.e., bounded sets in  are mapped into relatively compact sets). Then,
are mapped into relatively compact sets). Then, 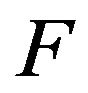 has a fixed point in
has a fixed point in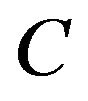 .
.
3. Existence of Mild Solutions
The following hypotheses well be needed in the sequel.
• (A)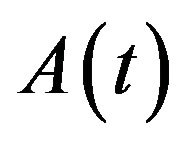 is a bounded linear operator on
is a bounded linear operator on 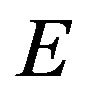 for each
for each 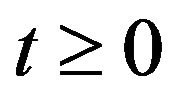 and generates a uniformly continuous evolution system
and generates a uniformly continuous evolution system 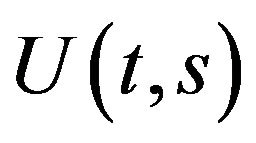 such that
such that
• 
• (Cf) (i)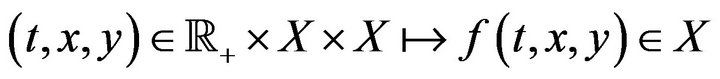 satisfies the Caratheodory type conditions, i.e.
satisfies the Caratheodory type conditions, i.e. 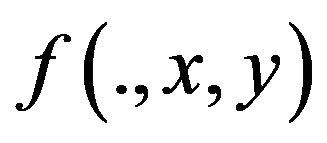 is measurable for
is measurable for 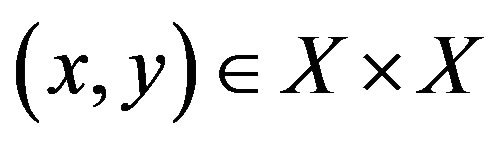 and
and 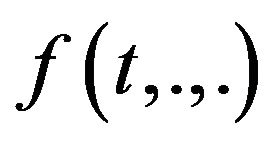 continuous for a.e.
continuous for a.e.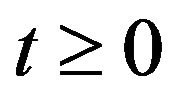 , (ii) there exists a continuous positive function
, (ii) there exists a continuous positive function 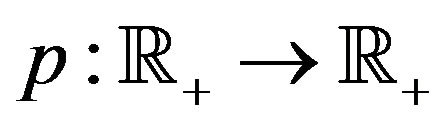 such that
such that
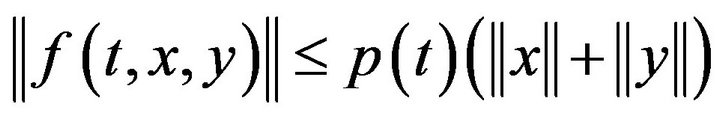
for a.e. 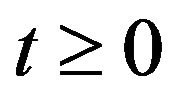 and all
and all .
.
• (Cu) (i) 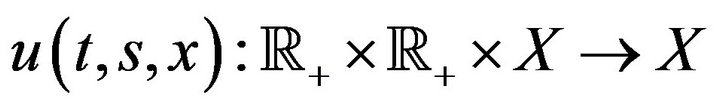 is continuous on
is continuous on
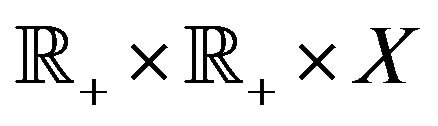 • (ii)
• (ii) 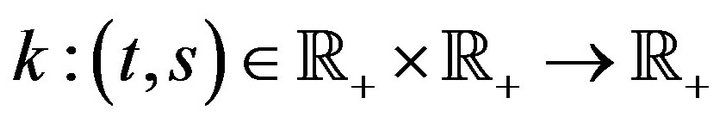 being continuous such that
being continuous such that
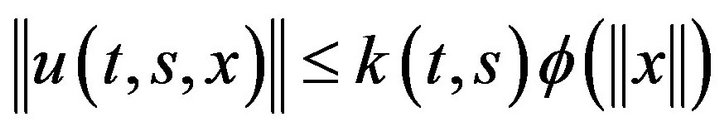 where
where 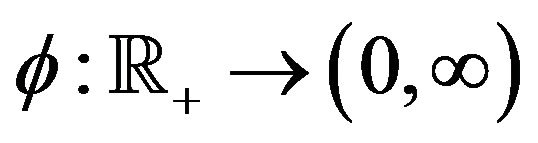 is continuous and increasing function with
is continuous and increasing function with
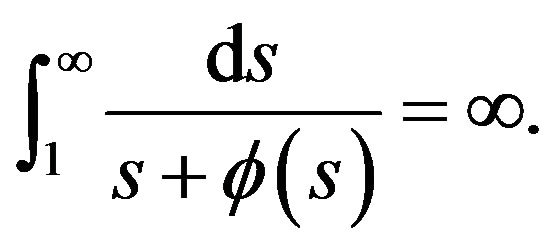 (3)
(3)
• (iii) For all positive function 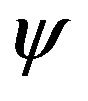 there exist
there exist 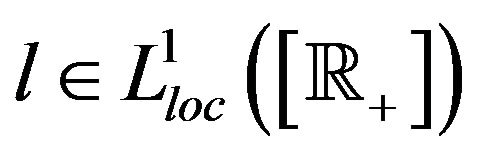 such that
such that
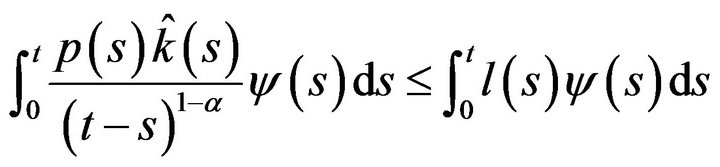 .
.
where 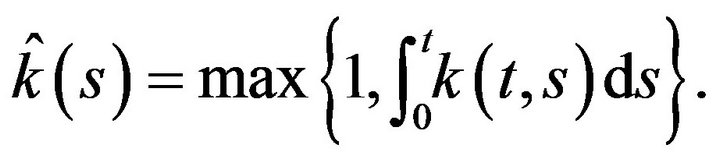
Definition 2 [14] A continuous function  is said to be a mild solution of (0.1) if
is said to be a mild solution of (0.1) if  satisfies to
satisfies to
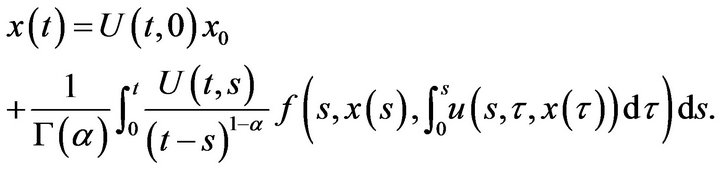
Our main result is given by the following theorem.
Theorem 2 If the Banach space 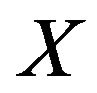 is separable.
is separable.
Assume that the hypotheses 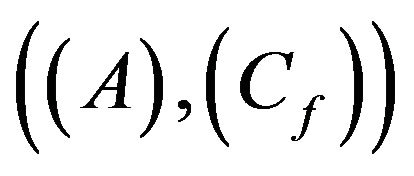 and
and 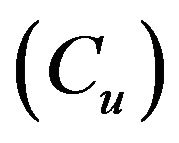 are satisfied. Then for each
are satisfied. Then for each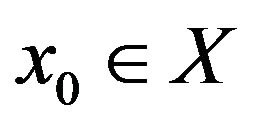 , the problem (0.1) has at least one mild solution
, the problem (0.1) has at least one mild solution 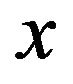 in
in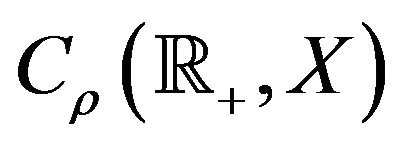 , for
, for 
Proof. Consider the operator 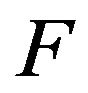 defined by the formula
defined by the formula
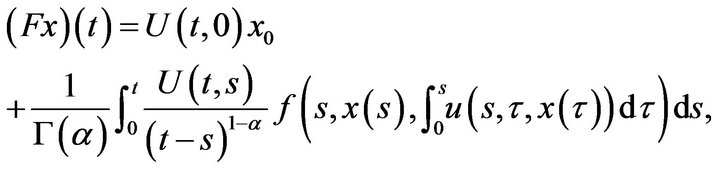 (4)
(4)
for 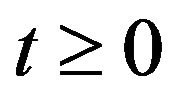 and
and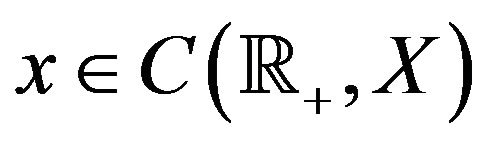 . Let
. Let
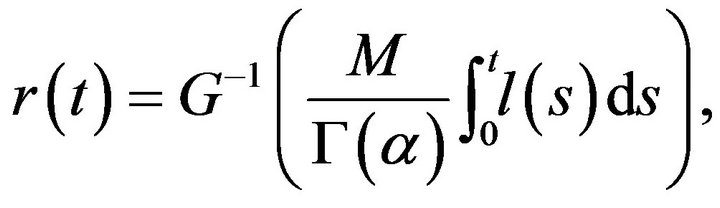
where
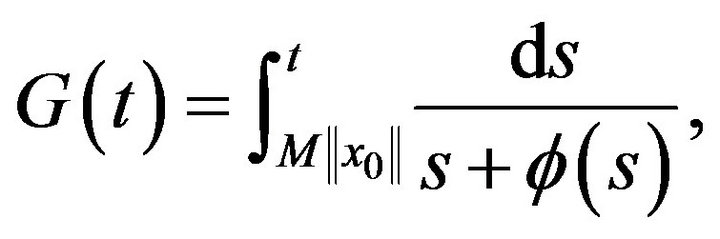
and
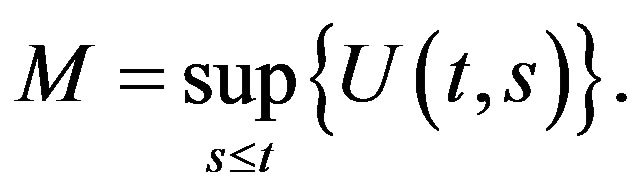
The estimate (0.3) guarantee the convergence of the integral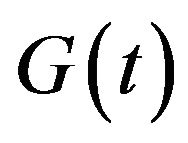 . In the other hand, observe that if
. In the other hand, observe that if 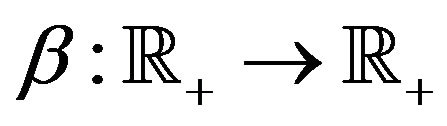 is nondecreasing function, then the function
is nondecreasing function, then the function 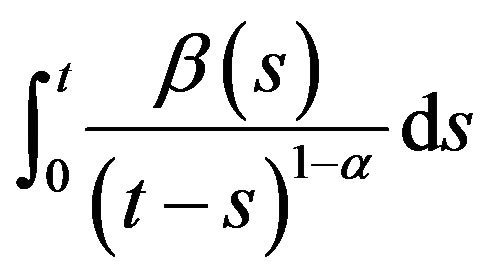 is also nondecreasing on
is also nondecreasing on .Therefore, the function
.Therefore, the function 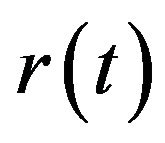 is will defined and nondecreasing on
is will defined and nondecreasing on  Next, put
Next, put
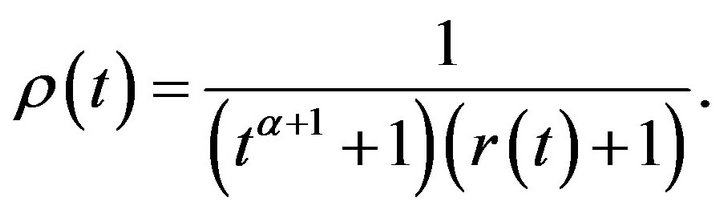 (5)
(5)
Obviously, the function 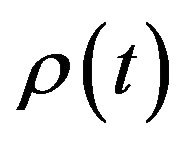 is continuous, positive and decreasing. In the space
is continuous, positive and decreasing. In the space 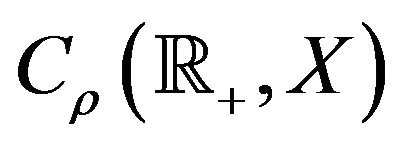 let us consider the set
let us consider the set
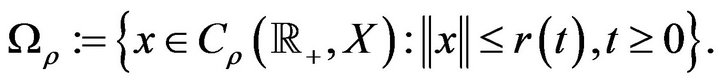 (6)
(6)
Clearly 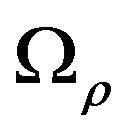 is closed convex of
is closed convex of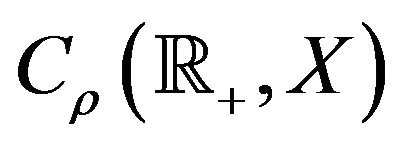 . Next, let
. Next, let 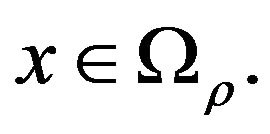 Applying assumptions
Applying assumptions 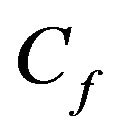 (1) and
(1) and 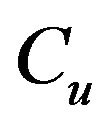 (2) we have
(2) we have
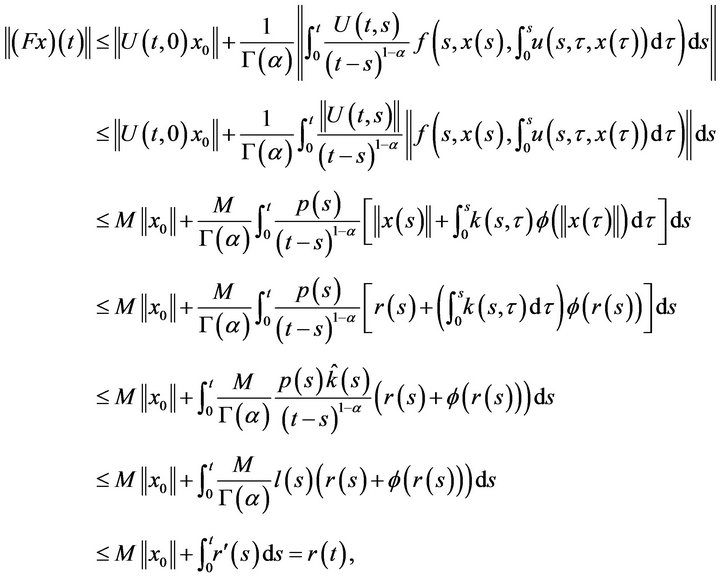 (7)
(7)
From the estimate (7), we deduce that 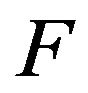 transforms
transforms 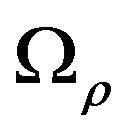 into itself. In what follows we show that
into itself. In what follows we show that 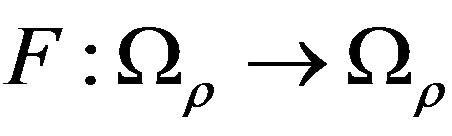 is continuous. To do this, let us fix
is continuous. To do this, let us fix 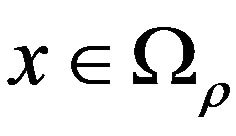 and take arbitrary sequence
and take arbitrary sequence 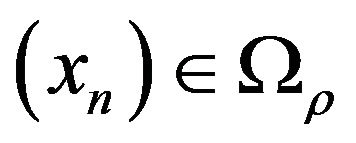 such that
such that 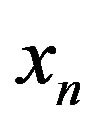 converge to
converge to 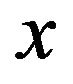 in
in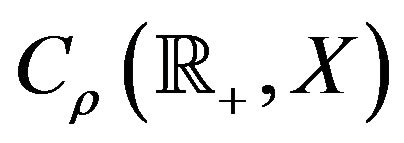 . Further, let us fix
. Further, let us fix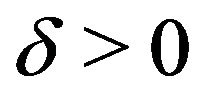 . Applying the properties of
. Applying the properties of 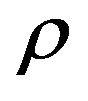 and
and 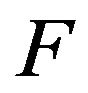 we get
we get
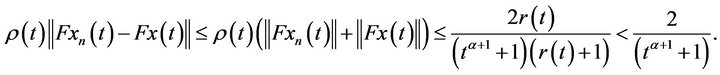
Then, keeping in mind that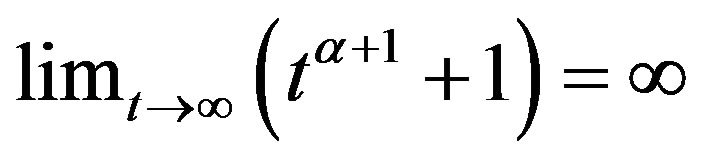 , we obtain, that there exists
, we obtain, that there exists  so big that
so big that
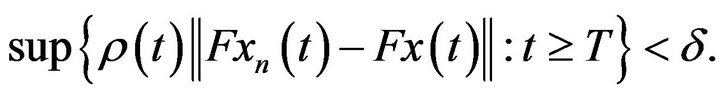 (8)
(8)
Next, for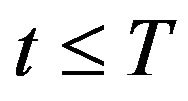 , denote
, denote 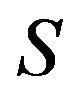 the operator defined by
the operator defined by
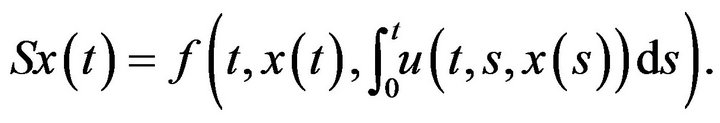
For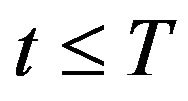 , we have,
, we have,
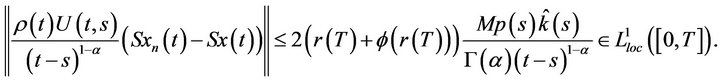
Next, by the Lebesgue dominated convergence theorem and (0.8) we derive that for suitable large  we have
we have 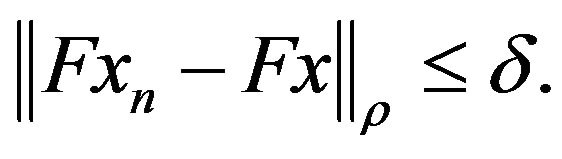 this fact proves that
this fact proves that  is continuous on
is continuous on .
.
Next, from (**) we see that to prove the compactness of , we should prove that
, we should prove that 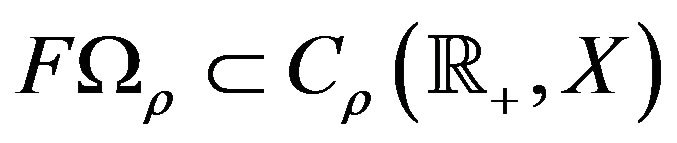 is equicontinuous on
is equicontinuous on 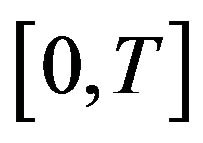 and
and 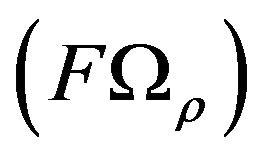 is relatively compact for each
is relatively compact for each 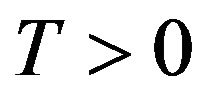 and
and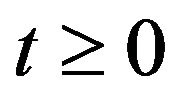 . For any
. For any 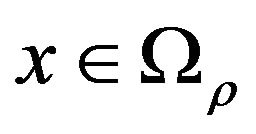 and
and 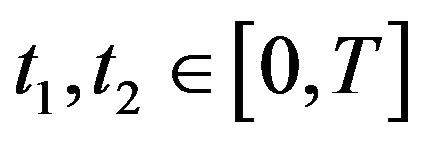
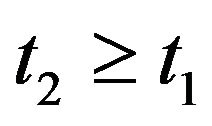 we get,
we get,
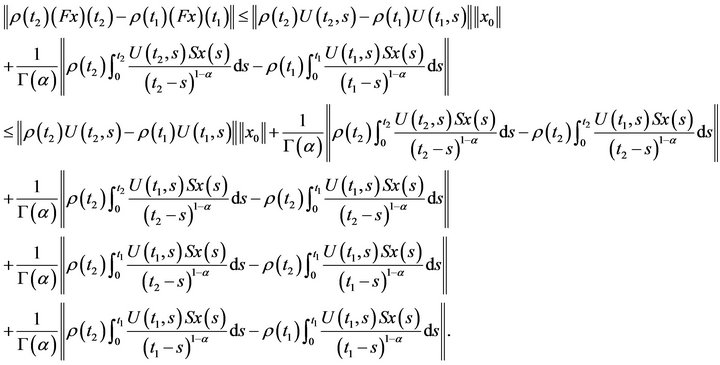
Thus,
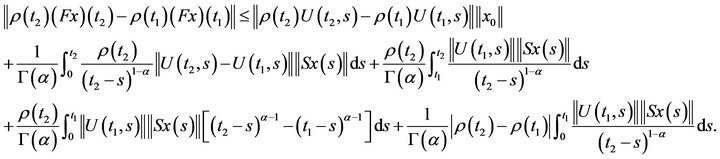 (9)
(9)
Observe that for any 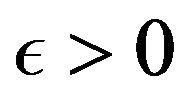 there exists
there exists 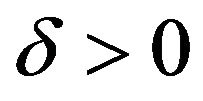 such that
such that 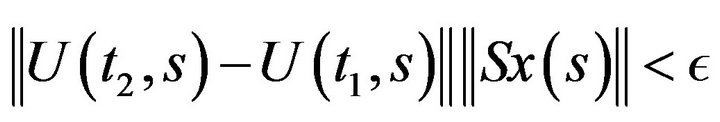 for all
for all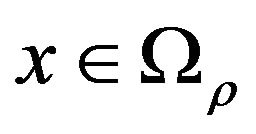 ,
,
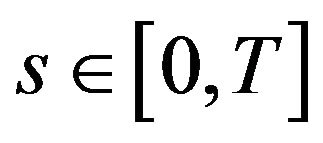 and
and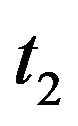 ,
, 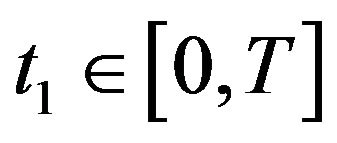 such that
such that 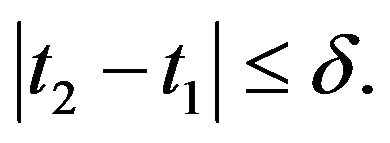
Then, by the monotonicity of 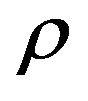 and for all
and for all 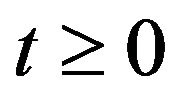
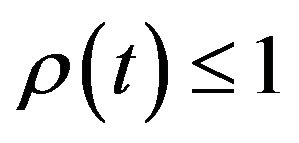 , we get
, we get
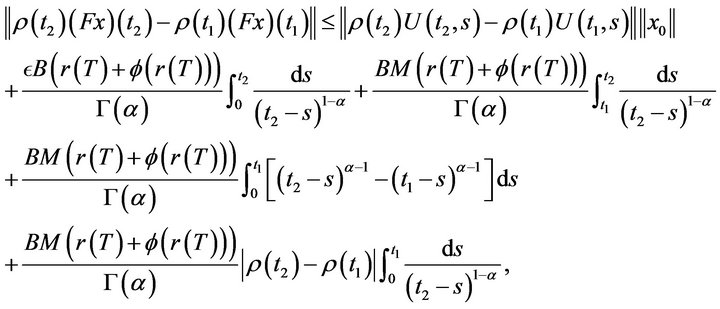 (10)
(10)
where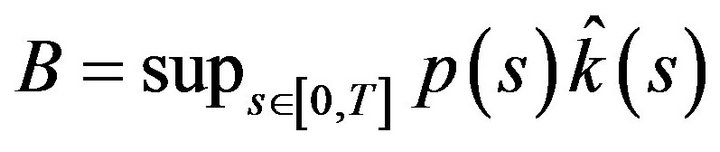 . Keeping in mind the continuity of
. Keeping in mind the continuity of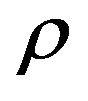 , the right-hand side of the above inequality tends to zero as
, the right-hand side of the above inequality tends to zero as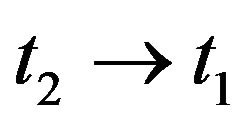 .
.
• If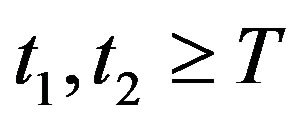 , then we have
, then we have
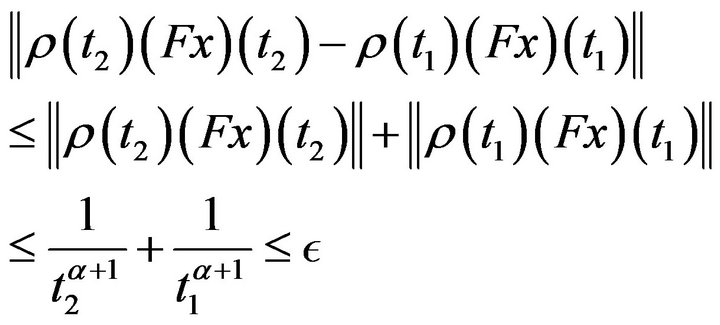 (11)
(11)
• If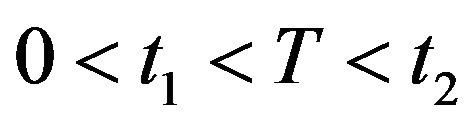 , note that
, note that 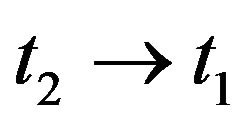 implies that
implies that  and
and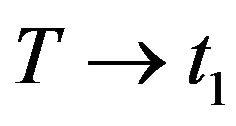 . According to the above results, we have
. According to the above results, we have
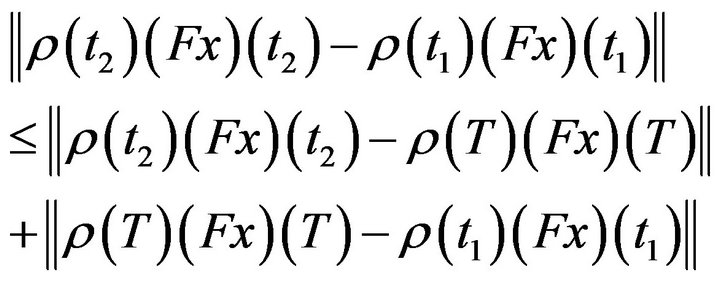 (12)
(12)
converging to 0 as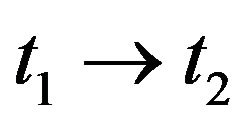 .
.
So for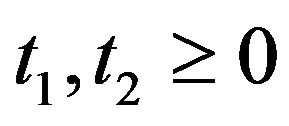 ,
, 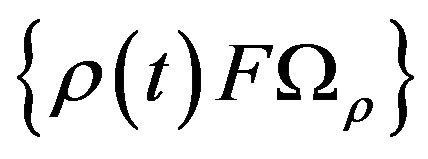 is equicontinuous. Meanwhile,
is equicontinuous. Meanwhile, 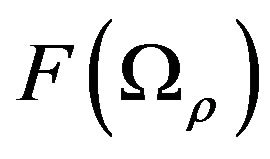 is relatively compact because that
is relatively compact because that 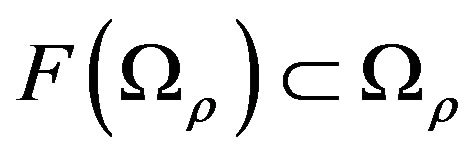 is uniformly bounded. Thus
is uniformly bounded. Thus  is completely continuous on
is completely continuous on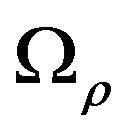 . By Schauder fixed point theorem, we deduce that
. By Schauder fixed point theorem, we deduce that 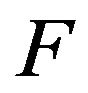 has a fixed point
has a fixed point  in
in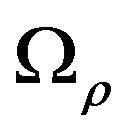 .
.
The last result in this article is to prove the existence of solutions to (0.1) but with the following conditions.
• 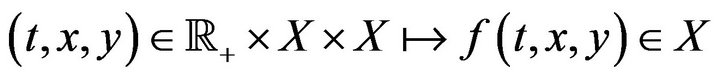 satisfies the Caratheodory type conditions, i.e.
satisfies the Caratheodory type conditions, i.e. 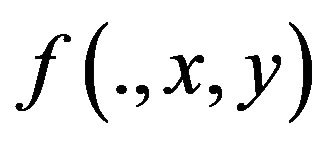 is measurable for
is measurable for 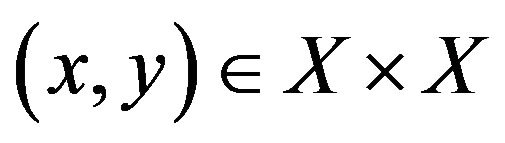 and
and 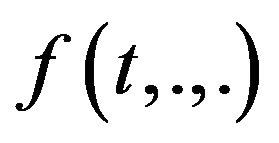 continuous for a.e.
continuous for a.e.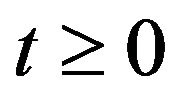 •
• 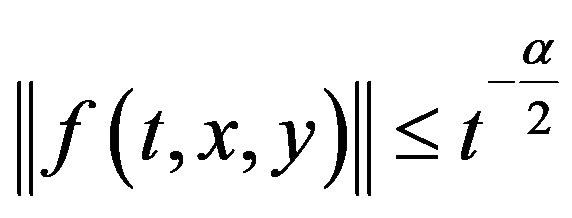 for a.e.
for a.e. 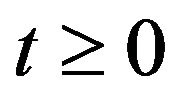 and all
and all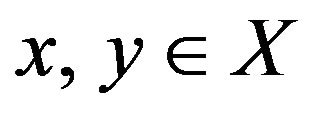 .
.
Theorem 3 If the Banach space 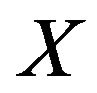 is separable. Assume that the hypotheses
is separable. Assume that the hypotheses  and
and 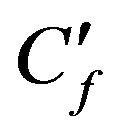 are satisfied. Then for each
are satisfied. Then for each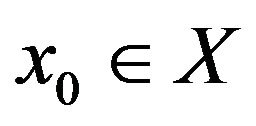 , the problem (0.1) has at least one mild solution
, the problem (0.1) has at least one mild solution 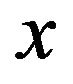 in
in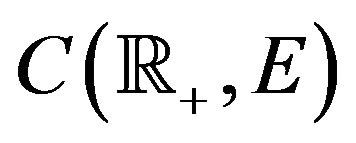 , for
, for 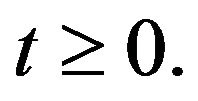
Proof. Define the operator  by:
by:
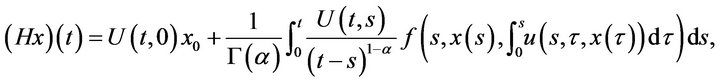 (13)
(13)
for 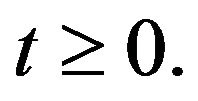 Kipping in mind the result of lemma (0.2), we get, for
Kipping in mind the result of lemma (0.2), we get, for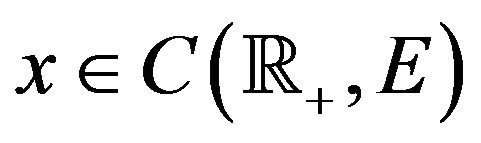 .
.
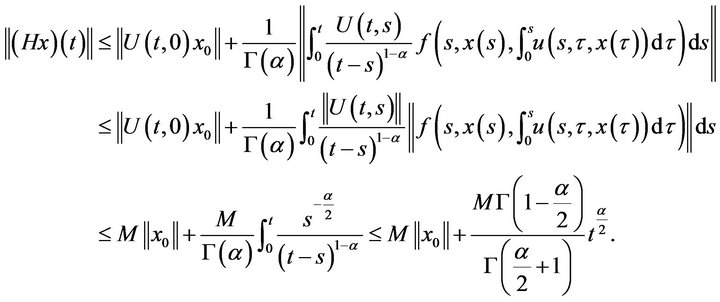 (14)
(14)
Put 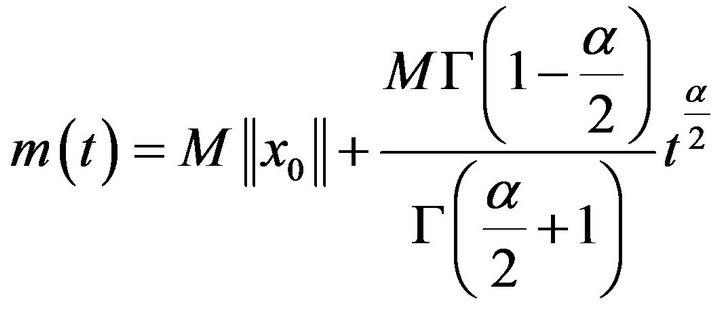 and
and
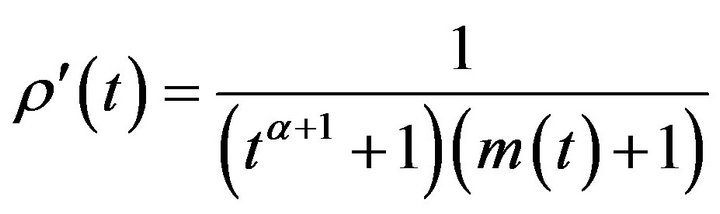
Next, define the set
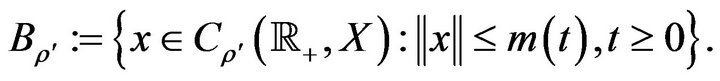 (15)
(15)
Then, we have that  is a self-mapping of
is a self-mapping of . We omit the proof of continuity
. We omit the proof of continuity  and
and  is relatively compact, because are similar to that in Theorem 2.
is relatively compact, because are similar to that in Theorem 2.
4. Example
In this section, we illustrate the main result contained in Theorem 2 by the following quadratic fractional differential equation
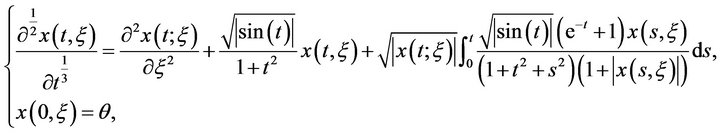 (16)
(16)
for 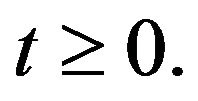 Let
Let 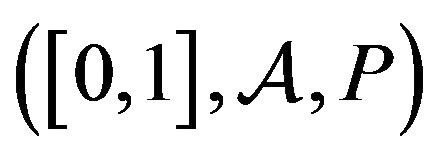 be a complete probability measure space. Let
be a complete probability measure space. Let 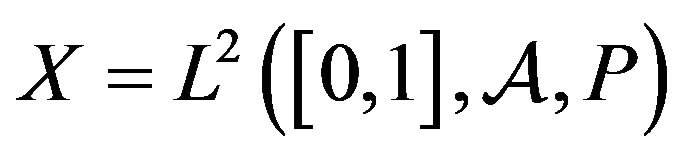 the space of
the space of
 -measurable maps
-measurable maps 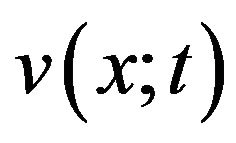 with
with
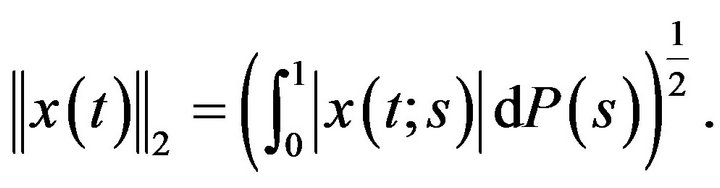
Consider the operator
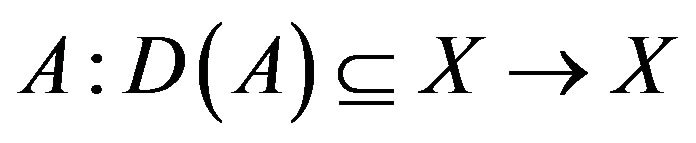
defined by
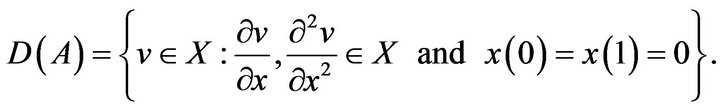
Put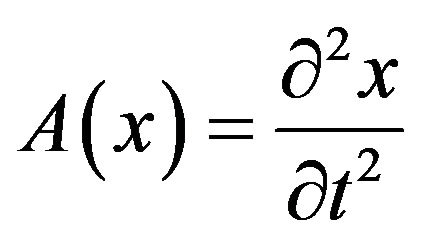 .
.
Clearly  is densely defined in
is densely defined in 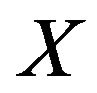 and is the infinitesimal generator of a strongly continuous semigroup
and is the infinitesimal generator of a strongly continuous semigroup
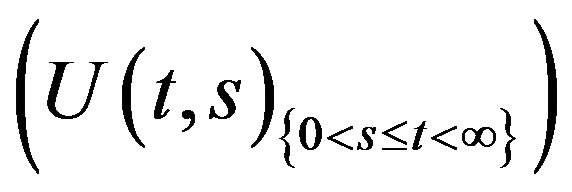 in
in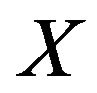 . Observe that the above equation is a special case of Equation (1) if we put
. Observe that the above equation is a special case of Equation (1) if we put 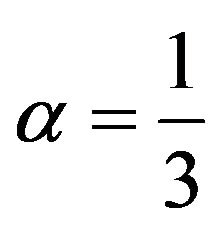 and
and
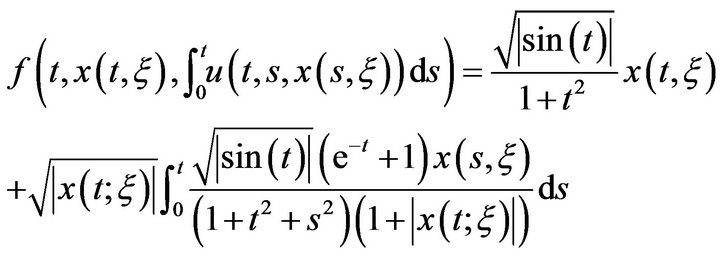
Bay using the Jensen’s inequality it is not difficult to see that
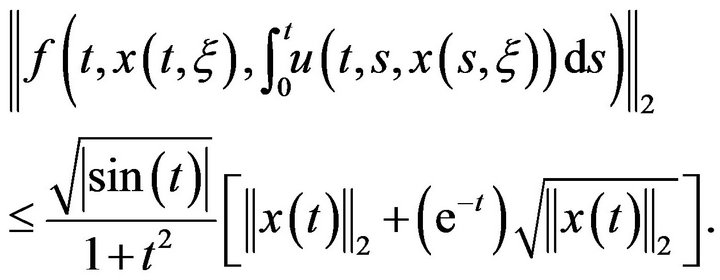
To check conditions 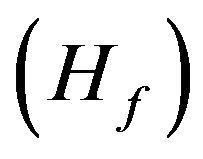 and
and 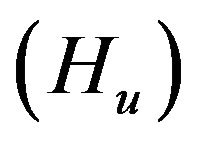 it is enough to take
it is enough to take
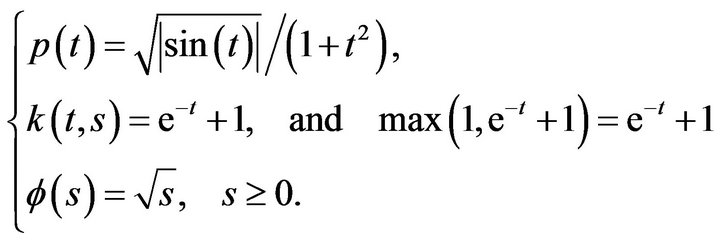
Let be a positive function 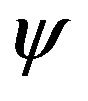 defined on
defined on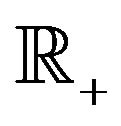 .
.
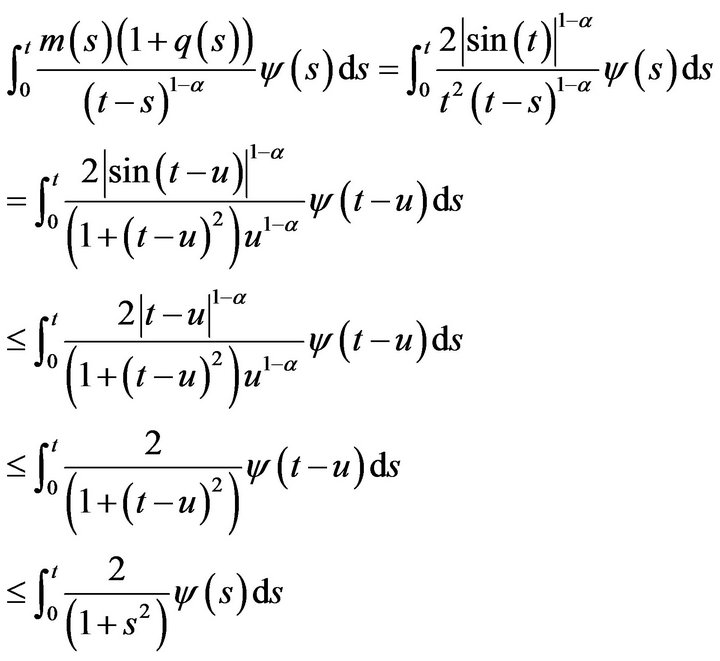
Thus, on the basis of Theorem 2, we conclude that Equation (4.1) has at least one mild solution in the space
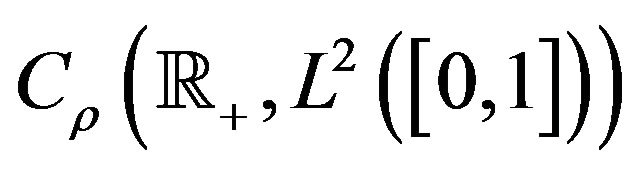 .
.
5. Acknowledgements
I thank the referee for their invaluable advices, comments and suggestions.
REFERENCES
- R. P. Agarwal, M. Benchohra and S. A. Hamani, “Survey on the Existence Results for Boundary Value Problems of Nonlinear Fractional Differential Equations and Inclusions,” Acta Applicandae Mathematicae, Vol. 109, No. 3, 2010, pp. 973-1033. 0Hdoi:10.1007/s10440-008-9356-6
- M. benchohra, J. Henderson and S. K. Ntouyas, “Impulsive Differential Equations and inclusions,” Hindawi Publishing Corporation, New York, 2006.
- M. Benchohra and B. A. Slimani, “Existence and Uniqueness of Solutions to Impulsive Fractional Differential Equations,” Electronic Journal of Differential Equations, Vol. 10, 2009, pp. 1-11.
- L. Byszewski, “Theorems about the Existence and Uniqueness of Solutions of a Semilinear Evolution Nonlocal Cauchy Problem,” Journal of Mathematical Analysis and Applications, Vol. 162, No. 18, 1991, pp. 494-505. 1Hdoi:10.1016/0022-247X(91)90164-U
- A. Karczewska and S. Wedrychowicz, “Existence of Mild Solutions for Semilinear Equation of Evolution,” Commentationes Mathematicae Universitatis Carolinae, Vol. 37, No. 4, 1996, pp. 695-706.
- J. Banas and K. Goebel, “Measures of Noncompactness in Banach Spaces, Lecture Notes in Pure and Applied Mathematics,” Marcel Dekker, New York, 1980.
- J. Banas, “On Existence Theorems for Differential Equations in Banach Spaces,” Bulletin of the Australian Mathematical Society, Vol. 32, No. 01, 1985, pp. 73-82. 2Hdoi:10.1017/S0004972700009734
- A. Jawahdou, “Mild Solutions of Functional Semilinear Evolution Volterra Integrodifferential Equations on an Unbounded Interval,” Nonlinear Analysis: Real World Applications, Vol. 74, No. 18, 2011, pp. 7325-7332. 3Hdoi:10.1016/j.na.2011.07.050
- A. Jawahdou and A. Karoui, “Monotonic Solutions of Nonlinear Integral Equations of Fractional Order,” Canadian Applied Mathematics Quarterly, Vol. 18, No. 1, 2010, pp. 41-58.
- L. Olszowy and S. Wedrychowicz, “Mild Solutions of Semilinear Evolution Equation on an Unbounded Interval and Their Applications,” Nonlinear Analysis: Real World Applications, Vol. 72, No. 3, 2010, pp. 2119-2126. 4Hdoi:10.1016/j.na.2009.10.012
- L. V. Kantorovich and G. P. Akilov, “Functional Analysis in Normed Spaces,” Pergamon Press, Oxford, 1982.
- M. W. Michalski, “Derivatives of Noninteger Order and Their Applications. Dissertationes Mathematicae, Polska Akademia Nauk,” Instytut Matematyczny, Warszawa, 1993.
- C. Corduneanu, “Integral Equations and Applications,” Cambridge University Press, New York, 1990.
- M. H. M. Rashid and Y. El-Qadri, “Semilinear Fractional Integro-Differential Equations with Compact Semigroup,” Nonlinear Analysis: Real World Applications, Vol. 71, No. 12, 2009, pp. 6276-6282. 5Hdoi:10.1016/j.na.2009.06.035

