Applied Mathematics
Vol. 3 No. 2 (2012) , Article ID: 17392 , 4 pages DOI:10.4236/am.2012.32024
Wronskian and Grammian Solutions for Generalized (n + 1)-Dimensional KP Equation with Variable Coefficients
1Normal College, Jinhua Vocational and Technique College, Jinhua, China
2Department of Mathematics, Zhejiang Normal University, Jinhua, China
Email: fhw5645@163.com
Received December 15, 2011; revised February 1, 2012; accepted February 8, 2012
Keywords: Generalized Variable Coefficient (n + 1)-Dimensional KP Equation; Hirota Bilinear Method; Wronskian Solution; Grammian Solution
ABSTRACT
The generalized (n + 1)-dimensional KP equation with variable coefficients is investigated in this paper. The bilinear form of the equation has been obtained by the Hirota direct method. In addition, with the help of Wronskian technique and the Pfaffian properties, Wronskian and Grammian solutions have been generated.
1. Introduction
Recently, there has been a growing interest in studying variable-coefficient nonlinear evolution equations (NLEEs). Quite a few researchers studied the variable-coefficient KP equations [1-3], which provides us with more realistic models in such physical situations as the canonical and cylindrical cases, propagation of surface waves in large channels of varying width and depth with nonvanishing vorticity and so on. In this paper, we consider the generalized variable-coefficient (n + 1)-dimensional KP equation
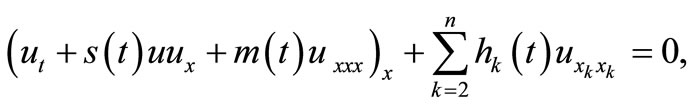 (1)
(1)
where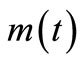 ,
, 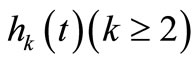 are arbitrary functions with respect to t. Equation (1) can be reduced to the (3 + 1)-dimensional KP equation
are arbitrary functions with respect to t. Equation (1) can be reduced to the (3 + 1)-dimensional KP equation
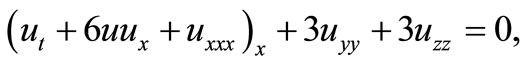 (2)
(2)
by setting
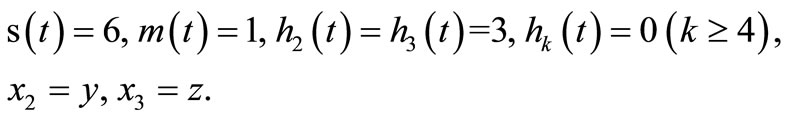
Equation (2) describes the dynamics of solitons and nonlinear waves in plasmas physics and fluid dynamics. Obviously, (1) is the generalization of (2).
It is well known that the bilinear method first proposed by Hirota provides us with a comprehensive approach to construct exact solutions [4-6]. Once a NLEE is written in bilinear form, we are able to derive systematically particular solutions including the multi-soliton solutions. Soliton solutions can also be written in Wronskian form, which was first introduced by Satsuma in 1979 [7]. Freeman and Nimmo developed the Wronskian technique, which admits direct verifications of solutions in Wronskian form to the bilinear equations [8]. It is noted that Grammian is another type of solution representation for soliton equations, which can be rewritten as a Pfaffian and the proof can easily be completed by virtue of Pfaffian properties [9,10].
The organization of the paper is as follows. In Section 2, based on the Hirota bilinear method, we obtain the bilinear forms of (1). Then the Wronskian and Grammian solutions of (1) are derived in Sections 3 and 4, respectively. Finally, the conclusions and discussions will be given in Section 5.
2. Bilinear Form of (1)
By the dependent variable transformation
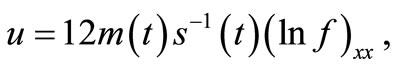 (3)
(3)
equation (1) can be transformed into the following bilinear form:
 (4)
(4)
where the Hirota bilinear operators 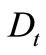
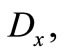 and
and 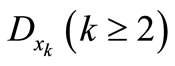 are defined by
are defined by
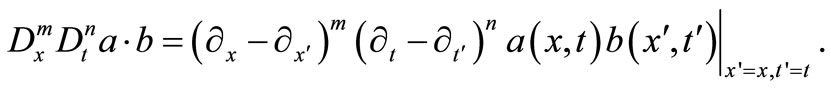 (5)
(5)
Equation (4) can be rewritten as
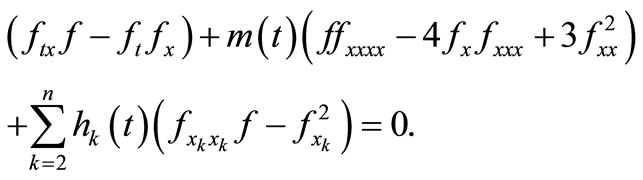 (6)
(6)
3. Wronskian Solution of (1)
In this section, the N-soliton solutions of (1) in Wronskian form have been generated.
Theorem 1. Equation (4) has the solution in terms of the Wronskian determinant
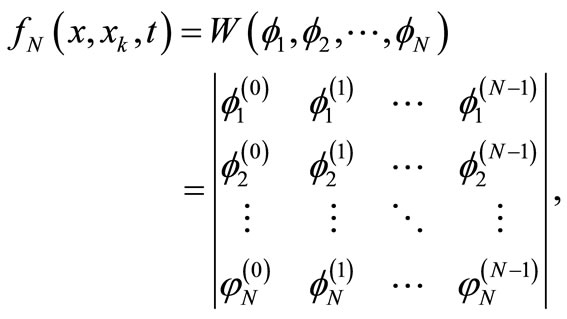 (7)
(7)
where 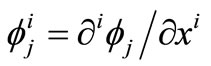 and
and 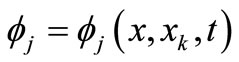 satisfy the set of linear partial differential equations
satisfy the set of linear partial differential equations
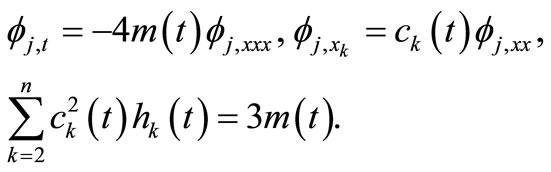 (8)
(8)
Proof. To conveniently write (7), we adopt the compact notation
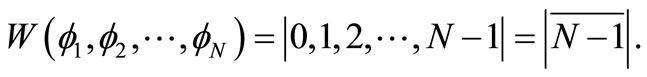 (9)
(9)
Under the properties of the Wronskian determinant and the conditions (8), we obtain
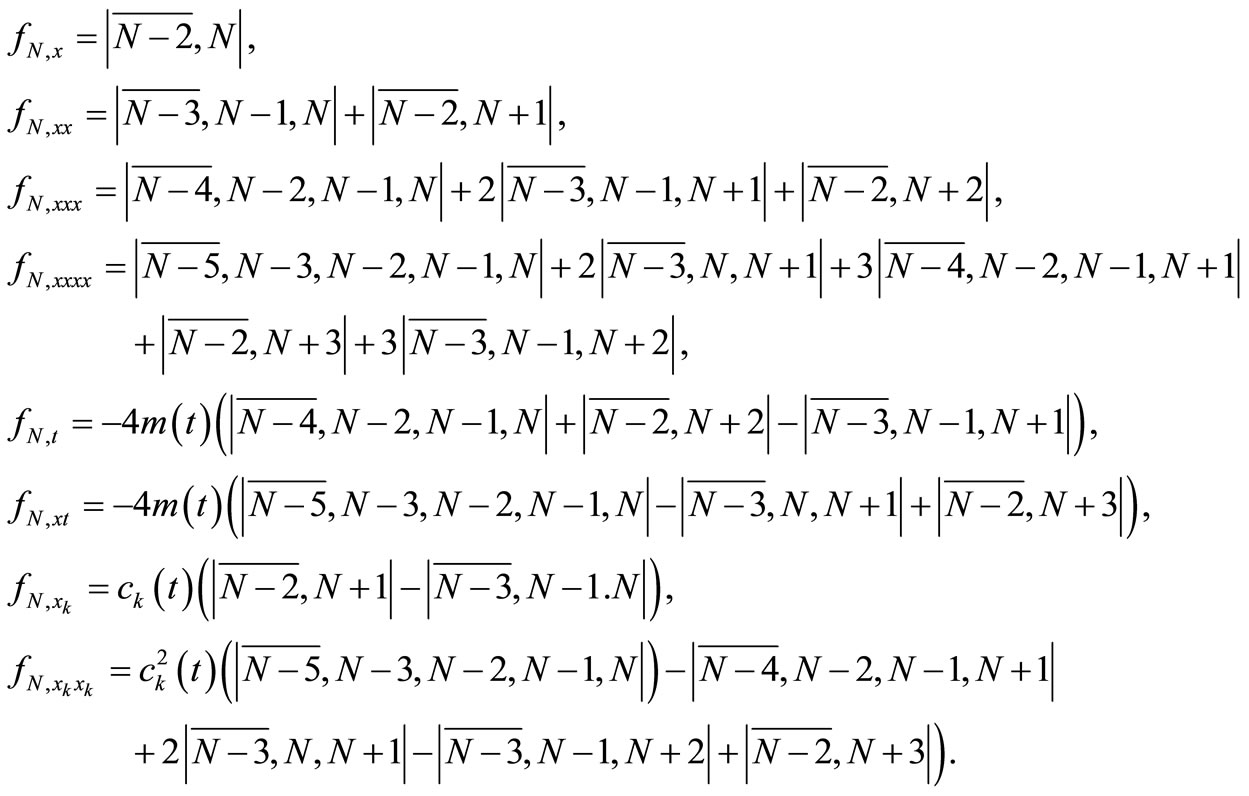 (10)
(10)
Substituting these derivatives into (6), the left side becomes
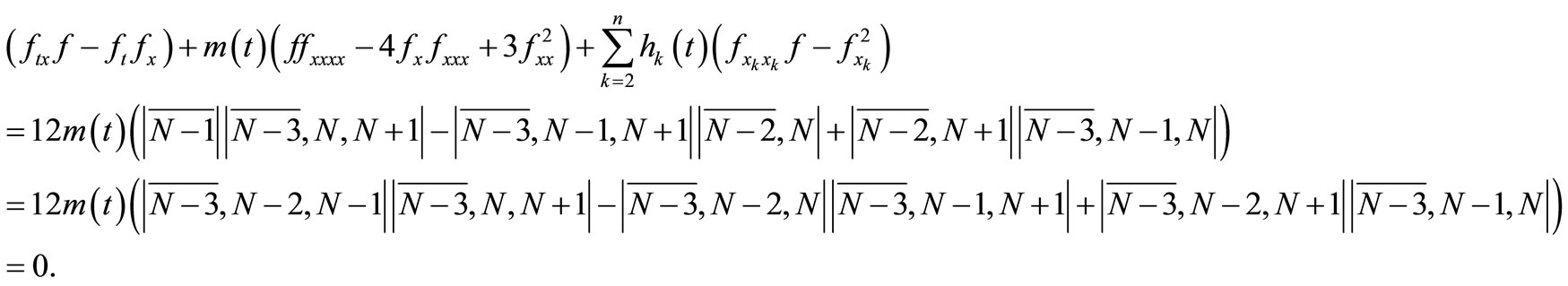 (11)
(11)
Thus, we have the N-soliton solutions of (1) in Wronskian form
 (12)
(12)
where 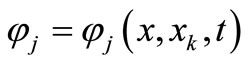 satisfies the conditions (8).
satisfies the conditions (8).
4. Grammian Solution of (1)
In what follows, we focus on the Grammian type solution and construct a broad set of sufficient conditions which make the Grammian determinant a solution of the bilinear Equation (4).
Theorem 2. Equation (4) has the Grammian solution as follows:
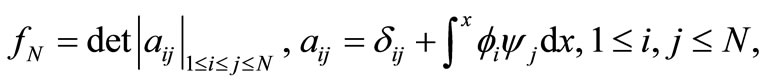 (13)
(13)
where the functions 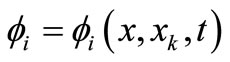 and
and 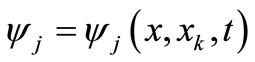 satisfy the two sets of conditions
satisfy the two sets of conditions
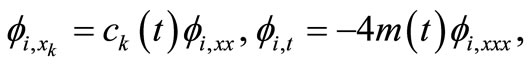 (14)
(14)
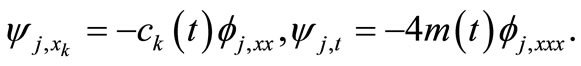 (15)
(15)
Proof. A differential of the determinant 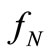 expressed by means of a Pfaffian is
expressed by means of a Pfaffian is
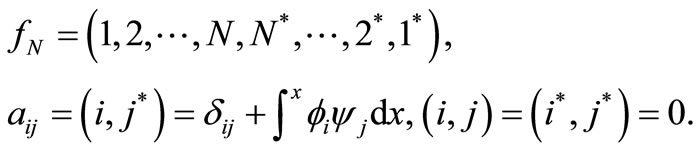 (16)
(16)
Next we introduce the Pfaffians  defined by
defined by
 (17)
(17)
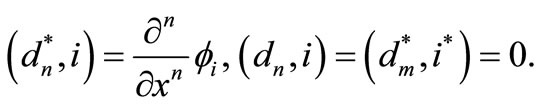 (18)
(18)
Based on the Pfaffians defined above, differentials of the elements 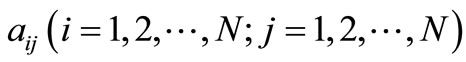 can be obtained as follows:
can be obtained as follows:
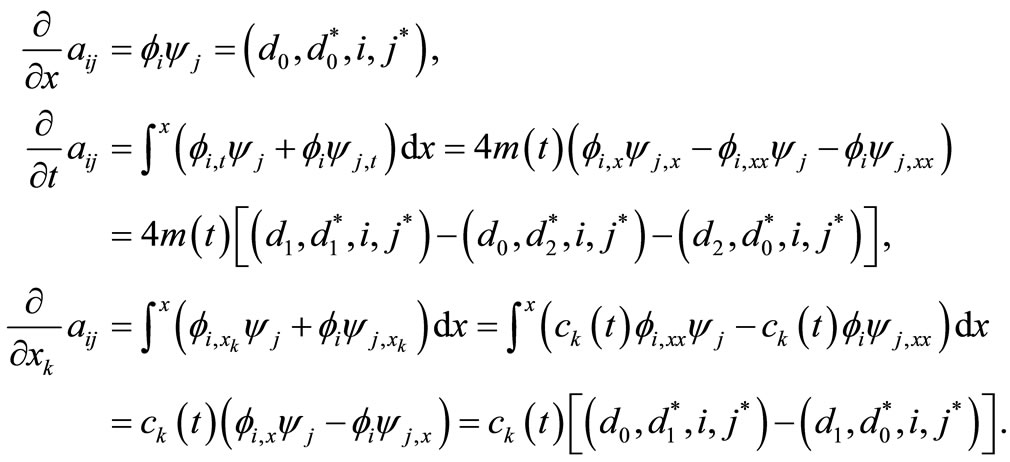 (19)
(19)
We denote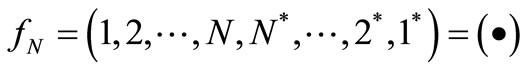 , then
, then
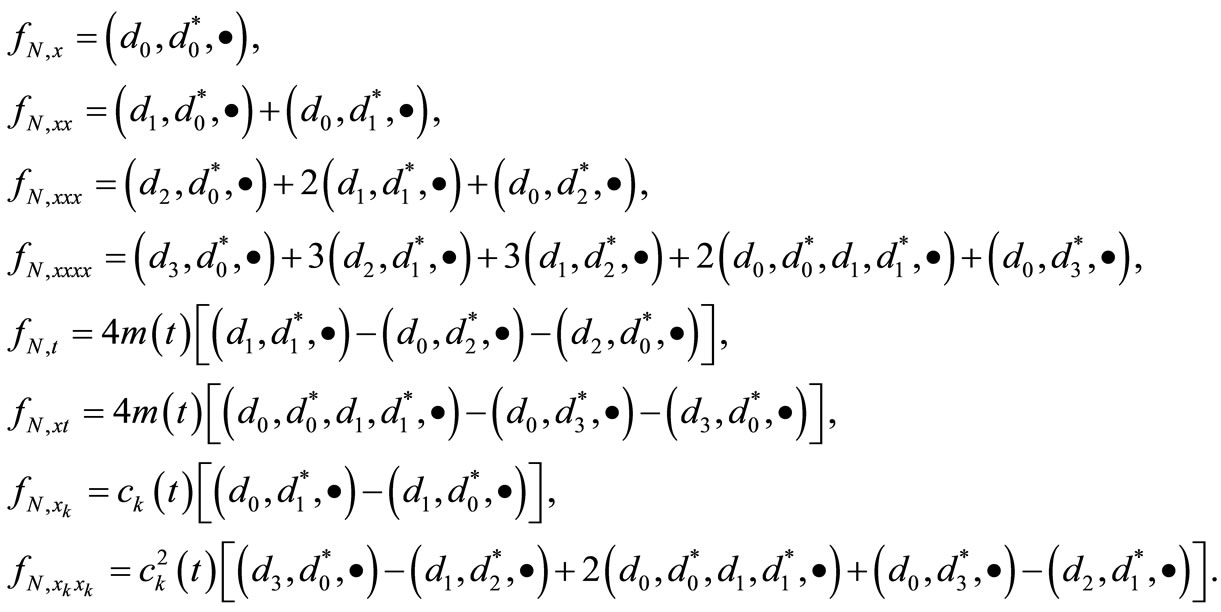 (20)
(20)
Substituting the above Pfaffians into (4), after some calculations, we have
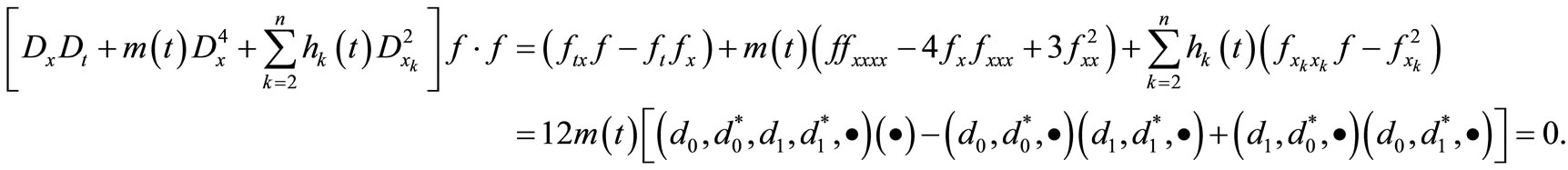 (21)
(21)
This shows that the Grammian determinant 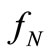 with the conditions of (14) and (15) solves (4).
with the conditions of (14) and (15) solves (4).
5. Conclusions and Discussions
In summary we have extended the Wronskian method and Pfaffian properties to the generalized variable-coefficient (n + 1)-dimensional KP equation (1). As a result, the Wronskian solutions and the Grammian solutions of (1) have been derived. It is known that if one gets the solutions of the conditions (8) or that of (14) and (15), then one can obtain the corresponding solutions of (1), which need to be further studied.
6. Acknowledgements
This work is supported by the National Natural Science Foundation of China (No 10771196 and No 10831003), the Foundation of Zhejiang Educational Committee (No. Y201018244) and Zhejiang Innovation Project (No T200905).
REFERENCES
- P. A. Clarkson, “Painlevé Analysis and the Complete Integrability of a Generalized Variable-Coefficient Kadomtsev-Petviashvili Equation,” IMA Journal of Applied Mathematics, Vol. 44, No. 1, 1990, pp. 27-53. doi:10.1093/imamat/44.1.27
- S. F. Deng, “Exact Solutions for A Nonisospectral and Variable-Coefficient Kadomtsev-Petviashvili Equation,” Chinese Physics Letters, Vol. 23, No. 7, 2006, pp. 1662- 1665. doi:10.1088/0256-307X/23/7/002
- L. Y. Ye, Y. N. Lv and Y. Zhang, “Grammian Solutions to a Variable-Coefficient KP Equation,” Chinese Physics Letters, Vol. 25, No. 2, 2008, pp. 357-358. doi:10.1088/0256-307X/25/2/002
- R. Hirota, “The Direct Methods in Soliton Theory,” Cambridge University Press, Cambridge, 2004.
- Y. Zhang, J. B. Li and Y. N. Lv, “The Exact Soliton and Integrable Properties to the Variable-Coefficient Modified Korteweg-de Vries Equation,” Annals of Physics, Vol. 323, No. 12, 2008, pp. 3059-3064. doi:10.1016/j.aop.2008.04.012
- Y. Zhang, Y. Song, L. Cheng, J. Y. Ge and W. W. Wei, “Exact Solutions and Painlevé Analysis of A New (2 + 1) -Dimensional Generalized KdV Equation,” Nonlinear Dynamics, in Press.
- J. Satsuma, “A Wronskian Representation of N-Soliton Solutions of Nonlinear Evolution Equations,” Journal of the Physical Society of Japan, Vol. 46, No. 1, 1979, pp. 359-360. doi:10.1143/JPSJ.46.359
- N. C. Freeman and J. J. Nimmo, “A Method of Obtaining the N-Solution of the Boussinesq Equation in Terms of a Wronskian,” Physics Letters A, Vol. 95, No. 1, 1983, pp. 4-6. doi:10.1016/0375-9601(83)90765-X
- Y. Zhang, T. F. Cheng, D. J. Ding and X. L. Dang, “Wronskian and Grammian Solutions for (2 + 1)-Dimensional Soliton Equation,” Communications in Theoretical Physics, Vol. 55, No. 1, 2011, pp. 20-24. doi:10.1088/0253-6102/55/1/04
- G. F. Yu and H. W. Tam, “On the Nonisospectral Kadomtsev-Petviashvili Equation,” Journal of the Physics A: Mathematical and General, Vol. 39, No. 13, 2006, pp. 3367-3373. doi:10.1088/0305-4470/39/13/014

