Advances in Pure Mathematics
Vol.2 No.5(2012), Article ID:22801,4 pages DOI:10.4236/apm.2012.25047
On Certain Properties of Trigonometrically ρ-Convex Functions
Department of Mathematics, Faculty of Basic Education, PAAET, Shamiya, Kuwait
Email: mss_ali5@yahoo.com
Received March 30, 2012; revised April 29, 2012; accepted May 11, 2012
Keywords: Generalized convex functions; trigonometrically ρ-Convex functions; supporting functions; average functions; extremum problems
ABSTRACT
The aim of this paper is to prove that the average function of a trigonometrically ρ-convex function is trigonometrically ρ-convex. Furthermore, we show the existence of support curves implies the trigonometric ρ-convexity, and prove an extremum property of this function.
1. Introduction
In 1908, Phragmén and Lindelöf ( See, e.g. [1]) showed that if  is an entire function of order
is an entire function of order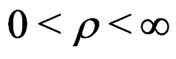 , then its indicator which is defined as:
, then its indicator which is defined as:

has the following property:
If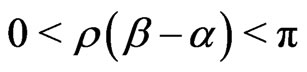 , and
, and 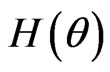 is the function of the form
is the function of the form
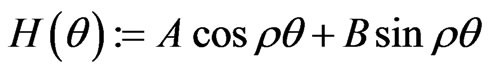
(such functions are called sinusoidal or ρ-trigonometric) which coincides with 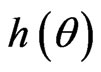 at
at  and at
and at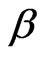 , then for
, then for  we have
we have
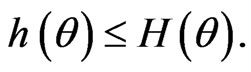
This property is called a trigonometric ρ-convexity ([1,2]).
In this article we shall be concerned with real finite functions defined on a finite or infinite interval 
A well known theorem [3] in the theory of ordinary convex functions states that: A necessary and sufficient condition in order that the function  be convex is that there is at least one line of support for
be convex is that there is at least one line of support for  at each point
at each point 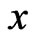 in
in 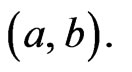
In Theorem 3.1, we prove this result in case of trigonometrically ρ-convex functions. In Theorem 3.2, we prove the extremum property [4] of convex functions in case of trigonometrically ρ-convex functions. And finally in Theorem 3.3, we show that the average function [5] of a trigonometrically ρ-convex function is also trigonometrically ρ-convex.
2. Definitions and Preliminary Results
In this section we present the basic definitions and results which will be used later , see for example ([1,2], and [6-9]).
Definition 2.1. A function 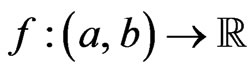 is said to be trigonometrically ρ-convex if for any arbitrary closed subinterval
is said to be trigonometrically ρ-convex if for any arbitrary closed subinterval 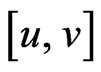 of
of 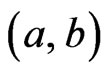 such that
such that 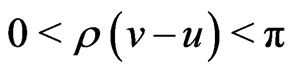 , the graph of
, the graph of  for
for  lies nowhere above the ρ-trigonometric function, determined by the equation
lies nowhere above the ρ-trigonometric function, determined by the equation
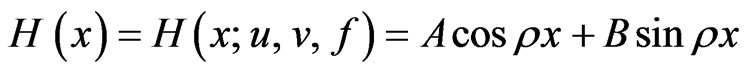
where  and
and  are chosen such that
are chosen such that  and
and 
Equivalently, if for all 
 (1)
(1)
The trigonometrically ρ-convex functions possess a number of properties analogous to those of convex functions.
For example: If 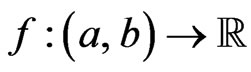 is trigonometrically ρ-convex function, then for any
is trigonometrically ρ-convex function, then for any  such that
such that 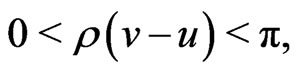 the inequality
the inequality  holds outside the interval
holds outside the interval 
Definition 2.2. A function

is said to be supporting function for 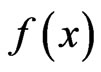 at the point
at the point 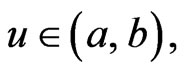 if
if
 (2)
(2)
That is, if 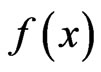 and
and 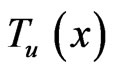 agree at
agree at 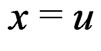 and the graph of
and the graph of 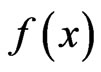 does not lie under the support curve.
does not lie under the support curve.
Remark 2.1. If  is differentiable trigonometrically ρ-convex function, then the supporting function for
is differentiable trigonometrically ρ-convex function, then the supporting function for 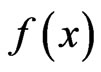 at the point
at the point 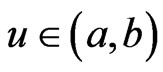 has the form
has the form
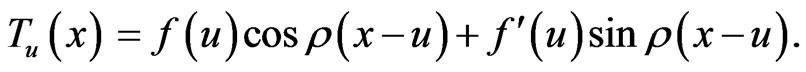
Proof. The supporting function 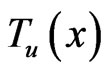 for
for 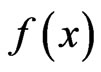 at the point
at the point  can be described as follows:
can be described as follows:

where  such that
such that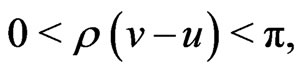 and as
and as

Then taking the limit of both sides as 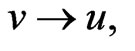 and from (1), one obtains
and from (1), one obtains
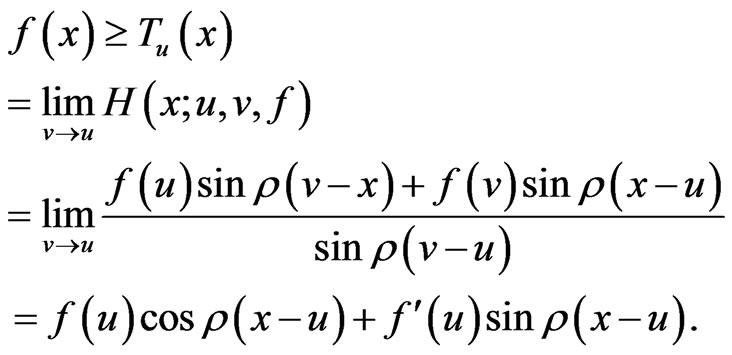
Thus, the claim follows.
Theorem 2.1. A trigonometrically ρ-convex function 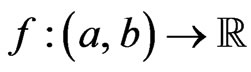 has finite right and left derivatives
has finite right and left derivatives 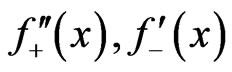 at every point
at every point 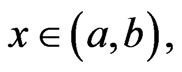 and
and 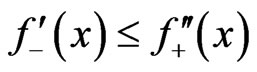 for all
for all 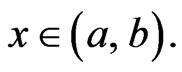
Theorem 2.2. Let 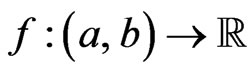 be a two times continuously differentiable function. Then
be a two times continuously differentiable function. Then  is trigonome-trically ρ-convex on
is trigonome-trically ρ-convex on 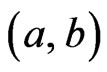 if and only if
if and only if 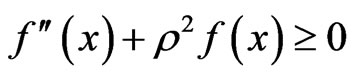 for all
for all 
Property 2.1. Under the assumptions of Theorem 2.1, the function 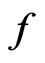 is continuously differentiable on
is continuously differentiable on 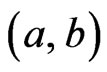 with the exception of an at most countable set.
with the exception of an at most countable set.
Property 2.2. A necessary and sufficient condition for the function 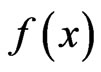 to be a trigonometrically ρ-convex in
to be a trigonometrically ρ-convex in 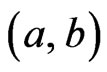 is that the function
is that the function
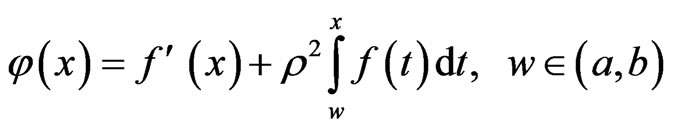
is non-decreasing in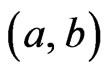 .
.
Lemma 2.1. Let 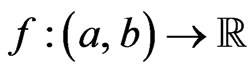 be a continuous,
be a continuous, 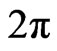 - periodic function, and the derivative
- periodic function, and the derivative 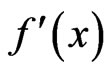 exists and piecewise continuous function and let
exists and piecewise continuous function and let  be a set of discontinuity points for
be a set of discontinuity points for  If
If
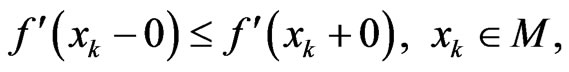 (3)
(3)
and 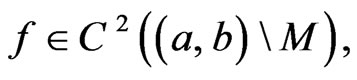 where
where
 (4)
(4)
Then  is trigonometrically ρ-convex on
is trigonometrically ρ-convex on .
.
Proof. Consider
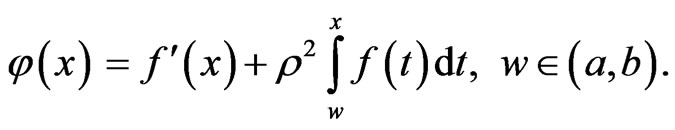 (5)
(5)
Two cases arise, as follows.
Case 1. Suppose 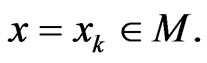 Using (5), we observe
Using (5), we observe
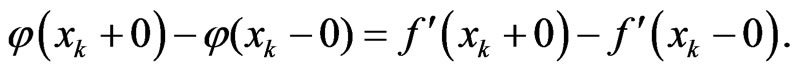
From (3), we get 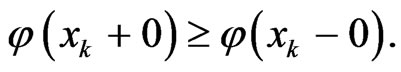
So, the function 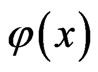 is non-decreasing in
is non-decreasing in  Case 2. Let
Case 2. Let  and
and
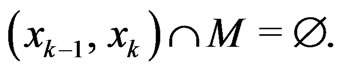
Differentiating both sides of (5) with respect to 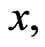 one has
one has
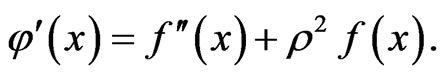
Using (4), one obtains
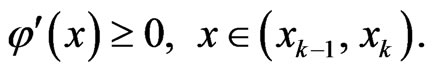
Thus,  is non-decreasing function in
is non-decreasing function in 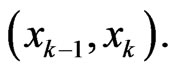
Therefore, from Property 2.2, we conclude that the function  is trigonometrically ρ-convex on
is trigonometrically ρ-convex on .
.
3. Main Results
Theorem 3.1. A function  is trigonometrically ρ-convex on
is trigonometrically ρ-convex on 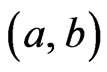 if and only if there exists a supporting function for
if and only if there exists a supporting function for  at each point
at each point 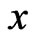 in
in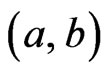 .
.
Proof. The necessity is an immediate consequence of F. F. Bonsall [10].
To prove the sufficiency, let 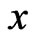 be an arbitrary point in
be an arbitrary point in 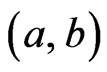 and
and 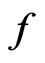 has a supporting function at this point. For convenience, we shall write the supporting function in the follwoing form:
has a supporting function at this point. For convenience, we shall write the supporting function in the follwoing form:
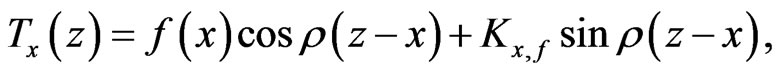
where 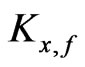 is a fixed real number depends on
is a fixed real number depends on 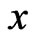 and
and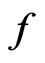 .
.
From Definition 2.2, one has

It follows that,
 (6)
(6)
For all 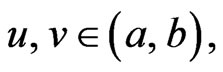 choose any
choose any 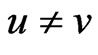 such that
such that  and
and  with
with 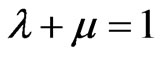 and let
and let 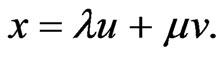
Applying (6) twice at 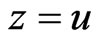 and at
and at 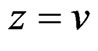 yields
yields
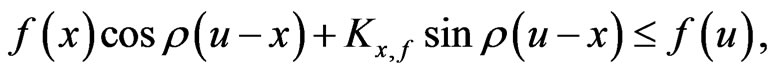
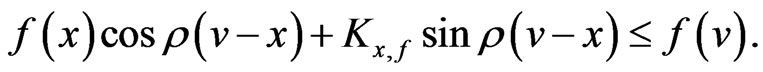
Multiplying the first inequality by 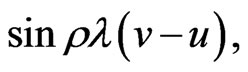 the second by
the second by  and adding them, we obtain
and adding them, we obtain

Consequently
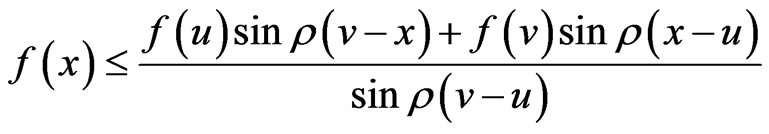
for all 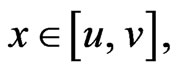 which proves that the function
which proves that the function  is trigonometrically ρ-convex on
is trigonometrically ρ-convex on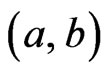 .
.
Hence, the theorem follows.
Remark 3.1. For a trigonometrically ρ-convex function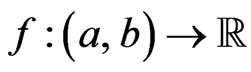 , the constant
, the constant  in the above theorem is equal to
in the above theorem is equal to 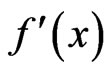 if
if  is differentiable at the point
is differentiable at the point 
in , otherwise,
, otherwise, 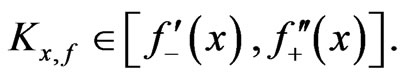
Theorem 3.2. Let 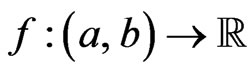 be a trigonometrically ρ-convex function such that
be a trigonometrically ρ-convex function such that  and let
and let 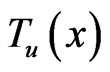 be a supporting function for
be a supporting function for  at the point
at the point  Then the function
Then the function
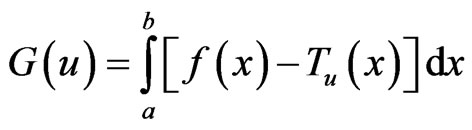
has a minimum value at 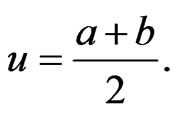
Proof. From Definition 2.2, we have
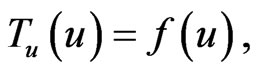 (7)
(7)
and
 (8)
(8)
and 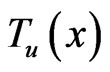 can be written in the form
can be written in the form
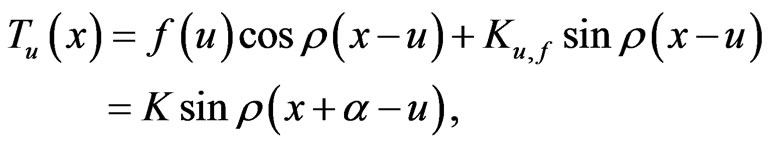 (9)
(9)
where 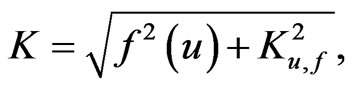 and
and 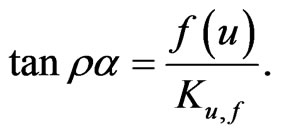
Using (9), one obtains

Consequently,
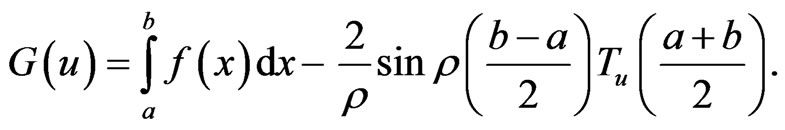 (10)
(10)
Using (7) at  the function
the function 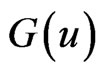 becomes
becomes
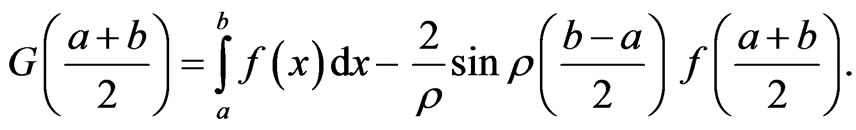 (11)
(11)
But from (8) ,we observe  for all
for all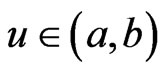 .
.
Now using (10) and (11), it follows that
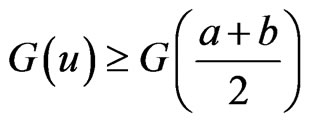 for all
for all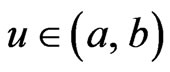 .
.
Hence, the minimum value of the function 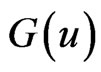
occurs at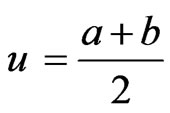 .
.
Theorem 3.3. Let 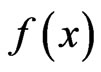 be a non-negative, 2π- periodic, and trigonometrically ρ-convex function with a continuous second derivative on
be a non-negative, 2π- periodic, and trigonometrically ρ-convex function with a continuous second derivative on  and let
and let 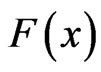 be a 2π-periodic function defined in
be a 2π-periodic function defined in 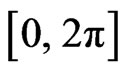 as follows
as follows
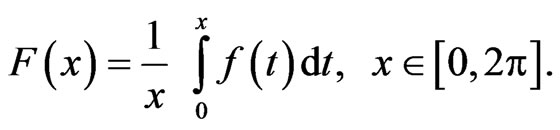 (12)
(12)
If 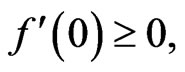 and
and
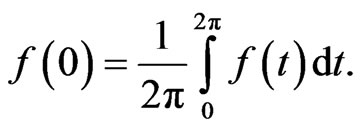 (13)
(13)
Then,  is trigonometrically ρ-convex function.
is trigonometrically ρ-convex function.
Proof. The proof mainly depends on Lemma 2.1. So, we show that the function  satisfies all conditions in this lemma.
satisfies all conditions in this lemma.
Suppose that
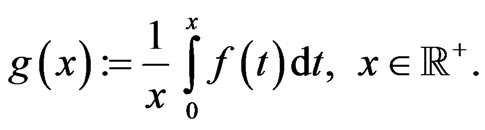 (14)
(14)
It is obvious that, 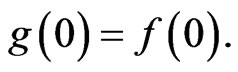
First, we study the behavior of the function 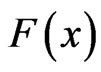 inside the interval
inside the interval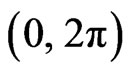 .
.
It is clear from (12) that 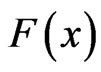 s is an absolutely continuous function, has a derivative of third order.
s is an absolutely continuous function, has a derivative of third order.
But from the periodicity of 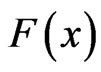 and (13), we get
and (13), we get
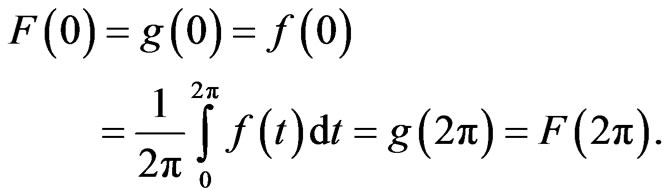 (15)
(15)
Using the following substitution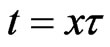 .
.
It follows that, 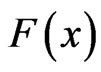 can be written as
can be written as
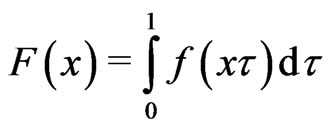 and
and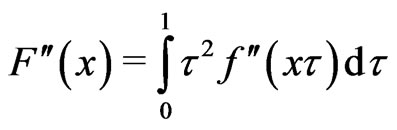 .
.
Consequently,
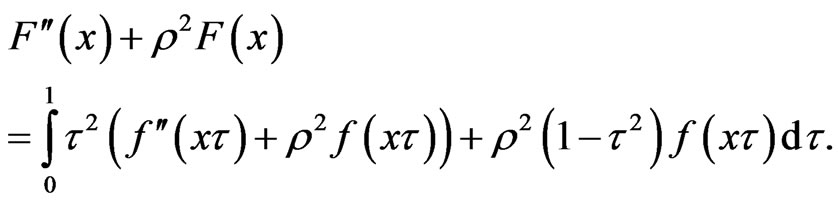 (16)
(16)
Since 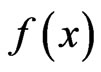 is non-negative, trigonometrically ρ-convex function, and
is non-negative, trigonometrically ρ-convex function, and  then from Theorem 2.2 and (16) it follows that
then from Theorem 2.2 and (16) it follows that
 (17)
(17)
Second, we prove that
 (18)
(18)
From the definition of 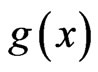 in (14) and the periodicity of
in (14) and the periodicity of 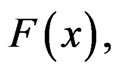 we observe that
we observe that 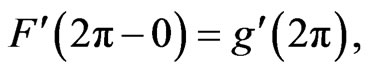 and
and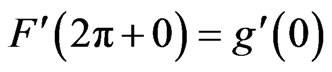 .
.
Again using (14), we have
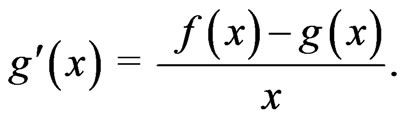 (19)
(19)
Thus, from (15) and (19), one has , and
, and
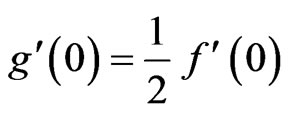 .
.
Hence, from (13), we infer that
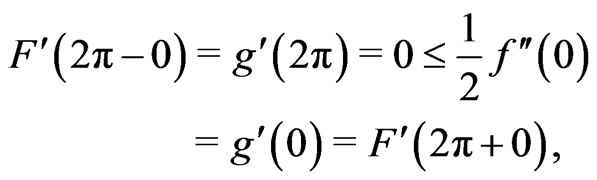
and the inequality in (18) is proved.
Now using (17), (18), and Lemma 2.1, we conclude that  is trigonometrically ρ-convex function, and the theorem is proved.
is trigonometrically ρ-convex function, and the theorem is proved.
4. Acknowledgements
The author wishes to thank the anonymous referees for their fruitful comments and suggestions which improved the original manuscript.
REFERENCES
- B. Ya. Levin, “Lectures on Entire Functions,” American Mathematical Society, 1996.
- L. S. Maergoiz, “Asymptotic Characteristics of Entire Functions and Their Applications in Mathematics and Biophysics,” Kluwer Academic Publishers, New York, 2003.
- A. W. Roberts and D. E. Varberg, “Convex Functions,” Academic Press, New York, 1973.
- M. J. Miles, “An Extremum Property of Convex Functions,” American Mathematical Monthly, Vol. 76, 1969, pp. 921-922. doi:10.2307/2317948
- A. M. Bruckner and E. Ostrow, “Some Functions Classes Related to the Class of Convex Functions,” Pacific Journal of Mathematics, Vol. 12, 1962, pp. 1203-1215.
- E. F. Beckenbach, “Convex Functions,” Bulletin of the American Mathematical Society, Vol. 54, No. 5, 1948, pp. 439-460. doi:10.1090/S0002-9904-1948-08994-7
- J. W. Green, “Support, Convergence, and Differentiability Properties of Generalized Convex Functions,” Proceedings of the American Mathematical Society, Vol. 4, No. 3, 1953, pp. 391-396. doi:10.1090/S0002-9939-1953-0056039-2
- M. M. Peixoto, “On the Existence of Derivatives of Generalized Convex Functions,” Summa Brasilian Mathematics, Vol. 2, No. 3, 1948, pp. 35-42.
- M. M. Peixoto, “Generalized Convex Functions and Second Order Differential Inequlities,” Bulletin of the American Mathematical Society, Vol. 55, No. 6, 1949, pp. 563- 572. doi:10.1090/S0002-9904-1949-09246-7
- F. F. Bonsall, “The Characterization of Generalized Convex Functions,” The Quarterly Journal of Mathematics Oxford Series, Vol. 1, 1950, pp. 100-111. doi:10.1093/qmath/1.1.100

