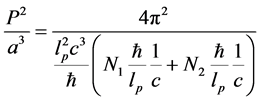International Journal of Astronomy and Astrophysics
Vol.06 No.02(2016), Article ID:67623,12 pages
10.4236/ijaa.2016.62017
Planck Quantization of Newton and Einstein Gravitation
Espen Gaarder Haug
Norwegian University of Life Sciences, Campus Ås, Norway

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 3 March 2016; accepted 20 June 2016; published 23 June 2016
ABSTRACT
In this paper we rewrite the gravitational constant based on its relationship with the Planck length and based on this, we rewrite the Planck mass in a slightly different form (that gives exactly the same value). In this way we are able to quantize a series of end results in Newton and Einstein’s gravitation theories. The formulas will still give exactly the same values as before, but everything related to gravity will then come in quanta. This also gives some new insight; for example, the gravitational deflection of light can be written as only a function of the radius and the Planck length. Numerically this only has implications at the quantum scale; for macro objects the discrete steps are so tiny that they are close to impossible to notice. Hopefully this can give additional insight into how well or not so well (ad hoc) quantized Newton and Einstein’s gravitation is potentially linked with the quantum world.
Keywords:
Quantized Gravitation, Gravitational Constant, Escape Velocity, Gravitational Time Dilation, Schwarzschild Radius, Planck Length, Bending of Light, Planck Mass, Planck Length

1. Foundation
We suggest that Newton’s gravitational constant [1] could be written as a function of Planck’s reduced constant
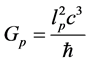 (1)
(1)
where 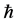 is the reduced Planck’s constant, c is the well tested round-trip speed of light, and
is the reduced Planck’s constant, c is the well tested round-trip speed of light, and 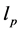 is the Planck length [2] . We could call this Planck’s form of the gravitational constant. This way of writing Newton’s gravitational constant does not change the value of the constant. If one knows the Planck length, then the gravitational constant is known, or alternatively and more practically one can calibrate the Planck length based on empirical measurements of the gravitational constant. There is still considerable uncertainty in the exact measurement of the gravitational constant. Experimentally, substantial progress has been made in recent years based on various methods. See, for example, [3] - [7] . Also the relationship between physical constants from the microcosms (subatomic world) and the macrocosms (cosmos) plays an important role in physics and a continuous effort is going into improving our measurements and understanding of these relationships. See, for example, [8] .
is the Planck length [2] . We could call this Planck’s form of the gravitational constant. This way of writing Newton’s gravitational constant does not change the value of the constant. If one knows the Planck length, then the gravitational constant is known, or alternatively and more practically one can calibrate the Planck length based on empirical measurements of the gravitational constant. There is still considerable uncertainty in the exact measurement of the gravitational constant. Experimentally, substantial progress has been made in recent years based on various methods. See, for example, [3] - [7] . Also the relationship between physical constants from the microcosms (subatomic world) and the macrocosms (cosmos) plays an important role in physics and a continuous effort is going into improving our measurements and understanding of these relationships. See, for example, [8] .
As shown by Haug [9] , the Planck form of the gravitational constant enables us to rewrite the Planck length as
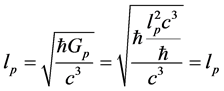 (2)
(2)
and the Planck mass as
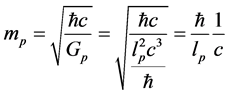 (3)
(3)
Using the gravitational constant in the Planck form, as well as the rewritten Planck units, we are easily able to modify a series of end results from Newton and Einstein’s gravitational theories to contain quantization as well.
2. Newton’s Universal Gravitational Force
Newton’s gravitational force is given by
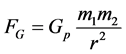 (4)
(4)
Using the gravitational constant of the form 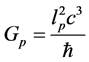 and the Planck mass of
and the Planck mass of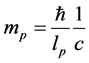 , we can rewrite Newtons gravitational force for two Planck masses as
, we can rewrite Newtons gravitational force for two Planck masses as
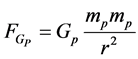
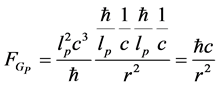 (5)
(5)
In the special case where , we get
, we get
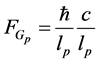 (6)
(6)
It seems from this that gravity potentially could be related to hits per second, even if the output naturally is the same as from the standard formula. For large masses the form will be
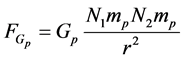
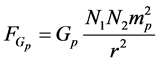
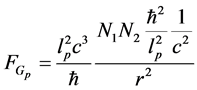

where 


3. Escape Velocity at the Quantum Scale
The traditional escape velocity [10] - [12] is given by

where G is the traditional gravitational constant, M is the mass of the object we are “trying” to escape from, and r is the radius of that object. In other words, we stand at the surface of the object, for example a hydrogen atom or a planet. Based on the gravitational constant written in the Planck form, we can find the escape velocity at Planck scale; see the Appendix for a derivation from “scratch”. It must be

where N is the number of Planck masses in the planet or mass in question.
A particularly interesting case is when we only have one Planck mass 


as the escape velocity for a particle with Planck mass is c. Next we will see if the formula above can also be used to calculate the escape velocity of Earth. The Earth’s mass is
The Earth’s mass in terms of the numbers of Planck masses must be

which is equal to 40,269 km/h, the well-known escape velocity from the Earth’s gravitational field. We think our new way of looking at gravity could have consequences for the understanding of gravity. Gravitation must come in discrete steps and the escape velocity must also come in discrete steps for a given radius; this is because the amount of matter likely comes in discrete steps.
4. Orbital Speed
The orbital speed is given by

We can rewrite this in the form of the Planck gravitational constant and the Planck mass as

This can also be written as

5. Gravitational Acceleration Field
The gravitational acceleration field in modern physics is given by

This can be rewritten in quantized form as

6. Gravitational Parameter
The standard gravitational parameter is given by

This can be rewritten in quantized form as

7. Kepler’s Third Law of Motion
The Newtonian “mechanics version” of Kepler’s third law of motion for a circular orbit is given by

where 

where 



where N is now the number of Planck masses in the Sun.
8. Gravitational Time Dilation at Planck Scale
Einstein’s gravitational time dilation [13] is given by

where 

Let’s see if we can calculate the time dilation at, for example, the surface of the Earth from Planck scale gravitational time dilation. The Earth’s mass is
Planck mass must be

That is for every second that goes by in outer space (a clock far away from the massive object), 0.99999999930391500 seconds goes by on the surface of the Earth. That is to say, for every year in outer space (very far from the Earth), there are about 22 milliseconds left to reach an Earth year. This is naturally the same as we would get with Einstein’s formula. Still, the new way of writing the formula gives additional insight.
Circular orbit’s gravitational time dilation
The time dilation for a clock at circular orbit1 is given by

where 

9. The Schwarzschild Radius
The Schwarzschild radius [14] [15] of a mass M is given by

Rewritten into the quantum realm as described in this article, it must be

For a clock at the Schwarzschild radius, we get a time dilation of

At the Schwarzschild radius, time stands still. For a radius shorter than that the gravitational time dilation equation above breaks down.2
Mass in Schwarzschild meters
The Schwarzschild mass in terms of meters is given by

This can be rewritten as

10. Quantized Gravitational Bending of Light
The angle of deflection in Einstein’s General Relativity theory is given by
This can be rewritten as

where N is the number of Planck masses making up the mass we are interested in. From the formula above, this means that the deflection of angles comes in quanta. Lets also “control” that our Planck scale deflection rooted
in Planck and GR is consistent for large bodies like the Sun, for example. The solar mass is



If we multiply this by


See for example [16] for derivations of bending of light under Newton’s gravitation.
11. Gravitational Redshift
Einstein’s gravitational redshift is given by

where 

Further, in the Newtonian limit when 

12. Einstein’s Field Equation
And finally we get to Einstein's field equation. It is given by
Table 1. The table shows some of the standard gravitational relationships given by Newton and Einstein and their expression in quantized form.

I am far from an expert on Einstein’s field equation, but based on the Planck gravitational constant given in this paper, we can rewrite it as

Bear in mind 

The potential interpretation and usefulness of this rewritten version of Einstein’s field equation we leave to other experts for consideration. An interesting question is naturally whether or not it is consistent with some of the derivations given above in this form.
13. Table Summary
Table 1 summarizes our rewriting of some gravitational formulas. The output is still the same, but based on this view of gravity, masses, gravitational time dilation, and even escape velocity all come in discrete steps.
14. Conclusion
By making the gravitational constant be a function form of the reduced Planck constant, one can easily rewrite many of the end results from Newton and Einstein’s gravitation in quantized form. Even if this is seen as an ad hoc method, it could still give new insight into what degree quantized Newton’s gravitation and General Relativity are consistent with the quantum realm.
Acknowledgements
Thanks to Victoria Terces for helping me edit this manuscript. Also thanks to an anonymous referee for useful comments.
Cite this paper
Espen Gaarder Haug, (2016) Planck Quantization of Newton and Einstein Gravitation. International Journal of Astronomy and Astrophysics,06,206-217. doi: 10.4236/ijaa.2016.62017
References
- 1. Newton, I. (1686) Philosophiae Naturalis Principia Mathematica. Joseph Streater, London.
- 2. Planck, M. (1901) Ueber das gesetz der energieverteilung im normalspectrum. Annalen der Physik, 309, 553-563.
http://dx.doi.org/10.1002/andp.19013090310 - 3. Bisnovatyi-Kogan, G.S. (2006) Checking the Variability of the Gravitational Constant with Binary Pulsars. International Journal of Modern Physics D, 15, 1047.
http://dx.doi.org/10.1142/S0218271806008747 - 4. Galli, S., Melchiorri, A., Smoot, G.F. and Zahn, O. (2009) From Cavendish to Planck: Constraining Newton’s Gravitational Constant with CMB Temperature and Polarization Anisotropy. Physical Review D, 80, Article ID: 023508.
http://dx.doi.org/10.1103/physrevd.80.023508 - 5. Fixler, B., Foster, G.T., McGuirk, J.M. and Kasevich, M.A. (2007) Atom Interferometer Measurement of the Newtonian Constant of Gravity. Science, 315, 74-77.
http://dx.doi.org/10.1126/science.1135459 - 6. Schlamminger, S. (2014) Fundamental Constant: A Cool Way to Measure Big G. Nature, 510, 478-480.
http://dx.doi.org/10.1038/nature13507 - 7. Rosi, G., Sorrentino, F., Cacciapuoti, L., Prevedelli, M. and Tino, G.M. (2014) Precision Measurement of the Newtonian Gravitational Constant Using Cold Atoms. Nature, 510, 518-521.
http://dx.doi.org/10.1038/nature13433 - 8. Ade, P.A.R., Aghanim, N., et al. (2015) Planck Intermediate Results. XXIV. Constraints on Variations in Fundamental Constants. Astronomy & Astrophysics, 580, A22.
- 9. Haug, E.G. (2016) The Gravitational Constant and the Planck’s Units: A Simplification of the Quantum Realm.
http://vixra.org/pdf/1603.0207v5.pdf - 10. Schutz, B. (2003) Gravity from the Ground up. Cambridge University Press, Cambridge.
http://dx.doi.org/10.1017/CBO9780511807800 - 11. Augousti, A.T. and Radosz, A. (2006) An Observation on the Congruence of the Escape Velocity in Classical Mechanics and General Relativity in a Schwarzschild Metric. European Journal of Physics, 376, 331-335.
http://dx.doi.org/10.1088/0143-0807/27/2/015 - 12. Tipler, P.A. and Llewellyn, R.A. (2012) Modern Physics. 6th Edition, W. H. Freeman and Company, New York.
- 13. Einstein, A. (1916) Näherungsweise integration der feldgleichungen der gravitation. Sitzungsberichte der KÖniglich Preussischen Akademie der Wissenschaften, Berlin, 668-696.
- 14. Schwarzschild, K. (1916) üeber das gravitationsfeld einer kugel aus inkompressibler flussigkeit nach der einsteinschen theorie. Sitzungsberichte der Deutschen Akademie der Wissenschaften zu Berlin, Klasse fur Mathematik, Physik, und Technik, Berlin, 424.
- 15. Schwarzschild, K. (1916) über das gravitationsfeld eines massenpunktes nach der einsteinschen theorie. Sitzungsberichte der Deutschen Akademie der Wissenschaften zu Berlin, Klasse fur Mathematik, Physik, und Technik, Berlin, 189.
- 16. Soares, D.S.L. (2009) Newtonian Gravitational Deflection of Light Revisited.
https://arxiv.org/abs/physics/0508030
Appendix: Escape Velocity
Derivation of the escape velocity from Planck scale

where 

This is a quantized escape velocity. Since 


where N is the number of Planck masses in the mass we are trying to escape from.
notes
1At orbital radius larger then
2Except if we assume the