Open Journal of Civil Engineering
Vol.04 No.04(2014), Article ID:52566,11 pages
10.4236/ojce.2014.44031
A Study on the Seismic Isolation Systems of Bridges with Lead Rubber Bearings
Woo-Suk Kim, Dong-Joon Ahn, Jong-Kook Lee*
School of Architecture, Kumoh National Institute of Technology, Gumi, Republic of Korea
Email: kimw@kumoh.ac.kr, djahn@kumoh.ac.kr, *ljk@kumoh.ac.kr
Copyright © 2014 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 12 September 2014; revised 8 October 2014; accepted 28 October 2014
ABSTRACT
This study consists of the development and presentation of example of seismic isolation system analysis and design for a continuous, 3-span, cast-in-place concrete box girder bridge. It is ex- pected that example is developed for all Lead-Rubber Bearing (LRB) seismic isolation system on piers and abutments which placed in between super-structure and sub-structure. Design forces, displacements, and drifts are given distinctive consideration in accordance with Caltrans Seismic Design Criteria (2004). Most of all, total displacement
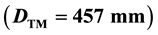 on design for all LRBs case is reduced comparing with combined lead-rubber and elastomeric bearing system
on design for all LRBs case is reduced comparing with combined lead-rubber and elastomeric bearing system
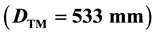 . Therefore, this represents substantial reduction in cost because of reduction of expansion joint. This presents a summary of analysis and design of seismic isolation system by energy mitigation with LRB on bridges.
. Therefore, this represents substantial reduction in cost because of reduction of expansion joint. This presents a summary of analysis and design of seismic isolation system by energy mitigation with LRB on bridges.
Keywords:
Seismic Isolation System, Bridge, Lead Rubber Bearing (LRB), Energy Mitigation

1. Introduction
Seismic isolation systems are the separating of structures (such as bridge, building, railway, road, airport, harbor, dam, and tunnel etc.) from ground motions generated by earthquakes which could induce damage to the structures. Among various seismic isolation systems, lead-rubber bearing (LRB) which has innovative mechanism can lead to increased effective stiffness and is accommodating force in reinforced concrete (RC) structures. LRB is a novel apparatus based on the combination of laminated layers rubber bearing using lead plugs (Constantinou, et al. (2006) [1] ). In this research, a bridge was selected to demonstrate the application of analysis and bearing design procedures for seismic isolation system. The bridge was used as an example of bridge design without an isolation system in the Federal Highway Administration (FHWA) Seismic Design Course, Design Example No. 4, prepared by Berger/Abam Engineers (1996) [2] . The bridge is a continuous, three-span, cast-in-place concrete box girder structure with a 30-degree skew. The two intermediate bents consist of two round columns with a crossbeam on top. The geometry of the bridge, section properties and foundation properties are assumed to be the same in the original bridge in the FHWA example (2000) [3] . It is presumed that the original bridge design is sufficient to sustain the loads and displacement demands when seismically isolated as described herein. Only minor changes in the bridge geometry were implemented in order to facilitate seismic isolation.
2. Description of Bridge
Figures 1-3 show, respectively, the plan and elevation, the abutment sections and a section at an intermediate bent. In Figure 3, the bent is shown at the skew angle of 30 degrees, whereas for the box girder the section is perpendicular to the longitudinal axis. The actual distance between the column centerlines is 7.92 m (Figure 1).
Figure 1. Bridge plan view and elevation.
Figure 2. Section at abutment.
Figure 3. Cross section at intermediate bent.
The bridge is isolated with two multi-directional seismic isolators at each abutment and pier location for a total of eight multi-directional seismic isolators with lead rubber bearings. The isolators are directly located above the circular columns. The plan views of the isolated bridge are shown in Figure 4.
The bridge is isolated with two isolators at each abutment and pier location for a total of eight isolators. The isolators are directly located above the circular columns. The use of two isolators versus a larger number is intentional for the following reasons:
・ With elastomeric bearings it is possible to achieve a larger period of isolation (more mass per bearing).
・ The distribution of load on each isolator is accurately calculated. The use of more than two isolators per location would have resulted in uncertainty in the calculation of the axial load in vertically stiff bearings.
・ Reduction in construction cost.
For better distribution of load to the bearings, diaphragms are included in the box girder at the abutment and pier locations above the isolators. An additional 596 kN weight at each diaphragm location is introduced by the addition of these diagrams. The bridge is considered to have three traffic lanes. Loadings were determined based on AASHTO LRFD Specifications (2001) [4] with live load consisting of truck, lane and tandem and wind load being representative of typical sites in the Western United States.
Figure 5 shows a model for the analysis of the bridge. The model may be used in static and multimode analysis.
The cross sectional properties of the bridge and weights are presented in Table 1. The modulus of elasticity of concrete is . Foundation spring constants are presented in Table 2.
. Foundation spring constants are presented in Table 2.
2.1. Analysis of Bridge for Dead, Live, Brake and Wind Loadings
The weight of the seismically isolated bridge superstructure is 22,650 kN. The difference is due to the presence now of diaphragms at the abutment and pier locations in order to transfer loads to the bearings. Kim (2007) [5] presents calculations for the bearing loads and rotations due to dead, live, braking and wind forces. Table 3 presents a summary of bearing loads and rotations. On the basis of the results in Table 3, the bearings do not experience uplift or tension for any combination of dead and live loadings.
Figure 4. Plan view of bridge isolated with lead-rubber bearings.
Figure 5. Model of bridge for single- and multimode analysis.

Table 1. Cross sectional properties and weights in bridge model.
Table 2. Foundation spring constants in bridge model.

Table 3. Bearing loads and rotations due to dead, live, brake and wind loads.
2.2. Seismic Loading
Seismic loading is defined per Caltrans Seismic Design Criteria (2004) [6] to be the 5%-damped response spectrum of Magnitude 7.25, 0.7 g acceleration and soil profile C. The horizontal response spectra for acceleration and displacement are shown in Figure 6. This earthquake is considered to be the Maximum Earthquake. Calculations are performed only for this earthquake level and isolator safety checks are performed on the basis of the calculated response at this level. The vertical earthquake is assumed to be described by the spectra of Figure 6 after multiplication by factor 0.70.
Figure 6. Horizontal 5%-damped response spectra for earthquake of magnitude 7.25 and soil profile type C (Adapted from Clatran Seismic Design Criteria (2004) [6] ).
3. Design Analysis of Lead-Rubber Isolation System
This case study is developed as an alternative to the combined lead-rubber and elastomeric bearing isolation system (Constatinou, et al., 2007 [7] ). The combined system may not be desirable because two different isolator types are used in an application with only eight bearings. An all lead-rubber bearing system is simpler and requires less testing.
3.1. Single Mode Analysis
Criteria for applicability of single mode analysis are presented in Table 4. Kim (2007) [5] presents the calculations for the analysis and safety check of the isolation system. The all pier abutment and pier bearings are lead-rubber bearings with 200 mm diameter lead core. All bearings are of identical construction and of identical materials. Drawing of the bearings is shown in Figure 7. The bearings need not be installed pre-deformed in order to accommodate displacements due to post-tensioning and shrinkage. The bearings are safe for a service displacement of 75 mm (includes shrinkage and post-tensioning effects) and 455 mm seismic displacement.
Table 5 presents a summary of the calculated displacement and force demands, the effective properties of the isolated structure and the effective properties of each type of bearing. These properties are useful in response spectrum, multi-mode analysis. The effective stiffness was calculated using
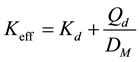 (1)
(1)
where (a) for abutment bearing,
 and
and
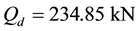 for lower bound and
for lower bound and
 and
and





Table 4. Applicability criteria for methods of analysis.
Table 5. Applicability criteria for methods of analysis.
1Based on one-directional excitation in longitudinal bridge direction; 2Based on three-directional excitation using 100% - 30% - 30% rule, and multiplying by Factor 1.1; 3Value is for 100% vertical + 30% transverse + 30% longitudinal combination (maximum axial load); 4Value is for 100% transverse + 30% vertical + 30% longitudinal combination (worst case for lead-rubber bearing safety check-combined with maximum bearing displacement).
Figure 7. Principle structure and size of LRB for bridge example.
3.2. Multimode Response Spectrum Analysis
Figure 8 shows the bridge model used for response spectrum analysis. For this analysis, each isolator is modeled as a vertical 3-dimentional beam element-rigidly connected at its two ends-of length






In Equation (2),





where








Torsional constant is set




Figure 8. First three modes of vibration of isolated bridge with lead-rubber bearing system in lower bound analysis. (a) First mode: T1 = 2.67 sec; (b) Second mode: T2 = 2.61 sec; (c) Third mode: T3 = 2.21 sec.

Response spectrum analysis was performed using the response spectrum of Figure 8 for 0.7 g which is 5%-damped spectrum after division by parameter B for periods larger or equal to 0.8 TM, where TM is the effective period and B is the parameter that relates the 5%-damped spectrum to the spectrum at the effective damping. Quantities TM, B and the effective damping are presented in Table 5. It should be noted that these quantities are given in Table 5 for the upper and lower bound cases, both of which are analyzed. Values of 0.8 TM are 1.3 sec for upper bound analysis and 2.0 sec for lower bound analysis. Values of parameters used in response spectrum analysis of lead-rubber bearing isolation system are presented in Table 6. Besides, values of spectral acceleration used in the analysis are presented in Table 7.
Eigenvalue and response spectrum analysis were performed in program SAP2000 [8] . Figure 8 presents the mode shapes of the first three modes of vibration of the isolated bridge in the lower bound analysis. They are two modes dominated by translational displacements in two orthogonal directions, and a third torsional mode of vibration. The results are qualitatively the same as those for the combined lead-rubber and elastomeric bearing system with the first mode characterized by deformation of both the isolators and the piers along the weak direction of the pier. The period matches nearly exactly the period that is calculated in simplified analysis accounting for the effects of substructure flexibility (T = 2.67 sec). The second mode is deformation along the strong axis of the pier and accordingly it consists primarily of isolator deformation. This period matches nearly the period calculated neglecting the effect of substructure flexibility (T = 2.58 sec).
Analysis was performed by separately applying the earthquake excitation in the longitudinal, transverse and vertical bridge directions. The vertical response spectrum was taken as a 70% portion of the horizontal 5%- damped spectrum without any modification for increased damping.
The results of these analyses are presented in Table 8 in terms of the bearing displacements; isolation shear force and bearing axial forces (only part due to earthquake). Table 8 presents a comparison of key response quantities obtained by the single and multimode-response spectrum methods of analysis. In each type of analysis, quantity DTM was calculated as the vectorial sum of bearing displacements due to longitudinal and transverse earthquake components combined using the 100% - 30% rule and then multiplying by Factor 1.1. The results demonstrate very good agreement in the calculated bearing displacement demands and isolation shear forces between the two methods of analysis.
Axial bearing forces are underestimated by the single mode analysis method due, primarily, to neglect of the skew in the calculations. Consideration of the skew angle is not difficult but the underestimation in the calculation of loads to have any significance in the safety of the bearings. Calculations show that the bearings have capacity to sustain the calculated loads in the maximum earthquake.
Table 6. Values of parameters h, A, I and E used in response spectrum analysis of lead-rubber bearing isolation system.

Table 7. Spectral acceleration values used in response spectrum analysis of isolated bridge with lead-rubber bearing system.

Table 8. Key response quantities obtained by multimode analysis isolated bridge with lead-rubber bearing system.
4. Conclusions
The examples presented in this paper demonstrate that single-mode and multi-mode analysis methods, when properly implemented, provide results in close agreement. On the basis of the results obtained in this study, the single mode method of analysis is sufficiently accurate and conservative to be used in analysis and design.
Examples of specifications which are consistent with the assumptions made in the analysis have been presented in this study. Table 9 presents a summary of key response quantities for the two example designs of this paper as obtained by the single mode method of analysis. These quantities are the total maximum dis-
Table 9. Comparison of key response quantities for two examples as obtained by single mode method of analysis (W = 22,650 kN).
1) Total displacement


2) In lower bound analysis, the benefit of all LRB versus Elastomeric bearing/LRB is almost 2% reduction in shear force at pier. When

3) Furthermore, while previous design requires tests for two types of bearing, another benefit is that construc- tion and testing is needed to only one type of bearing.
Acknowledgements
The authors would like to gratefully acknowledge Professor Michael C. Constantinou for his advice to this study. This research was supported by research fund, Kumoh National Institute of Technology.
References
- Constantinou, M.C., Whittaker, A.S., Kalpakidis, Y., Fenz, D.M. and Warn, G.P. (2006) Performance of Seismic Isolation Hardware under Service and Seismic Loading. Technical Report MCEER-07-0012, Multidisciplinary Center for Earth- quake Engineering Research, State University of New York at Buffalo, Buffalo.
- Berger/Abam Engineers, Inc. (1996) Federal Highway Administration Seismic Design Course, Design Example No. 4. (Document Available through NTIS, Document No. PB97-142111).
- Federal Emergency Management Agency (2000) Prestandard and Commentary for the Seismic Rehabilitation of Build- ings. FEMA 356, Washington DC.
- American Institute of Steel Construction (AISC) (2001) Manual for Steel Construction, Load and Resistance Factor Design. 3rd Edition, American Institute of Steel Construction-AISC, Chicago.
- Kim, W. (2007) Seismic Isolation of Bridges with Lead Rubber Bearings and Friction Pendulum Bearings with Viscous Dampers. State University of New York at Buffalo, Master Project Report, Buffalo.
- California Department of Transportation (2004) Caltrans Seismic Design Criteria. Version 1.3. California Department of Transportation, Sacramento.
- Constantinou, M.C., Whittaker, A.S., Fenz, D.M. and Apostolakis, G. (2007) Seismic Isolation of Bridges. Version 2, Report to Sponsor, California Department of Transportation, Sacramento.
- CSI (2002) SAP 2000 Analysis Reference Manual. Computers and Structures Inc., Berkeley.
NOTES
*Corresponding author.









