Open Journal of Business and Management
Vol.05 No.03(2017), Article ID:77540,11 pages
10.4236/ojbm.2017.53041
Expressing Efficiency as a Function of Key Performance Control Parameters: A Case Study of Hydrocyclone Unit Process at Josay Goldfields Limited, Tarkwa, Ghana
Obiri Yeboah1, Sampson Arthur2
1Technical Service at Gold Fields Ghana Limited, Tarkwa, Ghana
2Production at Gold Fields Ghana Limited, Tarkwa, Ghana
Copyright © 2017 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: May 18, 2017; Accepted: July 8, 2017; Published: July 11, 2017
ABSTRACT
Optimization of gold liberation is a function of hydrocyclone (cyclone) classification efficiency with regard to a given target of 80% passing 75 µm at Josay Goldfields Limited. Key performance parameters that control the classification efficiency are hydrocyclone feed density, hydrocyclone feed pressure and throughput under fairly constant grinding process conditions. The hydrocyclone feed density related linearly to overflow product of percentage passing 75 µm and showed statistical linearity at even 1% critical level of significance. The paper provides a relation between cyclone feed density and cyclone overflow product size fraction as a function of cyclone efficiency. Gradient of the relation establishes the standard unit of performance which depicts the classification efficiency as percentage passing 75 µm per percentage solids of cyclone feed density. This measurement provides a timely corrective action of key performance control parameters. The selected seven days samples space used in the assessment was due to the effect of a daily production deficiency on the overall profit margin of Josay Goldfields as company.
Keywords:
Hydrocyclones, Percentage Passing, Classification, Lineality

1. Introduction
Efficiency is the relationship between the amount of input (energy) into a machine and the output or amount that it produces [1] [2] . Invariably, efficiency is a direct function of profit made by a company due to the output factor which has production potential with subsequent revenue generation. Hence, achieving higher efficiency is paramount in the life cycle of profitable businesses, including Josay Goldfields Limited. This study was carried out at Josay Goldfields Limited, Tarkwa in the Western Region of Ghana, to optimize leaching process of the Carbon in leach (CIL) plant. Generally, efficiency measurement indicates the level of output product per the input resource usage or the level of set target achieve (output) with reference to the resource usage. On the other hand, the extent of target achievement as compare to the set target, depicts effectiveness [3] . Conventionally, the efficiency of a hydrocyclone (cyclone) can be measured by estimating the probability of the desired particle size appearing in a classified product (i.e. the use of cyclone underflow product for estimation of cyclone d50 as a convention for cyclone efficiency deduction). The d50 measures the 50% probability that, a cut-off point of a given particle size fraction in the feed will report at the underflow (spigot product). Per design description from the [4] , Josay Goldfields Limited leaching process requires overflow product of particle size fraction of 80% passing 75 µm for effective dissolution of gold. This place is important on the efficiency of the classification unit which produces overflow product as feed for the dissolution of gold at the leaching unit. Technically, the key performance control parameters for cyclone overflow product of the classification process are cyclone feed density, cyclone feed pressure and mill feed rate (throughput) under fairly constant grinding process conditions. This places premium on the work of the Mineral Engineer to ascertain the fineness (size fraction) of the feed reporting as overflow and the parameters to be controlled in order to achieve the set target for optimum leaching process [5] . Normally, the turnaround time for evaluating the size fraction by the conventional d50 method at the Josay Goldfields Limited laboratory, is about 24 hours which always come as postmortem result with regards to the uninterrupted nature of the production plant process dynamics. This postmortem result prevents well-timed operational parameter control to gain the required size fraction for the leaching process which is fed with the overflow product from the classification unit process.
Consequently, this investigation provides an efficiency assessment alternative to close the lack of well-timed operating parameter control gap for the hydrocyclones unit at Josay Goldfields Limited. This paper aims at enhancing managerial assessment of classification (hydrocyclone) efficiency. The framework for the assessment covers the use of statistical analysis to measure the level of efficiency of a hydrocyclone classification unit. Primarily, the extent of relationship of the underlining assumptions (i.e. fairly uniform hydrocyclone feed pressure and throughput under constant grinding process conditions) and operating limitations were authenticated.
2. The Hydrocyclone at Josay Goldfields Limited
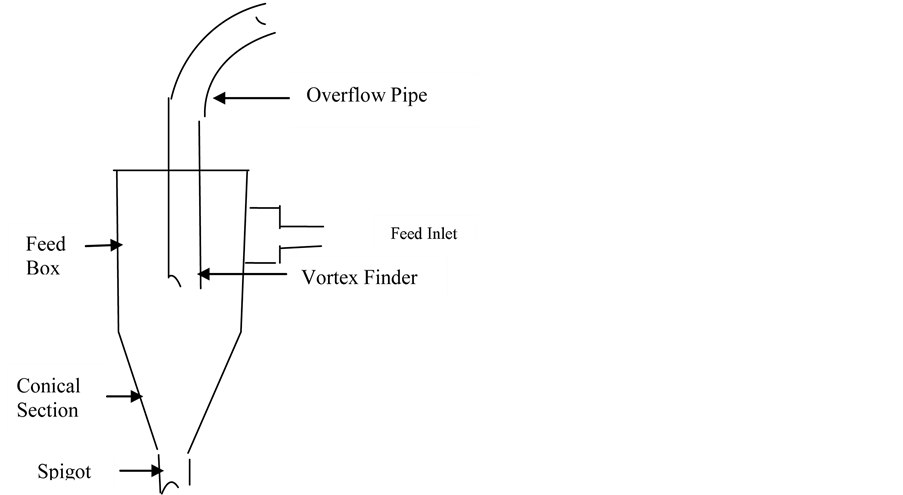
Conservatively, at Josay Goldfields Limited, hydrocyclone is used as classifying device that makes use of centrifugal force to increase the settling rate of particles. Two clusters of twenty four hydrocyclones are used at the classification unit process. Each hydrocyclone is made up of a cylindrical section (Feed box) connected to a conical shaped vessel with an opening at the apex (Spigot). The cylindrical section is closed off at the top with a pipe protruding into the body of the cyclone. This is called the vortex finder. The feed is introduced under pressure through the feed inlet, tangential to the cylindrical section of the cyclone. This produces a spiral motion, which generates a vortex in the cyclone with a low pressure zone along the vertical axis of the cyclone.
Operationally, particles in the pulp stream are subjected to an outward centrifugal force and inward drag force or centripetal force by the use of high capacity pump. The coarsest or heaviest particles are pulled by centrifugal force to the inner walls of the cyclone displacing the finer (i.e. lighter) particles and excess water towards the center. Thus particles are graded by size and mass from outside to inside of the spinning mixture. The coarsest or heaviest particles spiral down the walls and discharges through the apex as underflow product (spigot product).The finer or lighter particles and excess water report as overflow product. The split of particles is dependent on the balance between the centrifugal and drag forces. Hence the overall performance of a cyclone depends on the relative values of the radial and tangential velocities at all positions. Other factors that contribute to the overall cyclone performance are fluid viscosity, solids densities, fluids densities and effective values of cyclone components diameters [6] [7] . Figure 1 shows a sketch diagram of a Hydrocyclone at Josay Goldfields Limited.
3. Material and Method Used
Essentially, the inadequacy and ambiguity of information on the efficiency of
Figure 1. Sketch diagram of hydrocyclone at Josay Goldfields Limited.
hydrocyclone operation at the process plant is a recipe for production deficiencies. Therefore, hydrocyclone operation for seven days were collated from twelve months 2016 yearly production report to ascertain their respective efficiencies with regards to least square regression analysis equations. The seven days samples space was selected to reflect the sensitivity of a daily production deficiency of hydrocyclone operation on the profit margin of Josay Goldfields as company. That is each day’s deficiency of hydrocyclone operation has a direct reduction effect on the gold produce which is direct function of profit margin. Quantitative evaluations of the hydrocyclones overflow products were obtained to enhance pragmatic conclusions. [8] , affirmed the use of regression equation to predict values of a dependent variable from given values of one or more independent variable. From the 2016 yearly production report, different operating scenarios of cyclone feed parameters (input) and their corresponding overflow percentage passing 75 µm product (output), call for the use of statistical approach of regression analysis to evaluate the cyclone efficiency. Also, from [8] , the multi-stage sampling technique was used to form series of cluster sampling groups for mean representation which is tabulated as Table 1 and Table 2 for analysis.
4. Data and Analysis of Results
From the Josay Goldfields Limited 2016 yearly production report, quarterly clusters (three month per quarter) of hydrocyclone sample data were formed. Each quarterly data was evaluated to generate corresponding mean data of seven days for cyclone feed density, overflow density, overflow density of +75 µm, Mill feed rate and cyclone pressure at a sampling frequency of one hour interval. Subsequently, yearly mean data of seven days was obtained out of the quarterly data as shown in Table 1 and Table 2. Additionally, from the 2016 yearly production report description, each overflow sample was taken through wet sizing analysis using a screen with aperture of 75 µm. The respective +75 µm density was measured by the use of Marcy scale to deduce corresponding percentage -75 µm (percentage passing 75µm) from wet sizing log table (i.e. Log Table for percentage passing 75 µm with the ore Specific gravity (SG) of 2.7). Cyclone feed density, Mill feed rate and Cyclone pressure were taken from the automatic digital recorded reports [4] .
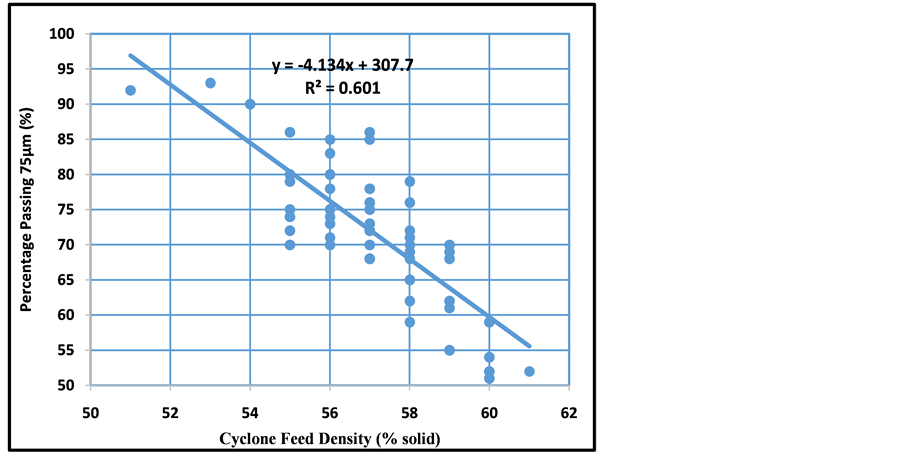
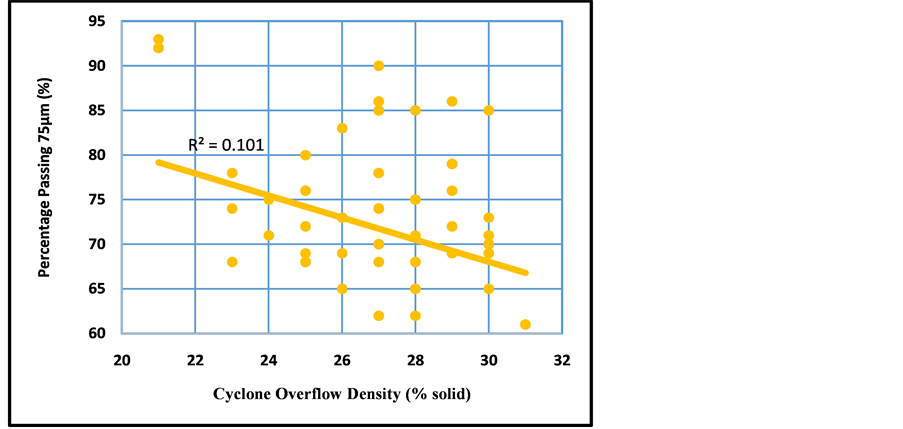
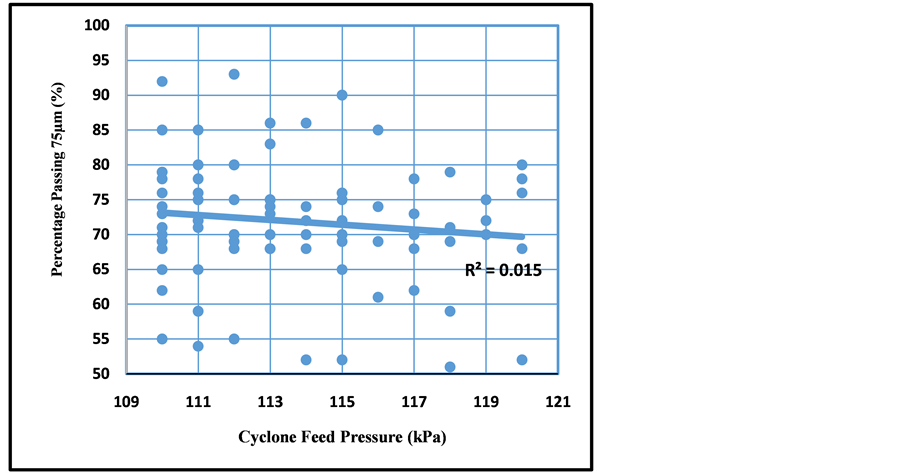
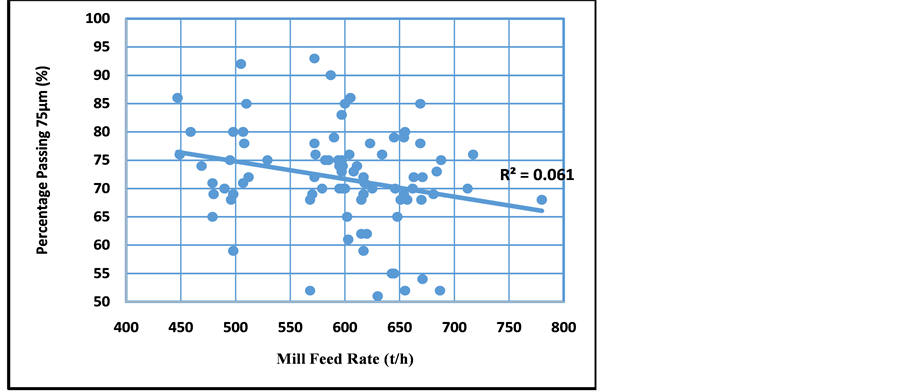
Subjecting each of the day’s feed densities and overflow percentage passing 75 µm variables to statistical linearity analysis, shows a linear relation at even 1% critical level of significance [9] [10] . Analytically, the regression of percentage passing 75 µm as Y-values which represent the output of the unit process on their respective inputs of cyclone feed density, overflow density, mill feed rate and cyclone pressure as X-values sequentially, gave corresponding linear graphs shown as Figures 2-5 with R2 of 0.6011, 01013, 0.0612 and 0.0151 for cyclone feed density, overflow density, mill feed rate and cyclone pressure respectively. The R2 for regression of percentage passing 75 µm on cyclone feed density, shows a strongest relation with highest dependency as compared to the other parameters [11] [12] . Consequently, the strongest R2 relationship factor points
Table 1. Hydrocyclone sample parameters for day 1 to 3.
Table 2. Hydrocyclone sample parameters for day 4 to 7.
Figure 2. Regression of Percentage passing 75 µm on Cyclone feed density.
to its regression equation variables as the most reliable parameters for controlling the cyclone efficiency.
5. Discussion
Basically, efficiency is given by the ratio of output to input and can be expressed as a percentage [13] . This implies, the input factor that shows the strongest relation with the output is the key parameter control factor. Hence, per the r2 values, the Figure 2 with linear equation; y = −4.1344x +307.77 and the highest r2 (i.e. r2 = 0.6011), depicts the most dependable relation with regard to key parameter controlling the cyclone efficiency as compared to Figures 3-5. The output product which is the cyclone overflow size fraction, is represented as percentage
Figure 3. Regression of Percentage passing 75 µm on Cyclone overflow density.
Figure 4. Regression of Percentage passing 75 µm on Cyclone feed pressure.
passing 75 µm (i.e. Y-axis) and input as cyclone feed density (i.e. X ? axis). This arrangement is in conformity with the operational principles of hydrocyclones which have cyclone feed density as input and cyclone overflow product as output. Pragmatically, the r2 of Figures 3-5 point to the fact that, the influence of these related parameters on the output cannot be neglected since r2 is not equal to zero. Therefore, these factors (input parameters of Figures 3-5) are to be kept under fairly uniform and constant state, to annul their effect on the overall result. That is, cyclone pressure and mill rate are to be kept under constant or uniform state as input parameters.
Figure 5. Regression of Percentage passing 75 µm on Mill feed rate.
The regression analysis equation (Y = a + bx) obtained from the Figure 2 data, shows negative sense (i.e. Negative gradient) [9] [11] [14] . This authenticates the fact that lower cyclone feed densities (input) favour higher percentage passing 75µm particle size fractions in the overflow products (output). That is, all things being equal, lower cyclone feed densities favour effective classification as result of favorable differential free settling condition created for the particles in the cyclone. The intercept on Y (percentage of −75 µm) axis is represented as “a” and the gradient is represented as “b” (i.e. Percentage passing 75 µm of overflow per percentage solids of feed density) in the equations. The gradient is therefore equal to output divided by input which gives a ratio that represents efficiency by definition. Therefore, gradients of the equations, express linear relationship between hydrocyclone feed density and overflow product size fraction as efficiency of the hydrocyclone unit process. Since the parameters for the equations are measured during the process running periods, well-timed parameter controls can be executed for efficiency improvement. The high linearity between cyclone feed density and percentage passing 75 µm variables, at even 1% critical level, justifies the reliability of this statistical method.
As the variable of x-axis approaches the higher cyclone feed percentage solids (i.e. x-intercept on the graphs), classification approaches a point of impossibility. That is, the classification process approaches a point of less water or liquid in cyclone feed content which is detrimental to differential settling enhancement within particles of the given slurry in the system. Convexly, as x (input or cyclone feed density) approaches zero (0), the y (output) approaches a point where there is less solid or only water flowing through the cyclone. The zero feed density corresponds to a point on y-axis, where practically only water comes as overflow which shows theoretical optimum percentage passing 75 µm as y-inter- cept on the graphs. Hence, per these deductions, the operational limits for this linear model are cyclone feed densities greater than zero and less than the point where classification is impossible. The linear regression method makes use of all variables of the individual samples under consideration, giving it a higher potential of a lower deviation as compared to conventional estimation of cyclone d50 method of forming a composite samples and subsequently dividing them for size analysis. Again, linear regression method gives a quicker and a more convenient method of estimating efficiency by expressing the efficiency as standard unit of the require size fraction of the cyclone feed that appears in the overflow product. Moreover, the linear relation method gives a better account of the end result by evaluating each sample value instead of conventional method which depends on average value with the probability of skewness. Furthermore, the linear representation offers the deduction within the managerial domain by assessing the cause and effect parameters that need attention to ensure high efficiency. Conversely, the conventional method expresses the probability of a particle size fraction appearance in at the underflow product (Spigot product) with ambiguity of assessment within the concepts outside the metallurgical domain.
6. Conclusion
The expression of linear relationship between hydrocyclone feed density and overflow product size fraction as a function of efficiency, enhances well-timed operational parameter control. This improves the production of required size fraction for the subsequent leaching process to the classification unit. On one hand, the conventional method is associated with errors that may occur in the numerous sequences of test works. It does not give direct relationship for immediate trouble shooting due to the long turnaround time of the test work process. On the other hand, linear relationship between hydrocyclone feed density and overflow product size fraction provides the means of control to achieve expected output target. This is due to short turnaround time of test work involved in the method and the expression of efficiency as percentage of the overflow percentage passing 75 µm (output) size fraction per percentage solid of the cyclone feed density (input). Additionally, statistical analysis of hydrocyclone efficiency has higher accuracy due to evaluation of the individual samples in sequential analysis as compared to conventional method of estimation under composite sampling process. The R2 for regression of overflow percentage passing 75 µm on cyclone feed density shows a strongest relation with highest dependency. This affirms the use of the linear relation between the two parameters as a control variable for the classification process. Notably, the assumptions for this linear model are cyclone feed density greater than zero (i.e. eliminating the occurrence of y-intercept situation) and less than the cyclone feed density at the point where classification is impossible (i.e. x-intercept on the graph). Limitation in this research is the existing probability of human error due to the manual wet size analysis for the determination of overflow percentage passing 75 µm. Finally, the linear representation enhances managerial evaluation of the cause and effects parameters that need attention to guarantee high efficiency.
Cite this paper
Yeboah, O. and Arthur, S. (2017) Expressing Efficiency as a Function of Key Performance Control Para- meters: A Case Study of Hydrocyclone Unit Process at Josay Goldfields Limited, Tarkwa, Ghana. Open Journal of Business and Management, 5, 476-486. https://doi.org/10.4236/ojbm.2017.53041
References
- 1. x Hornby, A.S. (2006) Oxford Advanced Learner’s Dictionary of Current English. Oxford University Press, Oxford, 469 p.
- 2. Patil, S.S., Verma, H.K. and Kumar, A. (2010) Efficiency Measurement of Hydro Machine by Thermodynamic Method, 182 p.
http://www.ighem.org/Paper2010/TSD05.pdf - 3. Lynch, F. (2004) Managing People. EPP Books Services, 75-76.
- 4. Production Report (2016) Production Report on Size Analysis 2016. Metallurgy (Technical) Department, Josay Goldfields Limited, Tarkwa, 1-8.
- 5. Wills, B.A. (1992) Mineral Processing Technology. Camborne School of Mines and Cornwall, England, 381-387.
- 6. Anon (1988) The Extraction and Recovery of Gold. Department of Minerals Engineering and Extractive Metallurgy Western Australian School of Mines, Australia.
- 7. Solberg, H.F. (1997) Hydrocyclone Efficiency. 2-4.
https://www.911metallurgist.com/blog/wp-content/uploads/2015/10/hydrocyclone-efficiency.pdf - 8. Saunders M., Lewis, P. and Thornhill, A. (2009) Research Methods for Business Students. 5th Edition, Pearson Education, Limited, Edinbuegh Gate, Harlow.
- 9. Al-Hassan, S. (2004) Lecture Notes on Statistical Models. MSc. Modular Programme,, Western University College, Tarkwa, 4, 11.
- 10. George, M.L., et al. (2005) The Lean Six Sigma Pocket Toolbook. McGraw-Hill, USA, 1-85.
- 11. Sharma, H. (2011) Predictive Analytics & Marketing—The Next Stage of Business Optimization. 24 p.
http://www.optimizesmart.com/introducing-predictive-marketing-stage-business-optimization - 12. Frost, J. (2013) Regression Analysis: How Do I Interpret R-Squared and Assess the Goodness-of-Fit?
http://blog.minitab.com/blog/adventures-in-statistics-2/regression-analysis-how-do-i-interpret-r-squared-and-assess-the-goodness-of-fit - 13. Nelkon, M. and Parker, P. (1995) Advanced Level Physics. 7th Edition, CBS Publishers & Distributors 11, Daryaganj, 46 p.
- 14. Michael, R. and Lindeburg, P.E. (1990) Engineer-in-Training Reference Manual. 8th Edition, Profession Publications, Inc. Belmont, 11-13.