ThereIsaWaytoComprise
Half-IntegerEigenvalues
forPhotonSpin
ShaoXuRen
InstituteofPhysicalScienceandEngineering
TongjiUniversity,200092,Shanghai,China
Received 13
July; Accepted 25 July; Published 28 July
———————————————————————————————————–
———————————————————————————————————–
Abstract
Inthisarticle,anattemptbasedonSpinTopologicalSpace,STS,togivea
reasonabledetailedaccountofthecauseofphotonicfermionizationphenomenaof
lightphotonismade.
STSisanunconventionalspinspaceinquantummechanics,whichcanbeused
toaccountforwheretheunconventionalhalf-integerspineigenvaluesphenomenon
oflightphotoncomesfrom.
Wesuggesttodectectthepossibleexistenceofphotonicone-third-spinization
phenomenonoflightphoton,byusingthreebeamsoflightphotonininterference
experiment.
Keywords
SpinTopologicalSpace,STS,Non-Hermitianmatrix,Casimiroperator,photonic
fermionizationphenomena,half-integerspineigenvalues,onethird,onefourthspin
eigenvaluesofphotonspin
———————————————————————————————————–
———————————————————————————————————–
1Introduction
KyleE.Ballantine,JohnF.DoneganandPaulR.Eastham[1]measuredthe
totalangularmomentumofthebeamoflightwiththeirinterferometer,and
observedsomecuriousopticalphenomena.Theyfound:theeigenvaluesofangular
momentumoflightphotonobviouslyshiftedawayfromthenormalphysicalvalues
thatareruledbythegeneralaxiomsacceptedintoday’squantummechanicsworld.
Normalangularmomentumquantumnumbersofthephotonmustbeintegers,in
unitsofthePlanckconstant:eigenvaluesofspinare−1,0,1andeigenvalues
oforbitalare0,1,2,3,...
However,asthetitleoftheirpaper,"Therearemanywaystospinaphoton:
Half-quantizationofatotalopticalangularmomentum"[1]shows:theexperimental
datain[1]werehalf-integer,/2and−/2,orevenmaybe1.5and−1.5...！
Itisanimportantphysicialexperimentresult,andindeed,lightphotonisboson,
howeverpossessesfermionic,spectrum!curiousphenomena...
Journal of Modern Physics, 2016, 7, 1364-1374
Published Online July 2016 in SciRes. http://www.scirp.org/journal/jmp
http://dx.doi.org/10.4236/jmp.2016.711123
How to cite this paper: Ren, S.X. (2016) There Is a Way to Comprise Half-Integer Eigenvalues for Photon Spin. Journal of
Modern Physics, 7, 1364-1374. http://dx.doi.org/10.4236/jmp.2016.711123

Thispresentarticle,"Thereisawaytocomprisehalf-integereigenvaluesfor
photonspin",isintheframeofSpinTopologicalSpace,STS[2]toconsiderthe
contributionsofspineffectsoflightphoton,andtriestoclearupthecauseofthe
photonicfermionizationphenomena,whichemergedfromtheexperiment[1].
Thecontributionsoforbitaleffectsoflightphoton,whichshowhalf-integer
eigenvalues,c ouldappealtothemechanismofNon-Hermitianorbitalangular
momentumL
3
,L
2
[3].
Normally,inquantummechanics,differentkindsofspinparticlespossess
differentdimensionalspaces,whichareexpressedbyfinitedimensionalmatrices,
andthesefinitedimensionalmatricesareallHermiticity.
AccordingtoSTS,spinangularmomentum
lofparticlesisexpressedby
infinitedimensionalmatricesinthree-physicalspace.Thefirstcomponent

1
land
thesecondcomponent

2
lareNon-Hermitianmatrices;thethirdcomponent
3
l
isHermitiandiagonalmatrix.Here,mark"l"indicatesthelthgenerationspin
particles,l1,2,3,...
2Threegroupsofmatrices

3,−3
(2),
2,−1
(1),
3/2,−3/2
(1)oflightphoton
particle
l,whichsatisfyspinangularmomentumcommutationrelus,playthe
majorroleinelaboratingthemachanismofphotonicfermionizationphenomena.

3,−3
(2)
3,−3
(2)i
3,−3
(2)(1)

2,−1
(1)
2,−1
(1)i
2,−1
(1)(2)

3/2,−3/2
(1)
3/2,−3/2
(1)i
3/2,−3/2
(1)(3)
Where

3,−3
(2){
1;3,−3
(2),
2;3,−3
(2),
3;3,−3
(2)}(4)

2,−1
(1){
1;2,−1
(1),
2;2,−1
(1),
3;2,−1
(1)}(5)

3/2,−3/2
(1){
1;3/2,−3/2
(1),
2;3/2,−3/2
(1),
3;3/2,−3/2
(1)}(6)
Orinsteadof(1),(2),(3),intermsofraisingmatrixoperator

j

,loweringmatrix
operator

k
−
and
3;j,k
,i.e.(7)below,torepresentcommutationrules(8),(9),(10)
oflightphotonwiththreedifferentkindsofspinstate(1):
{
j

(l),
k
−
(l),
3;j,k
(l)}(7)

3

(2)
−3
−
(2)–
−3
−
(2)
3

(2)2
3;3,−3
(2)(8.1)

3;3,−3
(2)
3

(2)–
3

(2)
3;3,−3
(2)
3

(2)(8.2)

3;3,−3
(2)
−3
−
(2)–
−3
−
(2)
3;3,−3
(2)−
−3
−
(2)(8.3)

2

(1)
−1
−
(1)–
−1
−
(1)
2

(1)2
3;2,−1
(1)(9.1)

3;2,−1
(1)
2

(1)–
2

(1)
3;2,−1
(1)
2

(1)(9.2)

3;2,−1
(1)
−1
−
(1)–
−1
−
(1)
3;2,−1
(1)−
−1
−
(1)(9.3)

3/2

(1)
−3/2
−
(1)–
−3/2
−
(1)
3/2

(1)2
3;3/2,−3/2
(1)(10.1)

3;3/2,−3/2
(1)
3/2

(1)–
3/2

(1)
3;3/2,−3/2
(1)
3/2

(1)(10.2)

3;3/2,−3/2
(1)
−3/2
−
(1)–
−3/2
−
(1)
3;3/2,−3/2
(1)−
−3/2
−
(1)(10.3)

Writedowntheexplicitrepresentationsofraisingmatrixoperatorsandlowering
matrixoperatorsthatappearintheabovethreef ormulas(8),(9),(10):

3

(2)
1
2
diag{,8,7,6,5,4,3,2,1,0,-1,-2,,}
2
(11)

−3
−
(2)
1
2
diag{,-2,-1,0,1,2,3,4,5,6,-7,-8,,}
−2
(12)

2

(1)diag{,7,6,5,4,3,2,1,0,-1,-2,-3,,}
1
(13)

−1
−
(1)diag{,-4,-3,-2,-1,0,1,2,3,4,5,6,,}
−1
(14)

3/2

(1)
1
2
diag{,13,11,9,7,5,3,1,-1,-3,-5,-7,,}
1
(15)

−3/2
−
(1)
1
2
diag{,-7,-5,-3,-1,1,3,5,7,9,11,-13,,}
−1
(16)
Subscripts"1"and"-1"representthef irstminortop-rightdiagonalandthe
firstminordown-leftdiagonal.
Subscripts"2"and"-2"representthesecondminortop-rightdiagonaland
thesecondminordown-leftdiagonal.
Subscripts"0"indicatesmajordiagonal,sometimesforconveniencebeomitted.
Inconditionforkeepingphoton’sCasimiroperatorinvariant,thatis,keeping

3,−3
2
(2)
2,−1
2
1
3/2,−3/2
2
1111I
0

2
2I
0

2
(17)
I
0
diag{,1,1,1,1,1,1,1,1,1,1,1,,}
0
(18)
Further,nextthreegroupsofmathseriesformsofthespinthirdcomponent

3
loflightphotonareobtainedasbelow

3;3,−3
(2)
diag{,3,2.5,2,
1.5,1,0.5,0,-0.5,-1,-1.5,–2,,}
0
(19)

3;2,−1
(1)
diag{,6,5,4,3,
2,1,0,-1,-2,-3,-4,,}
0
(20)

3;3/2,−3/2
1
diag{,5.5,4.5,3.5,2.5,
1.5,0.5,-0.5,-1.5,-2.5,-3.5,-4.5,,}
0
(21)
(19):AlternatingseriesformofIntegereigenvaluesandHalf-integereigenvalues
(20):Integereigenvaluesseriesform
(21):Half-integereigenvaluesseriesform
(19),(20),(21)arejustseperatelythefiguresofwhathappeninginKyleE.
Ballantine’sandhiscolleagues’experiment:
Integereigenvaluesseriesform(20)andhalf-integereigenvaluesseriesform(21)
givetheaccountsof"Onefamilyincludeshavetheexpectedbosonicspectrum
withintegereigenvalues,a ndotherf amily,hasafermionicspectrum,comprising
half-integereigenvalues."(quotedpassagefromthepaper[1]).

Bytheway,(20)
3;2,−1
(1)and(21)
3;3/2,−3/2
1,bothofthemaretogether
involvedin(19)

3;3,−3
(2).Soitseemsthatthereshoulde xistthethirdfamily,
alternatingseriesf ormofIntegereigenvaluesandHalf-integereigenvalues(19).
3Physicalbehaviormechanismofphotonicfermionizationoflightphoton
experiment
Now,matrices(8),(9),(10)canbeusedtodescribethee xperimentrusults(17)
and(19),(20),(21)ofphotonicfermionizationphenomenaoflightphoton,butfrom
whatkindofexperimentalprocedureofphysicalbehaviormechanism,these
experimentalresultsarise?
Forthisreason,deeperresearchisgiven.Beconcise,thesignof"(1)",is
omittedinf ollows.
Because

2,−1
and
m2,m−1
arespinangularmomentumsinSTS,itmeans：

2,−1

2,−1
i
2,−1
(2)

m2,m−1

m2,m−1
i
m2,m−1
(22)
Usingthelinearcombinanationof(2)with(22),anewspinangularmomentum

m/22, m/2−1
(23)iscomposed,anditobeyscommutationrule(24)

m/22, m/2−1

1
2
{
m2,m−1

2,−1
}...(23)

m/22, m/2−1

m/22, m/2−1
i
m/22, m/2−1
(24)

2,−1
,
m2,m−1
and
m/22, m/2−1
allarelightphoton,sincetheirCarsimir
operatorsequalto2
2
,i.e.

2,−1
2

m2,m−1
2

m/22, m/2−1
2
111I
0

2
2I
0

2
(25)
Writedownthethirdc omponentof(23),anditsexlicitformulation(26.m)as
below:

3;m/22, m/2−1

1
2
{
3;m2,m−1

3;2,−1
};m0,1,2,3,4,...(26.m)


3;4,1

1
2
{
3;6,3

3;2,−1
}
diag{,8,7,6,5,4,
3,2,1,0,-1,-2,,}(26.4)


3;7/2,1/2

1
2
{
3;5,2

3;2,−1
}

1
2
diag{,15,13,11,9,7,5,3,1,-1,-3,-5,,}(26.3)


3;3,0

1
2
{
3;4,1

3;2,−1
}
diag{,7,6,5,4,3,
2,1,0,-1,-2,-3,,}(26.2)


3;5/2,−1/2

1
2
{
3;3,0

3;2,−1
}

1
2
diag{,13,11,9,7,5,3,1,-1,-3,-5,-7,,}(26.1)


3;2,−1

1
2
{
3;2,−1

3;2,−1
}
diag{,6,5,4,3,2,
1,0,-1,-2,-3,-4,,}(26.0)


3;3/2,−3/2

1
2
{
3;1,−2

3;2,−1
}

1
2
diag{,11,9,7,5,3,1,-1,-3,-5,-7,-9,,}(26.-1)


3;1,−2

1
2
{
3;0,−3

3;2,−1
}
diag{,5,4,3,2,1,
0,-1,-2,-3,-4,-5,,}(26.-2)


3;1/2,−5/2

1
2
{
3;−1,−4

3;2,−1
}

1
2
diag{,9,7,5,3,1,-1,-3,-5,-7,-9,-11,,}(26.-3)


3;0,−3

1
2
{
3;−2,−5

3;2,−1
}
diag{,4,3,2,1,0,
-1,-2,-3,-4,-5,-6,}(26.-4)
From(26),twoimportantconclusionsaregiven
1)Thereexisttwodiffrentfamiliesofthethirdcomponentoflightphoton：
familyBP:BosonizationofPhoton,labelledby"",
familyFP:FermionizationofPhoton,labelledby""
Forlightphoton,theangularmomentumadditionoftwoangularmomentums,
oneangularmomentumBP
1
withotherangularmomentumFP
2
,maygeneratetwo
diffrentfamiliesofthethirdcomponentoflightphoton.
BPm
1
2
{BP
1
mBP
2
0};m0,2,4,6,...(27)
FPm
1
2
{BP
1
mBP
2
0};m1,3,5,7,...(28)
BPandFPalternatelyappearwithm.
2)Forafixedtermofthenewspinangularmomentum

3;m/22, m/2−1
,there
aremanyoptionstochoosef romthegeneralexpression(29).
BPm,m
′
,FPm,m
′

1
2
{BP
1
mBP
2
m
′
}(29)
m,m
′
0,1,2,3,...
FamilyBP(27)andFamilyFP(28)arethesimplestcouple,inwhichone
spinangularmomentumBP
2
0,
3;2,−1
iskeepinginvariant,asotherspinangular
momentumBP
1
m,
m2,m−1
varieswithminexpression
3;m/22, m/2−1
(26.m).
(20)

3;2,−1
(1)and(21)
3;3/2,−3/2
(1),whicharethepartofexpression

3;m/22, m/2−1
(26.m).Whenmequalsto0and-1,(20)and(21)arejust(26.0)
familyBP0and(26.-1)familyFP-1.
Therelationshipbetween(20)and(21),orequivalenttothatbetweenmatrices
(9)and(10),couldrefertothemathstatements(26.0)and(26.-1).Theyarethe
resultsoftheadditionsofspinangularmomentumphoton

3;2,−1
withphoton

3;2,−1
,andphoto
3;1,−2
withphoton
3;2,−1
inBallantine’sandhiscolleagues’
experiment.
Bytheway,theintervalsbetweentwoa djoiningBPandFPis

2
Δ
3;m/22, m/2−1
FPm1−BPm

2
I
0
(30)

4Predictionaboutone-third-spinizationphenomenonoflightphoton
Proceedinginaboveway,paralleltothemathstructureofthreegroupsof
matrices(11),(12)and(13),(14),(15),(16)f orphotonicfermionization,weguess
attheexistentofso-callphotonicone-third-spinizationphenomenonoflightphoton,
andfourgroupsofmatrices(31),(32)and(33),(34),(35),(36),(37),(38)aregiven,
belowlabelledby"♣".

4

(3)
1
2
diag{,9,8,7,6,5,4,3,2,1,0,-1,,}
3
(31)

−5
−
(3)
1
2
diag{,0,-1,-2,-3,-4,-5,-6,-7,-8,-9,-10,,}
−3
(32)

2

(1)diag{,7,6,5,4,3,2,1,0,-1,-2,-3,,}
1
(33)

−1
−
(1)diag{,-4,-3,-2,-1,0,1,2,3,4,5,6,,}
−1
(34)

5/3

(1)
1
3
diag{,20,17,14,11,8,5,2,-1,-4,-7,-10,,}
1
(35)

−4/3
−
(1)
1
3
diag{,11,8,5,2,-1,-4,-7,-10,-13,-16,-19,,}
−1
(36)

4/3

(1)
1
3
diag{,19,16,13,10,7,4,1,-2,-5,-8,-11,,}
1
(37)

−5/3
−
(1)
1
3
diag{,10,7,4,1,-2,-5,-8,-11,-14,-17,-20,,}
−1
(38)
Aswellasphoton’sCasimiroperator

4,−5
2
(3)
2,−1
2
1
5/3,−4/3
2
1
4/3,−5/3
2
1111I
0

2
2I
0

2
(39)
Accordingly,nextfourgroupsofmathseriesformsofthespinthirdcomponent

3
loflightphotonareobtainedasbelow

3;4,−5
3
diag{,2,5/3,4/3,1,2/3,
1/3,0,-1/3,-2/3,-1,-4/3,,}
0
(40)

3;2,−1
1
diag{,6,5,4,3,2,
1,0,-1,-2,-3,-4,,}
0
(41)
diag{,18/3,15/3,12/3,9/3,6/3,
3/3,0/3,-3/3,-6/3,-9/3,-12/3,,}
0

3;5/3,−4/3
1
diag{,17/3,14/3,11/3,8/3,5/3,
2/3,-1/3,-4/3,-7/3,-10/3,-13/3,,}
0
(42)

3;4/3,−5/3
1
diag{,16/3,13/3,10/3,7/3,4/3,
1/3,-2/3,-5/3,-8/3,-11/3,-14/3,,}
0
(43)
(41),(42),(43)combinetoform(40).Allofthemimplythatthethird
componenteigenvaluesoflightphotoncanbeinteger,one-third-integerseries.
Letushavesomeacquaintancewiththerelationshipamong(41),(42),(43),by
thegeneralformulaofadditionofspinangularmomentumoflightphoton(44.m).
Itisshownthat(41),(42),(43)are(44.0),(44.-1),(44.-2),whicharepartsof
generalformular(44)below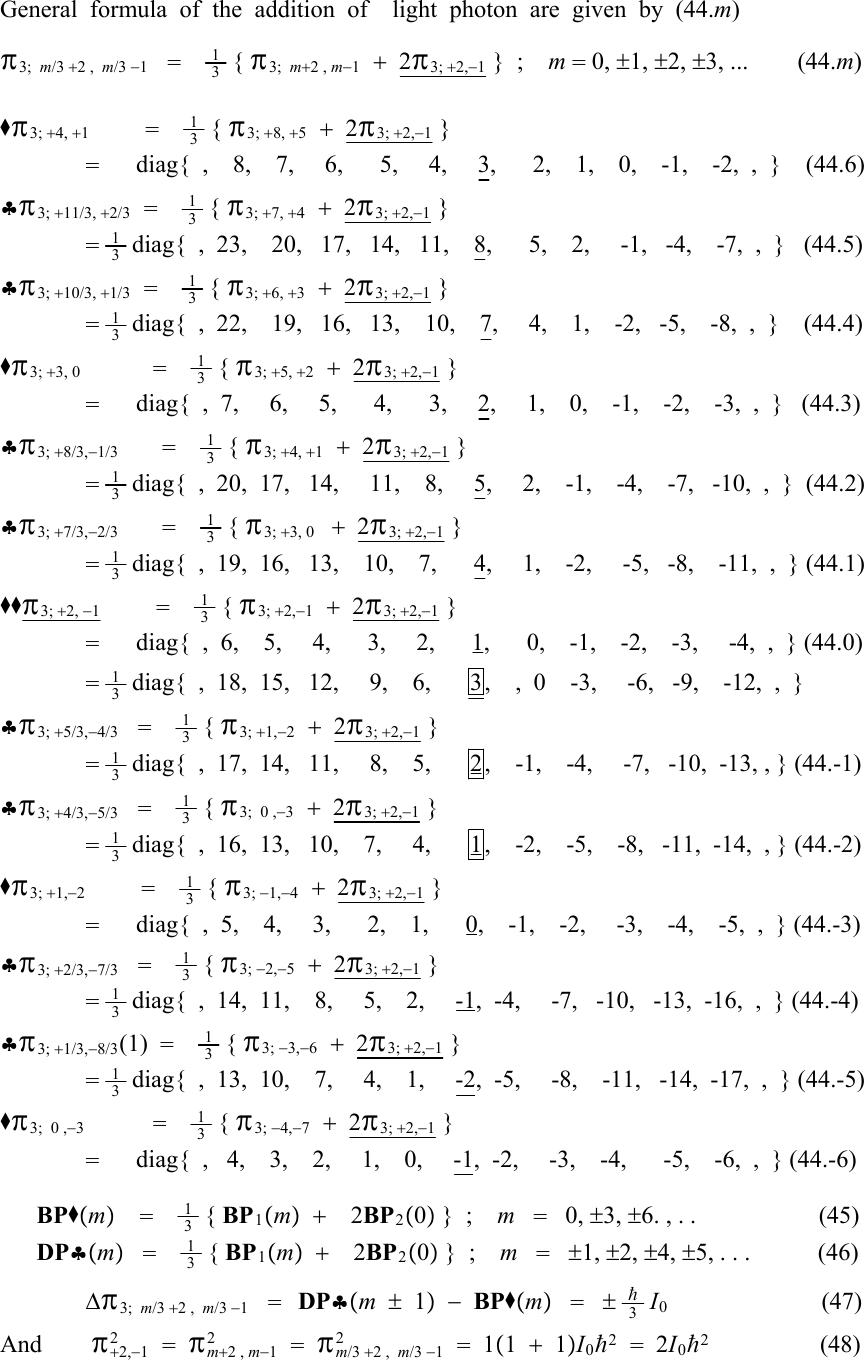

Generalformulaoftheadditionoflightphotonaregivenby(44.m)

3;m/32, m/3−1

1
3
{
3;m2,m−1
2
3;2,−1
}; m0,1,2,3,...(44.m)


3;4,1

1
3
{
3;8,5
2
3;2,−1
}
diag{,8,7,6,5,4,
3,2,1,0,-1,-2,,}(44.6)
♣

3;11/3,2/3

1
3
{
3;7,4
2
3;2,−1
}

1
3
diag{,23,20,17,14,11,8,5,2,-1,-4,-7,,}(44.5)
♣

3;10/3,1/3

1
3
{
3;6,3
2
3;2,−1
}

1
3
diag{,22,19,16,13,10,7,4,1,-2,-5,-8,,}(44.4)


3;3,0

1
3
{
3;5,2
2
3;2,−1
}
diag{,7,6,5,4,3,
2,1,0,-1,-2,-3,,}(44.3)
♣

3;8/3,−1/3

1
3
{
3;4,1
2
3;2,−1
}

1
3
diag{,20,17,14,11,8,5,2,-1,-4,-7,-10,,}(44.2)
♣

3;7/3,−2/3

1
3
{
3;3,0
2
3;2,−1
}

1
3
diag{,19,16,13,10,7,4,1,-2,-5,-8,-11,,}(44.1)


3;2,−1

1
3
{
3;2,−1
2
3;2,−1
}
diag{,6,5,4,3,2,
1,0,-1,-2,-3,-4,,}(44.0)

1
3
diag{,18,15,12,9,6,3, ,0 -3,-6,-9, -12,,}
♣

3;5/3,−4/3

1
3
{
3;1,−2
2
3;2,−1
}

1
3
diag{,17,14,11,8,5,2,-1,-4,-7,-10,-13,,}(44.-1)
♣

3;4/3,−5/3

1
3
{
3;0,−3
2
3;2,−1
}

1
3
diag{,16,13,10,7,4,1,-2,-5,-8,-11,-14,,}(44.-2)


3;1,−2

1
3
{
3;−1,−4
2
3;2,−1
}
diag{,5,4,3,2,1,
0,-1,-2,-3,-4,-5,,}(44.-3)
♣

3;2/3,−7/3

1
3
{
3;−2,−5
2
3;2,−1
}

1
3
diag{,14,11,8,5,2,-1,-4,-7,-10,-13,-16,,}(44.-4)
♣

3;1/3,−8/3
(1)
1
3
{
3;−3,−6
2
3;2,−1
}

1
3
diag{,13,10,7,4,1,-2,-5,-8,-11,-14,-17,,}(44.-5)


3;0,−3

1
3
{
3;−4,−7
2
3;2,−1
}
diag{,4,3,2,1,0,
-1,-2,-3,-4,-5,-6,,}(44.-6)
BPm 
1
3
{BP
1
m2BP
2
0}; m0,3,6.,..(45)
DP♣m
1
3
{BP
1
m2BP
2
0}; m1,2,4,5,...(46)
Δ

3;m/32, m/3−1
DP♣m1−BPm

3
I
0
(47)
And

2,−1
2

m2,m−1
2

m/32, m/3−1
2
111I
0

2
2I
0

2
(48)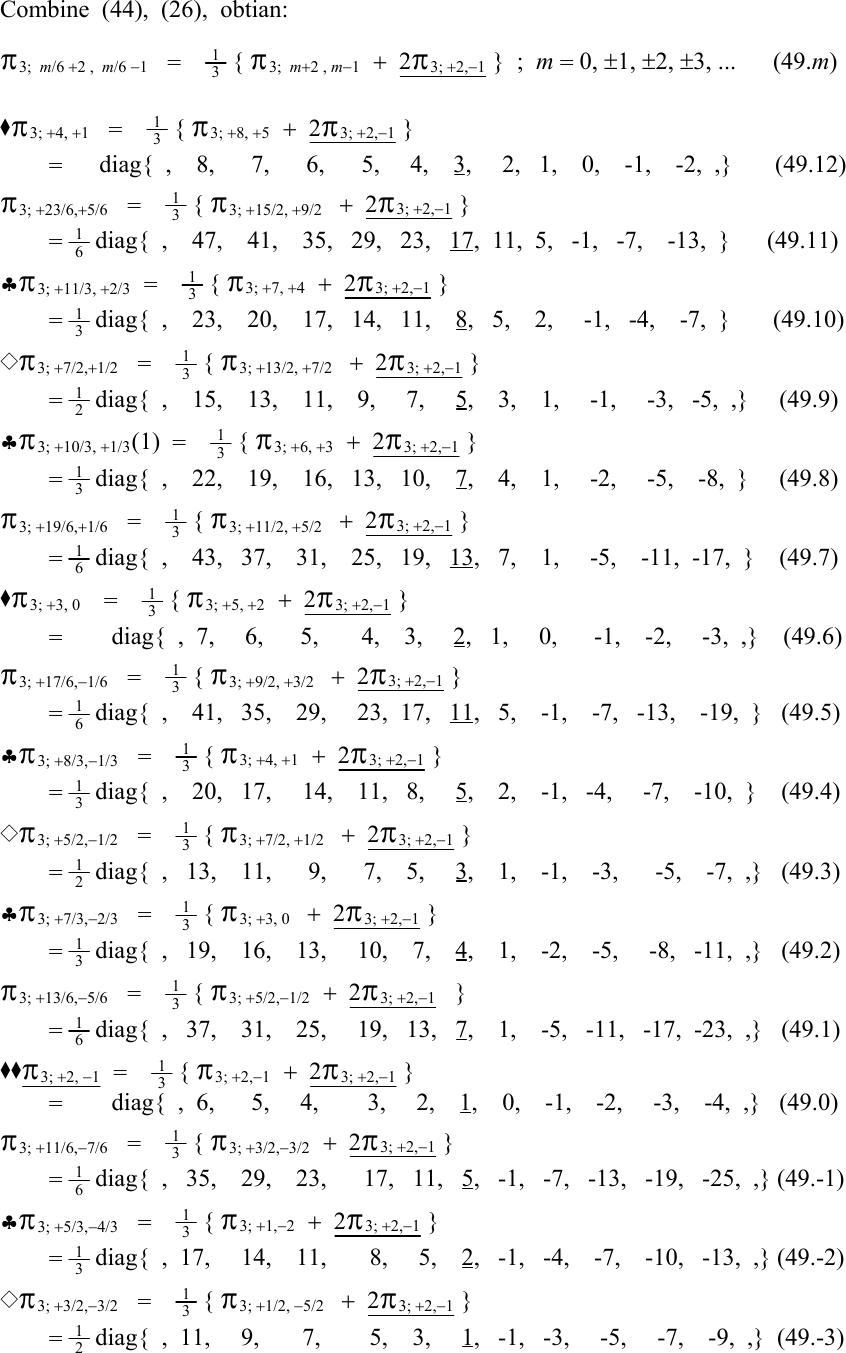

Combine(44),(26),obtian:

3;m/62, m/6−1

1
3
{
3;m2,m−1
2
3;2,−1
};m0,1,2,3,...(49. m)


3;4,1

1
3
{
3;8,5
2
3;2,−1
}
diag{,8,7,6,5,4,
3,2,1,0,-1,-2,,}(49.12)

3;23/6,5/6

1
3
{
3;15/2,9/2
2
3;2,−1
}

1
6
diag{,47,41,35,29,23,17,11,5,-1,-7,-13,}(49.11)
♣

3;11/3,2/3

1
3
{
3;7,4
2
3;2,−1
}

1
3
diag{,23,20,17,14,11,8,5,2,-1,-4,-7,}(49.10)


3;7/2,1/2

1
3
{
3;13/2,7/2
2
3;2,−1
}

1
2
diag{,15,13,11,9,7,5,3,1,-1,-3,-5,,}(49.9)
♣

3;10/3,1/3
(1)
1
3
{
3;6,3
2
3;2,−1
}

1
3
diag{,22,19,16,13,10,7,4,1,-2,-5,-8,}(49.8)

3;19/6,1/6

1
3
{
3;11/2,5/2
2
3;2,−1
}

1
6
diag{,43,37,31,25,19,13,7,1,-5,-11,-17,}(49.7)


3;3,0

1
3
{
3;5,2
2
3;2,−1
}
diag{,7,6,5,4,3,
2,1,0,-1,-2,-3,,}(49.6)

3;17/6,−1/6

1
3
{
3;9/2,3/2
2
3;2,−1
}

1
6
diag{,41,35,29,23,17,11,5,-1,-7,-13,-19,}(49.5)
♣

3;8/3,−1/3

1
3
{
3;4,1
2
3;2,−1
}

1
3
diag{,20,17,14,11,8,5,2,-1,-4,-7,-10,}(49.4)


3;5/2,−1/2

1
3
{
3;7/2,1/2
2
3;2,−1
}

1
2
diag{,13,11,9,7,5,3,1,-1,-3,-5,-7,,}(49.3)
♣

3;7/3,−2/3

1
3
{
3;3,0
2
3;2,−1
}

1
3
diag{,19,16,13,10,7,4,1,-2,-5,-8,-11,,}(49.2)

3;13/6,−5/6

1
3
{
3;5/2,−1/2
2
3;2,−1
}

1
6
diag{,37,31,25,19,13,7,1,-5,-11,-17,-23,,}(49.1)


3;2,−1

1
3
{
3;2,−1
2
3;2,−1
}
diag{,6,5,4,3,2,1,0,-1,-2,-3,-4,,}(49.0)

3;11/6,−7/6

1
3
{
3;3/2,−3/2
2
3;2,−1
}

1
6
diag{,35,29,23,17,11,5,-1,-7,-13,-19,-25,,}(49.-1)
♣

3;5/3,−4/3

1
3
{
3;1,−2
2
3;2,−1
}

1
3
diag{,17,14,11,8,5,2,-1,-4,-7,-10,-13,,}(49.-2)


3;3/2,−3/2

1
3
{
3;1/2,−5/2
2
3;2,−1
}

1
2
diag{,11,9,7,5,3,1,-1,-3,-5,-7,-9,,}(49.-3)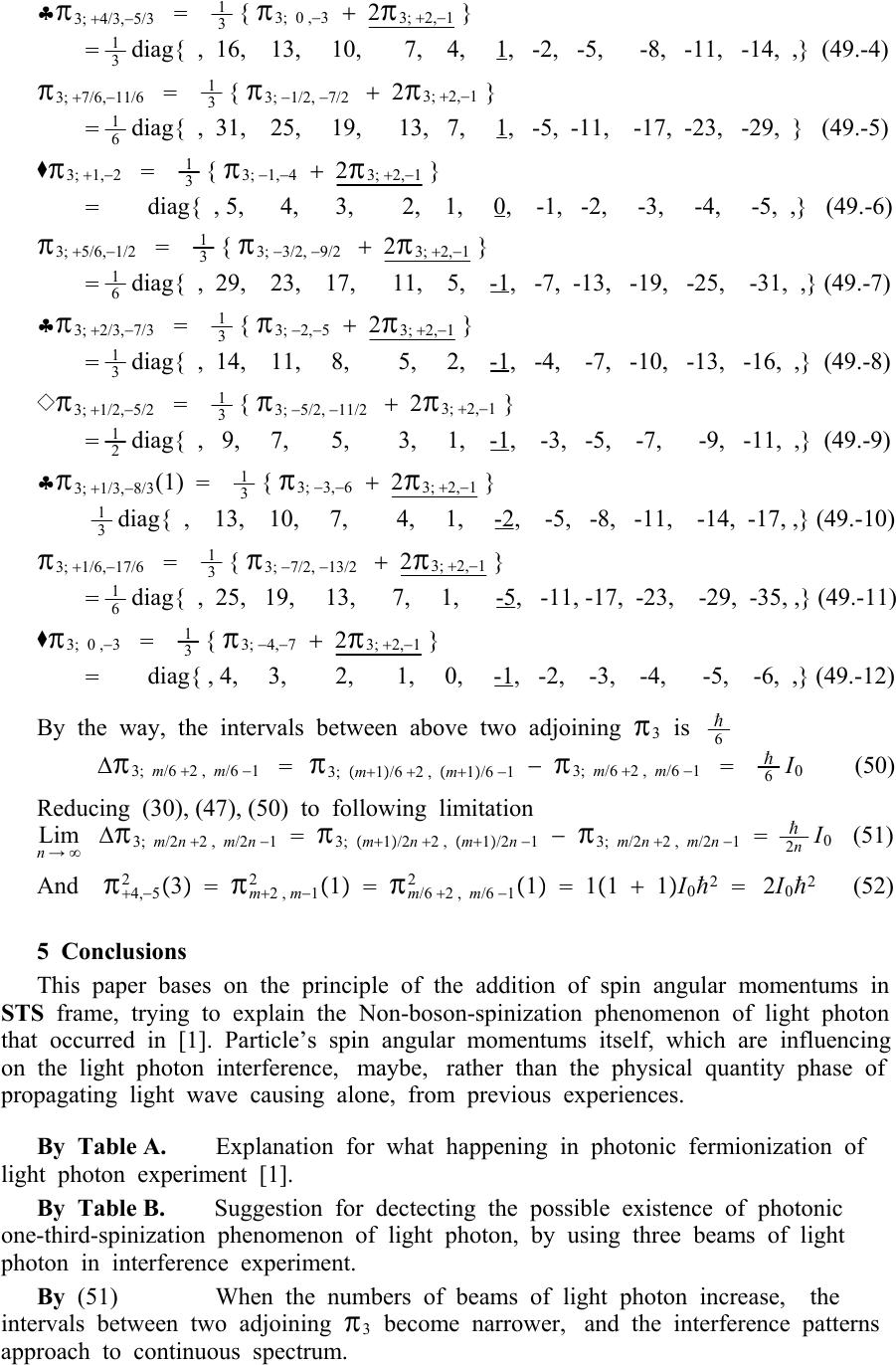

♣
3;4/3,−5/3

1
3
{
3;0,−3
2
3;2,−1
}

1
3
diag{,16,13,10,7,4,1,-2,-5,-8,-11,-14,,}(49.-4)

3;7/6,−11/6

1
3
{
3;−1/2,−7/2
2
3;2,−1
}

1
6
diag{,31,25,19,13,7,1,-5,-11,-17,-23,-29,}(49.-5)


3;1,−2

1
3
{
3;−1,−4
2
3;2,−1
}
diag{,5,4,3,2,1,
0,-1,-2,-3,-4,-5,,}(49.-6)

3;5/6,−1/2

1
3
{
3;−3/2,−9/2
2
3;2,−1
}

1
6
diag{,29,23,17,11,5,-1,-7,-13,-19,-25,-31,,}(49.-7)
♣

3;2/3,−7/3

1
3
{
3;−2,−5
2
3;2,−1
}

1
3
diag{,14,11,8,5,2,-1,-4,-7,-10,-13,-16,,}(49.-8)


3;1/2,−5/2

1
3
{
3;−5/2,−11/2
2
3;2,−1
}

1
2
diag{,9,7,5,3,1,-1,-3,-5,-7,-9,-11,,}(49.-9)
♣

3;1/3,−8/3
(1)
1
3
{
3;−3,−6
2
3;2,−1
}
1
3
diag{,13,10,7,4,1,-2,-5,-8,-11,-14,-17,,}(49.-10)

3;1/6,−17/6

1
3
{
3;−7/2,−13/2
2
3;2,−1
}

1
6
diag{,25,19,13,7,1,-5,- 11,-17,-23,-29,-35,,}(49.-11)


3;0,−3

1
3
{
3;−4,−7
2
3;2,−1
}
diag{,4,3,2,1,0,
-1,-2,-3,-4,-5,-6,,}(49.-12)
Bytheway,theintervalsbetweenabovetwoadjoining

3
is

6
Δ
3;m/62, m/6−1

3;m1/62, m1/6−1
−
3;m/62, m/6−1


6
I
0
(50)
Reducing(30),(47),(50)tofollowinglimitation
n
LimΔ
3;m/2n2, m/2n−1

3;m1/2n2, m1/2n−1
−
3;m/2n2, m/2n−1


2n
I
0
(51)
And

4,−5
2
3
m2,m−1
2
1
m/62, m/6−1
2
1111I
0

2
2I
0

2
(52)
5Conclusions
Thispaperbasesontheprincipleoftheadditionofspinangularmomentumsin
STSframe,tryingtoexplaintheNon-boson-spinizationphenomenonoflightphoton
thatoccurredin[1].Particle’sspinangularmomentumsitself,whichareinfl uencing
onthelightphotoninterference,maybe,ratherthanthephysicalquantityphaseof
propagatinglightwavecausingalone,frompreviousexperiences.
ByTableA.Explanationforwhathappeninginphotonicfermionizationof
lightphotonexperiment[1].
ByTableB.Suggestionfordectectingthepossibleexistenceofphotonic
one-third-spinizationphenomenonoflightphoton,byusingthreebeamsoflight
photonininterferenceexperiment.
By(51)Whenthenumbersofbeamsoflightphotonincrease,the
intervalsbetweentwoa djoining

3
becomenarrower,andtheinterferencepatterns
approachtocontinuousspectrum.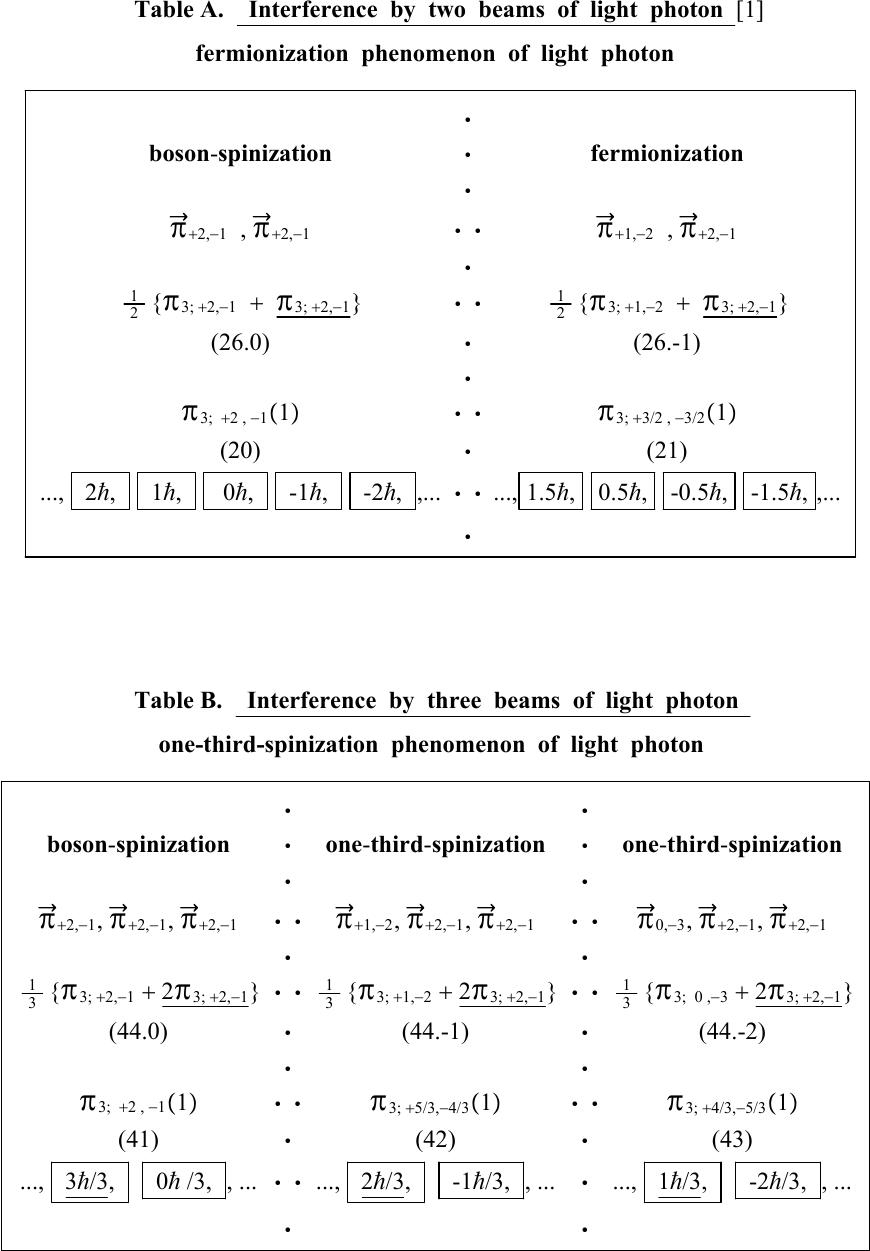

TableA.Interferencebytwobeamsoflightphoton[1]
fermionizationphenomenonoflightphoton

boson-spinizationfermionization


2,−1
,
2,−1

1,−2
,
2,−1

1
2
{
3;2,−1

3;2,−1
}
1
2
{
3;1,−2

3;2,−1
}
(26.0)(26.-1)


3;2,−1
1
3;3/2,−3/2
1
(20)(21)
...,2,1,0,-1,-2,,......,1.5,0.5,-0.5,-1.5,,...

TableB.
Interferencebythreebeamsoflightphoton
one-third-spinizationphenomenonoflightphoton

boson-spinizationone-third-spinizationone-third-spinization


2,−1
,
2,−1
,
2,−1

1,−2
,
2,−1
,
2,−1

0,−3
,
2,−1
,
2,−1

1
3
{
3;2,−1
2
3;2,−1
}
1
3
{
3;1,−2
2
3;2,−1
}
1
3
{
3;0,−3
2
3;2,−1
}
(44.0)(44.-1)(44.-2)


3;2,−1
1
3;5/3,−4/3
1
3;4/3,−5/3
1
(41)(42)(43)
...,
3/3,0/3,,......,2/3,-1/3,,... ...,1/3,-2/3,,...


References
[1]KyleE.Ballantine,JohnF.DoneganandPaulR.Eastham
ScienceAdvances29Apr2016:Vol.2,No.4,e1501748
DOI:10.1126/sciadv.1501748
[2]ShaoXuRenTheOriginsofSpinsofElementaryParticles
ISBN978-988-13649-7-5(2014);
JournalofModernPhysics,5,1848-1879
http:/dx.doi.org/10.4236/jmp.2014.517181;
InteractionoftheOriginsofSpinAngularMomentum
ISBN978-988-14902-0-9(20162ndedition);
JournalofModernPhysics,7,737-759
http:/dx.doi.org/10.4236/jmp.2016.78070
[3]ShaoXuRenTheThirdKindofParticlesISBN978-7-900500-91-5(2011),
ISBN978-988-15598-9-0(2012),ISBN988-3-659-17892-4(2012);
JournalofModernPhysics,5,800-869
http:/dx.doi.org/10.4236/jmp.2014.59090

Submit or recommend next manuscript to SCIRP and we will provide best service for you:
Accepting pre-submission inquiries through Email, Facebook, LinkedIn, Twitter, etc.
A wide selection of journals (inclusive of 9 subjects, more than 200 journals)
Providing 24-hour high-quality service
User-friendly online submission system
Fair and swift peer-review system
Efficient typesetting and proofreading procedure
Display of the result of downloads and visits, as well as the number of cited articles
Maximum dissemination of your research work
Submit your manuscript at: http://papersubmission.scirp.org/