Open Access Library Journal
Vol.03 No.04(2016), Article ID:69224,12 pages
10.4236/oalib.1102141
Gravitation
Angel Fierros Palacios
División de Energías, Instituto de Investigaciones Eléctricas, Alternas, México

Copyright © 2016 by author and OALib.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


Received 9 April 2016; accepted 25 April 2016; published 29 April 2016

ABSTRACT
In this paper, it is proved that the small deformation strain tensor can be used instead the fundamental metric tensor of the General Theory of Relativity, in order to formulate a Dynamic Theory of Gravitation. Also, a solution of the velocity of the gravitational interactions is given in terms of the escape velocity due to the apparent size of the heavenly bodies. This last paragraph is the motivation and the importance of the study here presented. Thus, when it has a couple of celestial bodies separated by a distance in space, its apparent sizes as seemed at a distance play a special role in the gravitational interactions. This is so because of some effect over the size due to the very big distance in space. In that situation, the values of their escape velocities are dependent on their mass, and critically on their apparent radius. It is proved that they are the medium used by the gravity to transmit its effects like propagating force of nature. Then, when the escape velocities meet in some point of the space between the bodies, they pull each other; because they are the carriers of the respective attractive gravitational fields. In other words, the escape velocity due to the apparent size is the exchanging coin in the gravitational interactions. Also it is proposed that such a dynamic process is the responsible for the strong link which is established between any couple of interacting heavenly objects in the Universe.
Keywords:
Small Deformation Strain Tensor, Velocity of the Gravitational Interactions, Escape Velocity
Subject Areas: Classical Mechanics

1. Introduction
Within the theoretical frame of classical fluid dynamics [1] , it is demonstrated that under the action of applied forces, the region occupied by some continuous medium exhibits deformations; which can be described mathematically by the small deformation strain tensor.
Consider an ordered set of N real variables xi, i = 1, 2, … N. These variables are called the coordinates of a point, in such a way that with all the points corresponding to an all values of the coordinates it has an N-dimen- tional space. In it let’s consider a region, and also two points close together. If over the boundary surface of the region is applied an external dynamic agent, the region is deformed. In order to mathematically describe that deformation, the procedure is as follows. The distance between the point before and after deformation is

and
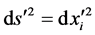 (1)
(1)
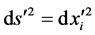
where 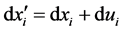 and
and 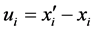 are the components of the displacement vector while dxi the i-component of the radius vector joining the points before the deformation, and dui , the radius vector joining them after deformation [2] . For small deformations, and from the second of the Equations (1), it can be obtained that
are the components of the displacement vector while dxi the i-component of the radius vector joining the points before the deformation, and dui , the radius vector joining them after deformation [2] . For small deformations, and from the second of the Equations (1), it can be obtained that
 (2)
(2)
where
 (3)
(3)
are the components of the small deformation strain tensor. On the other hand, after deformation the interval ds’2 between the nearby points can be written as follows:
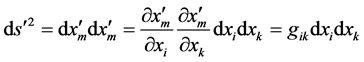 (4)
(4)
where
 (5)
(5)
are the components of the fundamental metric tensor. Then, if instead considering that the points are separate; we make them coincide in the undeformed initial situation; it is obtained that
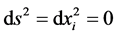 (6)
(6)
So that in (2) it has that
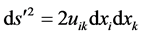 (7)
(7)
If the Equations (4) and (7) are compared it is obtained that [2] .
 (8)
(8)
The Equation (8) must be considered as a relation of congruence between Physics and Geometry, more than and equality [2] . This is so, because the General Theory of Relativity, constructed in terms of the fundamental metric tensor, is a geometrical description of the Universe, where forces and moment are not taken into account; in such a way that is very difficult to explain the space-time deformation, and the movement of the bodies, without the action of some dynamical agent; whereas the Dynamic Theory of Gravitation considers dynamical agents by means of the small deformation strain tensor. Those theories can be equally applied to object that travel at velocities lesser than the speed of light in vacuum; in such a way that the relation (8) makes congruent the geometrical description of the Universe, and the correspondent dynamical descriptions. From the mathematical viewpoint, both tensors are equivalents. That means that both tensors have the same properties [2] . However, from the physical viewpoint, those tensors have different significance. The small deformation strain tensor describes the geometrical properties of any region of the continuous space-time when is deformed because of the effect of some dynamic agent. On the other hand, the fundamental metric tensor describes the metrical properties of the same region of the continuous space-time, but once it is deformed. Thus, in one member of the relation (8), it has a dynamical object, and in the other a geometrical entity. Then, that fact gives the possibility to formulate a Dynamical Theory of Gravitation [2] . It is possible to demonstrate that from the small deformation straintensor, all the mathematical structures are needed to formulate that theory can be obtained.
Mathematical Structures
It is possible to prove that the expressions obtained are equal of those obtained using the fundamental metric tensor; that is to say
The Christoffel Symbols
The Differential Equation of a Geodesic
The Covariant Derivative of a Vector
The Covariant Derivative of a Tensor
The Riemann-Christoffel Tensor
Christoffel’s symbols are two quantities which are not tensors, called some times as the three-index symbols of the first and second kind [2] . They has the following form
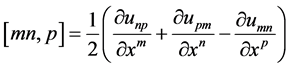
and
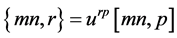 (9)
(9)
Those symbols defined in terms of the small deformation strain tensor, can be written as follows [mn, p] and {mn, r}. They have the important properties that there are symmetrical in m, n [2] .
The differential equation of a geodesic, which is the path between two points in the Riemannian space, is obtained using the calculus of variation, and the following condition
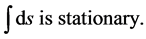 (10)
(10)
This absolute track is of fundamental importance in dynamics. Keeping the beginning and the end of the path fixed, we give every intermediate point an arbitrary infinitesimal displacement dxs so as to deform the path. The stationary condition is

According to definition (9) and making some algebra, it can be obtained the following result, which is the looked for differential equation

Now to obtain the covariant derivative of a vector, the procedure is as follows. Since dxm is a contravariant vector, and ds an invariant, dxm/ds a kind of velocity, is a contravariant vector. Hence if Am is any covariant vector, the inner product

The rate of change of this expression per unit interval ds along any assigned curve must also be independent of the coordinate system; that is to say

Again, and making some algebra, it can be obtained that [2]

This is called the covariant derivative of Am. The tensors 

The covariant derivatives of tensors of the second rank are formed as follows [2]



Finally, it is possible to construct the following expression [2]

This is called the Riemann-Christoffel Tensor [2] 1.
Thus, a stage has been reached at which the results obtained can be applied to the theory of gravitation. From the contracted Riemann-Christoffel Tensor it is obtained that [2] .

where u is the determinant of umu . The expression

in empty space, is chosen as the law of gravitation in a Dynamic Theory of Gravitation [2] .
At is easy to see, all those mathematical formulae have the same structure that those of the General Theory of Relativity, but in terms of the small deformation strain tensor. So that, it is possible to formulate a dynamical description of the Universe, instead of the General Relativity geometrical description [2] .
However, both theories give the same results when the speed of the objects is lesser than the speed of light in vacuum. But in the case of the gravitational interactions, the situation is different, because the speed of gravity is always greater than the light velocity. Apparently, gravity in contrast to light, has no detectable aberration or propagation delay for its action.
Newton’s Law of Gravitation
The most amazing thing about the celestial mechanics is that all gravitational interactions between bodies in all dynamical systems had to be taken as instantaneous. However, experiment shows that instantaneous interactions do not exist in nature. No one has expressed the objection to a such situation than I. Newton. He says that it is absurd to consider that one body may act upon another at a distance through a vacuum, without the mediation of anything else. But mediation requires propagation, and finite bodies should be incapable to propagate at infinite speeds since that would require infinite energy [3] . Einstein’s Special Theory of Relativity, an experimentally well established theory, proved that nothing with proper mass non-zero, could propagate at a speed greater than light in vacuum [3] .
Indeed, it is widely accepted that the velocity of gravity in Newton’s Universal Law is unconditionally infinite. This fact is not mentioned in the statement that General Relativity reduces to Newtonian Gravity in low-velocity, weak-field limit, because of the question it begs about how that can be true if the propagation speed in one model is the speed of light, and in the other model is infinite [3] . There are many objections related to that topic; in such a way that in discussions of gravity, the most frequently asked question is, what is the speed of gravity? and also, what is the exchanging object in the gravitational interactions?
The problem arises since Newton’s Discovery of the Law of Universal Gravitation,

That law expressed the fact that the force in magnitude between two particles having mases m1 and m2 separated by a distance r is an attraction acting along the line joining the particles. In that relationship G is a universal constant having the same value for all pairs of particles [4] .
It is important to say at once some features of this law to understand it clearly. The gravity forces between two particles are an action-reaction pair. On the other hand, the question of what means the distance between heavenly bodies arises immediately. In the case of the gravitational interaction between the Moon and the Earth, Newton regarded every particle of the Earth as contributing to the gravitational attraction it had on the Moon; but from different distances and directions. Then, Newton made the daring assumption that the Earth could be treated for such a proposal as if all the mass were concentered at its center. In other words, the Earth and also the Moon could be treated as interacting particles. That assumption was proved by Newton when he invent the Calculus.
However, that assumption is very important for any couple of interacting celestial objects. Perhaps, Newton considered that the apparent size of the bodies as seemed at a distance, as a useful concept.
The Speed of Gravity
The gravitational interactions between bodies is described in ordinary mechanics by means of a potential energy which appears as a function of the coordinates of the interacting bodies; and contains the assumption of instantaneous propagation, which is unaccepted because it seems to be a form of action at a distance. However, it can be shown that the gravity is a very fast propagating force greater than the light velocity in vacuum.
Let us consider now, the fundamental quadratic form, but in terms of the components of the small deformation strain tensor.
The interval between two neighboring events with coordinates (x1, x2, x3, x4) and (x1 + dx1, x2 + dx2, x3 + dx3, x4 + dx4)

where the coefficients u11, etc. are functions of x1, x2, x3, x4. That is to say, ds2 is some quadratic function of the differences of coordinates [5] .
Let us assume that there is a small region of the space throughout which the u’s can be treated as constants. In that case, and making a change of variables [5] , the right-hand side of (23) can be broken up into the sum of squares; in such a way that it is obtained [5]

However, that reduction is not in general possible for a large region, where the u’s have to be considered as functions, not as constants. Consider all the events for which y4 has some specified value. These will form a three-dimensional space. Since dy4 is zero for every pair of events, their mutual intervals are given by

But this is exactly like familiar space in which the interval is given by [3]

where x, y, z are rectangular coordinates.
Hence a section of the world by y4 = constant would appear as space, and y1, y2, y3 will appear as rectangular coordinates. The coordinate-frames y1, y2, y3 and x, y, z are examples of two systems s and s’ for which the intervals between corresponding pairs of mesh-corners are equal [5] . The two systems are therefore exactly alike observationally; and if one appears to be a rectangular frame in space, so also must the other [5] . Clearly, the coordinates for real events must be real. Taken into account the last observation, the reduced general expression is

Clearly y4 must involve the time, otherwise the location of events by the four coordinates would be incomplete. Consider that the following definition of equal time-intervals is accepted, if it has some mechanism capable of cyclic motion, in which its cycles will measure equal durations of time anywhere and anywhen, provided the mechanism, its laws of behavior, and all outside influences remain similar [5] . To this, the theory of relativity would add the condition that the mechanism must be at rest in the space-time frame considered, because it is well known that a clock in motion goes slower in comparison with a fixed clock [5] . Since then, it is agreed that the mechanism as a whole is to be at rest, and the moving parts return to the same positions after a complete cycle, it has for any pair of events marking the beginning and the end of the cycle that
in such a way that according to (27) it has that

Then, the cycles in all cases correspond to equal intervals ds. Hence, they correspond to equal values of dy4 . But by the above definition of time, they also correspond to equal lapses of time dt. Then, it must have dy4 proportional to dt; in such a way that it is proposed the following proportionality relationship

this is so, because there are some observational results which points out toward the fact that the velocity of the gravitational interactions is always greater than the speed of light in vacuum [3] . In the relation (28), 
a number which for the case of gravity is n > 1 always; and n ≤ 1 for the case of the material finite bodies, and also for de photons; and c the light speed, considered as the fundamental velocity in the Special Theory of Relativity. Then (27) becomes

The velocity of gravity
It is important to say that in relativity all the last it was said is subjected to the condition that it has dealing with a region of the space in which the g’s, and also the u’s for the gravity, are constants, or approximately constants. A region having that property is called a flat space; and the appropriate theory, is called the Einstein´s Special Theory of Relativity; in which is established the velocity of light as the greatest speed in the Universe. That theory proved that no material finite body could propagate beyond the light barrier. But in the gravitational interactions, this is not the case, because the gravity is a propagating force of Nature without mediation of anything, apparently [5] . In other words, is not a pure geometrical effect of curved space-time, which travel at light speed, as it is suggested by the General Theory of Relativity. In reality, the velocity of the gravitational interactions is the greatest speed of the Universe.
Now from (29) it is follows that when

ds = 0. This is important because in the Special Theory of Relativity, the track of a light-wave is a geodesic with ds = 0; and it is included there the identification of the light with the fundamental velocity [3] . Hence, in a Dynamic Theory of Gravitation, the track of the escape velocity, due to the apparent size of the celestial objects as a fundamental velocity; and which is the medium used by the gravity to transmit its effects like propagating force of nature, must also be a kind of geodesic with ds = 0 [5] . Then it is proposed that

where vg is the velocity of the gravitational interactions. Clearly.

Moreover, it can be proposed that vg is equal to the escape velocity of any massive source of gravitational force, which has an apparent size due to some kind of effect produced by the very big distances between the bodies in space.
The Lorentz transformation
Let us consider the following transformation of coordinates

where

and, w is any real constant not greater than c. It can be demonstrate that

Hence, from the condition about equal intervals, 

it has that

This is the Lorentz transformation. The essential property of the foregoing transformation is that it leaves de formula for ds2 unaltered. It is valid when the velocity of any object with proper massnon-zero is lesser than the speed of light in vacuum. That is to say, w < c for all finite objects, and w = c, only for the photon [5] .
However, let us consider that in the case of the speed of gravity w= nc, with n a number which can be very big. Then, the new transformation of coordinates is

in such a way that

It can be proved that

But, w2/n2 = c2, which is again the Equation (35)
So, in that case it is always true that the intervals ds2 and 
The velocity of the gravitational interactions
When the traditional experimental and scientific research methods are used to determine the velocity of gravity, they always yield propagation speeds of gravity faster than light speed. This is so, because the gravitational force, in contrast to light, apparently has no any propagation delay for its action. The finite value of light speed causes not only aberration, but also radiation pressure forces to have a non-radial component proportional to v/c. The last ratio is the deviation of light in transforming to a new reference system, and is a phenomenon known as the aberration of light. In that ratio, v is the orbital velocity of the Earth, and c the velocity of light in vacuum [6] . The gravity has no any force proportional to v/c. That means that the gravitational interactions apparently have no detectable aberration.
The gravitational interactions between bodies in all dynamic systems had always to be taken as instantaneous. This is unacceptable, due that, it was said before, it seemed to be a form of action at a distance through vacuum, without the mediation of anything. But mediation means propagation of something, and finite bodies should be incapable of travel faster than light speed, since that would require infinite energy.
The Einstein’s Special Theory of Relativity proved that no material body with a proper mass non-zero could propagate at speed greater than the velocity of light in vacuum. Then, when planetary orbits are determined, the astronomers use instantaneous interactions; they extract the position of some planet along its orbit at a time of interest, and calculate where that position would appear as seen from Earth by allowing for the finite propagation speed of light from there to here. It seems incongruous to allow for the finite propagation speed of light from the planet to Earth, but to take the effect of Earth’s gravity on the same planet as propagating from here to there, instantaneously [3] .
This kind of problems is not new. They have been discussed many times. Even today, the most frequently debated topic is centered in the question about the velocity of propagation of gravity.
2. Gravitational Interactions
Einstein’s Theory of Relativity suggests that gravitation, unlike electromagnetic forces, is a pure geometric effect of curved space-time, not a force of nature that propagates. In that case, the gravitational radiation, which surely does travel at light speed, but like a possible effect of the fifth order in v/c, is too small to play a role in explaining the difference in behavior between gravitational interactions and ordinary forces of nature. In this connection, in General Relativity, also exist problems with the causality principle, such as explaining how the external gravity fields between binary black holes [6] manage update without communication with the material bodies hidden behind event horizons [3] .
Many teachers and some books head off the question, assuring their students that the gravitational waves propagate at the speed of light, leaving the impression that the topic of gravitational propagation speed has already been solved [3] .
Anyone can verify the consequences of introducing a delay into gravitational interactions. The effect on the planetary orbits, is usually disastrous due to the fact that the conservation of angular momentum is destroyed [3] .
Indeed, it is widely accepted that the speed of gravity in Newton’s Law of Gravity is unconditionally infinite. This is not mentioned in the statement that General Relativity reduces to Newtonian Gravity in the low-velocity, weak-field limit, because of the obvious question it begs about how that can be true the propagation speed in one model is the velocity of light in vacuum, and in the other model it is infinite [3] . The same kind of question comes up in many other situations; that is to say, how can black holes have gravity when nothing can get out because escape velocity is greater than the speed of light?, and also, why do total eclipses of the Sun by the Moon reach the maximum about 40 seconds before the Sun and the Moon’s gravitational forces align? [3] . If gravity is a propagating force, the system Sun-Moon-Earth test implies that gravity propagates faster than light.
3. Escape Velocity
According to Einstein’s Special Theory of Relativity, no material body could possible travel with a velocity greater than the velocity of light in vacuum. Thus, it becomes obvious that none of them could be accelerated beyond the light barrier. This argument still stands. In its original paper, Einstein said that there is an upper limit of the velocity for the material bodies [7] . The only particle that always travels at that velocity is the photon. Thus, for a given distance, it can be written that

where x is any distance, c the velocity of light in vacuum, and tl the time that takes the photon to travel the given distance.
Let’s consider now that for the same distance x, it can be written the following relationship between the speed of gravity vg, and the time transit tt

Then, for a given distance the speed of gravity grows with the distance between the interacting bodies, whereas the transit time diminish, in such a way, that their product is always constant. In that case it is easy to see that

Now, it is necessary another independent equation in order to calculate vg. That relationship is the escape velocity; which is the medium used by the gravity to transmit its effects like a force of nature that propagates. By its definition, it has that

where G is the Gravitational Constant, M the mass of the object which is the source of gravitational interaction, and R its radius. Hence, the value of vo depends on the mass of the gravity source, and critically on its radius.
Let’s consider the following situation. A distant galaxy, per example, as appear seen from Earth, looks like a light point. In that apparent very small region of space, it is concentered the total mass of that galaxy. That fact is very important because the apparent size of heavenly bodies plays a special role in the gravitational interactions. This is so because of a some kind of effect over the size due to the very big distances in space. So that, when a couple of celestial bodies interact each other, they can be considered as sources of gravitational force, because there are certain degrees of reciprocity in that situation. So that, their escape velocities takes values that depends critically on its apparent size. Hence, in Equation (44) is has that

where 

In gravitational interactions, the question of what means by distance between the heavenly bodies arises immediately; because every of their particles can be considered as a contributing to the gravity attraction. But, and according to I. Newton, the distance and direction over each body, are different from each particle [4] . Then, it is possible make the assumption that the interacting bodies could be treated as if all their masses where concentrated at its apparent sizes. However, and by definition, a geometrical point is an object that has no dimension; so that it is not a good idea to take the size of a very far object as zero; in such a way that it is necessary to take another approach. Suppose that as the limit in size of the massive source of the gravitational force is taken as the size of the atom, or the atomic nucleus.
Thus, the value of 

4. The Total Solar Eclipse
This is an example of the difference in behavior between the velocity of the gravitational interactions and the velocity of light in vacuum.
The Moon and Earth are relatively nearby and sharing the Earth orbital motion around the Sun. It is well known that it has an aberration of just 20 arc seconds. It takes to the Moon about 38 seconds of time to move 20 arc seconds on the sky relative to the Sun [3] . Since the total solar eclipse agree with predicted times, to within a couple of seconds, it can be used the orbits of the Sun and the Moon near times of maximum total solar eclipse to compare the time of predicted gravitational maximum with the time of visible maximum eclipse. Thus, the maximum gravitational perturbation by the Sun on the orbit of the Moon near eclipse may be taken as the time when the Moon and Sun sizes are equal. In those conditions, the maximum eclipse occurs roughly 38.19 seconds of time before the time of gravity maximum [3] .
Hence, let tt = 38.19 seconds be, the transit time that takes the gravitational interaction to travel the distance between the Sun and the system Moon-Earth. Given that the light from the Sun requires 4.98 ´ 102 sec. to reach the system before mentioned, from Equation (43) it is easy to obtain that

that is to say,

Now, from the relationship (45), and using the following data

where the symbol 

It is proposed that this picture can be used to explain the behavior of any couple of interacting celestial objects; where one of them is the source of gravitational force that propagate with an escape velocity which depends on the apparent size of that body.
In conclusion, the Sun’s field cannot be treated as static and unchanging, even in the planetary system, because it propagates as a force of nature, with an escape velocity whose value depends on the Sun’s size, as seen from any planet of the Solar System. Thus, its gravity field is continuously updated, but with very little gravitational aberration, difficult to determine.
5. Conclusions
The small deformation strain tensor can be used as a fundamental metric tensor instead the usual fundamental metric tensor, in order to formulate a Dynamic Theory of Gravitation, in which the escape velocity is the greatest velocity of Nature [1] [2] .
For the Sun and Earth, or for any other planet of the Solar System and also for any other celestial body, the picture before given has certain degree of reciprocity. In other words, seen from the Sun any one planet or another body has an apparent size than generating its own appropriate apparent escape velocity. However, in the case of the planets, all of them have a very small size compared with Sun’s size, and also the mass of any of them is very small as compared with Sun’s mass.
Then, its apparent escape velocity is orders of magnitude smaller than the apparent escape velocity of the Sun. Hence, when the escape velocity of the Sun, in some point of the space, meets with the escape velocity of any planet, they pull each other; because they are the carriers of the respective gravitational fields.
Given that those gravitational forces are attractive, that dynamic process is the responsible for the strong link which is established between the Sun and every of the planets and other bodies of the Solar System. It is proposed that this argument is valid for any couple of objects in the Universe. Then, it can be said that the gravitational interactions exchanging coin are the escape velocity due to the apparent size of the celestial bodies.
Due to the finite value of the velocity of the gravitational interactions, the gravity force has a propagation delay for its action too. That means that it has a little aberration which, in despite of it smallness, has a minute non-radial component capable to accelerate the planets along its orbital motions. That effect is accumulative, in such a way, that along of thousand million years will tend to increase the angular momentum of the system, and will soon cause an appreciable change of period.
Maybe the minute, but accumulative effect of the non-radial component of the gravitational force, due to the gravity aberration, is the dynamic agent which produces the slow rotational motion of the galaxies.
Finally, given that the velocity of the gravitational interactions is in general terms, greater than the velocity of light in vacuum; and taken into account the fact that according to Einstein’s Special Theory of Relativity, no material particles or bodies can possible travel with a velocity greater than the light speed; the gravity is strong enough to slow down the Universal Expansion [5] . This argument points out toward a closed Universe Model.
Cite this paper
Angel Fierros Palacios, (2016) Gravitation. Open Access Library Journal,03,1-12. doi: 10.4236/oalib.1102141
References
- 1. Fierros, A. (2006) The Hamilton-Type Principle in Fluid Dynamics. Fundamentals and Applications to Magnetohydrodynamics, Thermodynamics, and Astrophysics. Springer-Verlag, Wien.
- 2. Fierros, A. (2015) The Small Deformation Strain Tensor as a Fundamental Metric Tensor. Journal High Energy Physics, Gravitation and Cosmology.
- 3. Van Flandern, T. (1998) The Speed of Gravity—What the Experiments Say. Physics Letters, 250, 1-11.
http://dx.doi.org/10.1016/S0375-9601(98)00650-1 - 4. Resnick, R. and Halliday, D. (1966) Physics. John Wiley and Sons, Inc., New York.
- 5. Eddington, A.S. (1923) The Mathematical Theory of Relativity. Chelsea Publishing Company, New York.
- 6. Londau, L.D. and Lifshitz, E.M. (1962) The Classic Theory of Fields. Addison-Wesley Publishing Company Inc.
- 7. Shipman, H.I. (1976) Black Holes, Quasars, and the Universe. Houghton Mifflin Company, Boston.
- 8. Einstein, A. (1923) The Principle of Relativity. Dover Publications Inc.
Appendix
The condition for flat space-time: A formal proof
It was shown that when the components of the small deformation strain tensor umu are constants, ds2 can be reduced to the sum of four squares, and a Galilean coordinates can be constructed. Thus, an equivalent definition of flat space-time is that it is such that coordinates can be found for which the umu are constants, and a region of the space is called flat if it is possible to construct in it a Galilean frame of reference.
If the µmu are constants the 3-index Christoffel simbols, all vanishes; but since they do not form a tensor, will not continue to vanish when other coordinates are substituted in the same flat region. Then, in such a condition, the Reimann-Christoffel Tensor, being composed of products and derivatives of the 3-index simbols, will vanish. Since it is a tensor, it will continue vanish when other coordinate system is used in the same region. Hence, the vanishing of that tensor is a necessary condition for flat space-time. But, that condition is also sufficient; in such a way that if that tensor is equal to zero, the space-time must be flat [2] [5] . Then, when

it is possible to construct a uniform vector-field by means of parallel displacements of a vector over the region.
Let 

or by the following equation [2]

These four uniform vector-fields can be used in order to define a new coordinate system distinguishes by accents. The unit mesh is a geometrical figure contained by the four vector at any point, so that the complete system will be formed by successive parallel displacements of the unit mesh until the whole region is filled [5] . One edge of it, given in the old coordinates by

has to become in the new coordinate system by

Similarly, the second edge
must become

Then, this requires the following law of transformation

Clearly, the new coordinate system depends on the possibility of constructing uniform vector-fields, and this depends on the relationship (A.1).
Since ds2 is an invariant, it is easy to see that
by the Equation (A.5). Hence

So that, by differentiation of (A.6) it has that

by the Equation (A.2). Now, by changing dummy suffixes, this become

by the following relationship [2] [5]

Hence, the components of the small deformation strain tensor 
stants throughout the region considered. We have thus constructed a coordinate-system fulfilling the condition that the u’s are constant, and it follows that the space-time is flat. [2] [5] .
NOTES
1The derivation process of the Equation (19) is not the main objective of the study. However, in reference [2] , that derivation process is given in my paper title The small deformation strain tensor as a fundamental metric tensor published by the Journal of High Energy Physics, Gravitation and Cosmologyin 28 July 2015.





