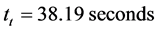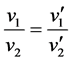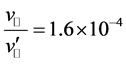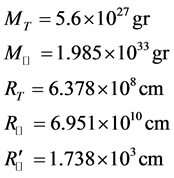Open Access Library Journal
Vol.03 No.03(2016), Article ID:69112,4 pages
10.4236/oalib.1102412
The Reciprocity Principle in Gravitational Interactions
Angel Fierros Palacios
Instituto de Investigaciones Eléctricas, División de Energías Alternas, Cuernavaca, México

Copyright © 2016 by author and OALib.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


Received 4 March 2016; accepted 20 March 2016; published 24 March 2016

ABSTRACT
In this paper it is proposed that the ratio between the apparent radius of any two celestial bodies separated by a very big distance, as seemed each other at a distance, is numerically equal to the ratio of the respective real values of their radius. That relationship is what is called in the paper the Reciprocity Principle. In other words, the apparent size of heavenly bodies, as seemed at a distance, plays a special role in the gravitational interactions. This is so because of some kind of effect over the size due to the very big distances in space. When a couple of celestial bodies interact each other, both of them can be considered as sources of the gravity attraction. In that situation, each body acts upon the other in such a way that it can be considered that there are some degree of reciprocal interaction. In this paper, a formal definition of that reciprocal effect is given.
Keywords:
Escape Velocity, Apparent Size, Reciprocity Principle
Subject Areas: Classical Mechanics

1. Introduction
Let us consider two heavenly bodies having masses m1 and m2, and radius r1 and r2, respectively, separated by a certain distance, and in gravitational attraction acting along the line joining them. In that situation, the values of their escape velocities due to its apparent size are dependent on their masses, and critically on their apparent radius [1] . When those celestial bodies interact each other, both of them must be considered as sources of gravity force. Then, along that dynamical process, as it was said before, there exist some degree of reciprocity [1] . By definition of escape velocity it has that [2]
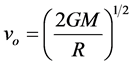 (1)
(1)
where G is the Gravitational Constant, M the mass of the source of the gravity force, and R its radius [1] .
2. The Reciprocity Principle
When the heavenly bodies before mentioned, are in gravitational interaction, it is easy to see that from Equation (1) the following relationship can be obtained
 (2)
(2)
where the 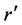 are the apparent radius of the bodies.
are the apparent radius of the bodies.
Let’s consider one of the bodies as the source of the gravity attraction; and be that body the one marked with the index 2. Its mass is m2, and its apparent radius is . So that, the equation (1) takes the following form [1] .
. So that, the equation (1) takes the following form [1] .
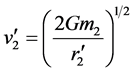 (3)
(3)
In order to obtain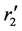 , and also
, and also 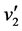 it can be use the following procedure. It is well known from Optics that the ratio of the image size q, to the object size p is the Magnification A, that is to say
it can be use the following procedure. It is well known from Optics that the ratio of the image size q, to the object size p is the Magnification A, that is to say
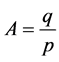 (4)
(4)
To obtain the apparent size of that body, it can be used some optical astronomical instrument that has a magnification A; in order to get the apparent image, and also the apparent size, given by the following formula
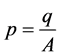 (5)
(5)
Given that A is a number, 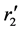 is equal to p/2. To calculate
is equal to p/2. To calculate 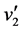 is enough to introduce into Equation (2) the result obtained. However, in that equation it has the unknown quantity
is enough to introduce into Equation (2) the result obtained. However, in that equation it has the unknown quantity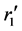 . In order to known its value, it is pro- posed that:
. In order to known its value, it is pro- posed that:
The ratio between the apparent radius 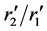 keep the same proportion among them as the ratio of the respectives real values of their radius; that is to say
keep the same proportion among them as the ratio of the respectives real values of their radius; that is to say
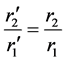 (6)
(6)
where r1 and r2 are the values of the real radius. The proposition before given is the Reciprocity Principle.
3. The Sun-Earth System
As an illustrative example, let’s consider the case of the gravitational interaction between the Sun and our planet. Therefore, Equation (2) becomes
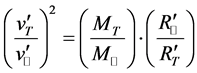 (7)
(7)
Then, it has that
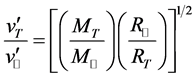
according to relationship (6).
Taking into account the values that appears in the Appendix, it is obtained that
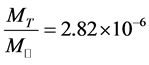
and
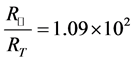
in such a way that in Equation (8) it has that
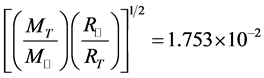
Substituting this result into Equation (8) the following value for the Earth’s escape velocity due to its apparent size is obtained; that is to say
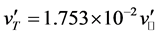
where 
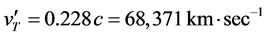
That result means that, due to the smallness of the Earth in comparison to the Sun, its escape velocity 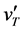
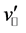
Finally, from Equation (10) it is easy to obtain that
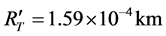
Thus,
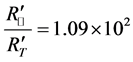
But, this is the same numerical value given in (10).
4. The Point of Meet
It is clear that in the meantime the escape velocity 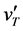
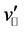
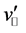
In that time 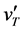

in the meantime 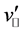
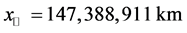
Those results indicate that the point of meet occurs near the Earth
5. Conclusions
It is proposed that in Gravitational Interactions, the Reciprocity Principle is valid for any couple of heavenly bodies that interact each other. Also, it is a useful concept, and a powerful tool to calculate the escape velocities and radius due to the apparent size of each body. In other words, its validity can be extended to the whole Universe. On the other hand, it is possible to prove that the ratios 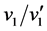

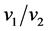
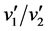
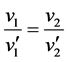
where the prime refers the escape velocity of these bodies due to their apparent size.
In the case of the Sun-Earth System, it has that
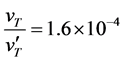
and also,
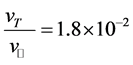
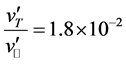
Cite this paper
Angel Fierros Palacios, (2016) The Reciprocity Principle in Gravitational Interactions. Open Access Library Journal,03,1-4. doi: 10.4236/oalib.1102412
References
Appendix